exampie Show that the points collinear Solution Direction
Last updated: 9/17/2023
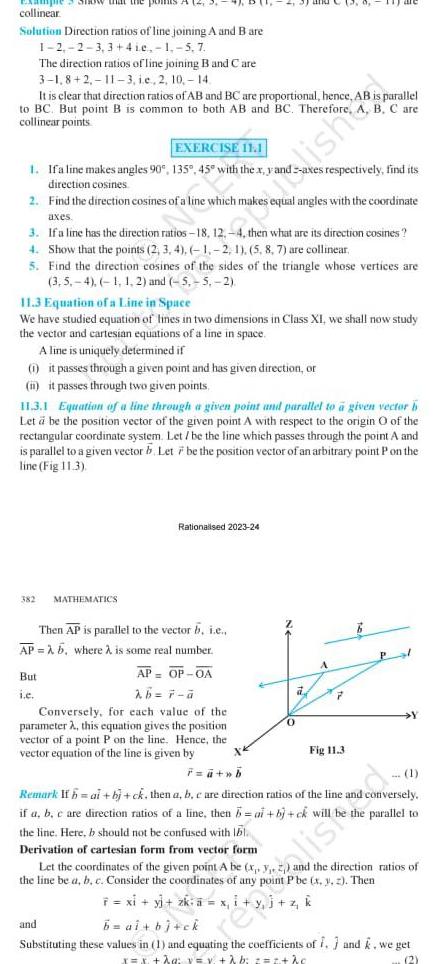
exampie Show that the points collinear Solution Direction ratios of line joining A and B are 1 2 2 3 3 4ie 1 5 7 1 It is clear that direction ratios of AB and BC are proportional hence AB is parallel to BC But point B is common to both AB and BC Therefore A B C are collinear points The direction ratios of line joining B and Care 3 1 8 2 11 3 ie 2 10 14 EXERCISE 11 1 1 If a line makes angles 90 135 45 with the x ya direction cosines 2 Find the direction cosines of a line which makes equal angles with the coordinate axes 3 If a line has the direction ratios 12 4 then what are its direction cosines 4 Show that the points 2 3 4 1 2 1 5 8 7 are collinear 5 Find the direction cosines of the sides of the triangle whose vertices are 3 5 4 1 1 2 and 5 5 2 11 3 Equation of a Line in Space We have studied equation of lines in two dimensions in Class XI we shall now study the vector and cartesian equations of a line in space A line is uniquely determined if 1 it passes through a given point and has given direction or n it passes through two given points 382 11 3 1 Equation of a line through a given point and parallel to a given vector b Let be the position vector of the given point A with respect to the origin O of the rectangular coordinate system Let be the line which passes through the point A and is parallel to a given vector b Let be the position vector of an arbitrary point P on the line Fig 11 3 wish But 1 0 MATHEMATICS Then AP is parallel to the vector b i e APA b where is some real number AP OP DA b 7 Conversely for each value of the parameter A this equation gives the position vector of a point P on the line Hence the vector equation of the line is given by X Rationalised 2023 24 s respectively find its and a 2 Fig 11 3 Y F a b Remark If hai b ck then a b c are direction ratios of the line and conversely if a b c are direction ratios of a line then b al by ck will be the parallel to the line Here b should not be confused with lb Derivation of cartesian form from vector form Let the coordinates of the given point A be x y z and the direction ratios of the line be a b c Consider the coordinates of any point P be x y z Then x t v t thit xitritzk b ai bj ck Substituting these values in 1 and equating the coefficients of i and k we get x x g v v b 2 2 kc 2