2 pts Let fR R be differentiable We say that f is convex if

Last updated: 2/11/2023

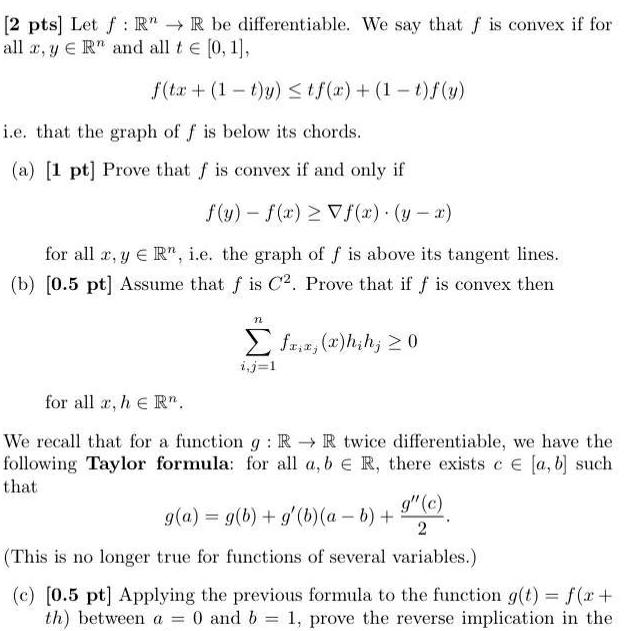
2 pts Let fR R be differentiable We say that f is convex if for all x y R and all t 0 1 f tx 1 t y tf x 1 t f y i e that the graph of f is below its chords a 1 pt Prove that f is convex if and only if f y f x Vf x y x for all r y R i e the graph of f is above its tangent lines b 0 5 pt Assume that f is C Prove that if f is convex then n fax x h h 20 i j 1 for all x h ER We recall that for a function g R R twice differentiable we have the following Taylor formula for all a b E R there exists c a b such that g c 2 This is no longer true for functions of several variables c 0 5 pt Applying the previous formula to the function g t f x th between a 0 and b 1 prove the reverse implication in the g a g b g b a b