Question:
Consider the following function. f(x) = cos (8x 9) Find the
Last updated: 7/20/2022
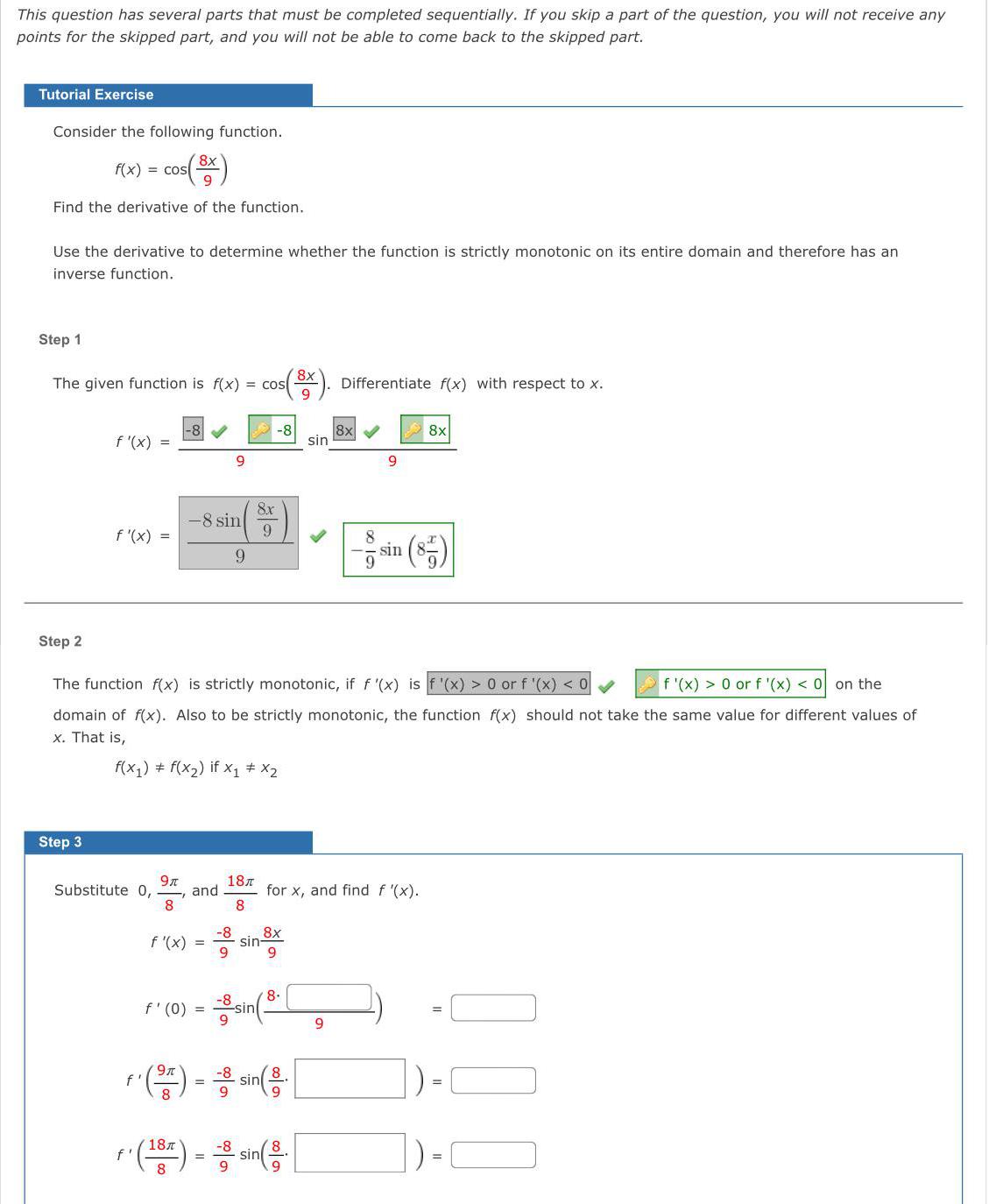
Consider the following function. f(x) = cos (8x 9) Find the derivative of the function. Use the derivative to determine whether the function is strictly monotonic on its entire domain and therefore has an inverse function. The given function is f(x) = cos(8x 9) Differentiate f(x) with respect to x. The function f(x) is strictly monotonic, if f '(x) is f '(x) > 0 or f '(x) < 0✔ f'(x) > 0 or f '(x) < 0 on the domain of f(x). Also to be strictly monotonic, the function f(x) should not take the same value for different values of x. That is, f(x₁) = f(x₂) if X₁ ≠ X2