Given the function g(x) = 4x³ – 24x² + 36x, find the first

Last updated: 8/11/2022

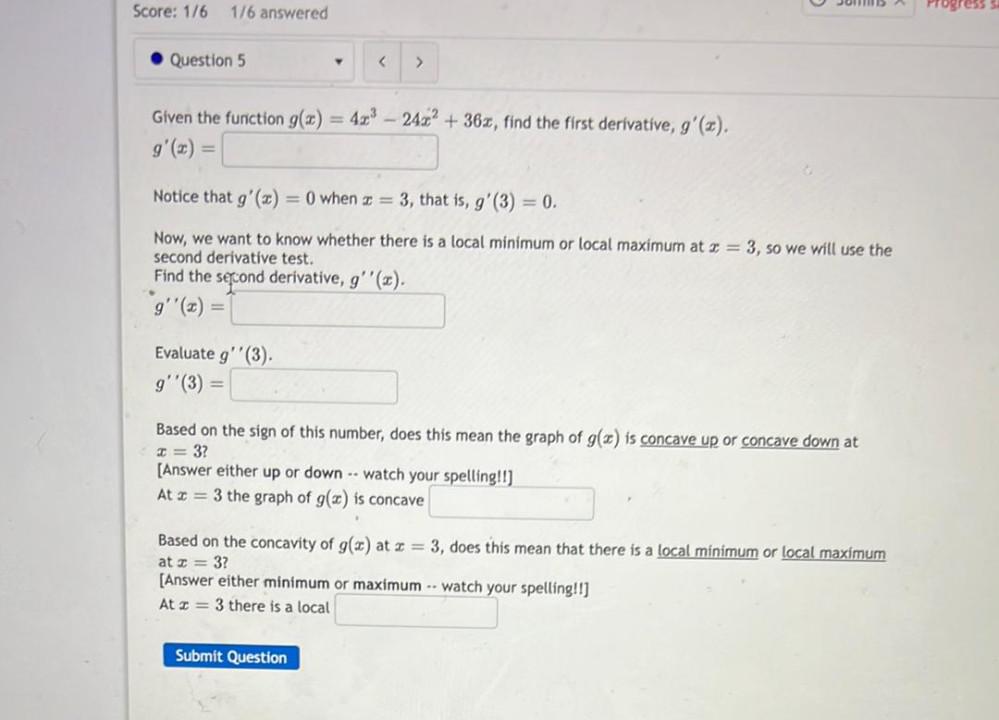
Given the function g(x) = 4x³ – 24x² + 36x, find the first derivative, g'(c). Notice that g'(x) = 0 when I = 3, that is, g'(3) = 0. Now, we want to know whether there is a local minimum or local maximum at I = 3, so we will use the second derivative test. Find the second derivative, g''(x). g''(x) Evaluate g''(3) g''(3) Based on the sign of this number, does this mean the graph of g() is concave up or concave down at = 3? [Answer either up or down -- watch your spelling!!] At = 3 the graph of g() is concave Based on the concavity of g(x) at I = 3, does this mean that there is a local minimum or local maximum at I = 3?