Is the set of negative integers a commutative group under

Last updated: 7/25/2022

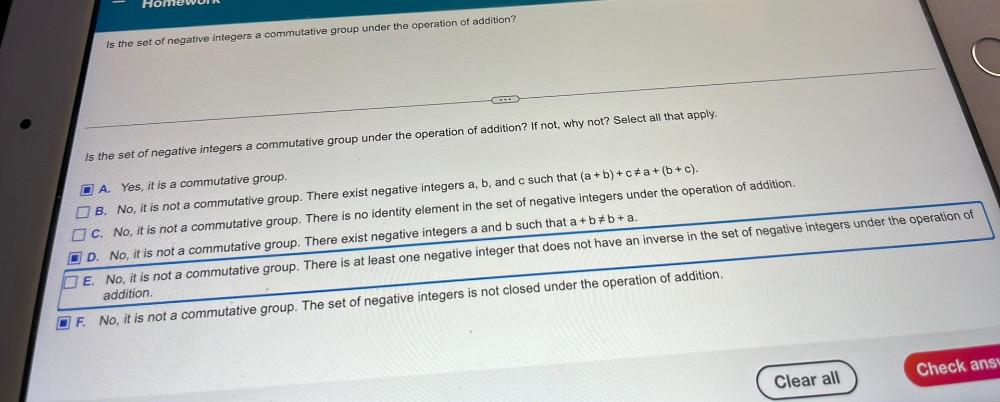
Is the set of negative integers a commutative group under the operation of addition? Is the set of negative integers a commutative group under the operation of addition? If not, why not? Select all that apply. A. Yes, it is a commutative group. B. No, it is not a commutative group. There exist negative integers a, b, and c such that (a + b) + c≠ a + (b + c). C. No, it is not a commutative group. There is no identity element in the set of negative integers under the operation of addition. D. No, it is not a commutative group. There exist negative integers a and b such that a+b≠b+a. E. No, it is not a commutative group. There is at least one negative integer that does not have an inverse in the set of negative integers under the operation of addition F. No, it is not a commutative group. The set of negative integers is not closed under the operation of addition.