Let A, B be (n x n)-matrices. Suppose AB = BA = In. (a) Let X, C be n × m matrices. Show that AX = C if and only if X = BC. (b)

Question

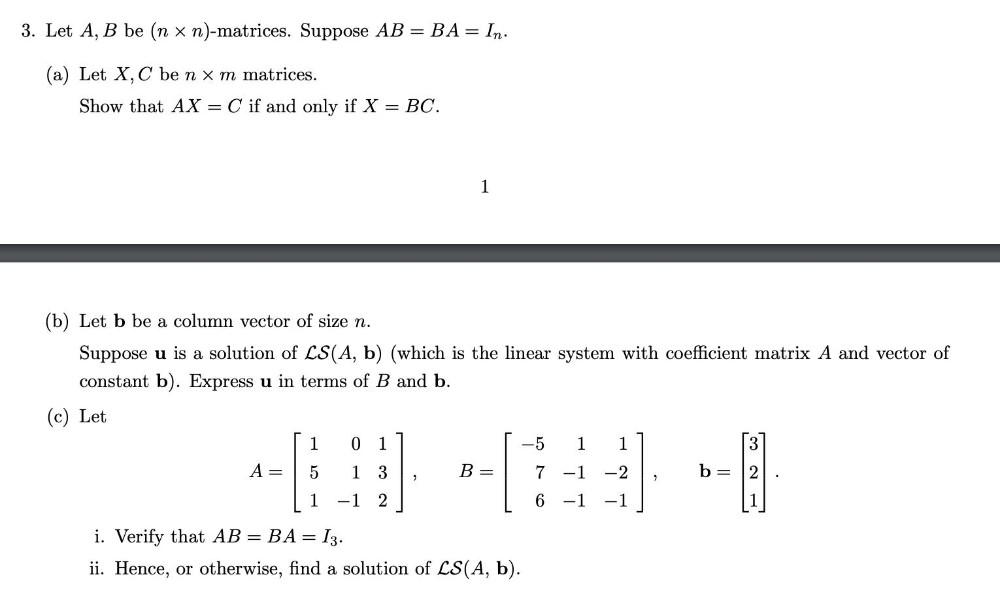
Let A, B be (n x n)-matrices. Suppose AB = BA = In.
(a) Let X, C be n × m matrices.
Show that AX = C if and only if X = BC.
(b) Let b be a column vector of size n.
Suppose u is a solution of LS(A, b) (which is the linear system with coefficient matrix A and vector of constant b). Express u in terms of B and b.
(c) Let       1 0 1                -5     1   1           3           
       a=       5 1 3        B=   7   -1  -2     b=   2
                  1 -1 2                6    -1   -1        1

I). Verify that AB = BA= I₃	.
ii). Hence, or otherwise, find a solution of LS(A, b).
"
=

Kunduz · Accepted Answer

Click to see the answer