Question:
Let F be the set of functions of the form f(x) = A sin(x) +
Last updated: 8/11/2022
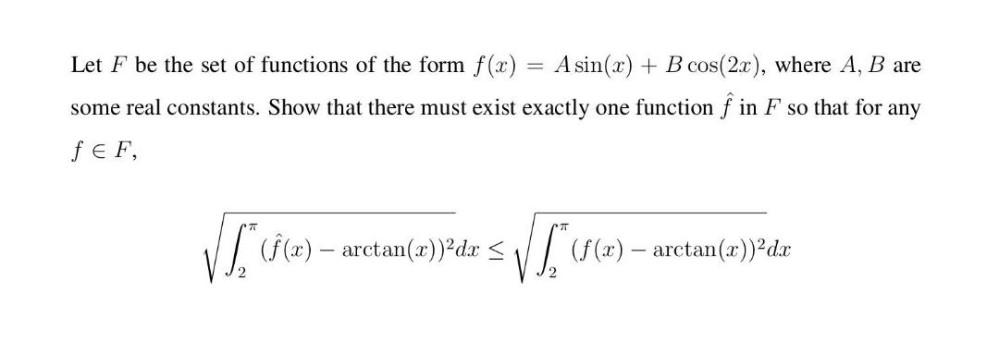
Let F be the set of functions of the form f(x) = A sin(x) + B cos(2x), where A, B are some real constants. Show that there must exist exactly one function f in F so that for any f∈F √∫(f(x)- arctan(x))²dx≤ √∫(f(x)- arctan(x))²dx