Let P be any point on the curve S = 0 such that tangents

Last updated: 7/7/2022

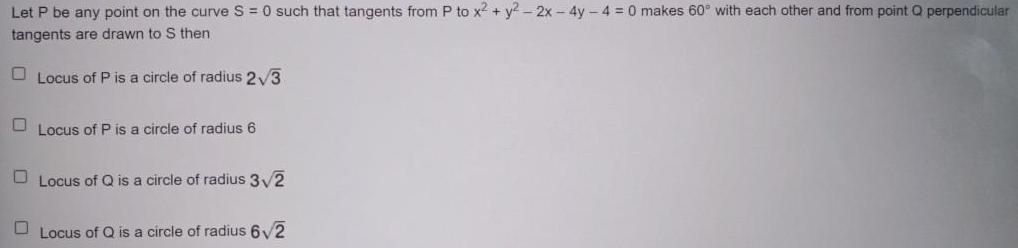
Let P be any point on the curve S = 0 such that tangents from P to x² + y2 - 2x - 4y -4 = 0 makes 60° with each other and from point Q perpendicular tangents are drawn to S then Locus of P is a circle of radius 2√3 Locus of P is a circle of radius 6 Locus of Q is a circle of radius 3√2 Locus of Q is a circle of radius 6√2