Recently the bowling scores of a certain bowler were
Last updated: 6/23/2023
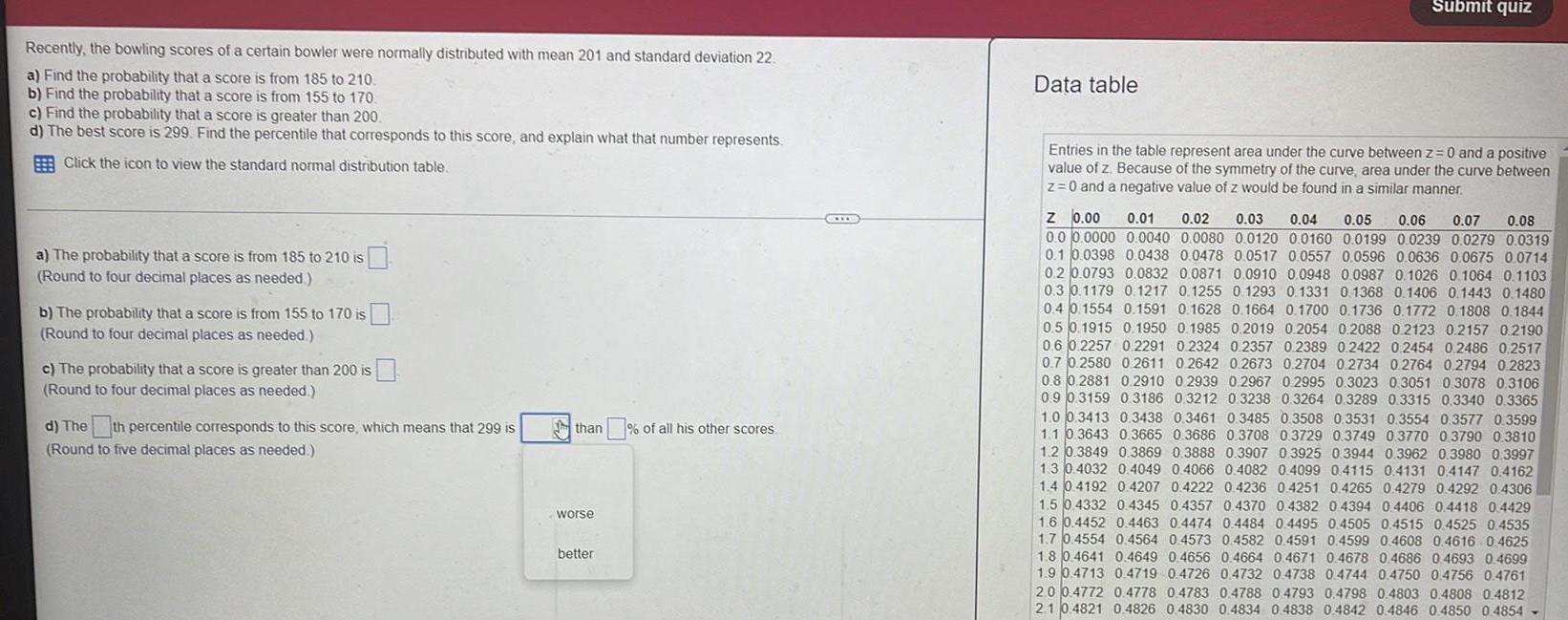
Recently the bowling scores of a certain bowler were normally distributed with mean 201 and standard deviation 22 a Find the probability that a score is from 185 to 210 b Find the probability that a score is from 155 to 170 c Find the probability that a score is greater than 200 d The best score is 299 Find the percentile that corresponds to this score and explain what that number represents Click the icon to view the standard normal distribution table a The probability that a score is from 185 to 210 is Round to four decimal places as needed b The probability that a score is from 155 to 170 is Round to four decimal places as needed c The probability that a score is greater than 200 is Round to four decimal places as needed d Theth percentile corresponds to this score which means that 299 is Round to five decimal places as needed than of all his other scores worse better Data table Submit quiz Entries in the table represent area under the curve between z 0 and a positive value of z Because of the symmetry of the curve area under the curve between z 0 and a negative value of z would be found in a similar manner Z 0 00 0 01 0 02 0 03 0 04 0 05 0 06 0 07 0 08 0 0 0 0000 0 0040 0 0080 0 0120 0 0160 0 0199 0 0239 0 0279 0 0319 0 1 0 0398 0 0438 0 0478 0 0517 0 0557 0 0596 0 0636 0 0675 0 0714 0 2 0 0793 0 0832 0 0871 0 0910 0 0948 0 0987 0 1026 0 1064 0 1103 0 3 0 1179 0 1217 0 1255 0 1293 0 1331 0 1368 0 1406 0 1443 0 1480 0 4 0 1554 0 1591 0 1628 0 1664 0 1700 0 1736 0 1772 0 1808 0 1844 0 5 0 1915 0 1950 0 1985 0 2019 0 2054 0 2088 0 2123 0 2157 0 2190 0 6 0 2257 0 2291 0 2324 0 2357 0 2389 0 2422 0 2454 0 2486 0 2517 0 7 0 2580 0 2611 0 2642 0 2673 0 2704 0 2734 0 2764 0 2794 0 2823 0 8 0 2881 0 2910 0 2939 0 2967 0 2995 0 3023 0 3051 0 3078 0 3106 0 9 0 3159 0 3186 0 3212 0 3238 0 3264 0 3289 0 3315 0 3340 0 3365 1 0 0 3413 0 3438 0 3461 0 3485 0 3508 0 3531 0 3554 0 3577 0 3599 1 1 0 3643 0 3665 0 3686 0 3708 0 3729 0 3749 0 3770 0 3790 0 3810 1 2 0 3849 0 3869 0 3888 0 3907 0 3925 0 3944 0 3962 0 3980 0 3997 1 3 0 4032 0 4049 0 4066 0 4082 0 4099 0 4115 0 4131 0 4147 0 4162 1 4 0 4192 0 4207 0 4222 0 4236 0 4251 0 4265 0 4279 0 4292 0 4306 1 5 0 4332 0 4345 0 4357 0 4370 0 4382 0 4394 0 4406 0 4418 0 4429 1 6 0 4452 0 4463 0 4474 0 4484 0 4495 0 4505 0 4515 0 4525 0 4535 1 7 0 4554 0 4564 0 4573 0 4582 0 4591 0 4599 0 4608 0 4616 0 4625 1 8 0 4641 0 4649 0 4656 0 4664 0 4671 0 4678 0 4686 0 4693 0 4699 1 9 0 4713 0 4719 0 4726 0 4732 0 4738 0 4744 0 4750 0 4756 0 4761 2 0 0 4772 0 4778 0 4783 0 4788 0 4793 0 4798 0 4803 0 4808 0 4812 2 1 0 4821 0 4826 0 4830 0 4834 0 4838 0 4842 0 4846 0 4850 0 4854