The campus of a college has plans to construct a rectangular

Last updated: 10/29/2023

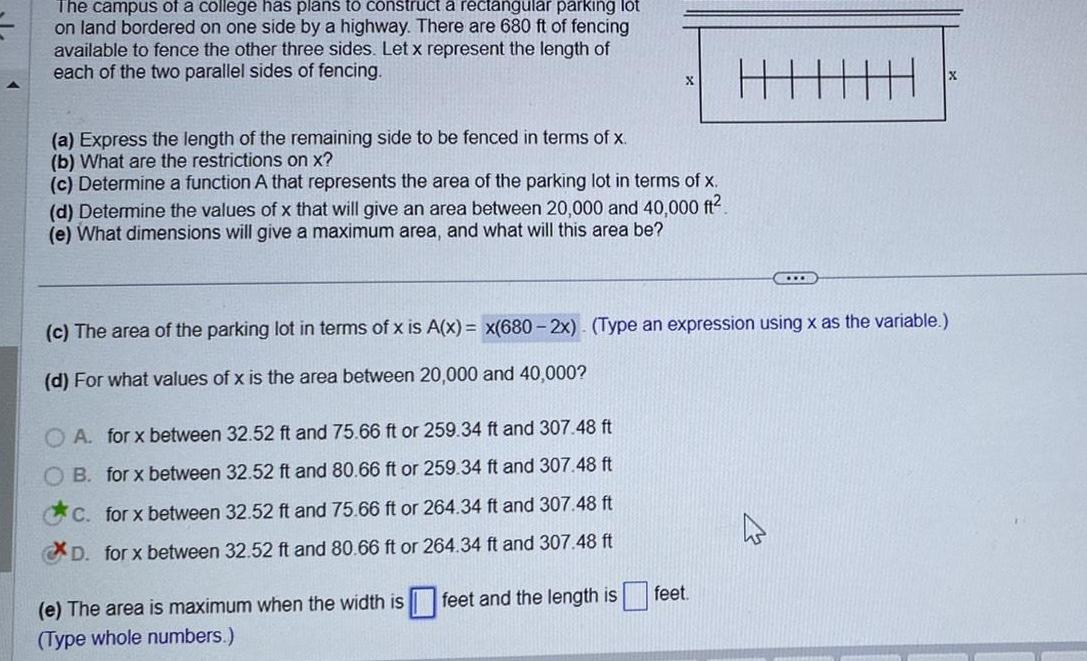
The campus of a college has plans to construct a rectangular parking lot on land bordered on one side by a highway There are 680 ft of fencing available to fence the other three sides Let x represent the length of each of the two parallel sides of fencing a Express the length of the remaining side to be fenced in terms of x b What are the restrictions on x c Determine a function A that represents the area of the parking lot in terms of x d Determine the values of x that will give an area between 20 000 and 40 000 ft e What dimensions will give a maximum area and what will this area be c The area of the parking lot in terms of x is A x x 680 2x Type an expression using x as the variable d For what values of x is the area between 20 000 and 40 000 O A for x between 32 52 ft and 75 66 ft or 259 34 ft and 307 48 ft B for x between 32 52 ft and 80 66 ft or 259 34 ft and 307 48 ft C for x between 32 52 ft and 75 66 ft or 264 34 ft and 307 48 ft D for x between 32 52 ft and 80 66 ft or 264 34 ft and 307 48 ft e The area is maximum when the width is feet and the length is Type whole numbers H feet