Using the Third Corollary to the Inscribed Angle Theorem The

Last updated: 5/1/2023

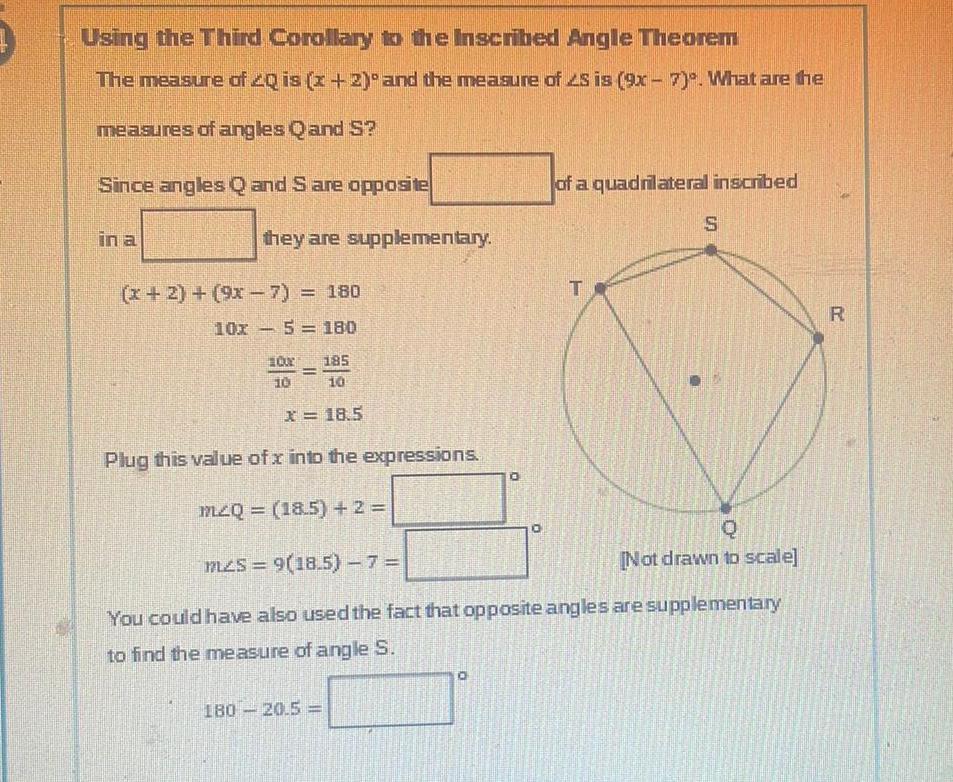
Using the Third Corollary to the Inscribed Angle Theorem The measure of 20 is x 2 and the measure of 2S is 9x 7 What are the measures of angles Q and S Since angles Q and S are opposite in a they are supplementary x 2 9x 7 180 10x5 180 Our 185 10 10 x 18 5 Plug this value of x into the expressions m20 18 5 2 180 20 5 0 0 of a quadrilateral inscribed T S m s 9 18 5 7 You could have also used the fact that opposite angles are supplementary to find the measure of angle S Q Not drawn to scale R