x 1 0 where p is the vapour pressure of pure component I at the same Similarly for component 2 P P x 11 where p represents the

Question

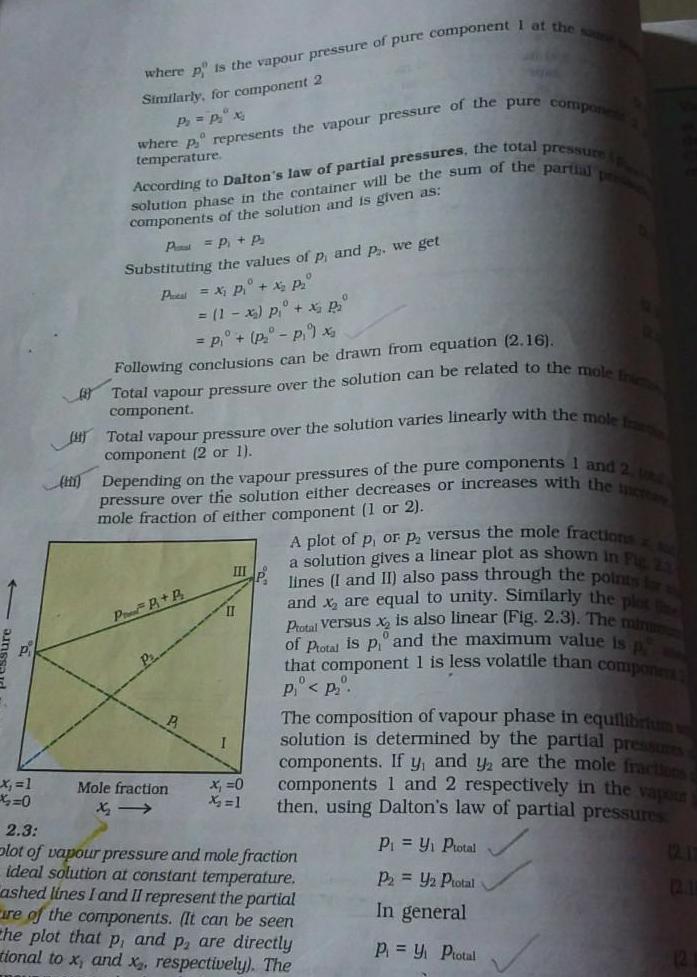
x 1 0 where p is the vapour pressure of pure component I at the same Similarly for component 2 P P x 11 where p represents the vapour pressure of the pure compone temperature solution phase in the container will be the sum of the partial pre According to Dalton s law of partial pressures the total pressure components of the solution and is given as P P P Substituting the values of p and p we get Pa X P X P 1 x p x P P P 9 x Following conclusions can be drawn from equation 2 16 Total vapour pressure over the solution can be related to the mole Ini component Total vapour pressure over the solution varies linearly with the mole fran component 2 or 1 H Depending on the vapour pressures of the pure components 1 and 2 U pressure over the solution either decreases or increases with the increas mole fraction of either component 1 or 2 a solution gives a linear plot as shown in Fig 2 A plot of p or p versus the mole fractions lines I and II also pass through the points for s and x are equal to unity Similarly the plot Ptotal versus x is also linear Fig 2 3 The minu of Ptotal is p and the maximum value is that component 1 is less volatile than compone P 20 PP B P P Mole fraction III II x 0 X 1 P P The composition of vapour phase in equilibrium solution is determined by the partial pressures components If y and y are the mole fractions components 1 and 2 respectively in the vapour then using Dalton s law of partial pressures P Y Ptotal P2 Y2 Ptotal In general P Y Ptotal 2 3 plot of vapour pressure and mole fraction ideal solution at constant temperature ashed lines I and II represent the partial are of the components It can be seen the plot that p and p are directly tional to x and x respectively The 12

Kunduz · Accepted Answer

Click to see the answer