Geometry Questions
The best high school and college tutors are just a click away, 24×7! Pick a subject, ask a question, and get a detailed, handwritten solution personalized for you in minutes. We cover Math, Physics, Chemistry & Biology.
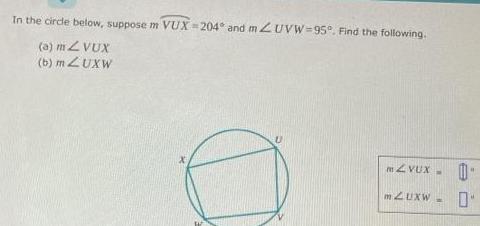
Geometry
Coordinate systemIn the circle below suppose m VUX 204 and mZUVW 95 Find the following a mZVUX b m ZUXW ZVUX 0 ZUXW
Geometry
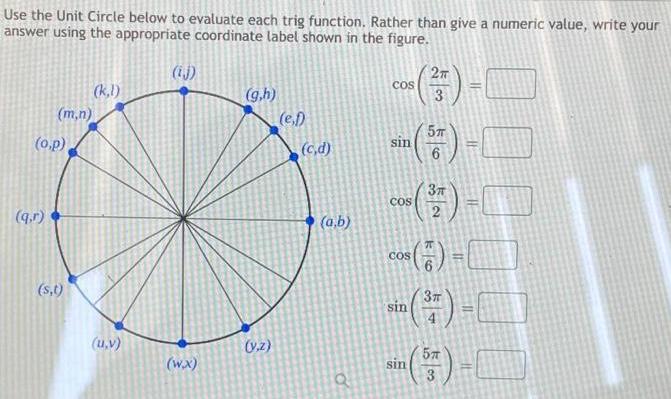
Solution of trianglesUse the Unit Circle below to evaluate each trig function Rather than give a numeric value write your answer using the appropriate coordinate label shown in the figure m n o p q r s t kl u v ij w x g h v z e f c d a b o COS sin 3 3T COG 3 sin 5T 6 COS 7 3 4 sin 5T 3

Geometry
2D Geometry1 A 6 10 B e D E A B C do rusal 3 DE AE AB 6 cm BC 10 cm Yukar daki verilere g re AE ka cm dir A 6 2 B 6 3 C 9 2 D 12 E 12 2
Geometry
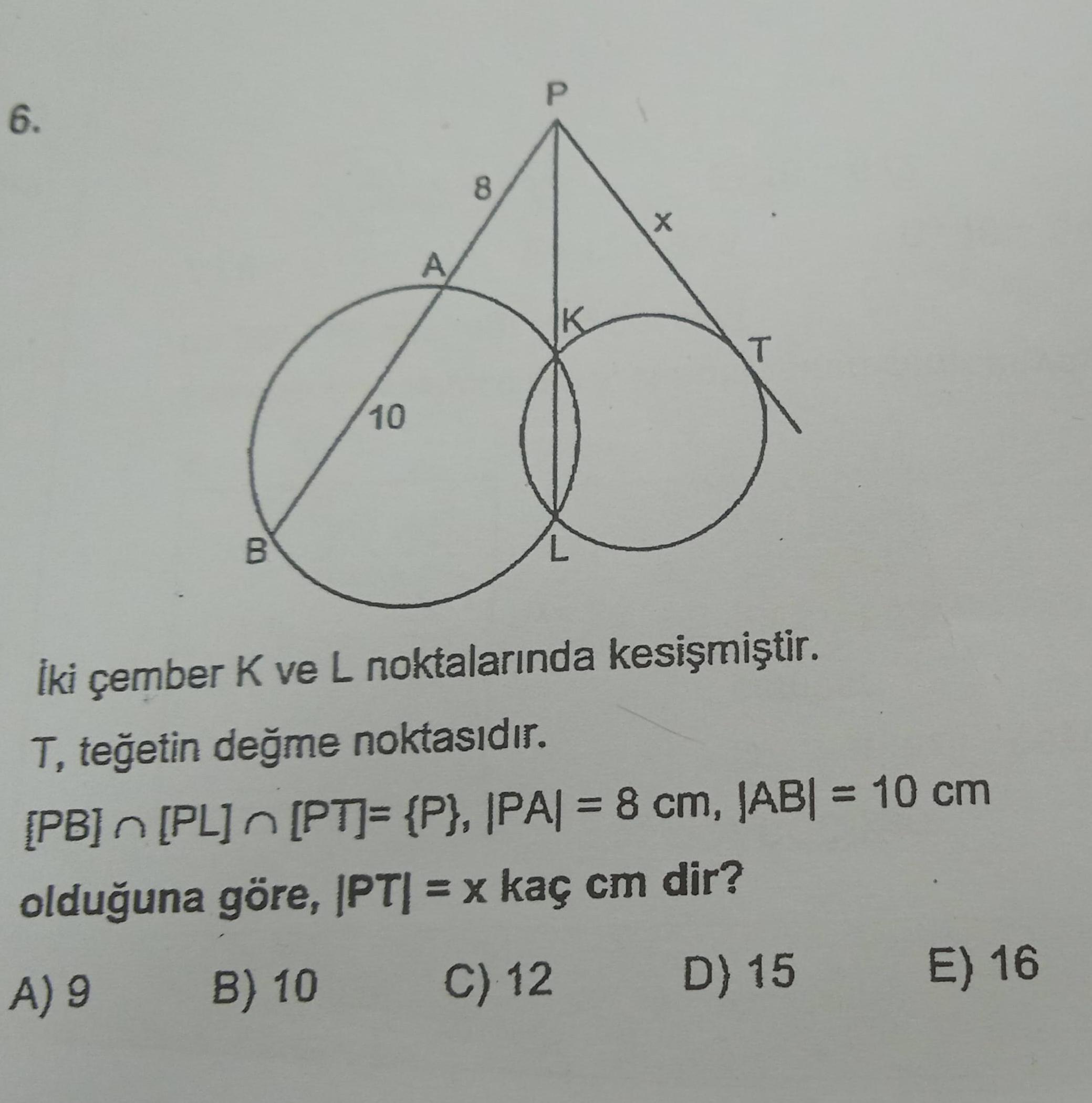
Area6 B 10 A 8 X T ki ember K ve L noktalar nda kesi mi tir T te etin de me noktas d r PB PL PT P PA 8 cm AB 10 cm oldu una g re PT x ka cm dir A 9 B 10 C 12 D 15 E 16

Geometry
Coordinate systemWhich of these is an equation of one of the asymptotes of the function f x 7 sec X x X X x 32 8 2 1 2 4 sec 1 2 xXx 1 x 4 None of the above


Geometry
2D GeometryComplete the proofs below by filling in the missing statements and reasons 19 Given 21 and 22 form a linear pair 21 23 Prove 22 and 23 are supplementary Statements 1 21 and 22 form a linear pair 2 21 and 22 are supplementary 3 m 1 m2 180 4 21 23 5 m 1 m 3 6 m 3 m2 180 7 22 and 23 are supplementary 1 2 3 4 5 6 7 Reasons 3 2
Geometry
3D GeometryEvaluate the flux integral SF ds where F x y z x 1 y 2 3 and C is the outward upward oriented half sphere z 9 x y 0 z 3

Geometry
2D Geometry28 Given WY XZ Prove WX YZ 1 WY XZ 2 WY XZ 3 WX XZ WZ WY YZ WZ 4 XZ YZ WZ Statements 5 WX XZ XZ YZ 6 WX YZ 7 WX YZ 1 2 3 4 5 6 7 W X Y Reasons

Geometry
Solution of triangles30 Given 21 and 23 form a right angle 21 and 22 are complementary Prove 22 43 Statements 1 21 and 23 form a right angle 2 21 and 23 are complementary 3 21 and 22 are complementary 4 22 23 1 2 3 4 A Reasons D C

Geometry
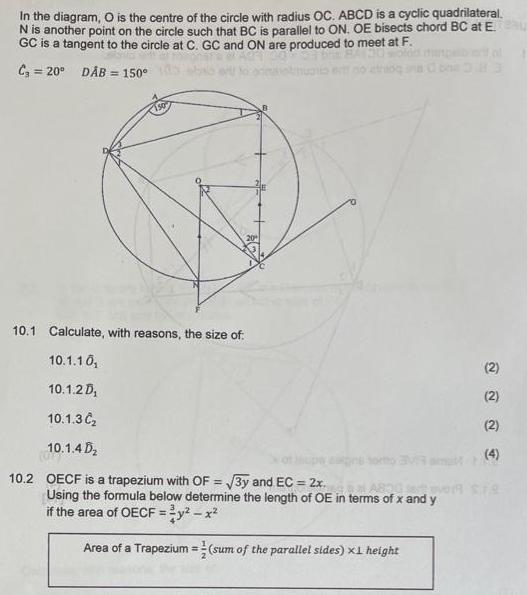
AreaIn the diagram O is the centre of the circle with radius OC ABCD is a cyclic quadrilateral N is another point on the circle such that BC is parallel to ON OE bisects chord BC at Eu GC is a tangent to the circle at C GC and ON are produced to meet at F C 20 D B 150 10 1 Calculate with reasons the size of 10 1 10 10 1 2 D 10 1 3 C 10 1 4 D 10 2 OECF is a trapezium with OF 3y and EC 2x Using the formula below determine the length of OE in terms of x and y if the area of OECF y x Area of a Trapezium sum of the parallel sides x1 height 2 2 2

Geometry
2D Geometryswer each of the following questions about transformations a Write the equation of a function that has been transformed up 4 adn right 3 from its parent function b In the function f x 3x4 8 what does the 3 coefficient tell you c In a function how do you determine if there is a horizontal stretch compression

Geometry
AreaWrite the complete factored form of fix 1x 3x 7x 7x 3 zeros 1 33 1x 0 Type your answer in factored form Use integer
Geometry
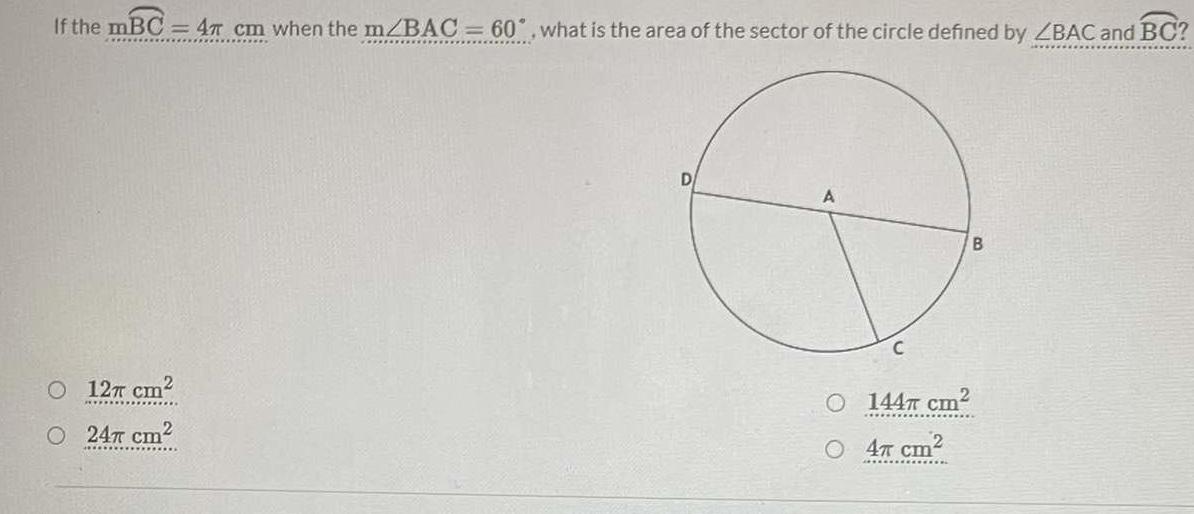
AreaIf the mBC 47 cm when the m BAC 60 what is the area of the sector of the circle defined by ZBAC and BC 12 cm 24 cm D A C B 144 cm 2 47 cm

Geometry
2D GeometryEd 16 5 O B LL F M FB is tangent to the circle shown Given FB 12 and AF 8 what is Al O 18 10
Geometry
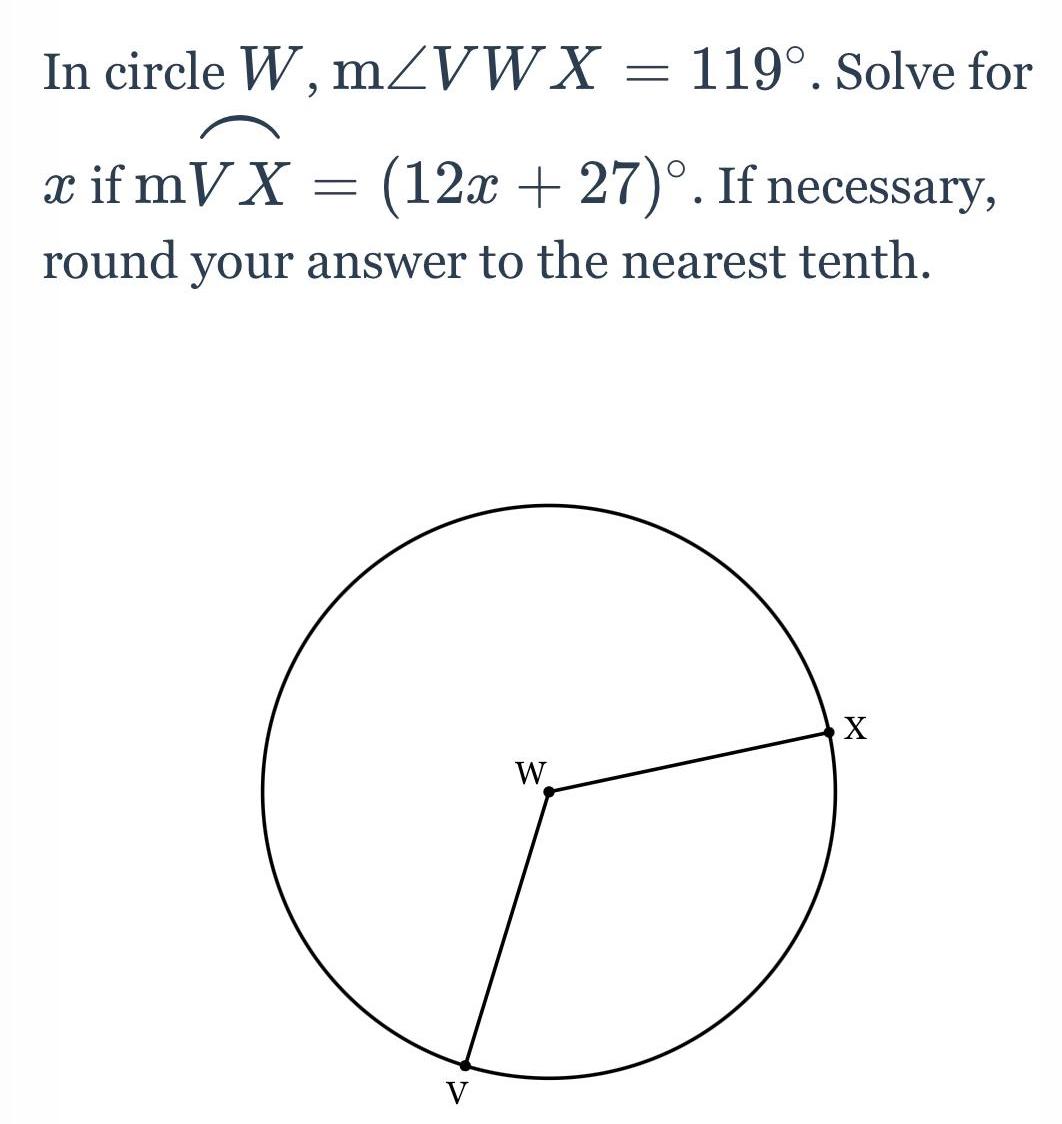
2D GeometryIn circle W m VWX 119 Solve for x if mVX 12x 27 If necessary round your answer to the nearest tenth V W X
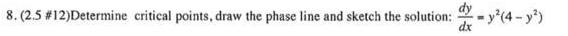
Geometry
2D Geometry8 2 5 12 Determine critical points draw the phase line and sketch the solution y 4 y dx
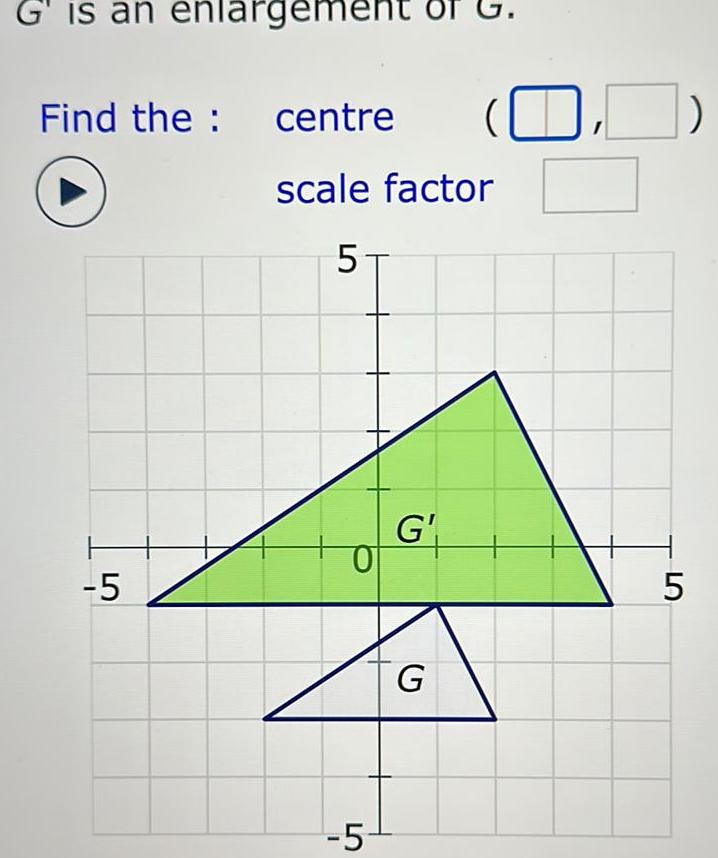
Geometry
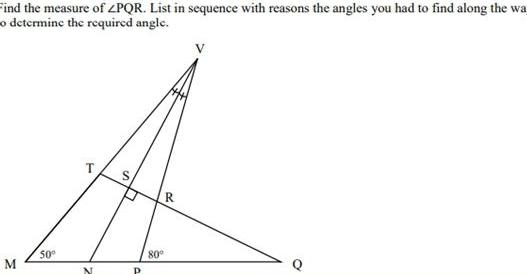
VectorsFind the measure of ZPQR List in sequence with reasons the angles you had to find along the wa o determine the required angle M 50 N S 17 P 80 R O

Geometry
2D Geometryf x x 2 The vertical stretch by 2 then the vertical shift up by 3 The vertical shift up by 3 then the vertical stretch by 2 Reflect Are the resulting graphs the same Does the order in which we apply the graph transformations matter This phenomenon is sometimes called the Socks then shoes vs Shoes then socks problem

Geometry
2D GeometryApply transformations in the column below that correspond to your chosen function to get new functions Use the button in the upper left corner of the page to add a new line for each of the transformations below Your subsequent graphs will be displayed in different colors Graph Transformations f x x2 The vertical stretch by 9 The vertical shrink by 9 The horizontal stretch by 3 The horizontal shrink by 3 Now consider the reflection question below You are not required to answer the question but pause to think about

Geometry
Vectors3 Estimate a polynomial for the sine function using the points sin0 0 sin 1 2 1 and sin 0 Hint rewrite as points in x y form to look more like the last problem Use your result to estimate sin 7 3
Geometry
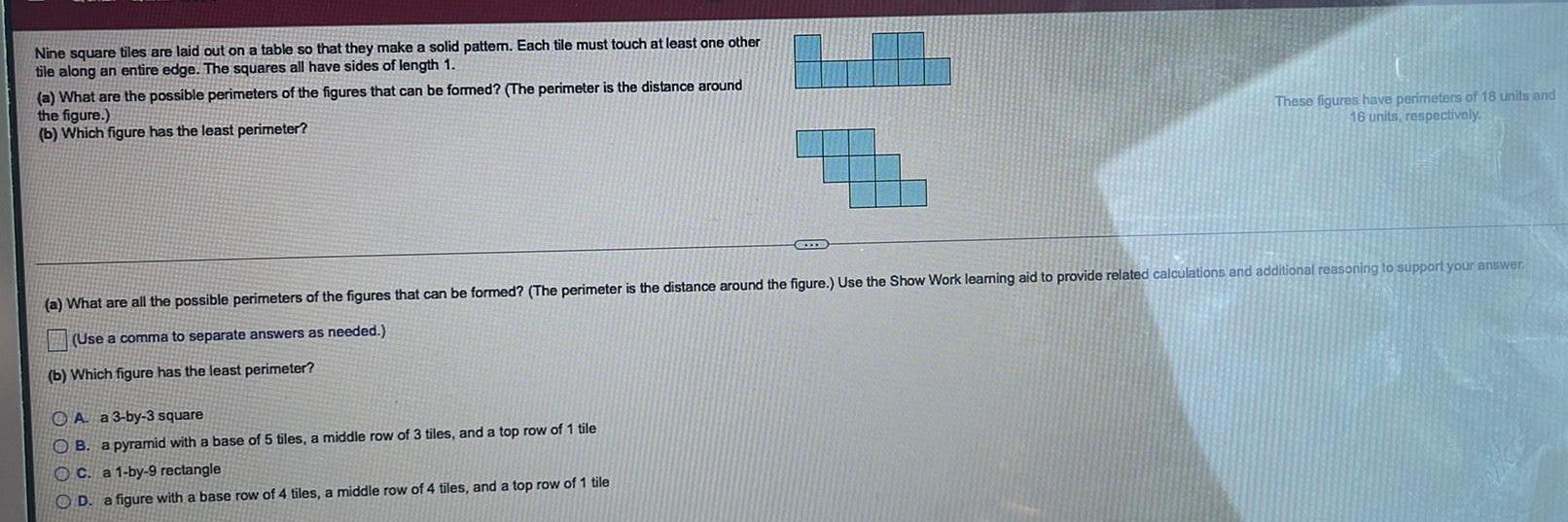
2D GeometryNine square tiles are laid out on a table so that they make a solid pattern Each tile must touch at least one other tile along an entire edge The squares all have sides of length 1 a What are the possible perimeters of the figures that can be formed The perimeter is the distance around the figure b Which figure has the least perimeter These figures have perimeters of 18 units and 16 units respectively a What are all the possible perimeters of the figures that can be formed The perimeter is the distance around the figure Use the Show Work learning aid to provide related calculations and additional reasoning to support your answer Use a comma to separate answers as needed b Which figure has the least perimeter A a 3 by 3 square B a pyramid with a base of 5 tiles a middle row of 3 tiles and a top row of 1 tile OC a 1 by 9 rectangle OD a figure with a base row of 4 tiles a middle row of 4 tiles and a top row of 1 tile
Geometry
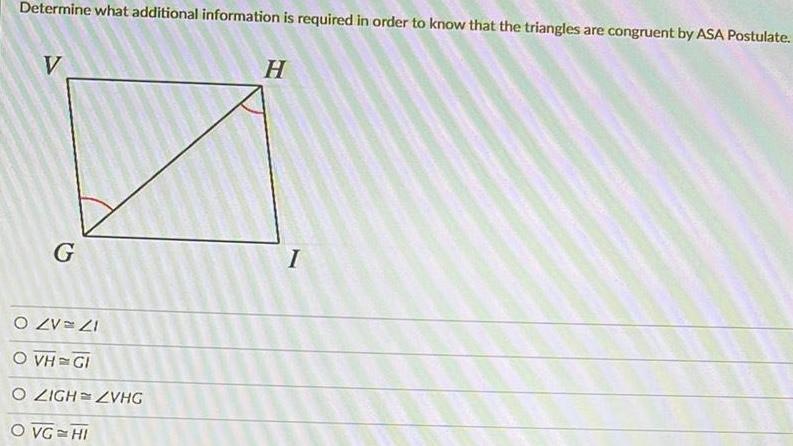
2D GeometryDetermine what additional information is required in order to know that the triangles are congruent by ASA Postulate V G O ZV 21 O VH GI O ZIGH ZVHG O VG HI H I
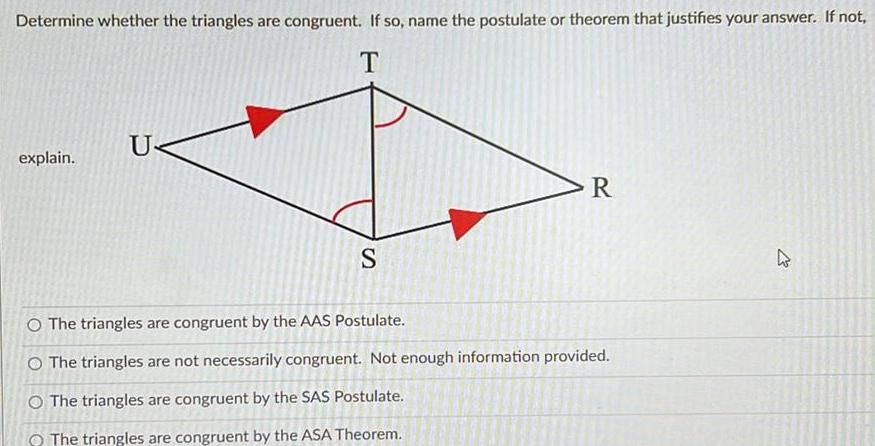
Geometry
Solution of trianglesDetermine whether the triangles are congruent If so name the postulate or theorem that justifies your answer If not T explain U S R O The triangles are congruent by the AAS Postulate O The triangles are not necessarily congruent Not enough information provided O The triangles are congruent by the SAS Postulate The triangles are congruent by the ASA Theorem 27

Geometry
2D GeometryWrite an equation a in slope intercept form and b in standard form for the line passing through 1 7 and perpendicular to 3x 7y 1 a The equation of the line in slope intercept form is Type your answer in slope intercept form Use integers or fractions for any numbers in the equation

Geometry
VectorsThe graph of a linear function f is shown a Identify the slope y intercept and x intercept b Write the equation that defines f The slope is Type an integer or a simplified fraction Ay 61 10 8 64 2 44 6 B E
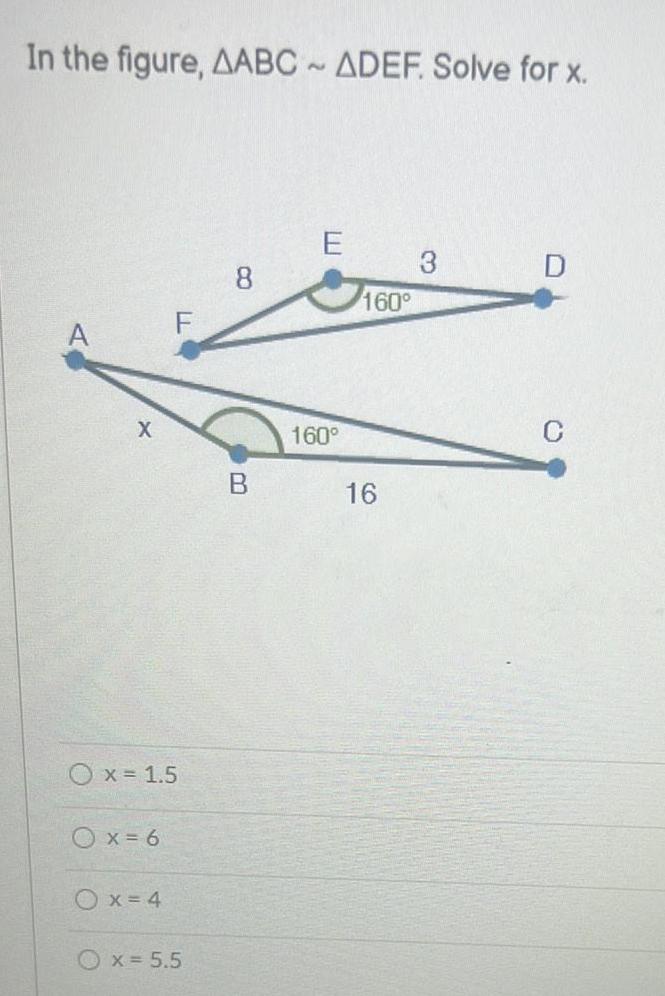
Geometry
Solution of trianglesIn the figure AABC ADEF Solve for x X O x 1 5 O x 6 F O x 4 x 5 5 8 B E 160 160 16 3 D C

Geometry
Coordinate system1 point Given the function f x 3x 4x 1 find the following a the average rate of change of f on 2 6 b the average rate of change of f on x x h
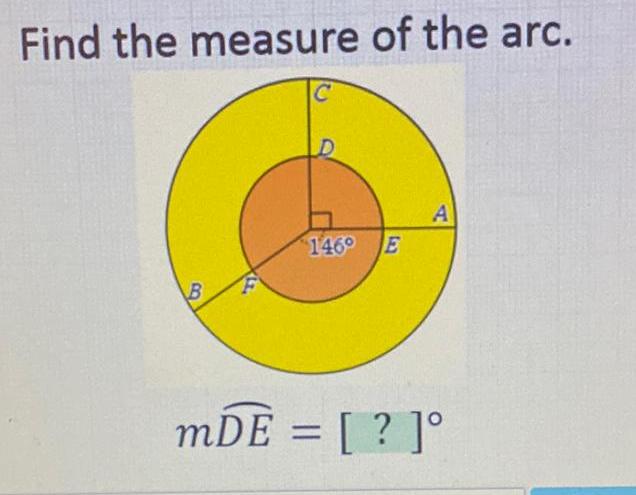
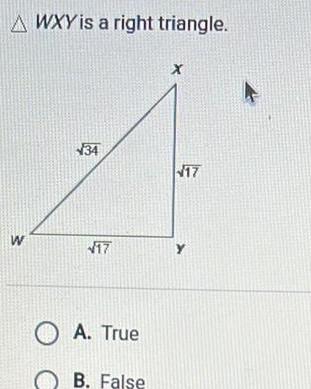

Geometry
Coordinate systemUse the following problem to answer questions 48 50 Find the dilation image AA B C of AABC with center at the origin 1 and k 48 A a 1 2 b 9 18 c 18 9 d 2 1 49 B a 0 1 b 9 0 c 1 0 d 0 9 50 C a 18 0 b 2 0 c 0 18 d 0 2


Geometry
Coordinate systemFind the central angle which subtends an arc of length 2 centimeters of a circle of radius 12 centimete 0 radians Type on intogor
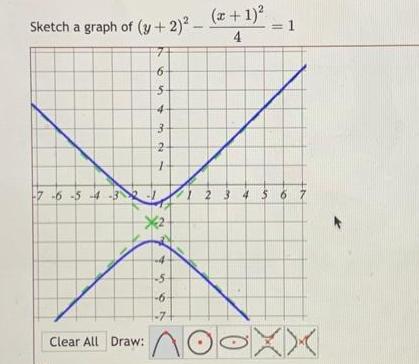
Geometry
Coordinate systemSketch a graph of y 2 7 6 5 4 3 Clear All Draw 6 54321 4 5 6 7 x 1 4 1 1 2 3 4 5 6 7 no XX

Geometry
2D GeometryPrepare a hand drawn plot of the two variables using the grid sheet in the lab manual or other graph paper a Include a title axis labels with units and a trendline 1pts b Estimate the circumference in centimeters of a circle with a diameter of 3 80 cm Circumference cm 1 pts c Estimate the diameter in centimeters of a circle with a circumference of 4 02 in Diameter cm
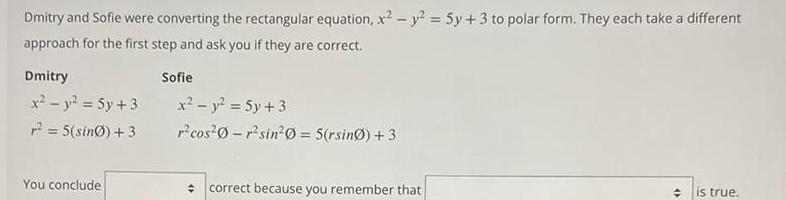
Geometry
3D GeometryDmitry and Sofie were converting the rectangular equation x y 5y 3 to polar form They each take a different approach for the first step and ask you if they are correct Dmitry x y 5y 3 r 5 sin0 3 You conclude Sofie x y 5y 3 r cos 0 r sin 0 5 rsin 3 correct because you remember that is true


Geometry
2D GeometrySelect the statements that are true Select one or more O a There are infinitely many ways to represent a point in rectangular coordinates O b There are infinitely many ways to represent a point in polar coordinates OC In polar coordinates y is a function of x Od All polar equations are functions

Geometry
2D GeometryWhen graphing the polar equation r 4 sin 60 as approaches from 0 the radius changes from to

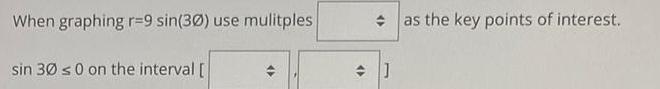
Geometry
2D GeometryWhen graphing r 9 sin 30 use mulitples sin 30 0 on the interval as the key points of interest

Geometry
2D GeometryGiven the rectangular equation 3x2 2y 18 Choose the correct first step s in converting this to polar form Select one or more Oa None of these O b O c O d 3rcos 2rsing 81 3 3 rcos 2 2rsin 9
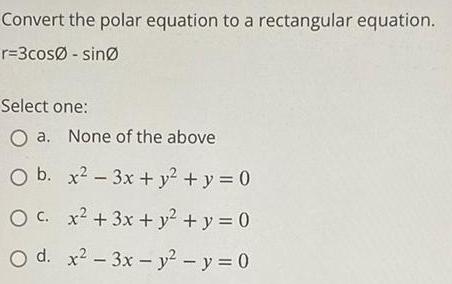
Geometry
Solution of trianglesConvert the polar equation to a rectangular equation r 3cos sin Select one O a None of the above O b x 3x y y 0 O c x 3x y y 0 O d x 3x y y 0

Geometry
2D GeometryWhen graphing the polar equation r 6 sin 60 as moves from O to radius changes from 0 to

Geometry
2D GeometryWhich of the following is NOT a true statement Select one O a In the polar coordinate r r is the distance from the origin O b A polar coordinate can be converted to a rectangular coordinate using r and sine and cosine OC A polar coordinate is represented by r Where r is the distance from the origin and is the angle measured the x axis moving counterclockwise Od In the Polar coordinate r is the angle measured from the x axis moving counterclockwise
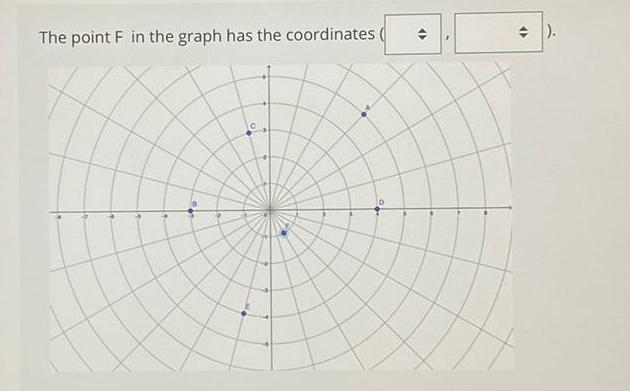
Geometry
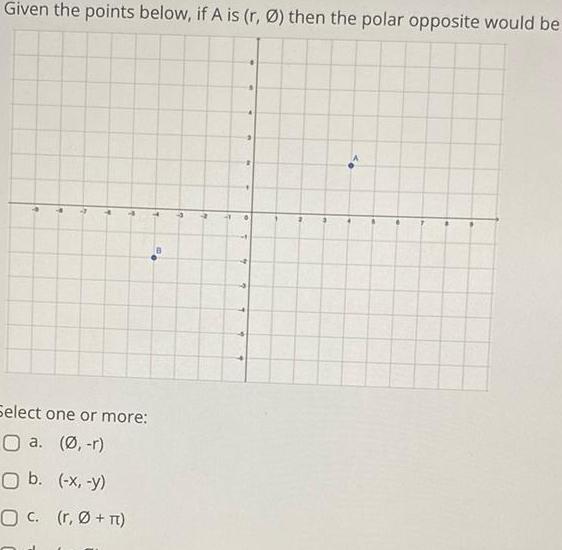
2D GeometryGiven the points below if A is r then the polar opposite would be Select one or more O a 0 r O b x y O C r
