Geometry Questions
The best high school and college tutors are just a click away, 24×7! Pick a subject, ask a question, and get a detailed, handwritten solution personalized for you in minutes. We cover Math, Physics, Chemistry & Biology.

Geometry
2D Geometry4 If m23 54 find each measure a m1 b m 2 c m 4 d m25 e m26 5 11 12 14 13 f m27 g m28 h m 9 i m 10 j m 11 k 1 m212 m 13 m m 14

Geometry
Coordinate systemTriangle ABC has the following coordinates A 1 1 B 5 4 C 4 1 and Triangle ABC will be translated 3 units right and 6 units up 1 Write a coordinate rule to describe the translation 2 What will be the coordinates of the image of point A

Geometry
Coordinate systemFind the coordinates of the vertices of the figure after the given transformation Take the following coordinates and translate each point 2 units down D 4 1 A 2 5 S 1 4 N 1 2 D 4 1 A 2 5 S 1 4 N 1 2 D 4 2 A 2 6 S 1 3 N 1 1 D 4 1 A 2 3 S 1 2 N 1 0 D 3 1 A 3 5 S 1 1 N 1 2

Geometry
Coordinate systemK Question 9 2 Use the currency exchange rates in the table for the following question You arrive in Montreal with 580 How many Canadian dollars can you buy You can buy Canadian dollars with 580 Simplify your answer Round to two decimal places as needed
Geometry
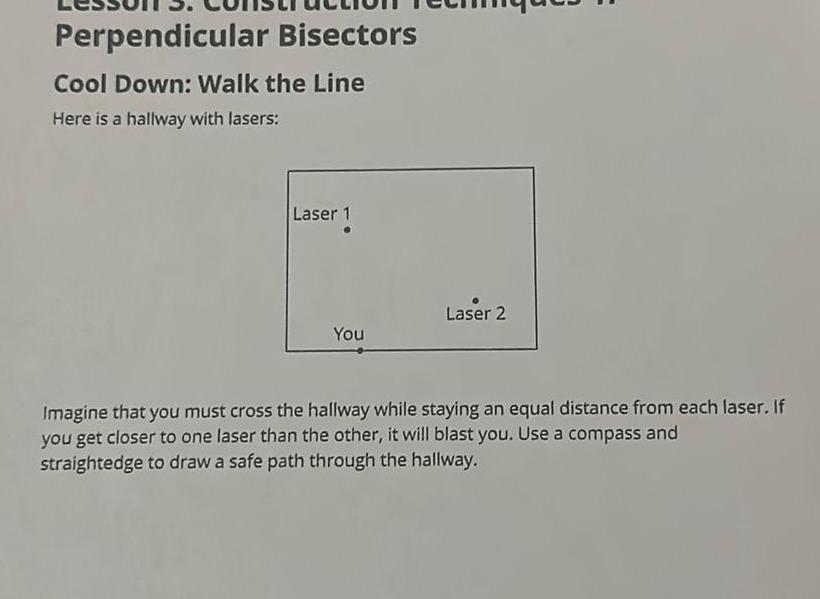
Coordinate systemPerpendicular Bisectors Cool Down Walk the Line Here is a hallway with lasers Laser 1 You Laser 2 Imagine that you must cross the hallway while staying an equal distance from each laser If you get closer to one laser than the other it will blast you Use a compass and straightedge to draw a safe path through the hallway

Geometry
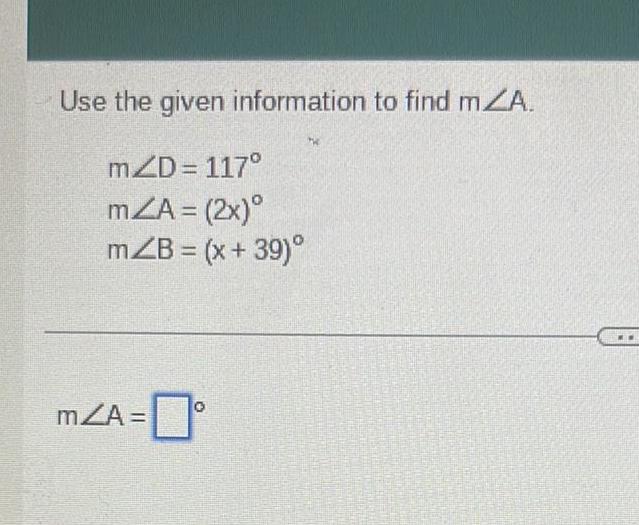
2D Geometry3 T I K 5 Find KL 9 E K 7 7 Find EC K U 10 32 D 20 26 H 49 G 11 16 30 K H L B 2 R 4 c G S K 6 Find HJ K 14 IK 8 Find IK H 13 D 30 12 49 K T 31 E 12 Points A B and C are collinear Point B is between A and C Find the length indicated L
Geometry
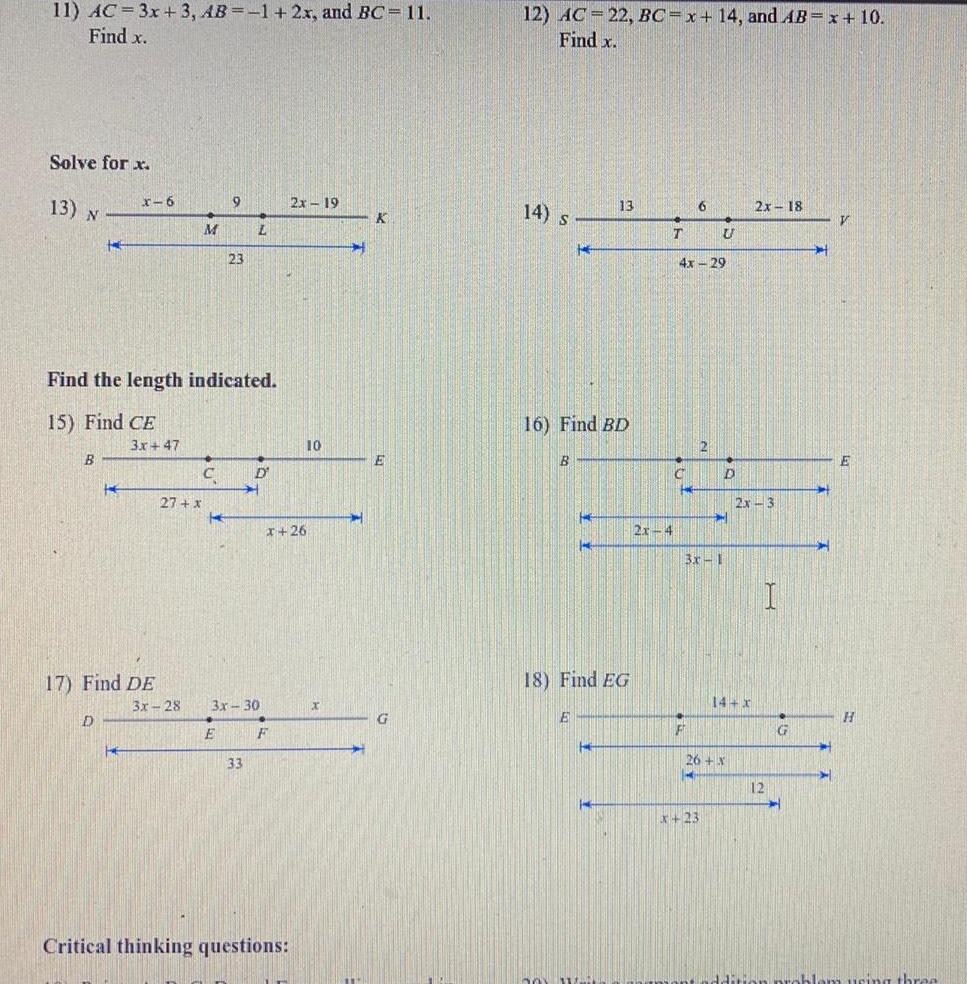
2D Geometry11 AC 3x 3 AB 1 2x and BC 11 Find x Solve for x 13 N B x 6 H D Find the length indicated 15 Find CE 3x 47 17 Find DE 27 x M 3x 28 C 9 23 E L 33 D 3x 30 H x 26 F 2x 19 Critical thinking questions 10 x K E G 12 AC 22 BC x 14 and AB x 10 Find x 14 s H B 16 Find BD HE 14 13 18 Find EG F T 2x 4 C 4x 29 HE F 6 2 x 23 U 3x 1 D 26 X A 14 x 2x 18 2x 3 12 H G V H addition problem using three
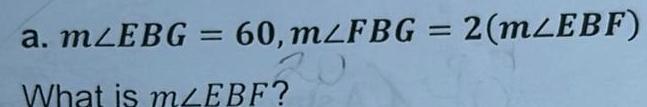
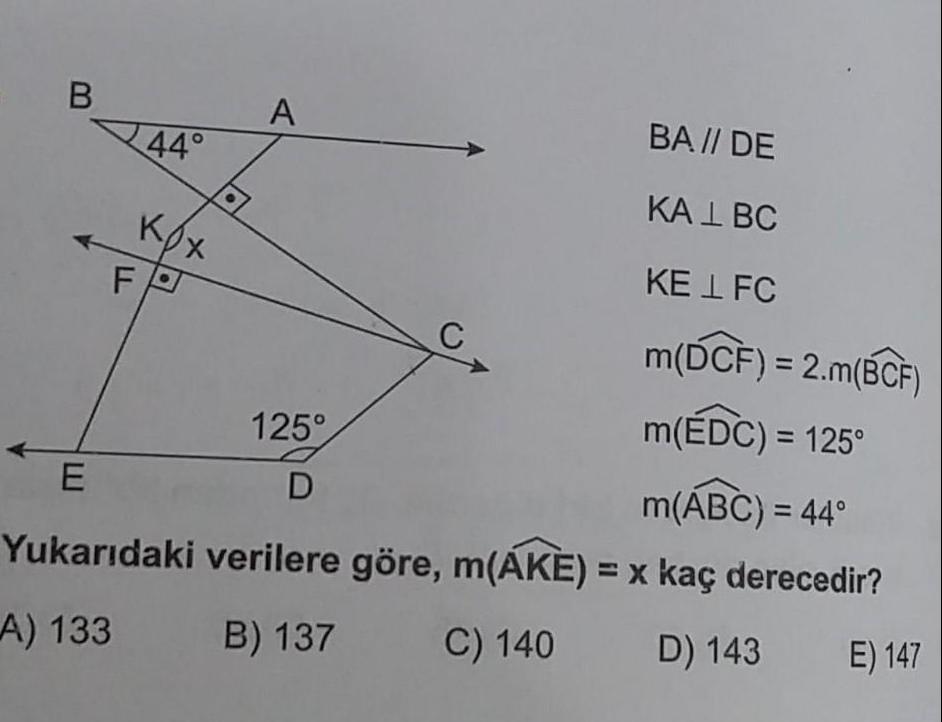
Geometry
2D GeometryB BA DE KAL BC KE LFC m DCF 2 m BCF m EDC 125 E m ABC 44 Yukar daki verilere g re m AKE x ka derecedir A 133 B 137 C 140 D 143 44 Kox FO A 125 D E 147
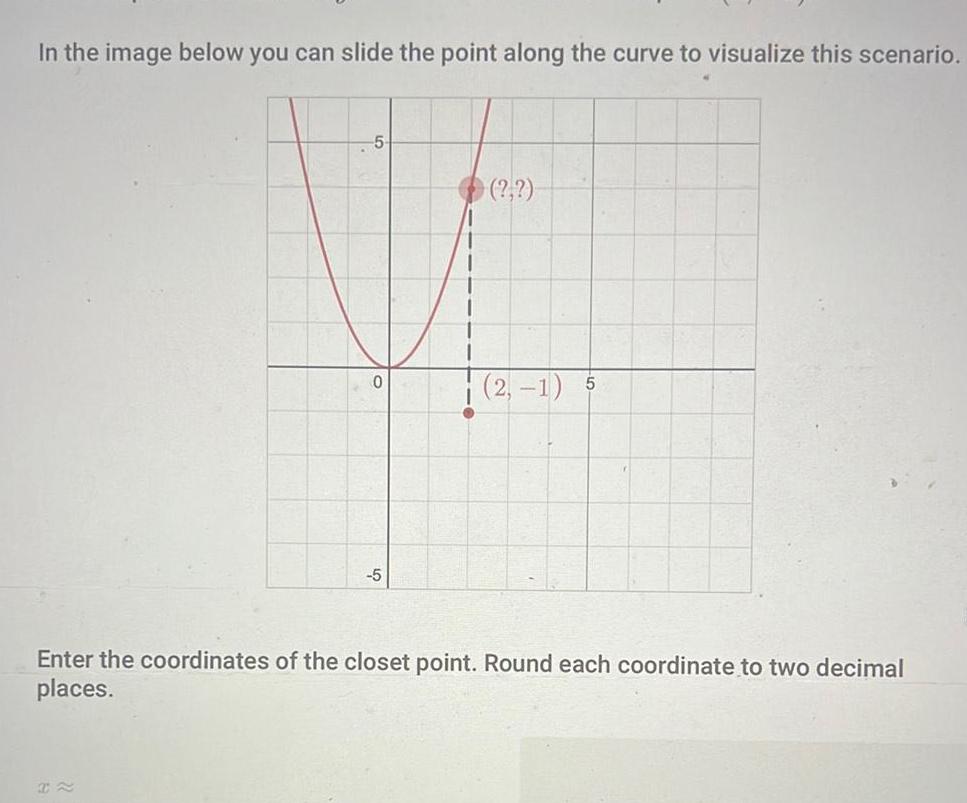
Geometry
AreaIn the image below you can slide the point along the curve to visualize this scenario 5 0 5 2 1 5 Enter the coordinates of the closet point Round each coordinate to two decimal places

Geometry
2D GeometryIn triangle ABC angle A is 60 and C is a right angle The length of side bis 9 as shown Find the lengths of the other sides and c Give the exact answers but you may not use sin cos or tan Use sqrt 3 or 3 5 for 3
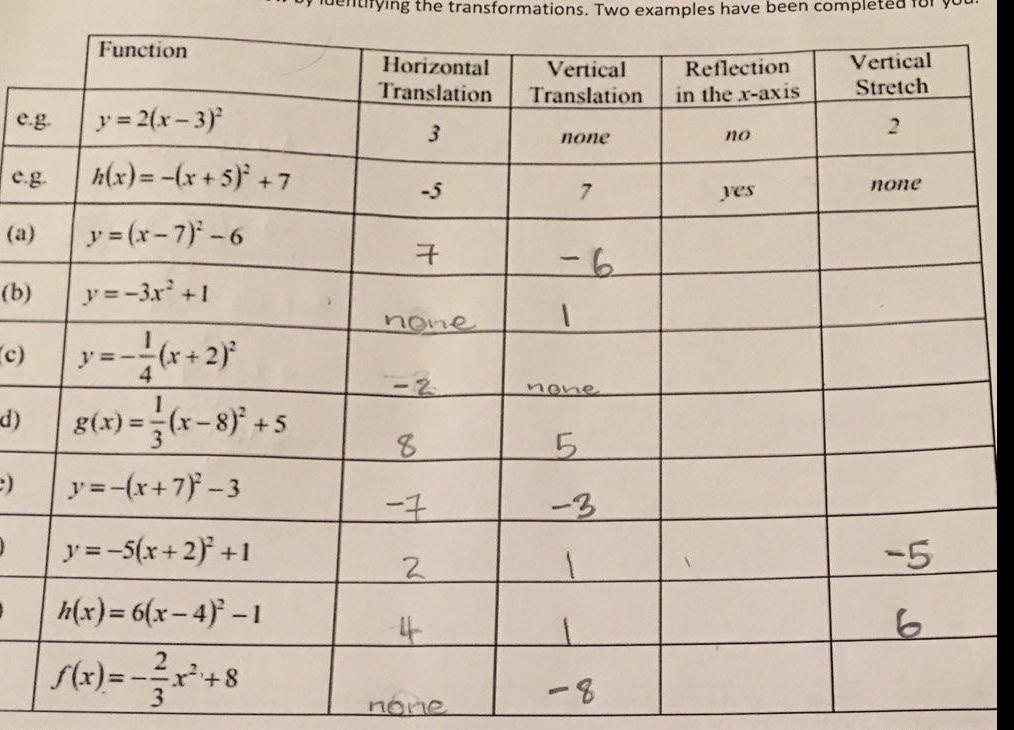
Geometry
Coordinate systeme g e g a 1 b Function y 3x 1 y x 2 d g x x 8 2 5 y x 7 3 0 y 5 x 2 1 h x 6 x 4 1 f x 1 x 8 3 y 2 x 3 h x x 5 7 y x 7 6 ying the transformations Two examples have been completed Horizontal Translation 3 5 7 none 2 8 7 2 4 none Vertical Translation none 1 7 none 5 3 Reflection in the x axis no yes Vertical Stretch 2 none 5

Geometry
2D GeometryWhich of the following represent deductive reasoning Select all that apply The measures of the two angles in a linear pair add to 180 ZNTR and ZMKG form a linear pair so the measures of NTR and ZMKG add to 180 Birds fly south for the winter A penguin is a bird so it will fly south in the winter The sum of the measures of the interior angles of any triangle is 180 Polygon QAG is a triangle so the sum of the measures of its interior angles must be 180 Traffic is always busy on Friday afternoons at 4pm It is Friday at 4pm so traffic will be busy All rectangles are quadrilaterals A square is a rectangle so a square must be a quadrilateral
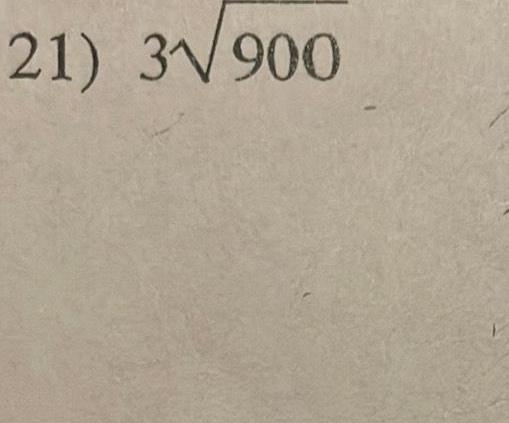

Geometry
Coordinate systemTriangle DEF is rotated about point P Find the measure of ZF P D D E 42 69 E F
Geometry
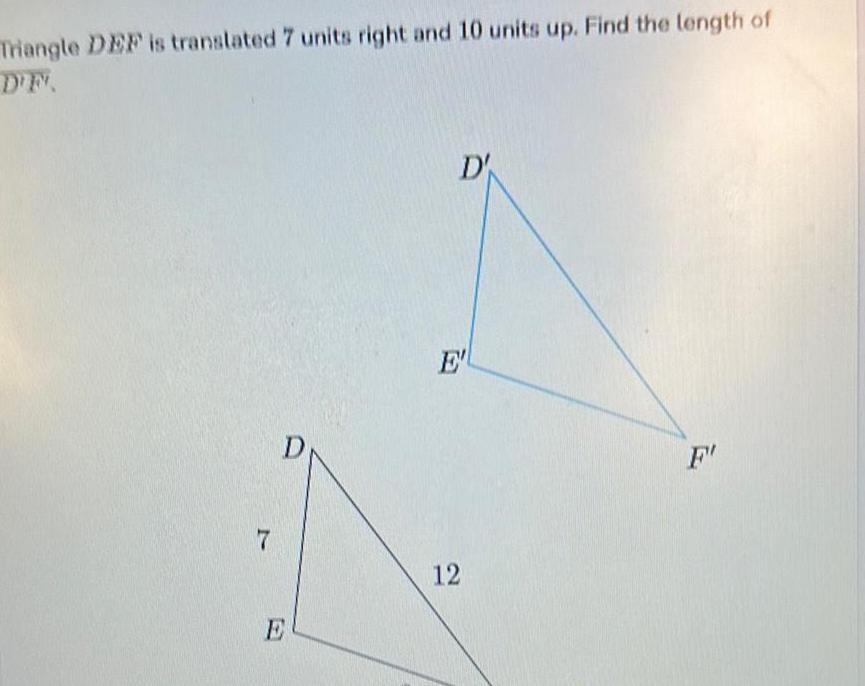
2D GeometryTriangle DEF is translated 7 units right and 10 units up Find the length of D F 7 D E 12 F

Geometry
Coordinate systemSoru 10 B 17 D 4 A 7 E X C ABC geninde m AED mA AD 4 br DB 17 br AE 7 br Buna g re EC x ka birimdir A 5 B 6 C 7 D 8

Geometry
2D Geometryraph the equation 40 y2 2x Ay 43 4 2 2 2 2 4x Evaluate the expression 41 2x 3y 6 4 2 41 2 2 4 2 4x

Geometry
2D Geometryopen top rectangular box is to be made from a piece of cardboard 26 inches long and 8 inches wide by cutting out identical squares from the four corners and turning up the sides In the image below you can adjust the location of the point to visualize different possible boxes Let x be the side length of each of the removed squares from the corners Express the volume V of the resulting box as a function of x V x powered by desmos What is the domain of this function Use interval notation Find the dimensions of the box that yields a maximum volume Round your answers to two decimal places
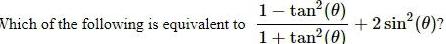

Geometry
AreaLesson 1 Practice Problems Here is a diagram of a straightedge and compass construction C is the center of one circle and B is the center of the other Explain why the length of segment BD is the same as the length of segment AB O
Geometry
Coordinate systemxly 2 2603 7 3x 2y 10 1157 0 6 2 10333 2 3 4 321 8 2y 4x 6 29 12 6 1 3200 0 32610 26 P 345 2 i Sy 6 12 M 4 1 1 9 y 3 1 1 4904 IMI 4 7 T LL 1 3

Geometry
Areaa y b PQ c QR 13 If AC 63 AB 4z 9 BC 3z 12 find the value of z Then find the length of AB and BC AC AB BC 42 9 32 12 63 72 24 63 21 L N 21 84 L 2 12 14 If DE is the same value as EF DE 3x and EF 2x 8 find the value of x Then find the length of DF Part 3 Construction Use a compass and straightedge only 15 Construct a circle L 7 Construct a semicircle by using the radius NO A D E 16 Construct a circle C by using the radius CD 18 Construct a quarter circle by using the radius NO

Geometry
2D Geometrysin 0 tan 0 csc 0 sec 8 cot 8 Need Help Submit Answer 3 5 Points sin 0 cos 0 Find the values of the trigonometric functions of 0 from the information given csc 0 4 0 in Quadrant I tan 0 Read It sec 0 DETAILS Watch It

Geometry
Coordinate systemThe angle 0 is an acute angle and sin i 180 Use the Pythagorean identity sin 20 cos 0 1 to find cos 0 8 www cos 0 Simplify your answer Type an exact answer using radicals as needed Rationalize all denominators
Geometry
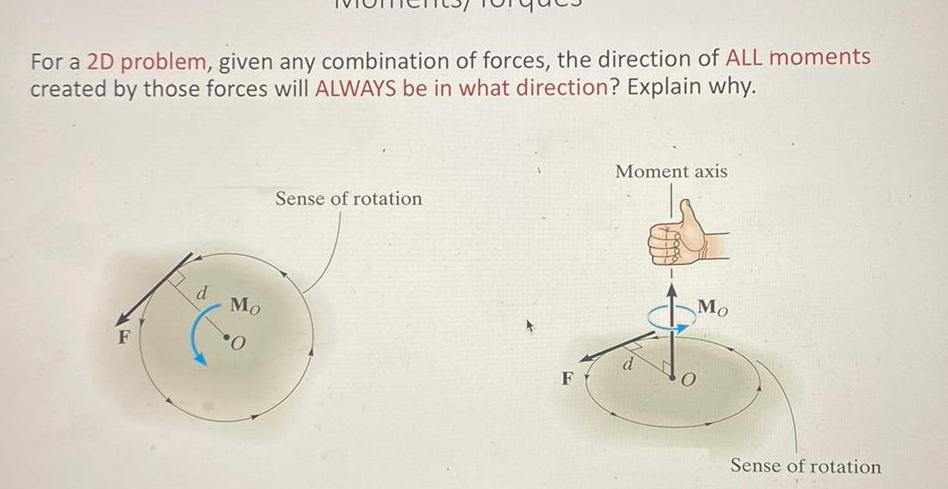
Coordinate systemFor a 2D problem given any combination of forces the direction of ALL moments created by those forces will ALWAYS be in what direction Explain why F d Mo O Sense of rotation Moment axis Mo O Sense of rotation
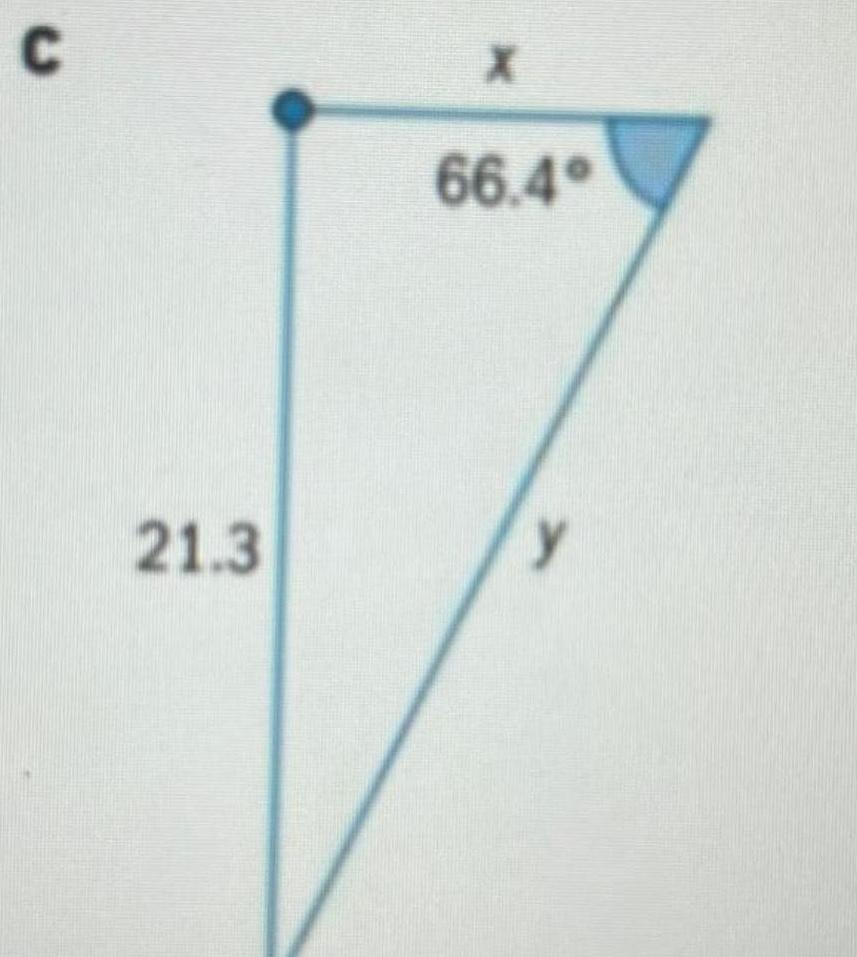
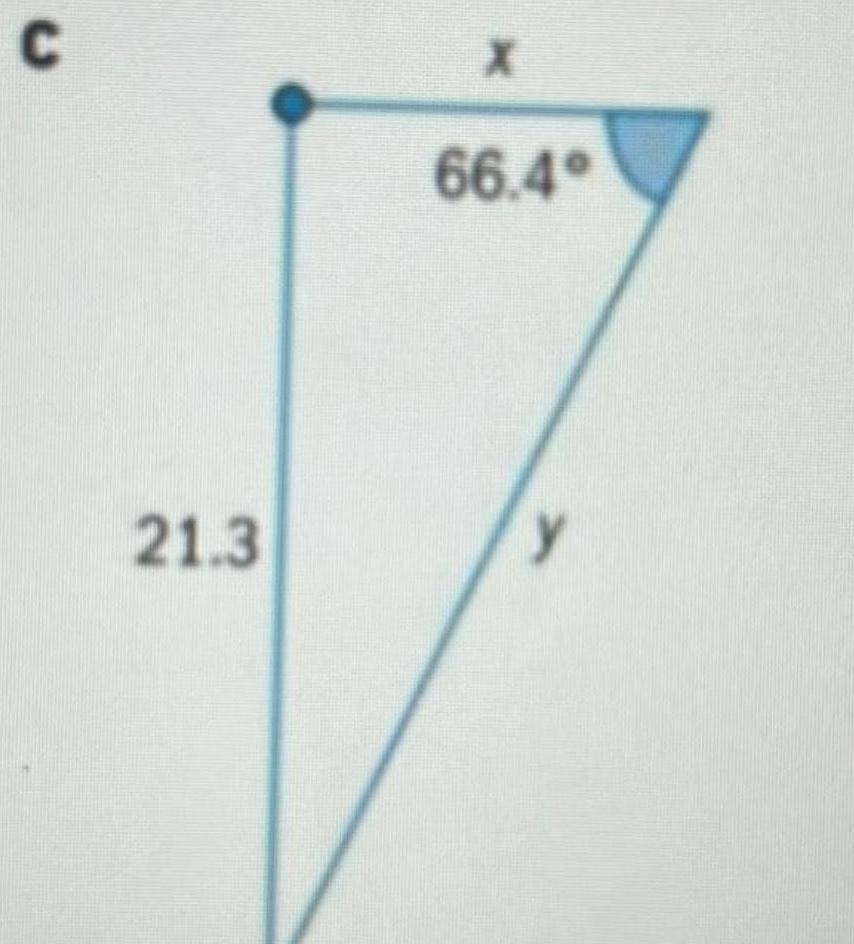
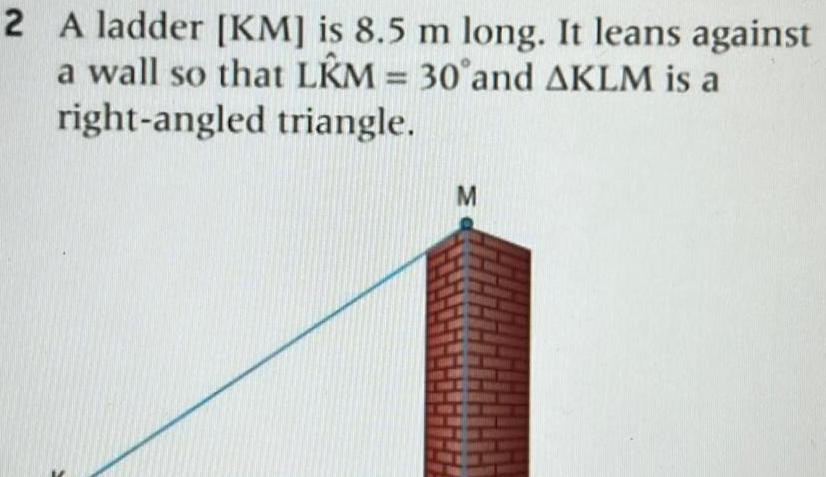
Geometry
2D Geometry2 A ladder KM is 8 5 m long It leans against a wall so that L M 30 and AKLM is a right angled triangle M

Geometry
Solution of triangles3 Scientists measure the depths of lunar craters by measuring the length of the shadow cast by the edge of the crater using photos In a photograph the length of the shadow cast by the edge of the Moltke crater is about 606 metres given to the nearest metre The sun s angle of elevation is 65 Find the depth of the crater giving your answer rounded to the nearest metre

Geometry
3D Geometry4 The height of a building is 72 m Find the angle of elevation from a point on ground level that is 55 m away from the base of the building 5 From a boat 160 m out at sea the angle of elevation to the coast is 18 The angle of elevation to the top of a lighthouse on the coast is 22 a Draw a diagram representing the situation b Find the height of the lighthouse

Geometry
2D Geometry1 The angle of depression from the top of a cliff to a boat at sea is 17 The boat is 450 m from the shore Draw a diagram representing the situation Find the height of the cliff giving your answer rounded to the nearest metre 2 A family wants to buy an awning for a French window that will be long enough to keep out the sun when it is at its highest point in the sky The height of the French window is 2 80 m The angle of elevation of the sun from this point to the bottom of the window is 70 The awning will be 90 to the window Find how long the awning should be Write down your answer correct to 2 dp a b
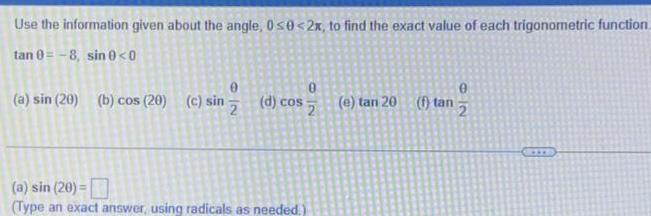
Geometry
2D GeometryUse the information given about the angle 0 0 2x to find the exact value of each trigonometric function tan 0 8 sin 0 0 0 a sin 20 b cos 20 c sin d cos 02 a sin 20 Type an exact answer using radicals as needed e tan 20 f tan 0 2

Geometry
2D Geometry3 A hiker whose eye is 1 6 metres above ground stands 50 metres from the base of a vertical cliff The angle between the line connecting her eye and the top of the cliff and a horizontal line is 58 a Draw a diagram representing the situation b Find the height of the cliff

Geometry
2D Geometryangles with the following sides are right angled triangles a 9cm 40 cm 41 cm b 10 m 24 m 26 m c 10 10 200 d 11 2 7 5 8 3 3 The spiral in the figure is made by starting with a right angled triangle with both legs of length 1 unit 1 1 1 11 1 a Find the hypotenuse of the first triangle Then the second right angled triangle is built with one leg measuring 1 unit and the other leg being the hypotenuse of the first triangle b Find the hypotenuse of this second triangle A third right angled triangle is built on the second triangle s hypotenuse again with the other leg measuring 1 unit c Find the hypotenuse of the third triangle The process is continued in the same fashion and the hypotenuse of the final triangle is denoted by x d Find the length of x 4 A right angled triangle has a hypotenuse of 8 2 cm and another side length of 4 3 cm Draw a diagram Calculate the area of the triangle Give your answer correct to 1 dp

Geometry
3D GeometryDetermine the length of each arc with radius r and central angle a given below Give your answer correct to 2 dp a r 5 cm 70 b r 4 cm 45 c r 10 5 cm a 130 The London Eye is a giant Ferris wheel in London It is the tallest Ferris wheel in Europe with a diameter of 120 m The passenger capsules are attached to the circumference of the wheel and the wheel rotates at 26 cm per second a the length that a passenger capsule would travel if the wheel makes a rotation of 200 b the time in minutes that it would take for a passenger capsule to make a rotation of 200 t the time to the nearest whole minute that it would take for a passenger capsule to make a full revolution

Geometry
Heights & Distances1 Determine the length of the unknown sides for each of the right angled triangles below b 10 2 9 cm 61 2 Z I Z y 34

Geometry
2D GeometryA door with width 4 20 m has an arc as shown in the diagram Find a b The radius of the arc to the nearest cm The length of the arc to the nearest cm 225 4 20 m

Geometry
Heights & Distancesdifferent regions in 1985 and in 2005 Population in Population 1985 in 2005 4 9 10 6 4 109 1 1 x 10 1 3 x 109 7 6 x 108 1 1 109 2 4 108 3 0 108 8 9 x 106 7 2 x 106 Place Entire world China India United States Bulgaria a Determine the ratio of the population of the entire world to that of China in 2005 giving your answer to the nearest integer b Find the increase in the world s population between 1985 and 2005 c Calculate the percentage change in the population of each of the four countries giving your answers accurate to 3 sf List the countries according to their percentage change from highest to lowest d State whether or not you agree that it



Geometry
Area7 In an experiment the probability that event A occurs is and the probability that event B 8 occurs is 334 If A and B are independent events what is the probability that A and B both occur Simplify any fractions

Geometry
2D Geometry3 The image of a speck of dust viewed through an electron microscope is 1 2 x 10 millimetres wide The image is 5 x 102 times as large as the actual size Determine the width in millimetres of the actual speck of dust
Geometry
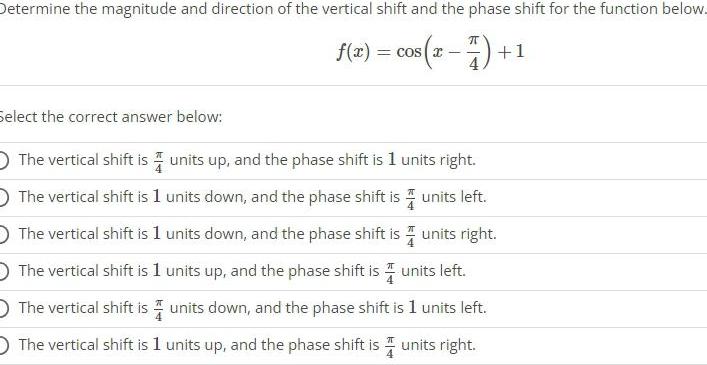
2D GeometryDetermine the magnitude and direction of the vertical shift and the phase shift for the function below f x cos x 7 Select the correct answer below The vertical shift is units up and the phase shift is 1 units right The vertical shift is 1 units down and the phase shift is The vertical shift is 1 units down and the phase shift is The vertical shift is 1 units up and the phase shift is units left O The vertical shift is units down and the phase shift is 1 units left The vertical shift is 1 units up and the phase shift is units right 1 units left units right

Geometry
Area2 In 1856 Andrew Waugh announced Mount Everest to be 8840 m high after several years of calculations based on observations made by the Great Trigonometric Survey More recent surveys confirm the height as 8848 m Calculate the percentage error made in the earlier survey 4 The temperature today in Chicago is 50 F Instead of using the standard conversion 5 formula C x F 32 Tommaso uses his grandmother s rule which is easier but gives an approximate value Subtract 32 from the value in F and multiply the result by 0 5


Geometry
3D Geometry3 With 72 million bicycles correct to the nearest million Japan is at the top of the list of countries with most bicycles per person On average Japanese people travel about 2 km by bicycle correct to the nearest km each day Calculate the upper bound for the total distance travelled by all the bicycles in Japan per day 4 To determine whether a business is making enough profit the following formula is used P C S C S where P is relative profit S is sales and C is costs If a company has 340 000 worth of sales and 230000 of costs correct to 2 significant figures calculate the maximum and minimum relative profit to the ate

Geometry
Solution of trianglesA triangle has a base length of 3 1 cm and corresponding height 4 2 cm correct to 1 decimal place Calculate the upper and lower bounds for the area of the triangle as accurately as possible 4 2 cm 3 1 cm

Geometry
2D GeometryFind the range of possible values for the following measurements which were rounded to the nearest mm tenth of m and hundredth of kg respectively b 3 2 m c 1 75 kg a 24 mm