High School Calculus Questions
The best high school and college tutors are just a click away, 24×7! Pick a subject, ask a question, and get a detailed, handwritten solution personalized for you in minutes. We cover Math, Physics, Chemistry & Biology.
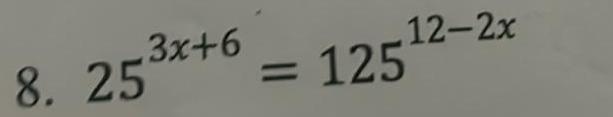
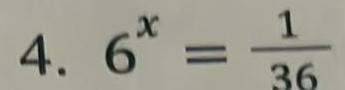
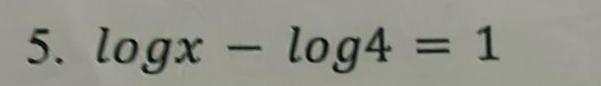
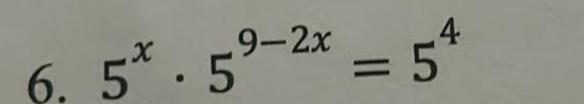
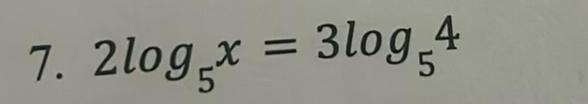

High School Calculus
Indefinite IntegrationIf log 2 0 4307 and log 3 0 6827 then using the product and power properties of logarithms what is the approximate value of log 6 Your final answer must be accurate to three places after the b decimal

High School Calculus
Vector Calculusse the change of base formula to evaluate the following expression log n
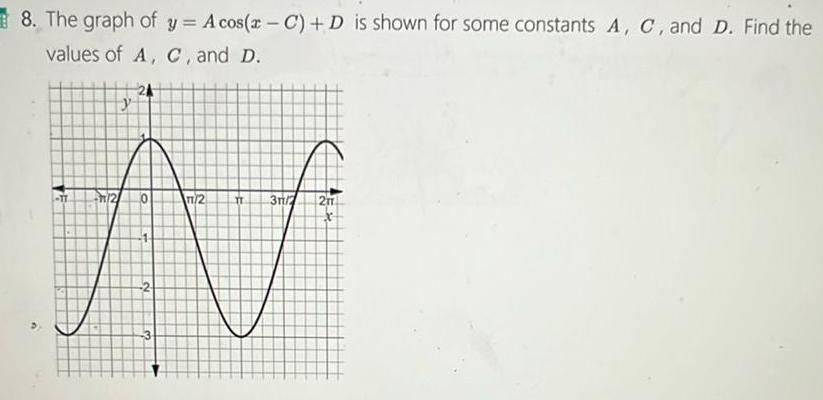
High School Calculus
Differentiation8 The graph of y A cos x C D is shown for some constants A C and D Find the values of A C and D 24 y TT 2 0 11 2 TT 31 2 211 M 2 3 5
High School Calculus
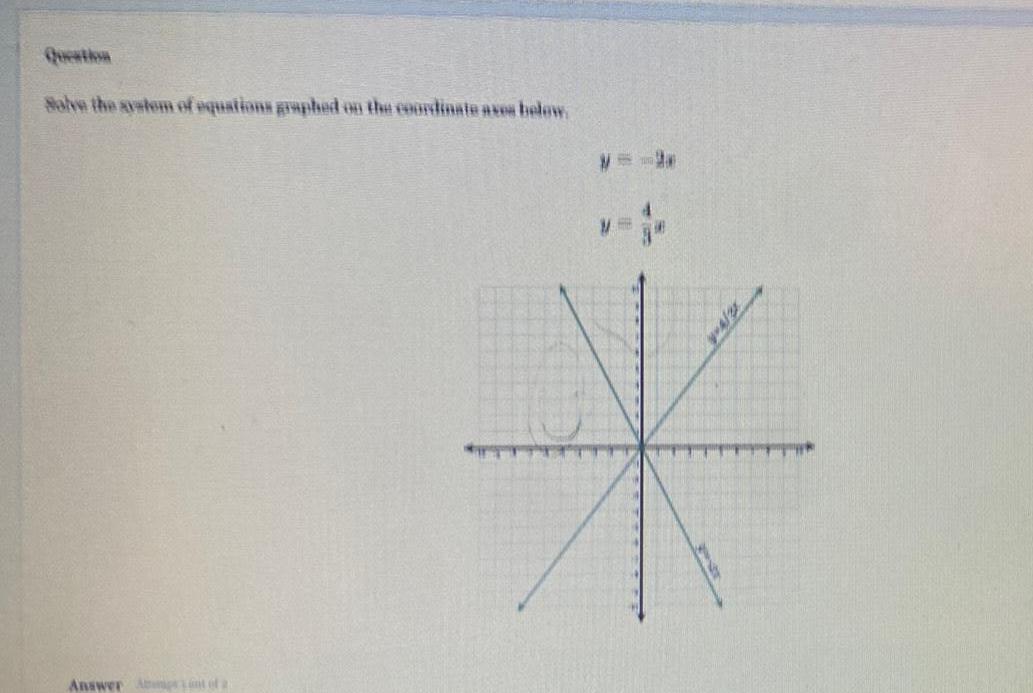
Vector CalculusSolve the system of equations graphed on the coordinate axes below Answer Amprint of 2 y V WA SX

High School Calculus
Application of derivatives2 Tiana started working at Pax River Ale House earning 9 25 per hour Every six months she gets a 3 25 raise If Tiana has worked at the Ale House for 4 years what is her current hourly rate

High School Calculus
Differentiation1 A cylindrical soup can of volume 500 cm is to be constructed The material for the top costs 0 4e cm while the material for the bottom and the sides costs 0 2e cm Find the dimensions correct to one decimal place that will minimize the cost for producing the can Justify your answer Cylinder formulae Vrh and Total SA 2arh 2r 8 marks

High School Calculus
Application of derivatives3 While skiing last weekend Mr Weisner noticed an ice sculpture that started out at 52 inches tall wa melting continuously at a rate of 3 per minute Find the height of the ice sculpture after 15 minu

High School Calculus
Limits & Continuity2 If sin 0 788 and cos 0 0 616 find each of the following Round to the nearest thousandth a sec 0 b cot 0 C csc 0 d tan
High School Calculus
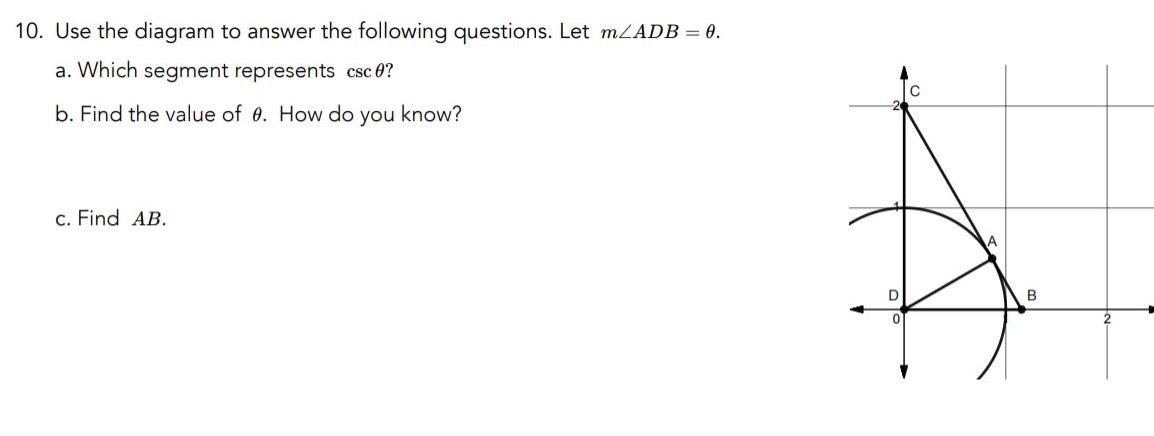
Differential equations10 Use the diagram to answer the following questions Let m ADB 0 a Which segment represents csc 0 b Find the value of 0 How do you know c Find AB A 2

High School Calculus
Application of derivatives5 If tan cot 0 and 0 0 A sin cos which of the following statements must be true B 1 sec 0 1 csc 0 C sin cos 0 1 D None of the above


High School Calculus
Definite Integrals7 Simplify each trig expression into one number or an expression with a single trig function 1 CSC x a b sin x cos x tan x C cot a csc x d 1 sin x 1 tan x
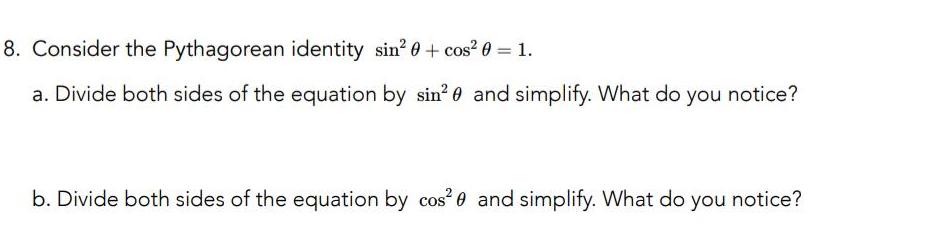
High School Calculus
Vector Calculus8 Consider the Pythagorean identity sin 0 cos 0 1 a Divide both sides of the equation by sin and simplify What do you notice b Divide both sides of the equation by cos and simplify What do you notice


High School Calculus
DifferentiationUse the table below with selected values of the twice differentlable function k Reach each explanation and decide whether you would apply IVT EVT or MVT X K x I 5 2 2 3 4 4 4 applies 5 3 6 2 7 O 6 Since k is differentiable it is also continuous Since k 6 2 and k 7 0 and since I is between 2 and O It follows by that K c 1 for some c between 6 and 7 7 Since k is differentiable and therefore also continuous and since 3 k 2 6 it follows by that k c 6 for some 3 2 c in the interval 2 3 8 There must be a minimum value for k at some r in 1 7 because k is differentiable and therefore also continuous Hence the q There must be some value a in 2 6 for which k a 0 because k 2 k 6 and since k is differentiable the applies 10 Since k is differentiable the guarantees some a in 4 5 for which k a 4 and also some b in 5 6 for which k b 1 Then since k is differentiable and therefore also continuous it follows by the applied to k that k c 0 for therefore in 46
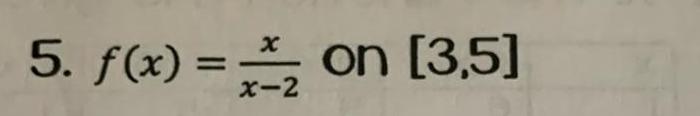

High School Calculus
Vector CalculusA health magazine reported that 27 of adult males smoke cigarettes To test this claim they select 40 adult males at random and record if they smoke cigarettes They calculate a 95 confidence interval for the proportion of adult males who smoke and find it is 22 to 28 Interpret the confidence interval O We are 95 confident that the interval from 0 22 to 0 28 captures the true proportion of adult males who smoke O If the magazine took many random samples of 40 adult males then 95 of the constructed confidence intervals would capture the true proportion of adult males who smoke The interval from 0 22 to 0 28 captures 95 of the adult males who smoke

High School Calculus
Vector CalculusFind the missing side lengths Leave your answers as radicals in simplest 1 2 3 5 3 3 30 60 12 18 E n 60 4 6 30 45 4 3 X 3 2 30 2 3
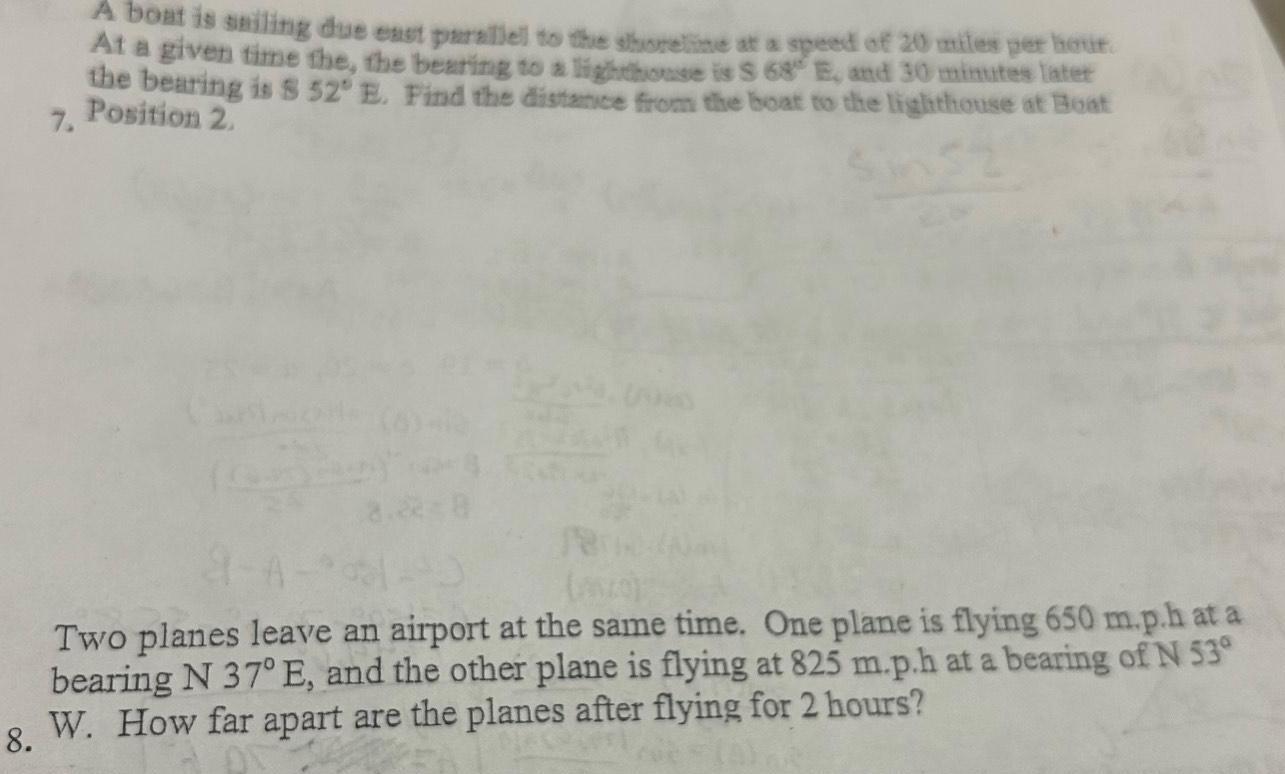
High School Calculus
Application of derivativesA boat is sailing due east parallel to the shoreline at a speed of 20 miles per hour At a given time the the bearing to a lighthouse is 68 E and 30 minutes later the bearing is 52 E Find the distance from the boat to the lighthouse at Boat Position 2 0 Two planes leave an airport at the same time One plane is flying 650 m p h at a bearing N 37 E and the other plane is flying at 825 m p h at a bearing of N 53 W How far apart are the planes after flying for 2 hours FORS 8

High School Calculus
Application of derivatives6 1 point What is the IQR of the set of the data below 52 61 71 63 54 69 63 65 68 58 57 11 10 15 000 019
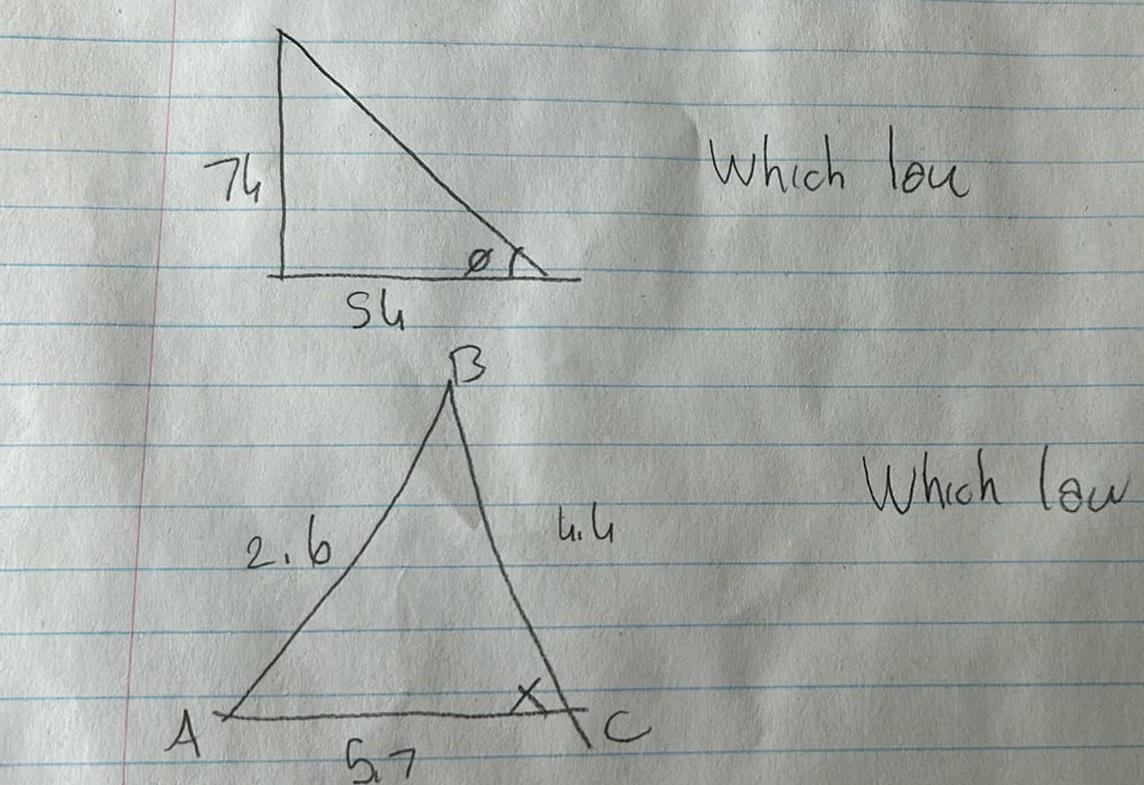

High School Calculus
Differential equationsa f x 1 M 2 1 0 1 1 Find g 2 g 2 and g 2 2 5 6 7 8 The function f is continuous on the interval 2 7 and consists of three line segments and a semi circle as shown in the figure above The function g is defined by g x d Let h x f 5x 9 Find h 3 2 X J f t dt 2 b Find the x coordinate of the critical points on g x on the interval 2 7 Classify each critical point found in part b as a relative minimum relative maximum or neither for g Justify your answers c Find the interval s where g is increasing and concave down Justify your answer
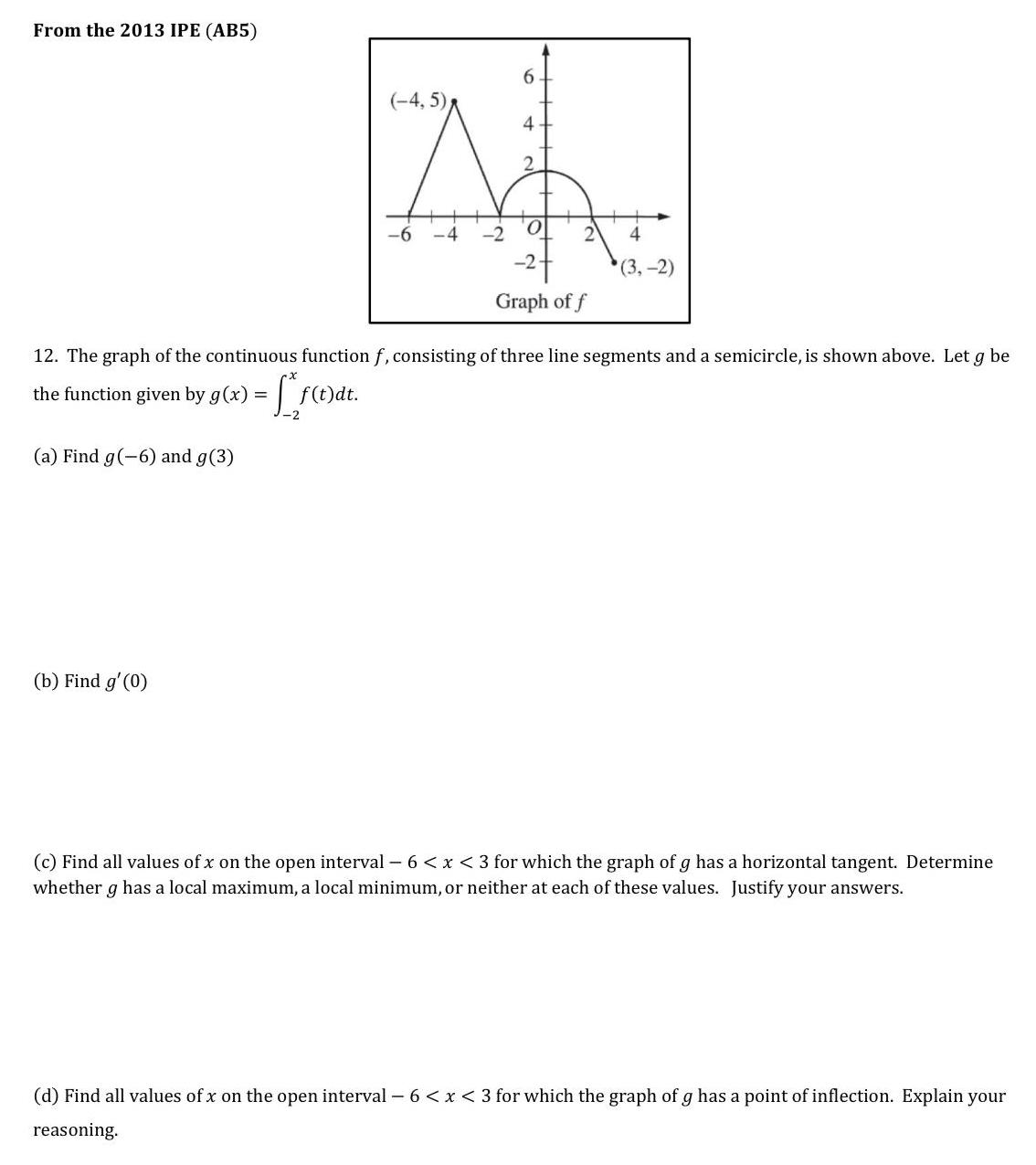
High School Calculus
Indefinite IntegrationFrom the 2013 IPE AB5 a Find g 6 and g 3 6 4 5 4 M 6 Graph of f 12 The graph of the continuous function f consisting of three line segments and a semicircle is shown above Let g be the function given by g x L f t dt b Find g 0 4 3 2 c Find all values of x on the open interval 6 x 3 for which the graph of g has a horizontal tangent Determine whether g has a local maximum a local minimum or neither at each of these values Justify your answers d Find all values of x on the open interval 6 x 3 for which the graph of g has a point of inflection Explain your reasoning
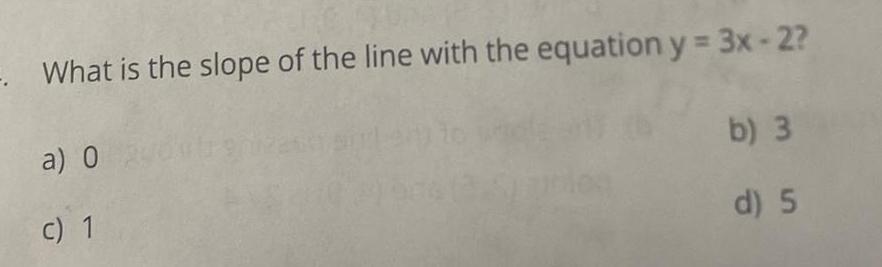
High School Calculus
Indefinite IntegrationWhat is the slope of the line with the equation y 3x 2 a 0 c 1 b 3 d 5


High School Calculus
Vector CalculusWrite and simplify a polynomial with 4 31 and 0 multiplicity 2 as roots Write yo

High School Calculus
Application of derivativesSuppose that 28 000 from a retirement account is invested in a large cap stock fund After 20 years the value is 86 000 Use the model A Pertto determine the average rate of return under continuous compounding Round to three decimal places Avoid rounding in intermediate steps Your Answer

High School Calculus
Application of derivativesPertor A P 1 nt to solve the following Use the model A Pertor A problem 45 000 is invested at 3 1 compounded monthly How long will it take for the investment to double Round to the nearest tenth of a year
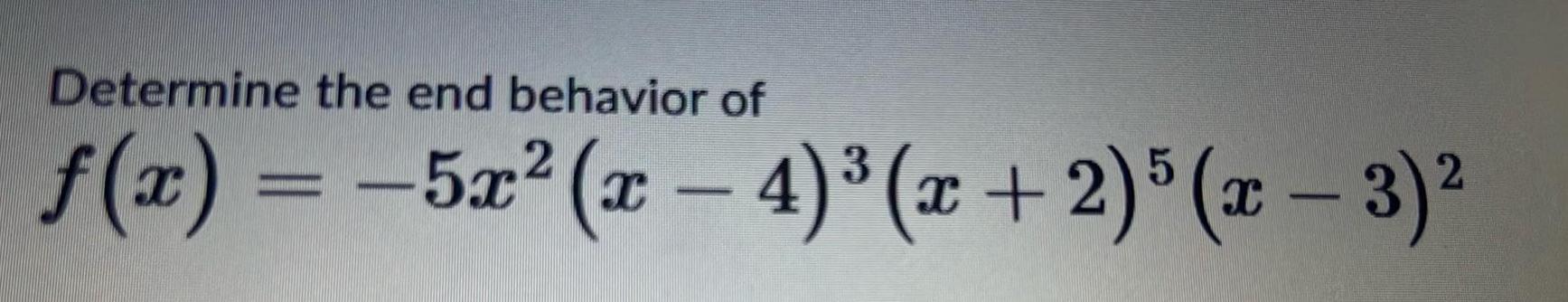

High School Calculus
Vector CalculusAmerica s dependency on foreign oil has always been a hot political topic with the amount of imported oil fluctuating over the years due to political climate public awareness the economy and other factors The amount of crude oil imported can be approximated by the function given where x represents the year since 1980 and A x represents the number of barrels imported in billions Give your answer to the nearest hundredth If the hundredths place is a zero please include it A x is listed below 0 044x 2 0 369x 1 5 0 x 8 8 x What was the minimum number of barrels imported between 1980 and 1988 0 072x 2 1 496x 1 5

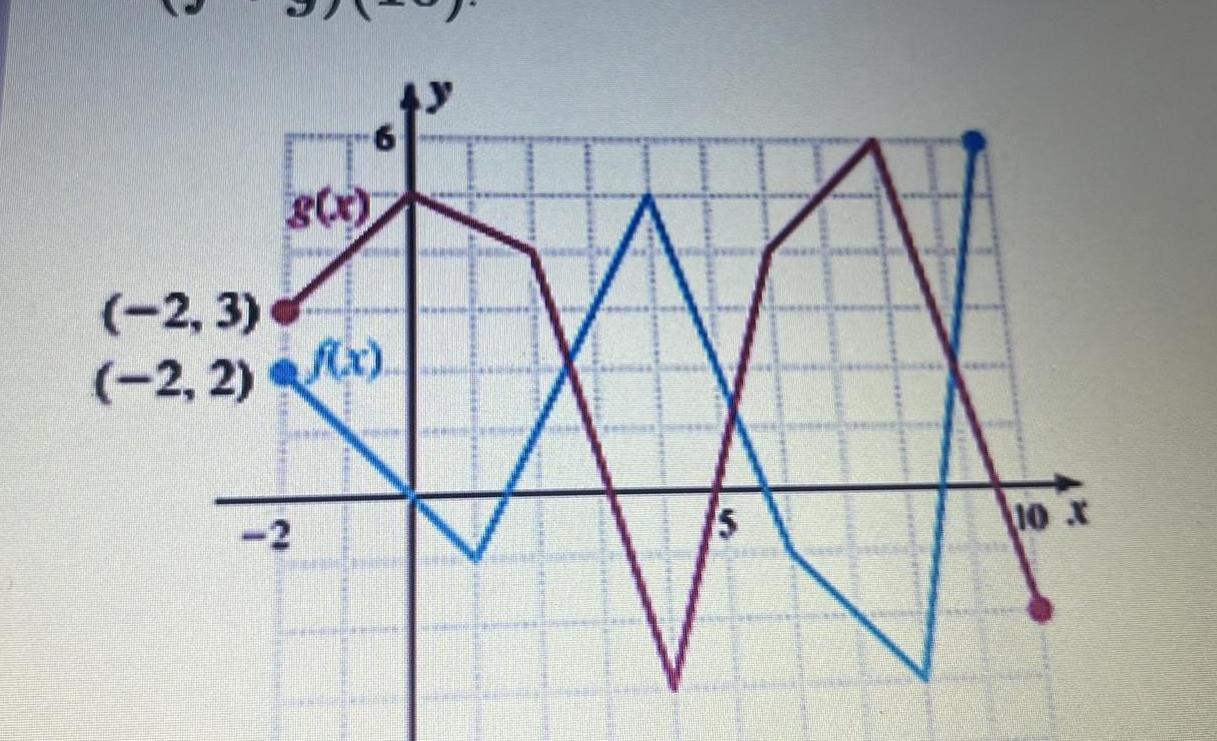
High School Calculus
Application of derivativesA polynomial of degree 12 may have at most relative extreme values maximums and minimums 12 A roots and at most

High School Calculus
Differentiation3 Water is dripping through the bottom of a conical coffee filter 4 inches across and 6 inches deep Given that the filter loses half a cubic inch of coffee per minute how fast is the level of the liquid dropping when it is 3 inches deep

High School Calculus
Differentiation2 A 19 foot ladder is being pushed up a building at a constant rate of 2 feet per minute How fast is the base of the ladder moving toward the building when the base of the ladder is q feet from the building

High School Calculus
Differentiation1 A stone dropped into a sill pond sends out a circular ripple whose radius increases at a constant rate of 4 ft s How rapidly is the area enclosed by the ripple increasing when the radius is 2 feet

High School Calculus
Indefinite Integration4 A function f t gives the rate of evaporation of water in liters per hour from a pond where t is measured in f t dt in the context described 10 hours since 12 noon Which of the following gives the meaning of A The total volume of water in liters that evaporated from the pond during the first 10 hours after 12 noon B The total volume of water in liters that evaporated from the pond between 4PM and 10PM C The net change in the rate of evaporation in liters per hour from the pond between 4PM and 10PM D The average rate of evaporation in liters per hour from the pond between 4PM and 10PM E The average rate of of change in the rate of evaporation in liters per hour per hour from the pond between 4PM and 10PM
High School Calculus
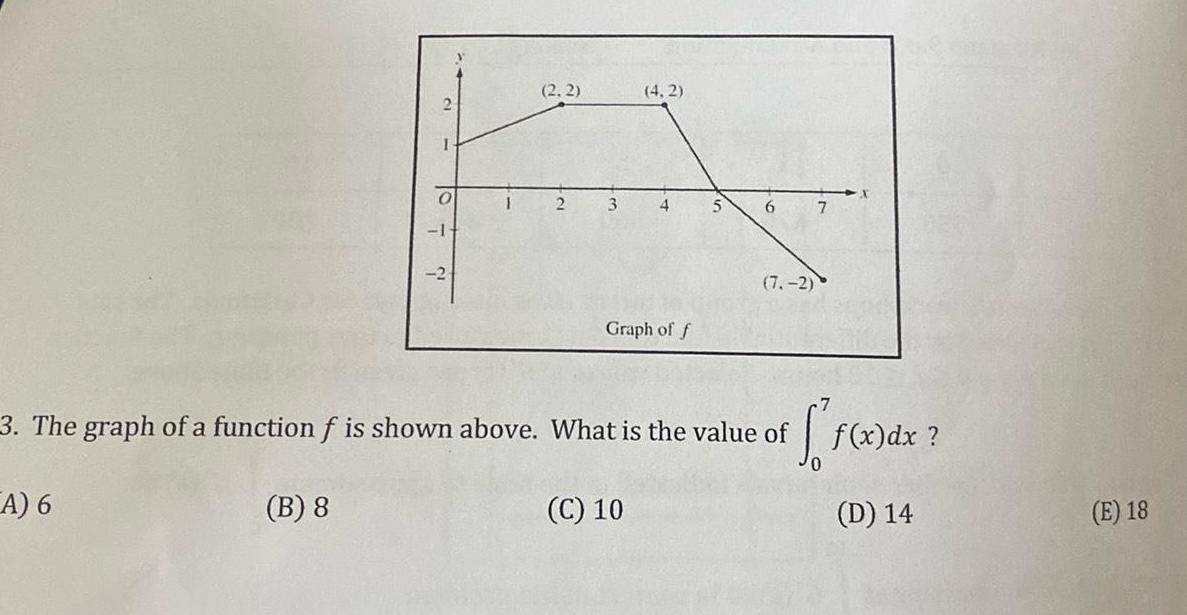
Definite Integrals2 2 2 4 2 4 Graph of f C 10 5 6 7 2 3 The graph of a function f is shown above What is the value of S A 6 B 8 f x dx D 14 E 18

High School Calculus
Limits & ContinuitySolve the logarithmic equation algebraically Approximate the result to three decimal places If there is no solution enter NO SOLUTION X 16 In x 8


High School Calculus
Vector Calculus8 In order for y sin to be a function the domain of y sina is restricted to a Explain why the domain was restricted to this interval b Identify two other intervals that could have been chosen as the domain



High School Calculus
Application of derivatives3 Kymani was asked to evaluate cos cos His answer and explanation are given below 1 COS cos because the inverse cosine function undoes the cosine function so the output must be the same as the original input Do you agree with his answer and explanation Explain

High School Calculus
Application of derivatives3 Which of the following pairs of functions have the same range Choose all that apply 1 f x sinx and g x cos x II f x tan x and g x arctan x III f x arcsin x and g x arctan x 1 IV f x cos a and g x sin x