Math Questions
The best high school and college tutors are just a click away, 24×7! Pick a subject, ask a question, and get a detailed, handwritten solution personalized for you in minutes. We cover Math, Physics, Chemistry & Biology.

Math
Straight linesIndicate the equation of the given line in standard form, writing the answer in the equation box below. The line containing the altitude to the hypotenuse of a right triangle whose vertices are P(-1, 1), Q(3, 5), and R(5,-5).
Math
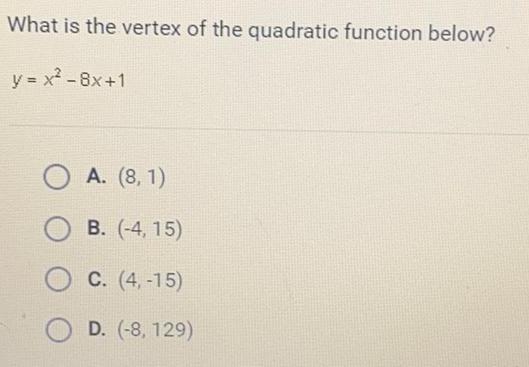
Basic MathWhat is the vertex of the quadratic function below?
y = x² - 8x+1
A. (8, 1)
B. (-4, 15)
C. (4, -15)
D. (-8, 129)
Math
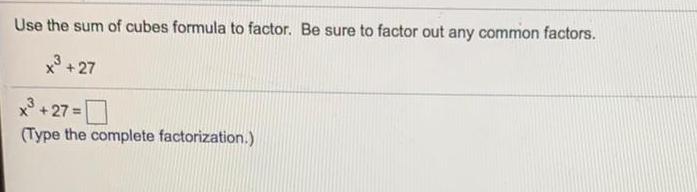
Basic MathUse the sum of cubes formula to factor. Be sure to factor out any common factors.
x³+27
Math
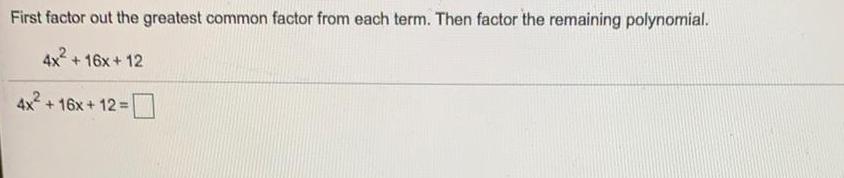
Basic MathFirst factor out the greatest common factor from each term. Then factor the remaining polynomial.
4x² + 16x+12
4x² + 16x + 12 =
Math
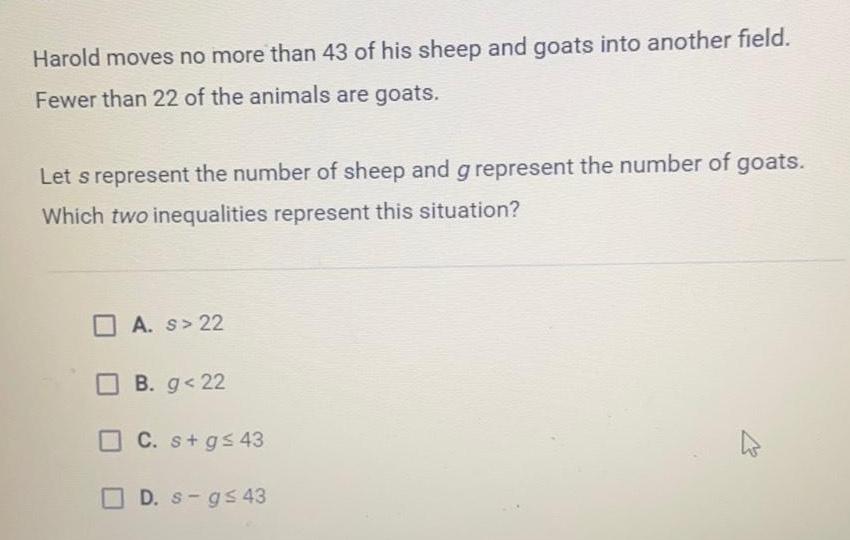
Basic MathHarold moves no more than 43 of his sheep and goats into another field. Fewer than 22 of the animals are goats.
Let s represent the number of sheep and g represent the number of goats. Which two inequalities represent this situation?
A. s> 22
B. g <22
C. s+ g≤ 43
D. s-gs 43
![If P = (5,4), find the image
of P under the following rotation.
180° counterclockwise about the origin
([?], [ ])
Enter the number that belongs in
the green box.
Enter](https://media.kunduz.com/media/sug-question/raw/51158698-1658398089.4972303.jpeg?w=256)
Math
Straight linesIf P = (5,4), find the image
of P under the following rotation.
180° counterclockwise about the origin
([?], [ ])
Enter the number that belongs in
the green box.
Enter

Math
FunctionsWhat are the domain and range of the function represented by the set of
ordered pairs?
{(-13, 9), (-7,-5), (5, 14), (11, -10)}
A. Domain: {-13, -7, 5, 11}
Range: (-10,-5, 9, 14)
B. Domain: {-10, -5, 9, 14)
Range: (-13, -7, 5, 11)
C. Domain: -13 ≤x≤ 11
Range: -10y≤ 14
D. Domain: -10 ≤ x ≤ 14
Range: -13 sys11

Math
Basic MathGrace bought a television for $329 and some DVDs for $5.75 each. Write an equation to find out how many DVDs Grace bought if she spent a total of $363.50.
329 +5.75m = 363.50

Math
Basic MathStacy is building a candy house. She worked on it 3 hours before lunch and 6 hours after lunch. How many total hours did Stacy spend working on the candy house?
Give your answer as a mixed number in simplest form. Enter the number that belongs in the green box.

Math
Basic MathThere is a spinner with 11 equal areas, numbered 1 through 11. If the spinner is spun one time, what is the probability that the result is a multiple of 2 or a multiple of 5?
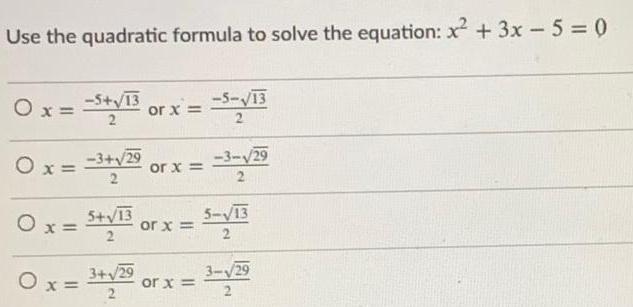
Math
FunctionsUse the quadratic formula to solve the equation: x² + 3x - 5 = 0
x = -5 + √13/2 or x = -5-√13/2
x = -3+√29/2 or x = -3-√29/2
x = 5+√13/2 or x = 5-√13/2
x = 3+√29/2 or x = 3-√29/2

Math
Straight linesIndicate the equation of the given line in standard form, in the equation box below.
The line through point (-3, 4) and perpendicular to a line that has slope 2/5
Math
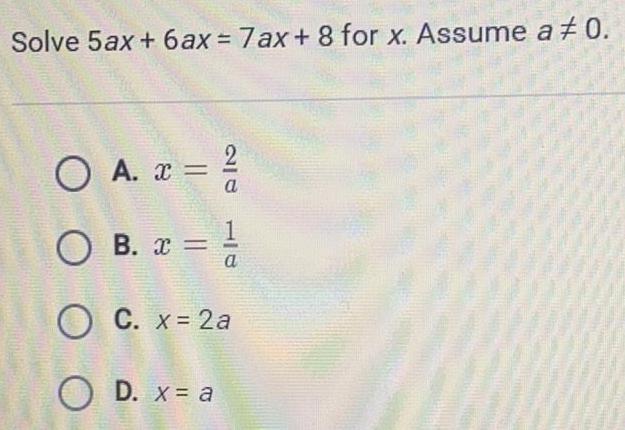
Basic MathSolve 5ax + 6ax = 7ax+8 for x. Assume a‡0.
O A. x = 2/a
O B. x = 1/a
O C. x = 2a
O D. x = a

Math
TrigonometryAn airplane ascends at an angle of elevation of 22" for a ground distance of 7850 feet. What height does the plane gain during this ascent? Draw a picture on paper to support your answer, and round your answer to the nearest whole foot.

Math
Basic MathThe circumference of a sphere was measured to be 86 cm with a possible error of 0.5 cm. Use linear
approximation to estimate the maximum error in the calculated surface area.
Estimate the relative error in the calculated surface area.
Math
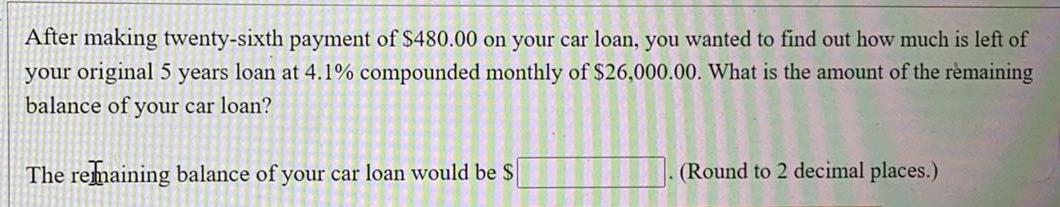
Basic MathAfter making twenty-sixth payment of $480.00 on your car loan, you wanted to find out how much is left of your original 5 years loan at 4.1% compounded monthly of $26,000.00. What is the amount of the remaining balance of your car loan?
The remaining balance of your car loan would be $ (Round to 2 decimal places.)

Math
ProbabilityMandy flipped a coin 50 times. The
coin landed on tails 20 times. What
was the experimental probability for
landing on tails?
A 3/5
B 2/5
C 4/5
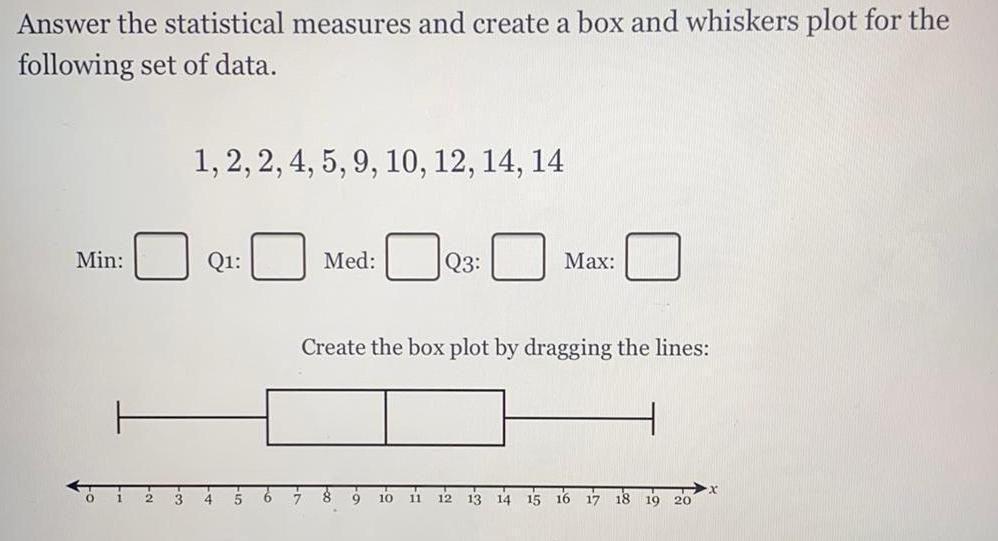
Math
StatisticsAnswer the statistical measures and create a box and whiskers plot for the
following set of data.
Min:
1, 2, 2, 4, 5, 9, 10, 12, 14, 14
Q1:
Med:
Q3:
Max:
Create the box plot by dragging the lines:

Math
Quadratic equationsThe following is the student's incorrect work in solving a quadratic using the zero product property. Explain the student's mistake.
Step 1: x²-3x - 4 = 8
Step 2: (x-4) (x + 1) = 8
Step 3: x 4 = 8 or x + 1 = 8
Step 4: x= 12 or x = 7
-

Math
Basic MathWhich of the following options is a 3rd degree polynomial with exactly 1 real root?
A. F(x)= x+3x²-9x-27
B. F(x)= x +9x² +27x+27
C. F(x)=x²-9x² +27x-27
D. F(x)= x+3x² +9x+27

Math
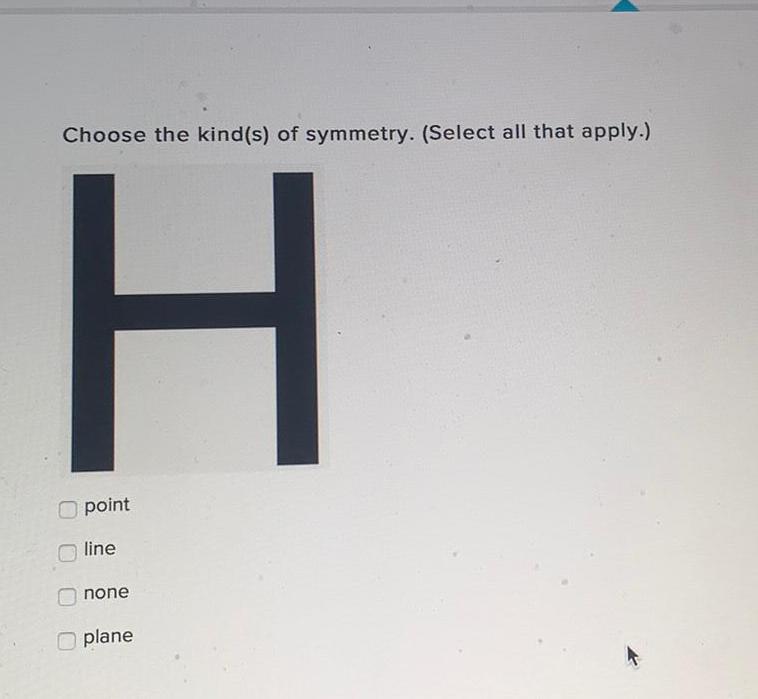
Basic MathName the kind or kinds of symmetry the following figure has: point, line, plane, or none. (Select all that
apply.)
2
line
point
plane
none

Math
Basic MathGiven that f(x) = x² - 13x + 36 and g(x) = x-9, find (f+g)(x) and express the result in standard form.

Math
VectorsFind the angle between the vectors (7, 2) and (9, -3). Carry your intermediate computations to at least 4 decimal places. Round your final answer to the nearest degree.
![Suppose a point has polar coordinates (3, π/2) with the angle measured in radians.
Find two additional polar representations of the point.
Write each coordinate in simplest form with the angle in [-2π, 2π].](https://media.kunduz.com/media/sug-question/raw/51131534-1658397805.042526.jpeg?w=256)
Math
Basic MathSuppose a point has polar coordinates (3, π/2) with the angle measured in radians.
Find two additional polar representations of the point.
Write each coordinate in simplest form with the angle in [-2π, 2π].
![Consider the function
f(x)=√2-x+7 for the domain (-∞, 2].
Find f(x), where
is the inverse of f.
1
Also state the domain off in interval notation.](https://media.kunduz.com/media/sug-question/raw/51003855-1658397798.8849702.jpeg?w=256)
Math
Basic MathConsider the function
f(x)=√2-x+7 for the domain (-∞, 2].
Find f(x), where
is the inverse of f.
1
Also state the domain off in interval notation.

Math
Linear ProgrammingWhen using the rational root theorem, which of the following is a possible root of the polynomial function below?
F(x) = 3x³x² + 4x +5
A. -5/3
B. -7
O C. 4/3
D. 6

Math
Functionsf(x)=√x - 11. Find the inverse of f(x) and its domain.
A. f¹(x) = x² +11; x2-11
B. ƒ ¹ (x) = (x + 11) ² ; x ≥ 0
C. ƒ ¹ (x) = x² + 11; x ≥ 0
D. f¹(x) = (x +11)²; x2-11
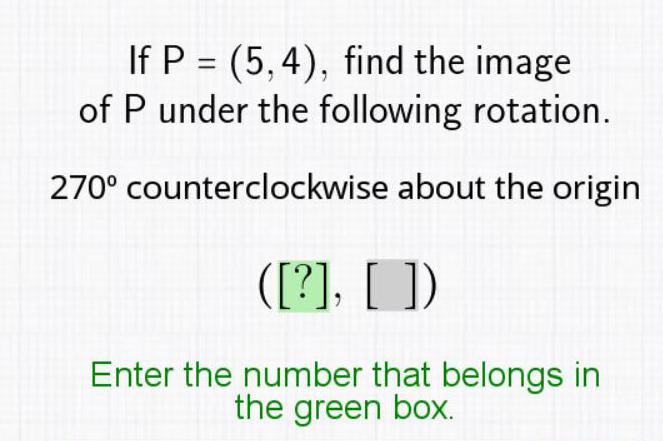
Math
Straight linesIf P (5,4), find the image. of P under the following rotation. 270° counterclockwise about the origin

Math
Basic MathFind the value of Q in the following system so that the solution to the system
is (4,2).
3x - 2y = 8
2x+3y = Q
A. 14
B. 10
C. 12
D. 8
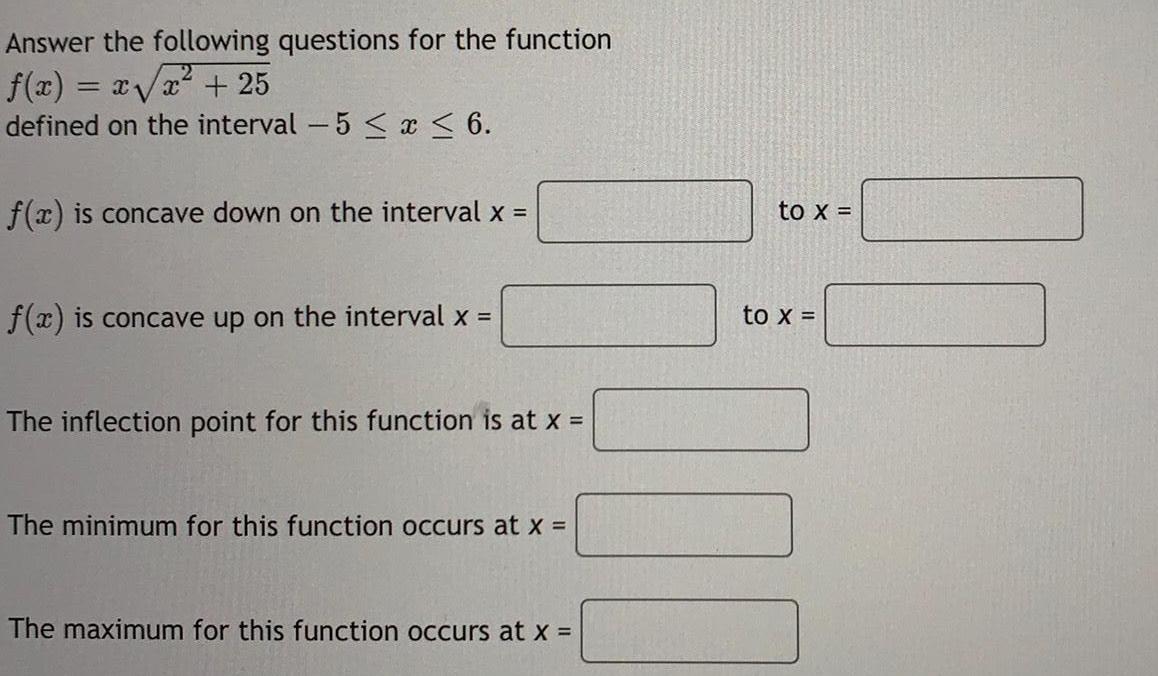
Math
Application of derivativesAnswer the following questions for the function
f(x)=x√x² + 25
defined on the interval -5 ≤ x ≤ 6.
f(x) is concave down on the interval x =
f(x) is concave up on the interval x =
The inflection point for this function is at x =
The minimum for this function occurs at x =
The maximum for this function occurs at x =
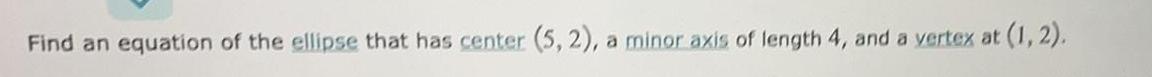
Math
EllipseFind an equation of the ellipse that has center (5, 2), a minor axis of length 4, and a vertex at (1,2).
Math
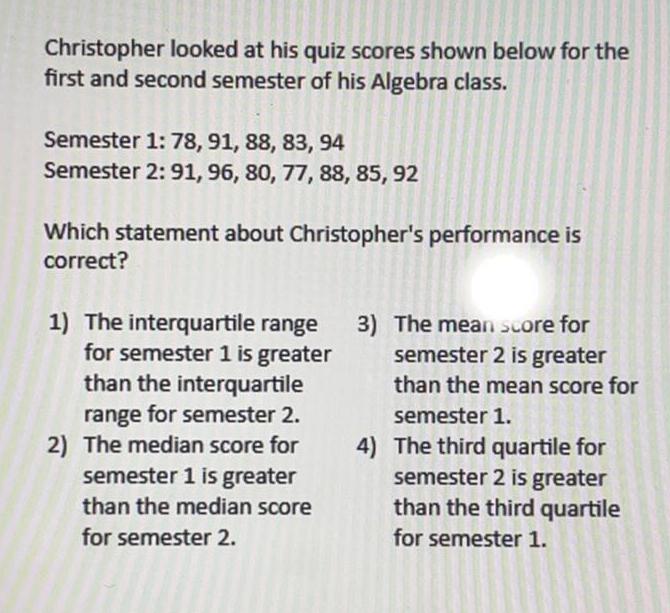
StatisticsChristopher looked at his quiz scores shown below for the
first and second semester of his Algebra class.
Semester 1: 78, 91, 88, 83, 94
Semester 2: 91, 96, 80, 77, 88, 85, 92
Which statement about Christopher's performance is
correct?
1) The interquartile range 3) The mean score for
for semester 1 is greater semester 2 is greater
than the interquartile
than the mean score for
range for semester 2.
semester 1.
2) The median score for
semester 1 is greater
than the median score
for semester 2.
4) The third quartile for
semester 2 is greater
than the third quartile
for semester 1.

Math
FunctionsWhich pair of functions are inverses of each other?
A. f(x) = 7/x - 2 and g(x) = x+2/7
B. f(x) = x/7 + 10 and g(x) = 7x - 10
C. f(x) = 9x - 6 and g(x) = x-6/9
D. f(x)= 3√11x and g(x) = (x/11)³

Math
Basic MathWhen Jose installs a new app on his phone, it creates a square icon on his home screen. The square starts with side lengths of 1 cm. The side lengths grow by 2.8 cm each second until the square reaches its maximum size. What is the perimeter of the square after 1.5 seconds? Recall that the perimeter of a shape is the sum of its side lengths.
16.8 centimeters
19.2 centimeters
20.8 centimeters
29.2 centimeters
![Find the maximum and minimum values of the function g(θ) = 2θ - 8 sin(θ) on the interval [0, π]](https://media.kunduz.com/media/sug-question/raw/51014580-1658397563.5641792.jpeg?w=256)
Math
Basic MathFind the maximum and minimum values of the function g(θ) = 2θ - 8 sin(θ) on the interval [0, π]
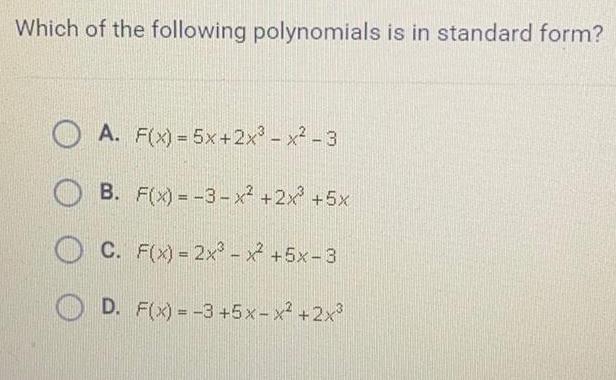
Math
Quadratic equationsWhich of the following polynomials is in standard form?
A. F(x) = 5x+2x³ - x² - 3
B. F(x) = -3-x²+2x³ +5x
C. F(x) = 2x³ -x +5x-3
D. F(x) = -3 +5x-x²+2x³

Math
ProbabilityThere are 20 chocolate candies in a bag. What is the probability of reaching in the bag and pulling out a strawberry?
A. 1, since all of the candies are chocolate, there is 100% chance of pulling out a strawberry
B. 1/2, because half of the time you will get a strawberry
C. 0, it would be impossible to pull out a strawberry because there are no strawberries in the bag
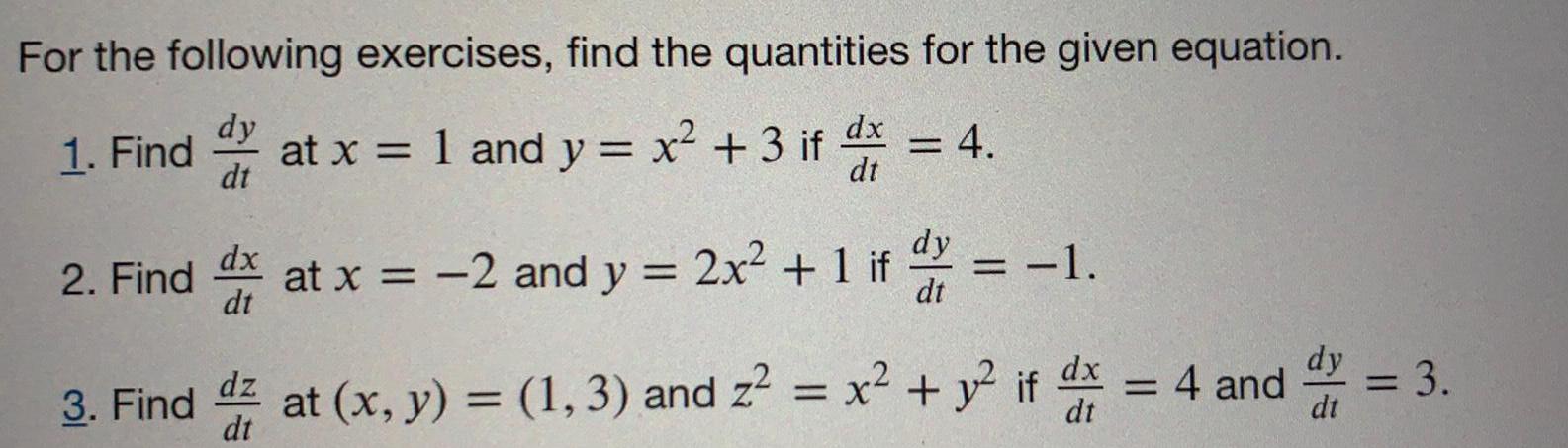
Math
DifferentiationFor the following exercises, find the quantities for the given equation.
1. Find dy/dt at x = 1 and y = x² + 3 if dx/dt = 4
2. Find dx/dt x = -2 and y = 2x² + 1 if dy/dt = -1.
3. Find dx/dt at (x, y) = (1, 3) and z² = x² + y² if dx/dt = 4 and dy/dt= 3.

Math
VectorsFind the angle between the vectors (-9, -8) and (-8, 7). Carry your intermediate computations to at least 4 decimal places. Round your final answer to the nearest degree.
![Suppose a point has polar coordinates (-4, -11π/6) with the angle measured in radians.
Find two additional polar representations of the point.
Write each coordinate in simplest form with the angle in [-2π, 2π].](https://media.kunduz.com/media/sug-question/raw/51132510-1658397392.955185.jpeg?w=256)
Math
Basic MathSuppose a point has polar coordinates (-4, -11π/6) with the angle measured in radians.
Find two additional polar representations of the point.
Write each coordinate in simplest form with the angle in [-2π, 2π].

Math
Basic MathWhat is the first step when factoring the trinomial ax²+bx+c?
A. Look for a common factor in each term.
B. Factor the simplified trinomial.
C. List the factors of the leading coefficient.
D. List the factors of the constant term.

Math
Basic MathLine j passes through the points (0, 0) and (c, d). Line k passes through the points (0,0) and (-d, c). Lines j
and k are
perpendicular
neither
parallel

Math
Application of derivativesConsider the function f(x) = 8(x - 2)^2/3. For this function there are two important intervals: (-∞, A)
and (A, ∞) where A is a critical number.
A is
For each of the following intervals, tell whether f(x) is increasing or decreasing.
(-∞, A):
(A, ∞):
For each of the following intervals, tell whether f(x) is concave up or concave down.
(-∞, A):
(A, ∞):

Math
Basic MathLine m passes through the points (-4, 3) and (-4, 7). What is the slope of the line that is perpendicular to
line m?
-4
undefined
zero

Math
Basic MathRachel spent $24.15 on vegetables. She bought 2 lbs of onions, 3 lbs of carrots,
and 1 1/2 lbs of mushrooms. If the onions cost $3.69 per lb, and the carrots cost $
4.29 per lb, what is the price per lb of mushrooms?
a. $2.60
b. $2.25
c. $2.80
d. $3.10
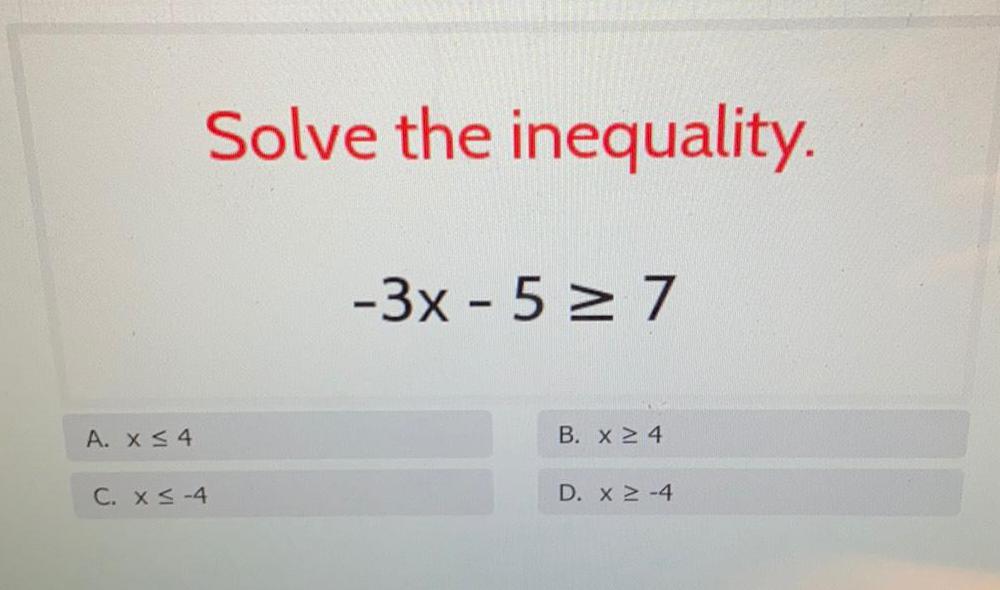
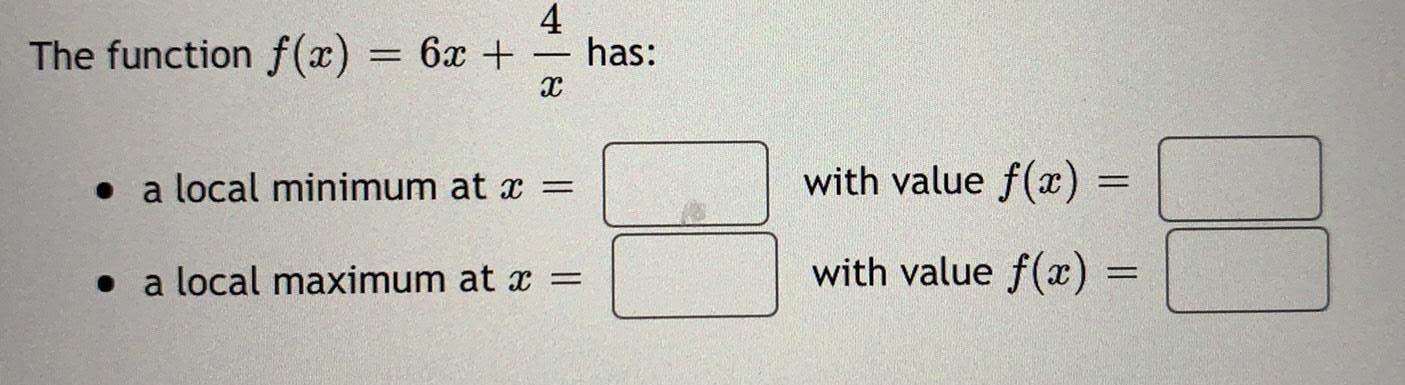
Math
Application of derivativesThe function f(x) = 6x + (4/x) has:
• a local minimum at x = with value f(x) =
• a local maximum at x = with value f(x) =

Math
Linear ProgrammingSolve the quadratic equation using completing the square: x² - 12x + 23 = 0
x=-6+ √59 or x = -6-√√/59
x=6+ √13 or x = 6 - √13
x = 6 + √59 or x = 6 -√√59
x=-6+√13 or x = -6 -√13

Math
DifferentiationGiven that 26x⁹ + 5x³⁹ y + y^8
dy/dx
Now find an equation of the line tangent to the graph of 26x9 + 5x³9 y + y = 32 at (1, 1):