Math Questions
The best high school and college tutors are just a click away, 24×7! Pick a subject, ask a question, and get a detailed, handwritten solution personalized for you in minutes. We cover Math, Physics, Chemistry & Biology.

Math
FunctionsThe function f(x)=x+4 is one-to-one.
Find an equation for f-1(x), the inverse function.
(Type an expression for the inverse. Use integers or fractions for any numbers in the expression.)

Math
EllipseAn arch in the shape of the upper half of an ellipse is used to support a bridge that is to span a river 20 meters wide. The center of the arch is 6 meters above the center of the river. Write the equation for this ellipse.

Math
ProbabilityA bag has 2 red balls, 4 blue balls, and 3 yellow balls. Two balls are taken out of the bag 1 at a time. Is each statement True or False?
A The probability of drawing 2 blue balls is 1/3.
True False
B If the first ball drawn is red, the probability that the second ball is also red is 1/36.
True False
C The probability of drawing a blue ball and then a red ball is 1/9.
True False

Math
Basic MathAn observer standing on the top of a 360 ft cliff spots a boat at an angle of depression of 28°. How far is the boat from the base of the cliff? Round your answer to the nearest tenth. Distance from the base of the cliff =

Math
StatisticsIn a board game, players take turns spinning a wheel with four spaces and values of $100, $300, $400, $800. The probability of landing on $100 is 4/9. The probability of landing on $300 is 2/9. The probability of landing on $400 is 2/9. The probability of landing on $800 is 1/9. What is the expected value of spinning the wheel once?

Math
StatisticsThe unemployment rate in a city is 14%. If 7 people from the city are sampled at random, find the probability that fewer than 3 of them are unemployed. Carry your intermediate computations to at least four decimal places, and round your answer to two decimal places. (If necessary, consult a list of formulas.)

Math
Basic MathTwo pools are being filled with water. To start, the first pool had 819 liters of water and the second pool was empty. Water is being added to the first pool at a rate of 18 liters per minute. Water is being added to the second pool at a rate of 39 liters per minute.
Let x be the number of minutes water has been added.

Math
ProbabilityThe probability that Gary and Jane have a child with blue eyes is 0.4, and the probability that they have a child with blond hair is 0. 25. The probability that they have a child with both blue eyes and blond hair is 0. 1. Given this information, the events blue eyes and blond hair are 1: dependent
II: independent
III: mutually exclusive
(A) I, only
(B) II, only
(C) I and III
(D) II and III

Math
Permutations and CombinationsA lottery system uses six digits. The possible entries in these digits are the numbers 1, 2, 3, 4, or letters n, m, o, q, s, w, and z. The numbers or letters drawn can be repeated. What is the total number of lottery sequences that can be made?
823,543
1,771,561
1,000,000
121,745

Math
Basic MathOver time, the value of the property at 397 West Lake Street increased by 215%. If the initial value of the property was P, what expression represents the property's current value? Why?
315 P
215 P
3.15 P
2.15 P

Math
Basic MathThere were 1200 teams competing in the first annual world math tournament. After each round, the number of teams are cut in half.
a=
a. Find the a and the b for this problem.
b =
b. Write the equation that models this situation.
C.
How many teams will be left after 4 rounds?
d. How many teams will be left after 8 rounds?
y =
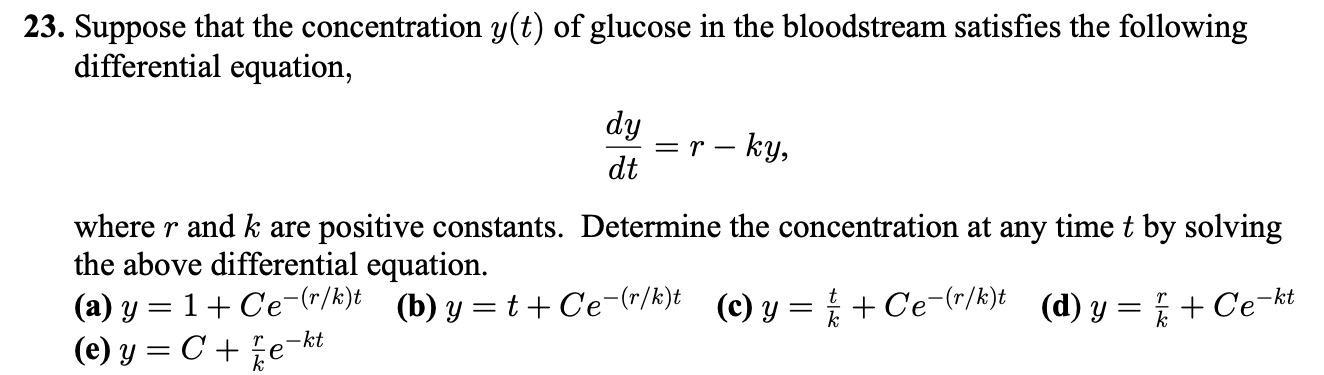
Math
Differential equationsSuppose that the concentration y(t) of glucose in the bloodstream satisfies the following
differential equation,
dy/dt =r-ky,
where r and k are positive constants. Determine the concentration at any time t by solving
the above differential equation.
(a) y = 1+ Ce(r/k)t (b) y=t+Ce-(r/k)t (c) y = // + Ce(r/k)t (d) y = / + Ce-kt
(e) y = C + e-kt

Math
EllipseConvince me that the equation of an ellipse that has foci at (2,0) and (-2,0) and a major axis length of 6 has an equation of x^2/9 + y^2/5 = 1

Math
Basic MathSuppose the statement below is true: "John wen to the gym or John wen to the bank." What can be said about the statement J = John went to the gym.
insufficient information
false
true
justified

Math
Basic MathThe cooking time for a mini-loaf of bread is 5 minutes longer than half the time it takes to bake a regular-sized loaf of bread. If it takes 25 minutes to bake a mini-loaf, how long does it take to bake a regular-sized loaf?
10 minutes
20 minutes
30 minutes
40 minutes

Math
Basic MathAn empty ice cream cone has a height of 3.02 inches and a radius of 0.98 inches. What is the approximate volume of ice cream it will hold within the cone (not h over)?
3 in³
1 in ³
12 in³
9 in³

Math
LogarithmsLet P(t) = 30000 (1.03)t be the population of a town t years after the year 2000.
Estimate in which year the population will reach 32782.
Year =

Math
Basic MathThe graph of the function f(x) = e-* - 5 can be obtained from the graph of g(x) = e² by one of the following actions:
(a) reflecting the graph of g(x) in the x-axis;
(b) reflecting the graph of g(x) in the y-axis;
your answer is (input a or b)
then, by one of the following actions:
(a) shifting the resulting graph to the right 5 units;
(b) shifting the resulting graph to the left 5 units;
(c) shifting the resulting graph upward 5 units;
(d) shifting the resulting graph downward 5 units;
Your answer is (input a, b, c, or d)
Is the domain of the function f(x) still (
Your answer is (input Yes or No)
The range of the function f(x) is (A, ∞),
the value of A is ∞, ∞)?

Math
Basic MathJaquin hopes to earn $700 in interest in 3.6 years time from $28,000 that he has available to invest. To decide if it's feasible to do this by investing in an account that
compounds semi-annually, he needs to determine the annual interest rate such an account would have to offer for him to meet his goal. What would the annual rate of
interest have to be? Round to two decimal places.

Math
Basic MathMarsha gave the cashier $20 to pay for 3 pairs of socks. The cashier gave her $5.03 in change. Each pair of socks cost the same amount. What is the cost in dollars and cents for each pair of socks?


Math
StatisticsThere were approximately 6.08 billion people in the world in the year 2000. The population grew about 1.23% each year since. Write an exponential function and use it to estimate the world population today.

Math
Basic MathSally worked 7 hours on Monday, 6 hours on Tuesday, 6.75 hours on Wednesday, 5.75 hours on Thursday, and 3.5 hours on Friday. If she is paid $10.18 per hour. Find her gross earnings for the week.

Math
VectorsTo avoid a storm, a pilot leaves airport A and flies at a bearing of 172°. He travels for one
and one-half hours at a speed of 650 mph. At the end of the one and one-half hours, the pilot
changes direction and flies at a bearing of 68° for two hours at the same speed. At the end of
the two hours, he is at point B, and is now able to continue with a normal flight plan since he
has passed the storm. If there had been no storm, the pilot would have flown directly from
Airport A to point B.
How much further did the plane have to travel because of the storm?
b. What is the bearing of the normal flight plan (from A to B)?

Math
StatisticsGiven the linear correlation coefficient r and the sample size n, determine the critical
values of r and use your finding to state whether or not the given r represents a
significant linear correlation. Use a significance level of 0.05.
r = 0.539, n = 25
Critical values: r = 0.487, no significant linear
correlation
Critical values: r = 0.396, significant linear
correlation
Critical values: r = 0.487, significant linear
correlation
Critical values: r = 0.396, no significant linear
correlation
![5. A sequence is defined by a₁ = 1, an+1 =1/2(an+3). Which of the following statements is
true?
(a) {an} is increasing and bounded above by.
(b) {an} is decreasing and bounded below by 1.
(c) {an] is decreasing and bounded below by 2.
(d) {an} is decreasing and bounded above by 2.
(e) {an} is increasing and bounded above by 3.](https://media.kunduz.com/media/sug-question/raw/43511503-1658251516.821821.jpeg?w=256)
Math
Sequences & Series5. A sequence is defined by a₁ = 1, an+1 =1/2(an+3). Which of the following statements is
true?
(a) {an} is increasing and bounded above by.
(b) {an} is decreasing and bounded below by 1.
(c) {an] is decreasing and bounded below by 2.
(d) {an} is decreasing and bounded above by 2.
(e) {an} is increasing and bounded above by 3.

Math
Heights and DistancesA kite string is being held 3 ft above the ground. The string is 185 ft long and is flying at an angle of elevation of 36°. Round your answer to the nearest tenth.
Height of the the kite =

Math
Basic MathThe harmonic motion function d (t) = 5cos(2t), in which dis measured in centimeters and t is measured in seconds, gives the distance a swinging pendulum is from its resting position at time t. How far was the pendulum from its resting position when the motion began? What is the period of the pendulum's motion? How far is the pendulum from its resting position after 7.5 seconds?

Math
Mathematical ReasoningA food truck sells salads for $6.50 each and drinks for $2.00 each. The food truck's revenue from selling a total of 209 salads and drinks in one day was $836.50. How many salads were sold that day?
77
93
99
105

Math
Basic MathCarter Motor Company claims that its new sedan, the Libra, will average better than 31 miles per gallon in the city. Assuming that a hypothesis test of the claim has been conducted and that the conclusion is to reject the null hypothesis, state the conclusion in nontechnical terms.
There is not sufficient evidence to support the claim that the mean is less than 31 miles per gallon.
There is not sufficient evidence to support the claim that the mean is greater than 31 miles per gallon.
There is sufficient evidence to support the claim that the mean is less than 31 miles per gallon.
There is sufficient evidence to support the claim that the mean is greater than 31 miles per gallon.

Math
StatisticsThe mean weight of a box of cereal filled by a machine is 16.0 ounces, with a
standard deviation of 0.3 ounce. If the weights of all the boxes filled by the machine are normally distributed, what percent of the boxes will weigh less than 15.5 ounces?
Type or write all your computations to support the answer
Round your answer to 0.01%, for example, 22.22%

Math
ProbabilityIn the game of roulette, a player can place a $10 bet on the number 7 and have a 1/38 probability of winning. If the metal ball lands on 7, the player gets to keep the $10 paid to play the game and the player is awarded an additional $350. Otherwise, the player is awarded nothing and the casino takes the player's $10. Find the expected value E(x) to the player for one play of the game. If x is the gain to a player in a game of chance, then E(x) is usually negative. This value gives the average amount per game the player can expect to lose.
The expected value is $
(Round to the nearest cent as needed.)

Math
Basic MathEduardo wants to determine the fuel consumption of his vehicle. He knows that 20 L of gasoline will get him 220 km and that 11 L of gasoline will get him 115 km.
a. What is the independent variable?
b. What is the dependent variable?
c. What would two coordinates be?

Math
Basic MathA radioisotope is used as a power source for a satellite. The power output is given by the equation P = 50e t/250, where P is the power in watts and t is the time in days.
a. Find the power available after 100 days.

Math
Basic MathZachary earns $8.00 per hour. He get paid time and a half for any hours over 40 that he works in a week. If Zachary works 9 hours per day from Monday through Friday, what are his gross earnings for the week?

Math
Basic MathA farmer is comparing the number of eggs laid by two hens. The first hen laid 6 eggs per week for x weeks. The second hen laid 9 eggs per week for y weeks.
The total number of eggs laid by the first hen was greater than the total number of eggs laid by the second hen. Write an inequality showing this relationship.

Math
3D GeometryWhich statement below describes two polygons which are similar?
a) Corresponding angles and sides are congruent.
b) Corresponding angles are congruent and corresponding sides are proportional.
c) corresponding angles and sides are proportional.
d) Neither corresponding angles nor corresponding sides are congruent.

Math
ProbabilityA survey of TV viewing habits of 50 men and 50 women found the following information: Of the 50 men, 20 prefer watching baseball and 30 prefer football. Of the 50 women, 25 prefer watching baseball and 25 prefer football. Construct a data table
for this data and answer this question: Out of the entire survey, what is the probability of choosing a man who prefers
football?
1/5
3/10
2/5
1/2

Math
Basic MathThe exponential model A = 883.1 e 0.014t describes the population, A, of a country in millions, t years after 2003. Use the model to determine the population of the country in 2003. The population of the country in 2003 was million.

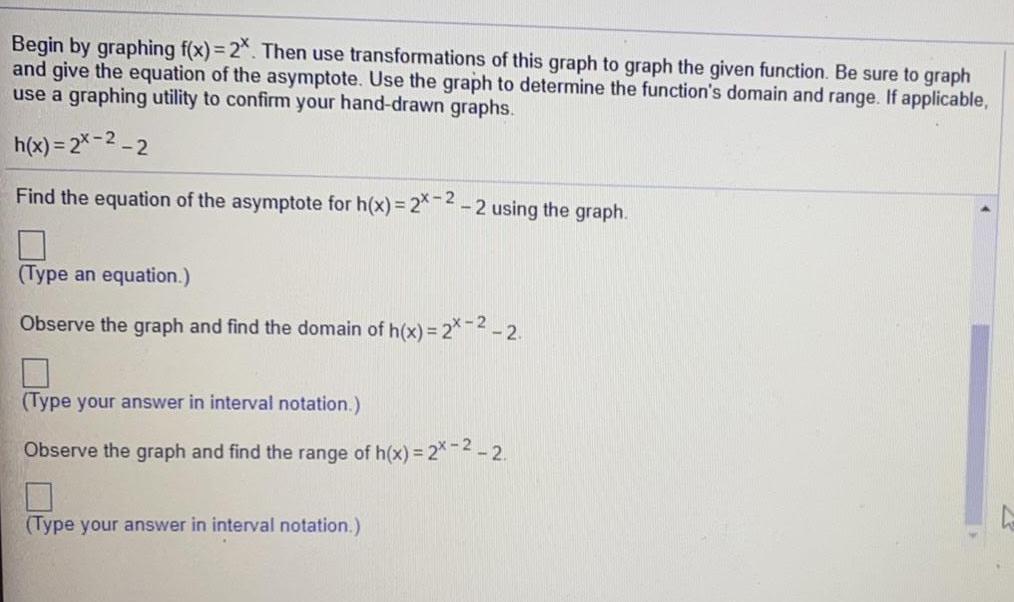
Math
FunctionsBegin by graphing f(x)=2*. Then use transformations of this graph to graph the given function. Be sure to graph and give the equation of the asymptote. Use the graph to determine the function's domain and range. If applicable, use a graphing utility to confirm your hand-drawn graphs. h(x)=2x-2-2
Find the equation of the asymptote for h(x)=2x-2-2 using the graph.
(Type an equation.)
Observe the graph and find the domain of h(x) = 2*-2 -2.
(Type your answer in interval notation.)
Observe the graph and find the range of h(x)=2x-2-2.
(Type your answer in interval notation.)

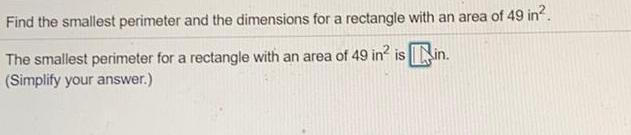
Math
Application of derivativesFind the smallest perimeter and the dimensions for a rectangle with an area of 49 in².
The smallest perimeter for a rectangle with an area of 49 in² is in. (Simplify your answer.)

Math
Basic MathSuppose there are five firms in an industry. Their sales (that is, total revenue) are as follows:
• Firm 1: $96 million
• Firm 2: $48 million
• Firm 3: $36 million
• Firm 4: $14 million
• Firm 5: $6 million
The Herfindahl-Hirschman Index (HHI) for this industry is
(Enter your response as an integer.)

Math
Basic MathGrande Prairie to Medicine Hat, Alberta, is 1013 km and takes Suzie
10 hrs and 45 minutes to drive. Suzie's SUV is travelling at a constant rate
of speed from Grande Prairie to Medicine Hat.
a. What are the independent and dependent variables?
b. How could you represent Suzie's starting coordinates and end
coordinates?

Math
Basic MathAfter a 70% reduction, you purchase a new sofa on sale for $204. What was the original price of the sofa? The original price was $

Math
Binomial theoremThe average number of cavities that thirty-year-old Americans have had in their lifetimes is 9. Do twenty-year-old have fewer cavities? The data show the results of a survey of 16 twenty-year-old who were asked how many cavities they have had. Assume that the distribution of the population is normal. 11, 8, 5, 6, 10, 9, 6, 9, 11, 9, 6, 10, 11, 7, 6, 11 What can be concluded at the a = 0.01 level of significance? Write hypothesis, give test stat and p-value. Write conclusions about HO and claim.

Math
Basic MathA delivery truck is driving along a straight road, and after, hours its distance (in miles) east of its
starting point is s = 24t² - 4t³ At time t = 2 hours, where is the truck located?
B) 64 miles
C) 48 miles
A) 96 miles
D) 100 miles

Math
Straight linesWHAT IS THE EQUATION OF THE LINE THAT PASSES THROUGH THE POINT (4.4) AND
HAS A SLOPE OF 1/47
Show your work below and put your answer in the blue box. Use
point-slope form to start.

Math
Sequences & SeriesThe bear population has a growth rate of 1.02. There are 1573 bears this year. Write a
function that models the bear population. How many bears will there be in 10 years?
a. Find the a and the b for this problem.
b. Write the equation that models this situation.
How many bears will there be in 10 years?