Math Questions
The best high school and college tutors are just a click away, 24×7! Pick a subject, ask a question, and get a detailed, handwritten solution personalized for you in minutes. We cover Math, Physics, Chemistry & Biology.

Math
Mathematical InductionCarly wants to invest $1000 in an account that earns 8% interest, compounded quarterly. How many years will it take for he account to have a value of $5000? Round to the nearest whole number.

Math
Basic MathCarly wants to invest $1000 in an account that earns 6.2% interest, compounded quarterly. How many years will it take for he account to have a value of $5000? Round to the nearest whole number
Math
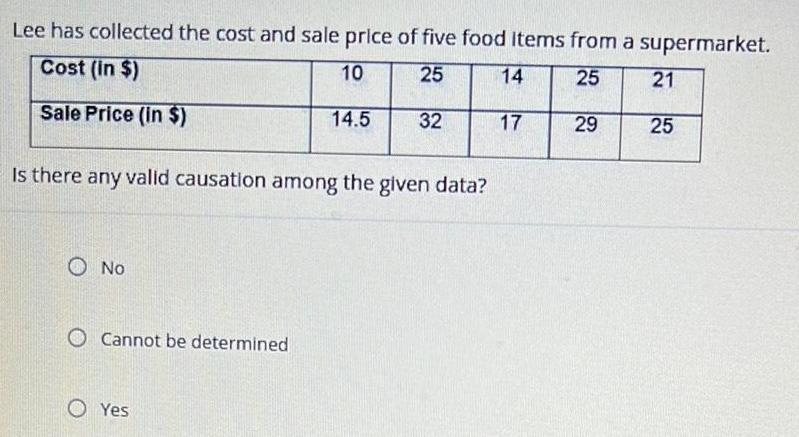
Basic MathLee has collected the cost and sale price of five food items from a supermarket. Cost (in $) 10 25 14 25 Sale Price (in $) 14.5 Yes 32 17 29 21 25
Is there any valid causation among the given data?
No
Cannot be determined
Yes

Math
Basic MathIn past years, the proportion of engineering students at the local college who did not own a
calculator was 0.15. Angelina believes the proportion of current population of engineering
students who do not own a calculator is lower. She randomly selects 55 students and finds that
0.05 and calculates a p-6 of them do not own a calculator. She uses a significance level of a -
value of 0.198. What null and alternative hypothesis did Angelina use for this test, and what
conclusion can she make? Note: The item below has been reviewed and is scheduled to be
updated. All students will receive full credit for any response to the following.
(1 point)
H0: p=15
He: p > 15
Angelina fails to reject the null hypothesis because the p-value is greater than the significance level.
H0: p=0.15
He: p<0.15
Angelina rejects the null hypothesis because the p-value is greater than the significance level.
H0: p=15
He: p<0.15
Angelina fails to reject the null hypothesis because the p-value is greater than the significance level.
H0: µ= 0.15
He: µ<0.15

Math
Basic MathHow much would you have to deposit in an account that pays an interest rate of 4.6% compounded monthly, if at the end of 10 years you want $8500 in the account? Use the TMV calculator to fill in the tal
N
1%
PV
PMT 0
FV
P/Y 12
CIY

Math
StatisticsA professor sampled 46 students from a large university to obtain a 95% confidence interval for the proportion of students in favor of raising ASB fees. The interval was (.356, .397). If the professor had used a 90% confidence interval instead, the confidence interval would have been
A Wider and would have a smaller chance of missing the true proportion.
B Wider, but the chance of missing the true proportion cannot be determined.
C Wider and would have a larger chance of missing the true proportion.
D Narrower and would have a smaller chance of missing the true proportion.
E Narrower and would have a larger chance of missing the true proportion.
Math
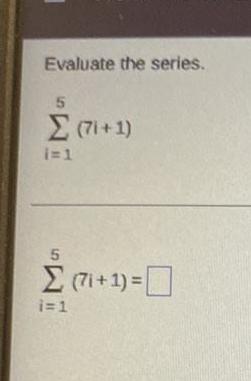
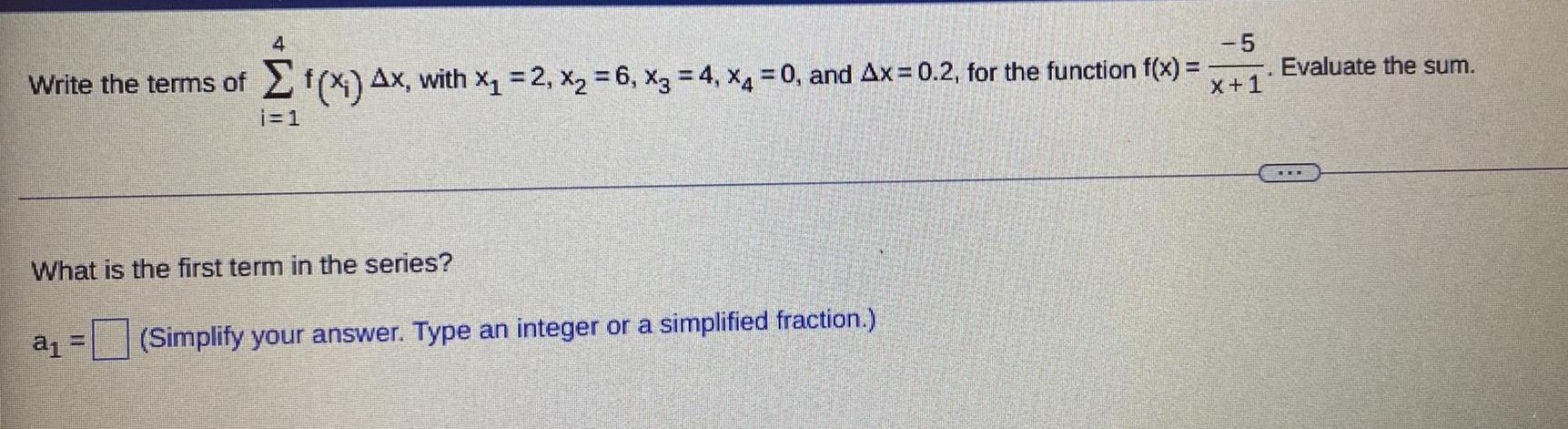
Sequences & SeriesWrite the terms of 4Σi=1 f(xi) Δx., with x₁ = 2, X₂ = 6, x3 = 4, x4 = 0, and Ax=0.2, for the function f(x) =-5/x+1
What is the first term in the series?
a₁ = (Simplify your answer. Type an integer or a simplified fraction.)
Evaluate the sum.

Math
Basic MathOn Melissa's 6th birthday, she gets a $4000 CD that earns 5% interest,
compounded semiannually. If the CD matures on her 12th birthday, how much
money will be available?
The amount available will be
(Simplify your answer. Round to the nearest cent.)

Math
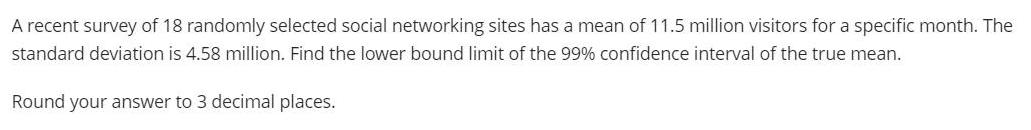
Basic MathA recent survey of 18 randomly selected social networking sites has a mean of 11.5 million visitors for a specific month. The standard deviation is 4.58 million. Find the lower bound limit of the 99% confidence interval of the true mean.
Round your answer to 3 decimal places.

Math
Basic MathData set 14 "Oscar Winner Age " in Appendix B lists ages of actresses when they won Oscars, and the summary statistics are n=87, x= 36.2 years, and s=11.5 years. Use a 0.01 significance level to test the claim that the mean age of actresses when they win Oscars is greater than 30 years.

Math
StatisticsA random sample of state gasoline taxes (in cents) is shown here for 12 states. Use the data to estimate the lower bound of the true population mean gasoline tax with 99% confidence.
38.4 40.9 67 32.5 51.5 43.4
...
38 43.4 50.7 35.4 39.3 41.4
Round your answer to 2 decimal places.
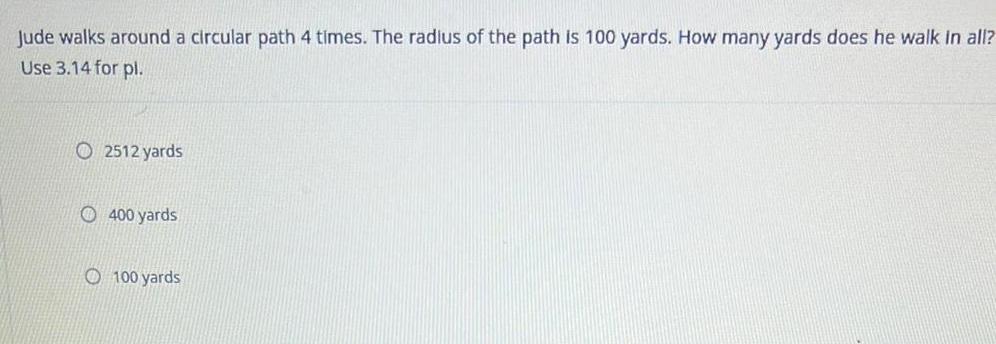
Math
CircleJude walks around a circular path 4 times. The radius of the path is 100 yards. How many yards does he walk in all?
Use 3.14 for pl.
A. 2512 yards
B. 400 yards
C. 100 yards

Math
Basic MathThe standard deviation of SAT scores is 126 points. A researcher decides to take a sample of 600 students' scores to estimate the mean score of students in your state. What is the standard deviation of the sample mean?

Math
StatisticsWhich of the following statements is false?
A Alpha is the proportion in the tails of the distribution which are outside of the confidence interval.
B) There is a different z-value (i.e., critical value) used for each level of alpha.
c) In practice, the population means is an unknown quantity that is to be estimated.
D) It is possible to construct a 100% confidence interval estimate for the population means.
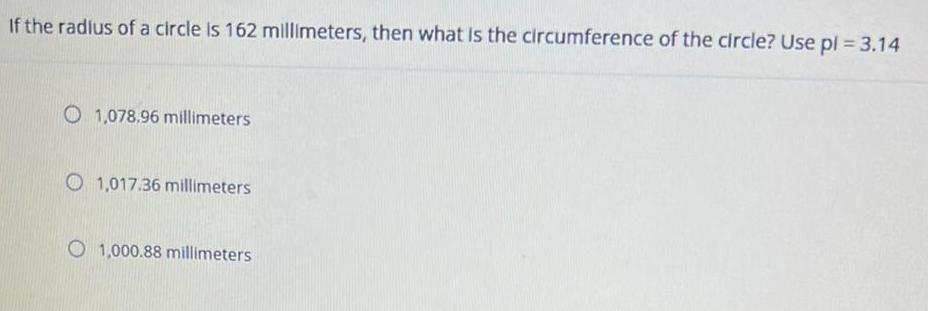
Math
CircleIf the radius of a circle is 162 millimeters, then what is the circumference of the circle? Use pl = 3.14
A. 1,078.96 millimeters
B. 1,017.36 millimeters
C. 1,000.88 millimeters
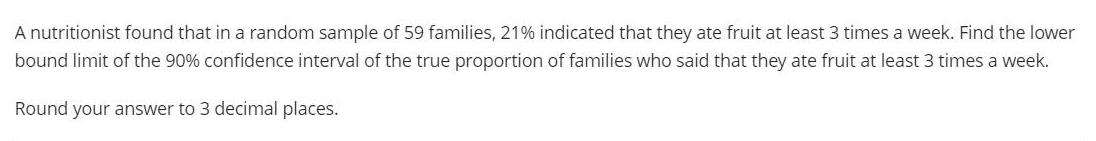
Math
StatisticsA nutritionist found that in a random sample of 59 families, 21% indicated that they ate fruit at least 3 times a week. Find the lower bound limit of the 90% confidence interval of the true proportion of families who said that they ate fruit at least 3 times a week.

Math
StatisticsIn a survey of 1000 U.S. adults, 700 think police officers should be
required to wear body cameras while on duty. Construct 99% confidence
interval for the population proportion.

Math
Basic MathThe Great Pyramid of Giza, located in Egypt, is one of the Seven Wonders of the Ancient World. The length of one side of its square base is 440 cubits (an ancient unit of length). The Pyramid was 280 cubits tall when it was
constructed over 4000 years ago. Which of the following values most closely represents the volume of the Great Pyramid when it was first built?
A. 16,800,000 cubic cubits
B. 18,100,000 cubic cubits
C. 34,500,000 cubic cubits
D. 54,200,000 cubic cubits
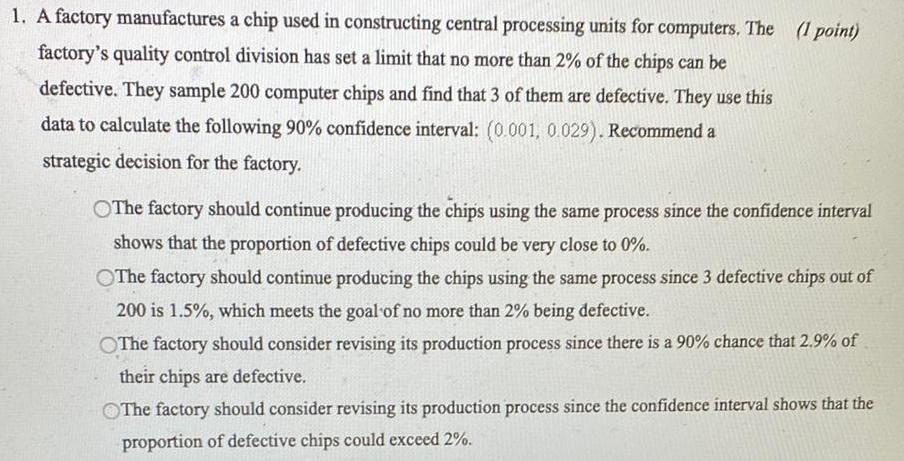
Math
StatisticsA factory manufactures a chip used in constructing central processing units for computers. The factory's quality control division has set a limit that no more than 2% of the chips can be defective. They sample 200 computer chips and find that 3 of them are defective. They use this data to calculate the following 90% confidence interval: (0.001, 0.029). Recommend a
strategic decision for the factory.
A. The factory should continue producing the chips using the same process since the confidence interval
shows that the proportion of defective chips could be very close to 0%.
B. The factory should continue producing the chips using the same process since 3 defective chips out of
200 is 1.5%, which meets the goal of no more than 2% being defective.
C. The factory should consider revising its production process since there is a 90% chance that 2.9% of their chips are defective.
D. The factory should consider revising its production process since the confidence interval shows that the proportion of defective chips could exceed 2%.

Math
Basic MathWhich of the following statements on the central limit theorem is true?
A) if the sample size increases then the sampling distribution much approach an exponential distribution
B. if the sample size increases sampling distribution must approach a normal distribution
C. if the sample size decreases then the sample distribution must approach a normal distribution
D if the sample size decreases then the sampling distribution much approach an exponential distribution
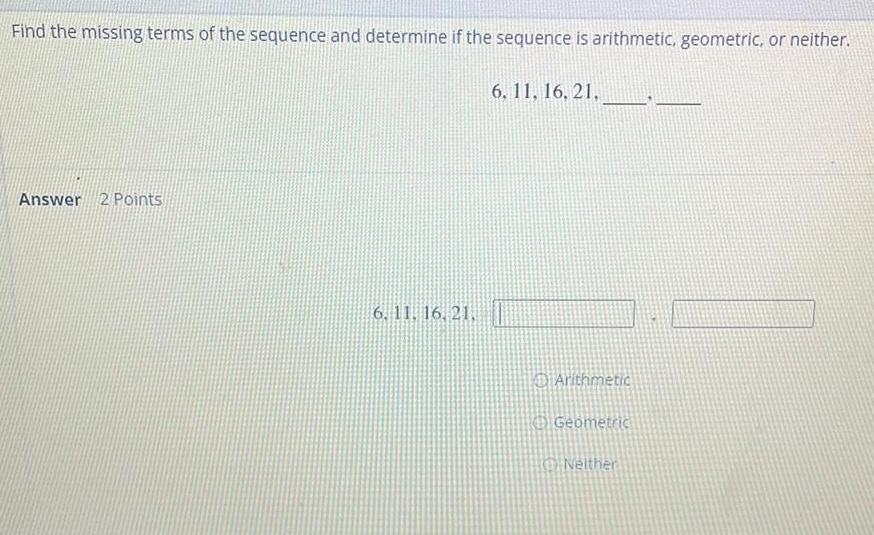
Math
Sequences & SeriesFind the missing terms of the sequence and determine if the sequence is arithmetic, geometric, or neither.
6, 11, 16, 21,_______,______ .
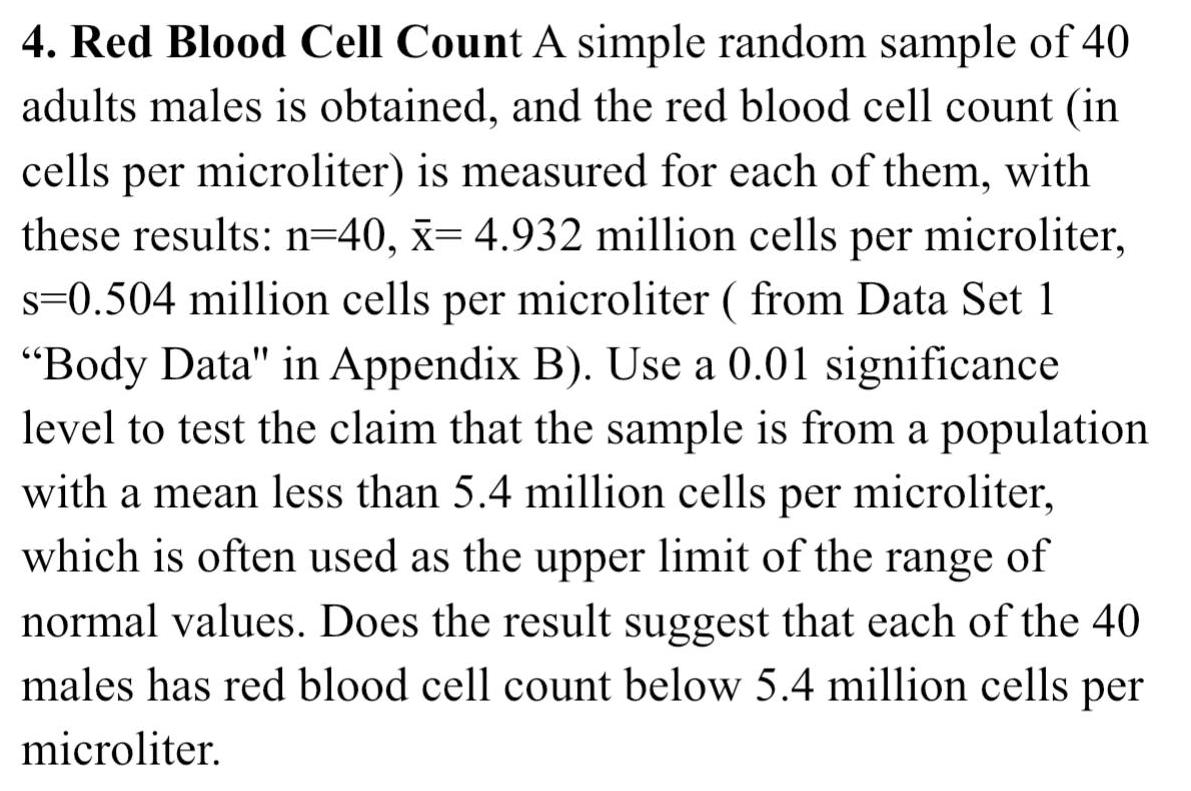
Math
StatisticsRed Blood Cell Count A simple random sample of 40 adults males is obtained, and the red blood cell count (in cells per microliter) is measured for each of them, with these results: n=40, x= 4.932 million cells per microliter, s=0.504 million cells per microliter ( from Data Set 1 "Body Data" in Appendix B). Use a 0.01 significance level to test the claim that the sample is from a population with a mean less than 5.4 million cells per microliter, which is often used as the upper limit of the range of normal values. Does the result suggest that each of the 40 males has red blood cell count below 5.4 million cells pern microliter.

Math
Basic MathFind the surface area of a square pyramid whose side length of the base is 9 cm and has a slant height of 6 cm.
A. 254 cm²
B. 142 cm²
C. 189 cm²

Math
StatisticsA recent study of 25 randomly selected employees of a company showed that the mean of the distance they traveled to work was 14.71 miles. The standard deviation of the sample mean was 2.62 miles. Find the upper bound 95% confidence interval of the true mean.
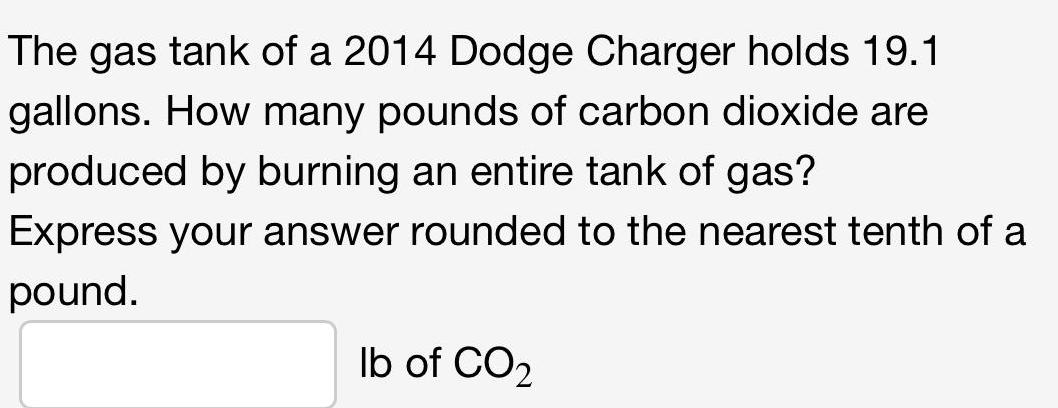
Math
Basic MathThe gas tank of a 2014 Dodge Charger holds 19.1 gallons. How many pounds of carbon dioxide are produced by burning an entire tank of gas?
Express your answer rounded to the nearest tenth of a pound.
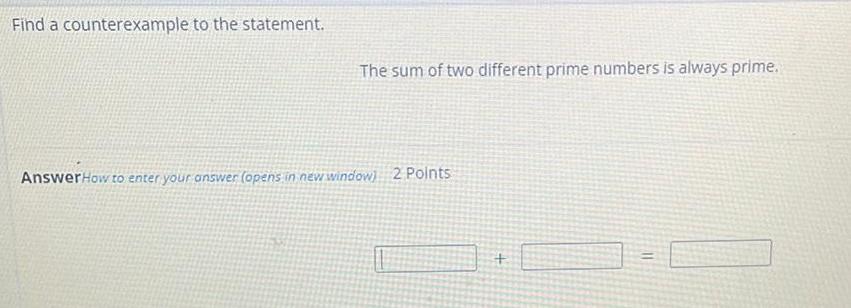
Math
Basic MathFind a counterexample to the statement.
The sum of two different prime numbers is always prime.

Math
TrigonometryThe following problem refers to triangle ABC, find all missing parts. Round degrees to 1 decimal places and sides to the nearest whole number.
A = 21.6°, B = 51.4°, a = 130 inches
A =
B
C=
a= ___________ inches
b= ____________Inches
C=______________ inches
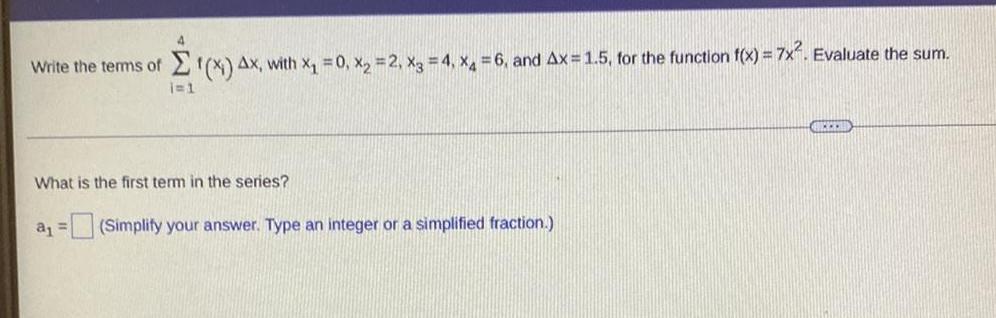
Math
Sequences & SeriesWrite the terms of 4Σi=1 f(x1) Δx with x₁ = 0, x₂ = 2, x3 = 4, x4= 6, and Δx=1.5, for the function f(x) = 7x². Evaluate the sum.
What is the first term in the series?
a₁ = (Simplify your answer. Type an integer or a simplified fraction.)
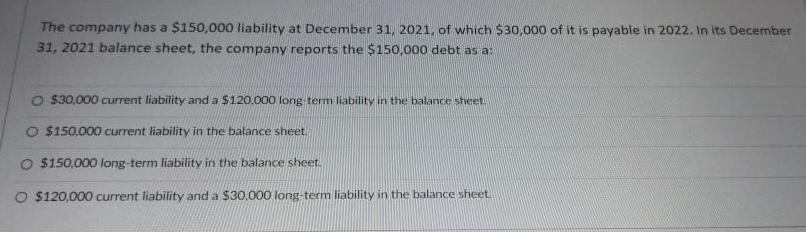
Math
Basic MathThe company has a $150,000 liability at December 31, 2021, of which $30,000 of it is payable in 2022. In its December 31, 2021 balance sheet, the company reports the $150,000 debt as a:
$30,000 current liability and a $120.000 long-term liability in the balance sheet.
$150,000 current liability in the balance sheet.
$150,000 long-term liability in the balance sheet.
$120,000 current liability and a $30,000 long-term liability in the balance sheet.
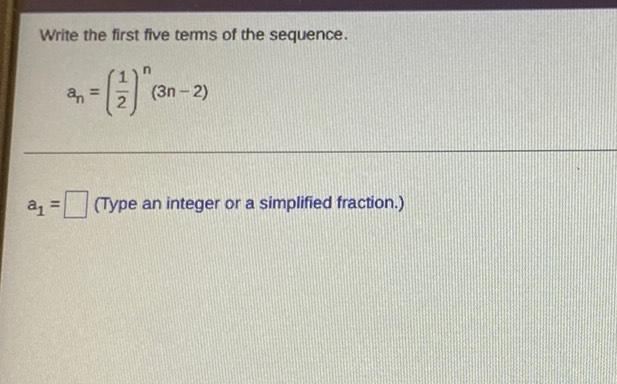
Math
Basic MathWrite the first five terms of the sequence.
an=(1/2)n(3n-2)
a₁ = (Type an integer or a simplified fraction.)

Math
AreaA pen stand made of wood is in the shape of a cubold with four conical depressions to hold pens. The dimensions of
the cubold are 15 cm by 10 cm by 3.5 cm. The radius of each of the depressions is 0.5 cm and the depth is 1.4 cm.
Find the volume of wood in the entire stand. Use it = 22/7.
529.68 cm³
520.48 cm³
523.53 cm³
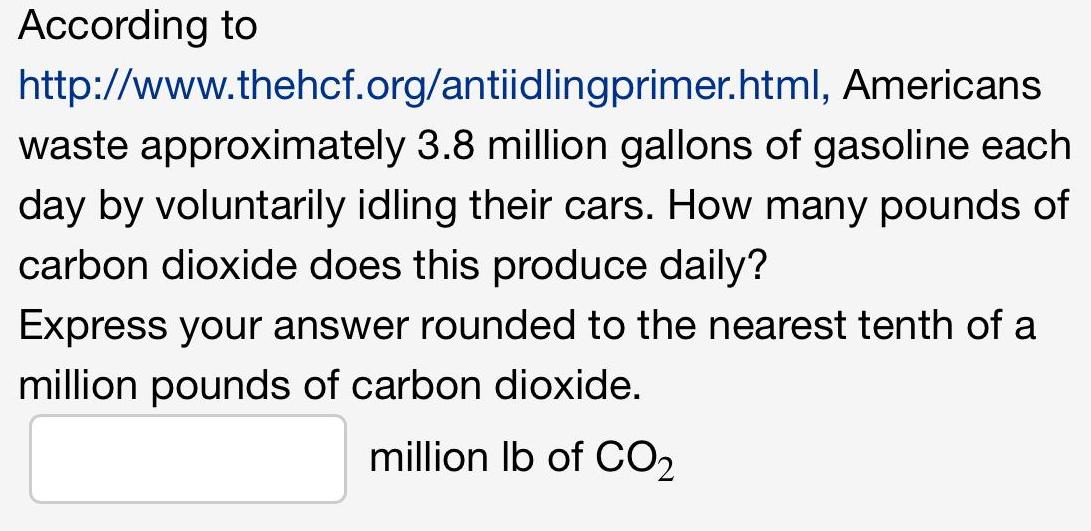
Math
Basic MathAccording to http://www.thehcf.org/antiidlingprimer.html, Americans waste approximately 3.8 million gallons of gasoline each day by voluntarily idling their cars. How many pounds of
carbon dioxide does this produce daily?
Express your answer rounded to the nearest tenth of a
million pounds of carbon dioxide.
million lb of CO₂

Math
StatisticsFrederick is creating a plan to conduct a randomized survey. He will be asking guests at a baseball stadium to rate their experience. At which location should he take the survey to ensure randomization?
the section of seats directly behind home plate
the hot dog stand
the stadiums front gate
the gift shop

Math
Definite IntegralsThe volume produced by revolving about the x-axis the region above the curve y= x³, below the
line y = 1 and between x = 0 and x = 1 is ...
A π/42
B π/7
C √2/2π
D 6π/7

Math
Basic MathA sample of 15 measurements of the breaking strengths of cotton threads gave a mean of 7.3 oz and a standard deviation of 2.82 oz. Find an upper bound 95% confidence limits for the actual mean breaking strength.

Math
Basic MathA 95% confidence interval estimate for the difference between two population means, 12000, is determined to be (62.75,
68.52.) If the confidence level is reduced to 90%, the confidence interval
(A) insufficient information
(B) becomes narrower
(C) becomes wider
(D) remains the same
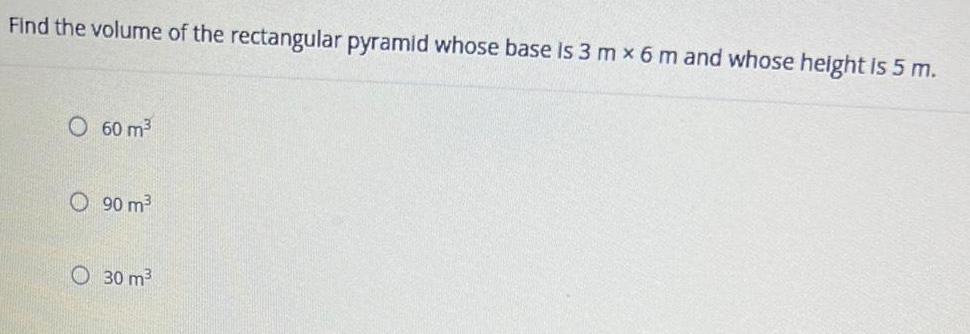
Math
AreaFind the volume of the rectangular pyramid whose base is 3 m x 6 m and whose height is 5 m.
60 m³
90 m³
30 m³
![Find the exact value of the expression.
tan cos[-1(√3/2)]
Select the correct choice and fill in any answer boxes in your choice below.
A.=tan cos[-1(√3/2)]=36.7
(Simplify your answer, including any radicals. Use integers or fractions for any numbers in the
B. There is no solution.](https://media.kunduz.com/media/sug-question/raw/77082248-1660207853.4426715.jpeg?w=256)
Math
Inverse Trigonometric functionsFind the exact value of the expression.
tan cos[-1(√3/2)]
Select the correct choice and fill in any answer boxes in your choice below.
A.=tan cos[-1(√3/2)]=36.7
(Simplify your answer, including any radicals. Use integers or fractions for any numbers in the
B. There is no solution.

Math
Statistics6. BMI for Miss America A claimed trend of thinner
Miss America winners has generated charges that the
contest encourages unhealthy diet habits among young
women. Listed below are body mass indexes (BMI) FOR
RECENT Miss America winners. Use a 0.01 significance
level to test the claim that recent winners are from a
population with a mean BMI less than 20.16, which was
the BMI for winners from the 1920's and 1930's. Given
that BMI is a measure of the relative amounts of body fat
and height, do recent winners appear to be significantly
smaller than those from the 1920's and 1930's.
19.5 20.3 19.6 20.2 17.8 17.9 18.8 17.6 16.8 19.1
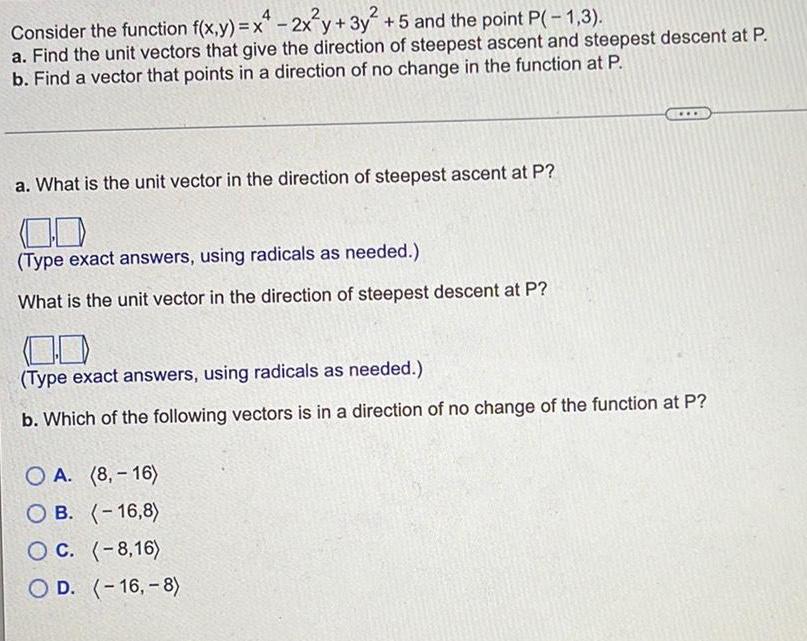
Math
VectorsConsider the function f(x,y) = x² - 2x²y+3y² +5 and the point P(-1,3).
a. Find the unit vectors that give the direction of steepest ascent and steepest descent at P.
b. Find a vector that points in a direction of no change in the function at P.
a. What is the unit vector in the direction of steepest ascent at P?
<_,_>
(Type exact answers, using radicals as needed.)
What is the unit vector in the direction of steepest descent at P?
<_,_>(Type exact answers, using radicals as needed.)
b. Which of the following vectors is in a direction of no change of the function at P?
A. (8,-16)
B. (-16,8)
C. (-8,16)
D. (-16,-8)
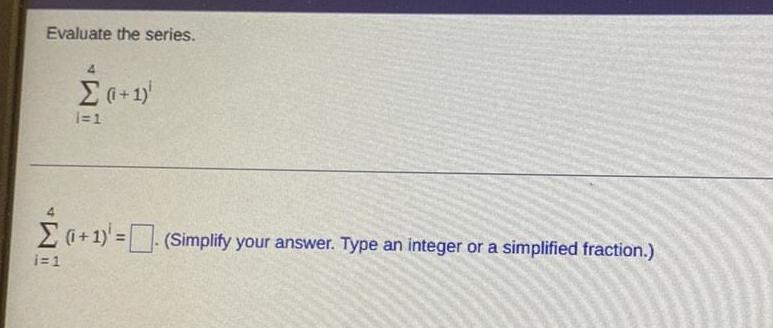
Math
Complex numbersEvaluate the series.
4Σ (i+1)
i=1
i=14Σ (i+1) = (Simplify your answer. Type an integer or a simplified fraction.)

Math
StatisticsA group of 625 students has a mean age of 15.8 years with a standard deviation of 0.6 years. The ages are normally distributed. What is the probability that a randomly selected student is older than 16.5 years old?

Math
StatisticsEach bag of different colored jelly beans is supposed to have 30% blue jelly beans. Ramon believes there are actually a greater proportion of blue jelly beans. He randomly selects 25 bags of jelly beans and finds the proportion of blue jelly beans to be 36%. He uses a significance level of a = 0.1 and calculates a p-value of 0.256. What null and alternative hypothesis did Ramon use for the test, and what conclusion can he make?
(1 point)
H0: p=0.36
He p > 0.36
Ramon fails to reject the null hypothesis because the p-value is greater than the significance level.
H0: p=0.3
He p > 0.3
Ramon fails to reject the null hypothesis because the p-value is greater than the significance level.
H0: p=0.3
He P=0.3
Ramon rejects the null hypothesis because the p-value is greater than the significance level.
H0 p = 0.3
He: p=0.3
Ramon rejects the null hypothesis because the p-value is greater than the significance level.

Math
Basic MathWhich is an example of an experiment?
A company asks its website users to rate its new features to navigate the site.
Researchers working for a company test their new sneaker design to see if it helps athletes run faster.
A scientist watches a group of local birds to see what type of tree they prefer to make their nests in.
An ice cream company asks people to identify their top three ice cream flavors.

Math
Coordinate systemThere are three types of solutions to any system of two equations. What are the types? What do those solutions look like graphically?

Math
StatisticsSylvia makes pizzas at an Italian restaurant. A large pizza is listed on the menu as being 16 inches. Sylvia wants to know how accurate she is at throwing dough to that size. A coworker measures all the pizzas Sylvia throws, and they randomly select 35 data points. They use this data to calculate the following 95% confidence interval: (15.85, 16.10). Use this to recommend a strategic decision.
Sylvia should use more dough since the least value of the confidence interval is less than 16 inches.
Sylvia should use less dough since the greatest value of the confidence interval is greater than 16 inches.
Sylvia should continue using the same amount of dough since 16 inches is contained within the
confidence interval.
Sylvia should use more dough so that the entire confidence interval is greater than 16 inches.

Math
Basic MathFor the rotation 1409°, find the coterminal angle from 0° 0 < 360°, the quadrant and the reference angle.
The coterminal angle is __° which lies in Quadrant __
with a reference angle of __ °
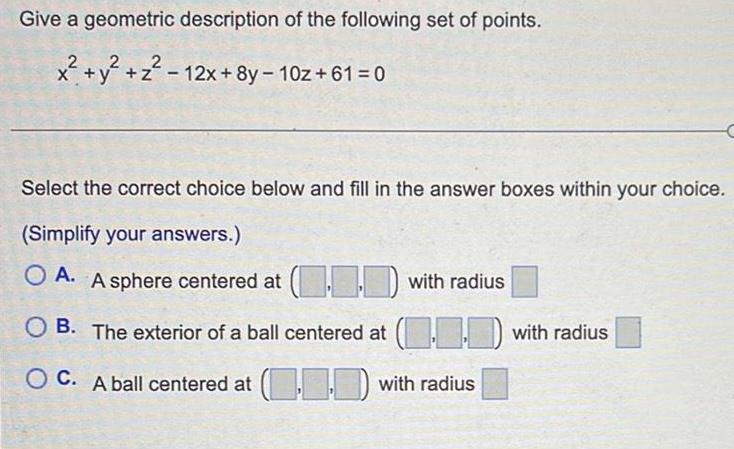
Math
Basic MathGive a geometric description of the following set of points.
x² + y² +2²-12x+8y-10z+61 = 0
Select the correct choice below and fill in the answer boxes within your choice.
(Simplify your answers.)
A. A sphere centered at (__,__,__) with radius __
B. The exterior of a ball centered at (__,__,__) with radius __
C. A ball centered at ;(__,__,__) with radius __