Math Questions
The best high school and college tutors are just a click away, 24×7! Pick a subject, ask a question, and get a detailed, handwritten solution personalized for you in minutes. We cover Math, Physics, Chemistry & Biology.

Math
Trigonometry5/6. From the outside the weightroom, Drake sees Cade walking at the track. If the height of the track stairs is 30 feet from the bottom to the top, and Drake is 35 feet from the bottom of the stairs, what is the angle of elevation fom Drake to the track? Round to the nearest 10th.
Picture
Equation:
Answer:

Math
Basic MathSuppose Solid A and Solid B are similar. Solid A has an edge length of 18 cm, and the
corresponding edge in Solid B is 12 cm. If the volume of Solid A is 229.5 cm³, what is
the volume of Solid B in cubic centimeters?

Math
StatisticsWhat is the probability of randomly selecting 1 woman with a height less than 65.3 inches?
(Round to four decimal places as needed.)
What is the probability of selecting a sample of 18 women with a mean height less than 65.3 inches?
(Round to four decimal places as needed.)
Are you more likely to randomly select 1 woman with a height less than 65.3 inches or are you more likely to select a sample of 18 women with a mean height less than 65.3 inches? Choose the correct answer below.
A. It is more likely to select 1 woman with a height less than 65.3 inches because the probability is lower.
B. It is more likely to select a sample of 18 women with a mean height less than 65.3 inches because the sample of 18 has a lower probability.
C. It is more likely to select 1 woman with a height less than 65.3 inches because the probability is higher.
D. It is more likely to select a sample of 18 women with a mean height less than 65.3 inches because the sample of 18 has a higher probability.

Math
Coordinate systemIf you apply the transformations below to the quadratic parent function, F(x) = x², what is the equation of the new function?
Shift six units right.
Shift four units down.
A. G(x) = (x-6)²-4
B. G(x) = (x-4)² +6
C. G(x) = (x-4)²-6
D. G(x) = (x+6)² - 4
Math
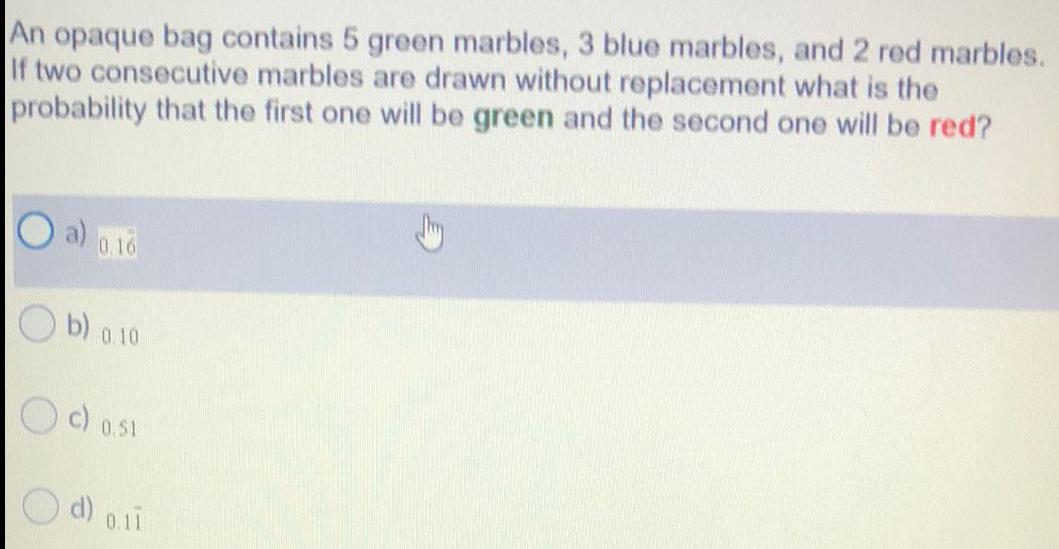
ProbabilityAn opaque bag contains 5 green marbles, 3 blue marbles, and 2 red marbles.
If two consecutive marbles are drawn without replacement what is the
probability that the first one will be green and the second one will be red?
a)0.16
b) 0.10
c) 0.51
d) 0.11
Math
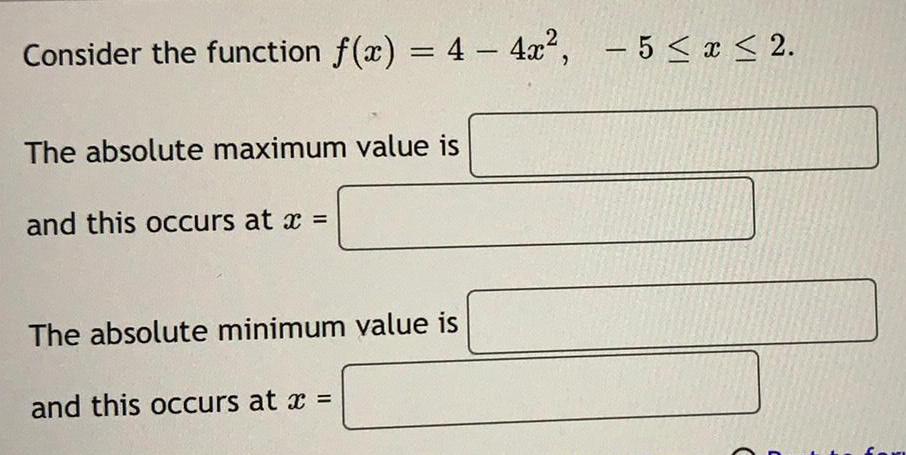
Basic MathConsider the function f(x) = 4 - 4x², -5 ≤ x ≤ 2.
The absolute maximum value is
and this occurs at x =
The absolute minimum value is
and this occurs at x =
Math
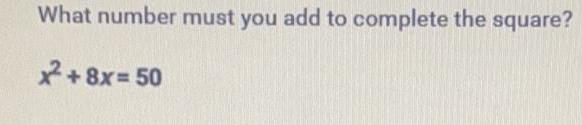
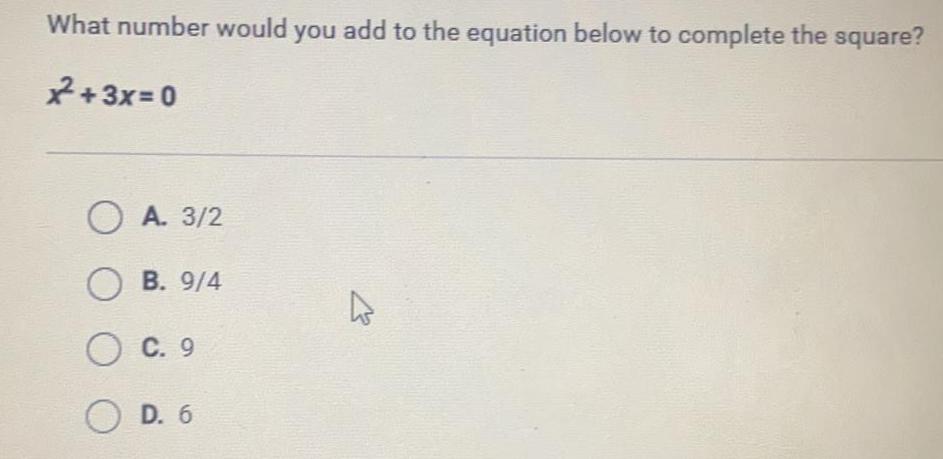
Quadratic equationsWhat number would you add to the equation below to complete the square?
x^2+3x=0
A. 3/2
B. 9/4
C. 9
D. 6

Math
Basic MathQuestion Help ▼
In a survey of 1000 drivers from Region A, 864 wear a seat belt. In a survey of 1000 drivers from Region B, 915 wear a seat belt. At a=0.05, is there evidence to support the claim
that the proportion of drivers who wear seat belts in Region A is less than the proportion of drivers who wear seat belts in Region B? Assume that the samples are random and
independent. Complete parts (a) through (e) below.
(a) Identify the claim and state Ho and Ha-
Identify the claim. Let population 1 be drivers from Region A and let population 2 be drivers from Region B. Choose the correct answer below.
A. The claim is that the proportions of drivers who wear seat belts in both regions add up to 1.
B. The claim is that the proportions of drivers who wear seat belts in both regions are equal.
C. The claim is that the proportion of drivers who wear seat belts in Region A is less than the proportion of drivers who wear seat belts in Region B.
D. The claim is that the proportion of drivers who wear seat belts in Region A is greater than the proportion of drivers who wear seat belts in Region B.
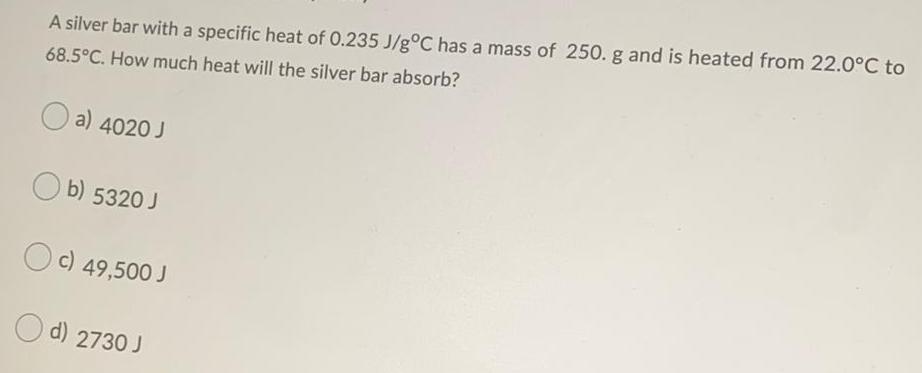
Math
Application of derivativesA silver bar with a specific heat of 0.235 J/g°C has a mass of 250. g and is heated from 22.0°C to 68.5°C. How much heat will the silver bar absorb?
a) 4020 J
b) 5320 J
c) 49,500 J
d) 2730 J

Math
Basic MathThe volume of a cone is 800 cubic feet. If the radius of the base is doubled and the height remains the same, what will be the volume of the new cone? .
6,400 ft³
1,200 ft
1,600 ft³
3,200 ft³
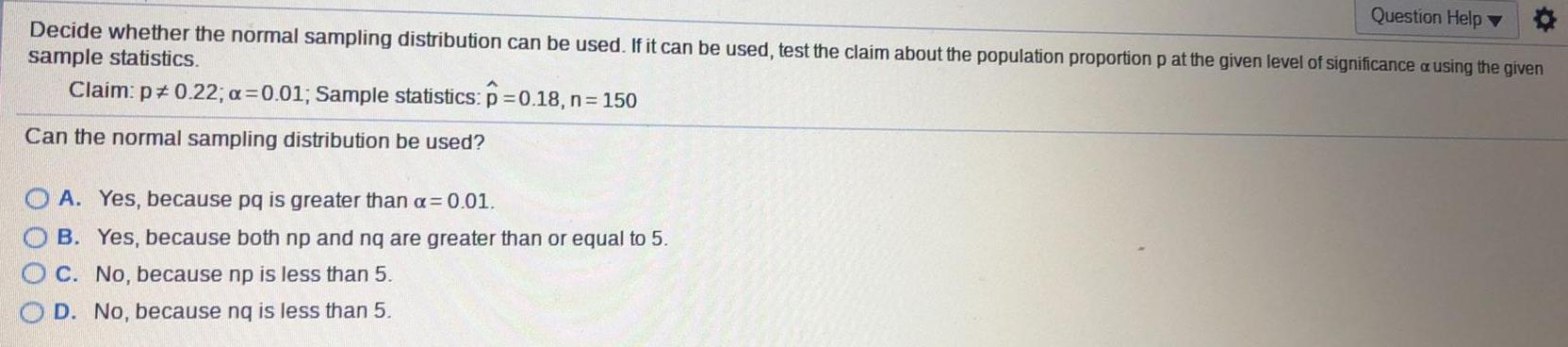
Math
StatisticsQuestion Help Decide whether the normal sampling distribution can be used. If it can be used, test the claim about the population proportion p at the given level of significance a using the given sample statistics.
Claim: p=0.22; x = 0.01; Sample statistics: p=0.18, n = 150
Can the normal sampling distribution be used?
A. Yes, because pq is greater than α=0.01.
B. Yes, because both np and nq are greater than or equal to 5.
C. No, because np is less than 5.
D. No, because ng is less than 5.

Math
Coordinate systemChoose the best definition of SAS Similarity.
When two triangles have corresponding angles that are congruent and the included corresponding sides with identical ratios.
None of the other answers are correct
Sides that are in the same position in different plane figures.
When two triangles have corresponding angles that are congruent, the triangles are similar.
the result of a transformation.


Math
Coordinate systemA directed line segment begins at F(-10, -4), ends at H(8, 4), and is divided in the ratio 6 to 2 by G.
What are the coordinates of G?
(3.3, 5)
None of the other answers are correct
(-1.6, 5)
(3.5, 2)
(2.5, 2)

Math
Basic MathFind the difference and enter it in the box below. Enter exponents using the caret (^). For example, write 4x² as 4x^2. (2x + 4x4-2x+3) - (4x4-2x³+3)
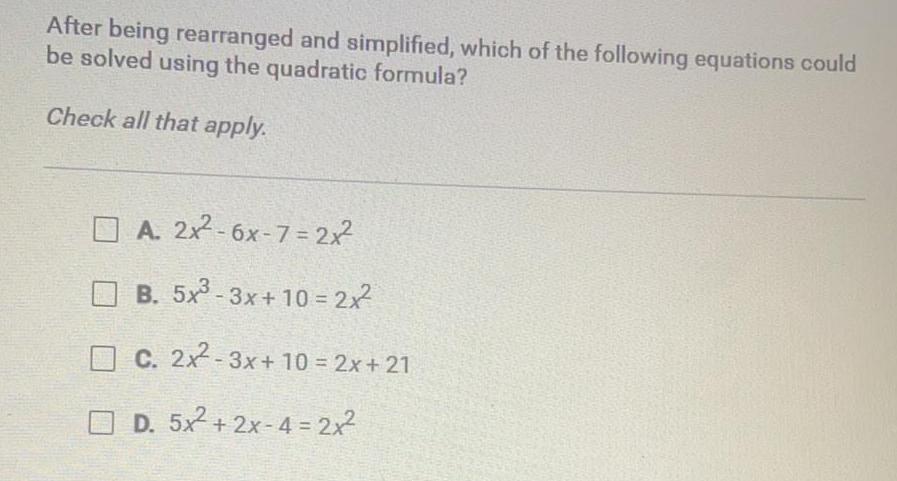
Math
Basic MathAfter being rearranged and simplified, which of the following equations could be solved using the quadratic formula?
Check all that apply.
A. 2x²-6x-7 = 2x²
B. 5x³-3x+10= 2x²
C. 2x²-3x+ 10 = 2x+ 21
D. 5x²+2x-4 = 2x²

Math
AreaA grocery store wants to paint 6 concrete spheres that are in their parking lot in front of the store. If the diameter of each sphere is 3.5 feet, which value is closest to the total surface area that will be painted in square inches? Show your work.
A. 22,170 in.²
B. 5,540 in.2
C. 33,250 in.2
D.17,600 in.2
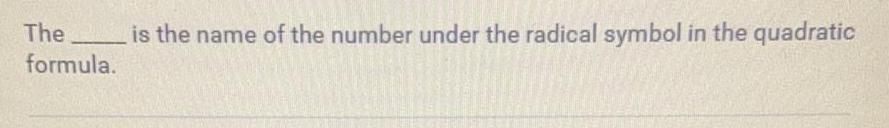
Math
Quadratic equationsThe ________ is the name of the number under the radical symbol in the quadratic formula.

Math
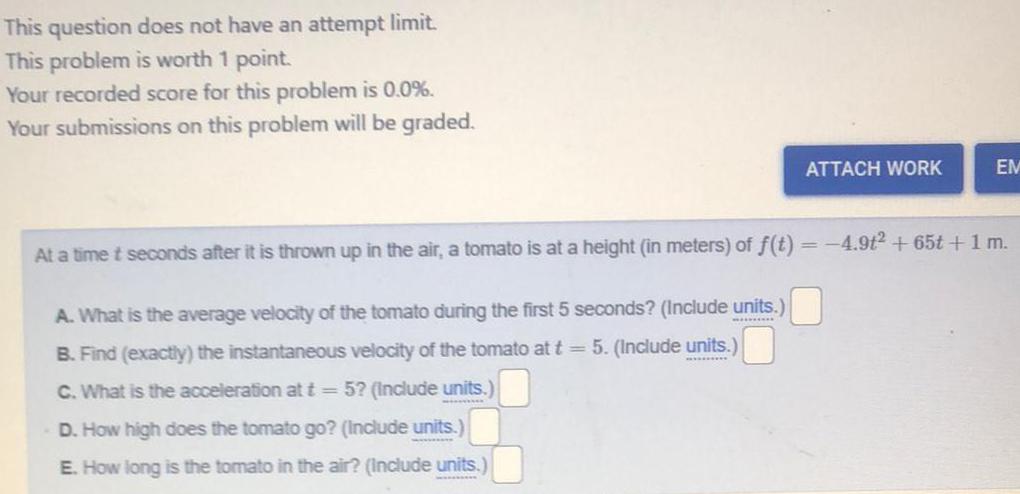
Basic MathAt a time t seconds after it is thrown up in the air, a tomato is at a height (in meters) of f(t) = -4.9t² + 65t +1 m.
A. What is the average velocity of the tomato during the first 5 seconds? (Include units.)
B. Find (exactly) the instantaneous velocity of the tomato at t = 5. (Include units.)
C. What is the acceleration at t = 5? (Include units.)
D. How high does the tomato go? (Include units.)
E. How long is the tomato in the air? (Include units.)

Math
ProbabilityDetermine if the scenario Involves mutually exclusive events. Then find the probability. A box of chocolates contains three milk chocolates, four dark chocolates, and three white chocolates. You randomly select a chocolate. What is the probabil Is a milk chocolate or a dark chocolate?
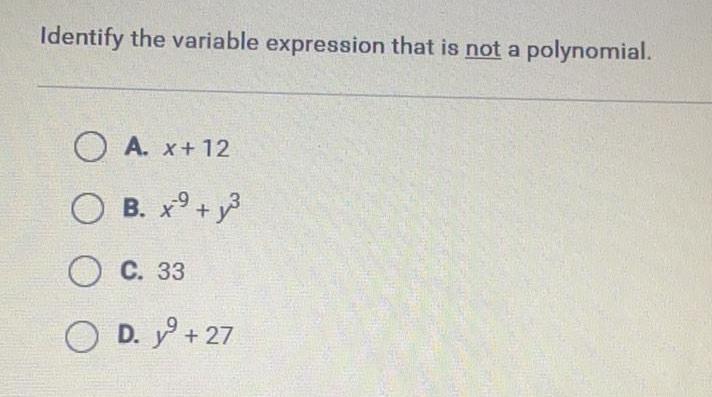
Math
Basic MathIdentify the variable expression that is not a polynomial.
A. x+12
B. x^-9 + y^3
C. 33
D. y^9+ 27

Math
Basic MathA directed line segment begins at F(-10, -2), ends at H(4, 4), and is divided in the ratio 4 to 2 by G. What are the coordinates of G?
(-0.87, 2)
(-1.6, 2)
(3.3, 5)
None of the other answers are correct
(-0.67, 2)
Math
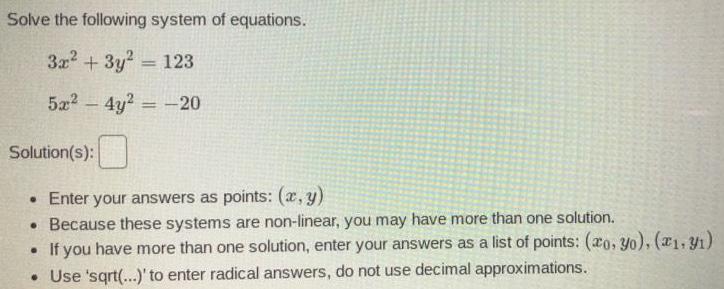
Basic MathSolve the following system of equations.
3x² + 3y² = 123
5x² - 4y² = -20
Solution(s):
• Enter your answers as points: (x, y)
• Because these systems are non-linear, you may have more than one solution.
• If you have more than one solution, enter your answers as a list of points: (co, y0), (1.1)
• Use 'sqrt(...)' to enter radical answers, do not use decimal approximations.
Math
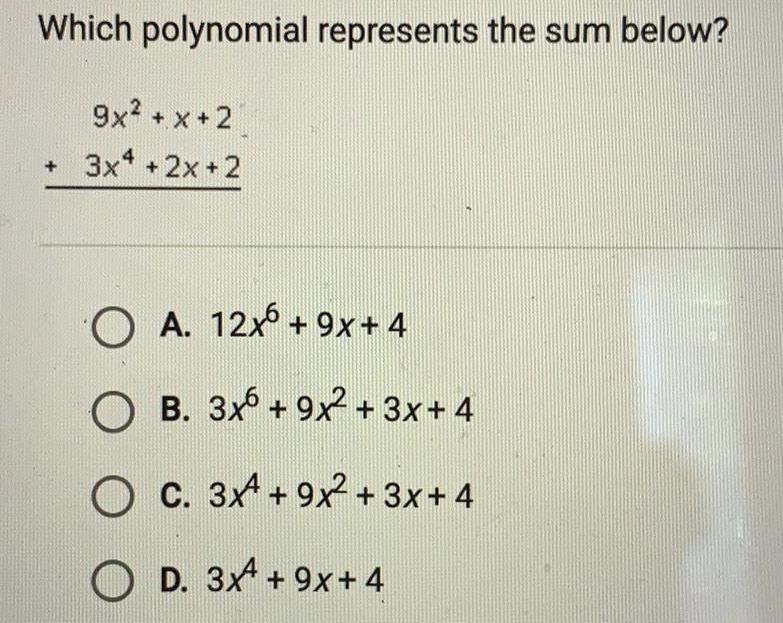
Basic MathWhich polynomial represents the sum below?
9x² + x + 2
+ 3x^4+2x+2
A. 12x^6 + 9x+4
B. 3x^6 + 9x² + 3x+4
C. 3x^4 + 9x² + 3x+4
D. 3x^4 + 9x+4

Math
Basic MathEach letter of the alphabet is written on separate cards in red ink. The cards are placed in a container. Each letter of the alphabet is also written on separate cards in black ink. The cards are placed in the same container. What is the probability that a card randomly selected from the container has a letter written in black ink or the letter A or Z?
8/13
15/26
1/2
7/13

Math
StatisticsAn education researcher claims that at most 4% of working college students are employed as teachers or teaching assistants. In a random sample of 500 working college students, 6% are employed as teachers or teaching assistants. At a=0.10, is there enough evidence to reject the researcher's claim? Complete parts (a) through (e) below. (a) Identify the claim and state Ho and Ha
Identify the claim in this scenario. Select the correct choice below and fill in the answer box to complete your choice. (Type an integer or a decimal. Do not round.)
A. % of working college students are employed as teachers or teaching assistants.
B. More than % of working college students are employed as teachers or teaching assistants.
C. At most % of working college students are employed as teachers or teaching assistants.
D. The percentage of working college students who are employed as teachers or teaching assistants is not %.

Math
Basic MathIf a plane intersects a right cylinder, the cross-section is a circle.
Which of the following describes a counterexample to this statement?
A. If the plane is parallel to the bases, the cross-section is a circle.
B. If the plane is perpendicular to the bases, the cross-section is a rectangle.
C. If the plane is tangent to the bases, the cross-section is a line.
D. None of these, the original statement is always true.
(4 pts.)

Math
Coordinate systemA scientist concerned about dehydration of soccer players on hot days measured the fluld consumption of twenty-six
soccer players. The mean fluid consumption per day was 2,780 milliliters with a standard deviation of 567 milliters.
What is the probability that a player consumed between 1,646 and 3,347 milliliters? Use the 68-95-99.7 normal
distribution curve.
97.35%
81.5%
83.85%
81.3%
Math
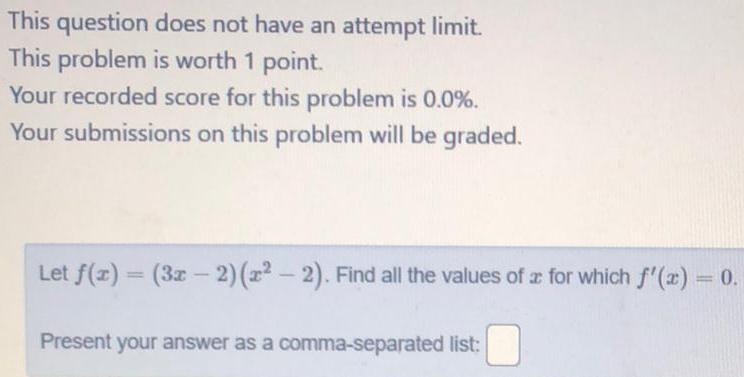
Basic MathThis question does not have an attempt limit.
This problem is worth 1 point.
Your recorded score for this problem is 0.0%.
Your submissions on this problem will be graded.
Let f(x) = (3x - 2) (x²-2). Find all the values of x for which f'(x) = 0.
Present your answer as a comma-separated list:

Math
Application of derivativesA cylinder shaped can needs to be constructed to hold 300 cubic centimeters of soup. The material for the sides of the can costs 0.03 cents per square centimeter. The material for the top and bottom of the can need to be thicker, and costs 0.05 cents per square centimeter. Find the dimensions for the can that will minimize production cost.
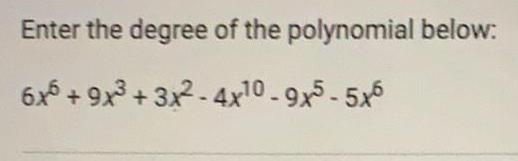


Math
Basic MathWhich of the following is a polynomial?
A. (x6-2)/(x4 + 3)
B. + x4 + 16
C. xy + 2
D. x²-1

Math
Application of derivatives(A) Find the average rate of change of the area of a circle with respect to its radius r as r changes from
(i) 5 to 6 →→ Average rate of change =
(ii) 5 to 5.4 → Average rate of change =
(iii) 5 to 5.1 → Average rate of change =
(B) Find the instantaneous rate of change when r = 5.
Instantaneous rate of change =

Math
StatisticsIn the past two years, both product A and product B have had similar sales and the production Investment is also the same for both of them. This year, the manufacturer feels that Product A may have more sales as compared to Product B . Determine the null and alternate hypotheses, as well as what each potential error represents in terms of reality and researcher conclusion. Which potential error do you think is more severe?
A Type I error has more severe effects in this scenario.
A Type II error has more severe effects in this scenario.
A Type I error and a Type Il error have equally severe consequences in this scenario.
It is impossible to tell which error type has more severe consequences in this scenario.

Math
ParabolaIf you horizontally compress the quadratic parent function, F(x)=x2, by 7 units, what is the equation of the new function?
A. G(x) = (x+7)²
B. G(x) = 7x²
C. G(x) = (7x)²
D. G(x)=x²-7

Math
Basic MathSodium chlorate crystals are easy to grow in the shape of a cube by allowing a solution of water and sodium chlorate to evaporate slowly. If V is the volume of such a cube with side length z, calculate dV/dz when x = 5 mm. dV/dz =

Math
Basic MathThe mean age of the people in room 5 is 29 years. The mean age of the people in room 6 is 35 years. If there are 27 people in room 5 and 22 people in room 6, then what is the mean age of all the people in room 5 and room 6 put together? (nearest tenth).
30.7
31.7
29.6
32

Math
Heights and DistancesA plane is flying at an elevation of 24000 feet. It is within sight of the airport and the pilot finds that the angle of depression to the airport is 14°.
Find the diagonal distance between the plane and the base of the airport. Round your answer to the nearest foot. .
Note that for this problem, we are using degrees to measure angles. Make sure your calculator is set to degrees instead of radians.

Math
Basic MathIn 2000, the population of St. Louis was 346,904, and it decreased to 319,257 in 2010. If this population decrease were modeled by an exponential decay function, what value would represent the y-intercept? Explain your reasoning.
Math
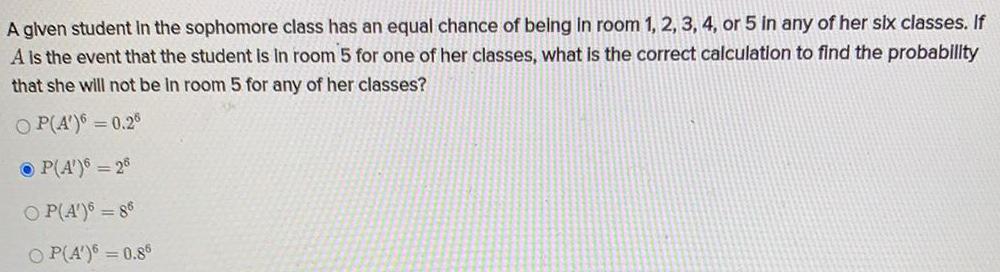
ProbabilityA given student in the sophomore class has an equal chance of being in room 1, 2, 3, 4, or 5 in any of her six classes. If A is the event that the student is in room 5 for one of her classes, what is the correct calculation to find the probability that she will not be in room 5 for any of her classes?
P(A) = 0.28
P(A) = 28
P(A) = 88
P(A')6 = 0.86
![A box with a square base and open top must have a volume of 48668 cm³. We wish to find the dimensions of the box that minimize the amount of material used.
First, find a formula for the surface area of the box in terms of only a, the length of one side of the square base.
[Hint: use the volume formula to express the height of the box in terms of x.]
Simplify your formula as much as possible.
A(x) =
Next, find the derivative, A'(x).
A'(x) =
Now, calculate when the derivative equals zero, that is, when A'(x) = 0. [Hint: multiply both sides by x².]
A'(x) = 0 when x =
We next have to make sure that this value of a gives a minimum value for the surface area. Let's use the second derivative test. Find A"(x).
A"(x)=
Evaluate A"(x) at the x-value you gave above.
NOTE: Since your last answer is positive, this means that the graph of A(x) is concave up around that value, so the zero of A'(x) must indicate a local minimum for A(x). (Your boss is happy now.)](https://media.kunduz.com/media/sug-question/raw/47858147-1658333755.3716078.jpeg?w=256)
Math
Application of derivativesA box with a square base and open top must have a volume of 48668 cm³. We wish to find the dimensions of the box that minimize the amount of material used.
First, find a formula for the surface area of the box in terms of only a, the length of one side of the square base.
[Hint: use the volume formula to express the height of the box in terms of x.]
Simplify your formula as much as possible.
A(x) =
Next, find the derivative, A'(x).
A'(x) =
Now, calculate when the derivative equals zero, that is, when A'(x) = 0. [Hint: multiply both sides by x².]
A'(x) = 0 when x =
We next have to make sure that this value of a gives a minimum value for the surface area. Let's use the second derivative test. Find A"(x).
A"(x)=
Evaluate A"(x) at the x-value you gave above.
NOTE: Since your last answer is positive, this means that the graph of A(x) is concave up around that value, so the zero of A'(x) must indicate a local minimum for A(x). (Your boss is happy now.)
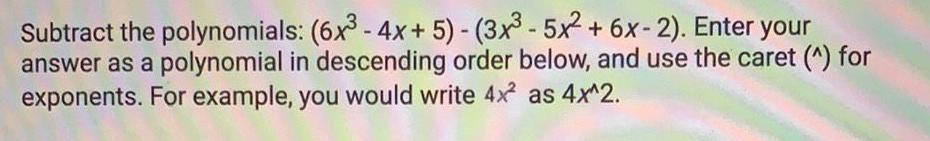
Math
Basic MathSubtract the polynomials: (6x³ - 4x + 5)-(3x3-5x² + 6x-2). Enter your
answer as a polynomial in descending order below, and use the caret (^) for exponents. For example, you would write 4x2 as 4x^2.

Math
TrigonometryThe angle of elevation to the top of Wayne Tower in Gotham City is found to be 11° from the ground at a distance of 5,280 feet from the
base of the building. Using this information, find the height of Wayne Tower.
The height of Wayne Tower is
feet. Round your answer to the nearest tenth of a foot.
. Note that for this problem, we are using degrees to measure angles. Make sure your calculator is set to degrees instead of radians.
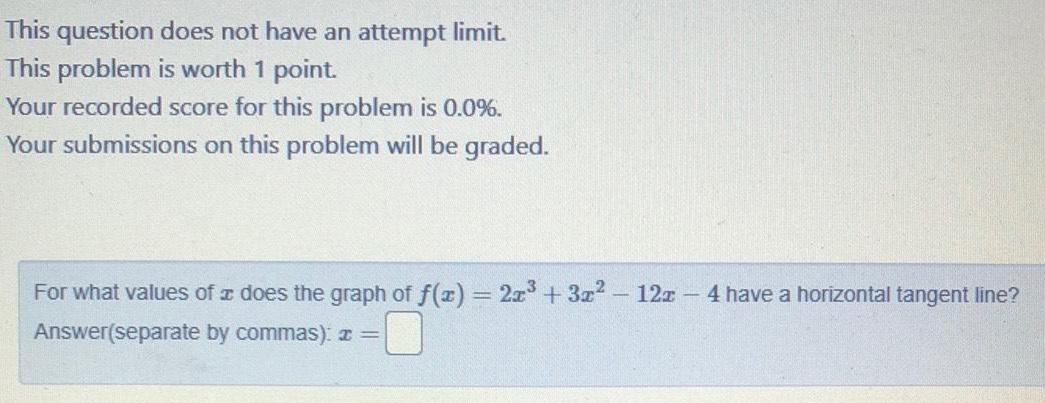
Math
Application of derivativesFor what values of a does the graph of f(x) = 2x³ + 3x² - 12z - 4 have a horizontal tangent line?
Answer(separate by commas): x =

Math
Basic MathSelect all of the transformations that always maintain a shape's congruency? *
A reflection
A dilation
A reflection then a dilation
A rotation then a translation
A translation then a reflection

Math
Permutations and CombinationsA jar has two red, three blue, one white, and three yellow jelly beans. How many ways are there to line up the jelly
beans?
5,040
20,160
60,480
362,880

Math
LogarithmsThe population of a certain city was 3,674 in 1995. It is expected to decrease by about 0.31% per year. Write an exponential decay function, and use it to approximate the population in 2020. The exponential decay function where f(x) is the population of the city x years after 1995 is f(x) =