Math Questions
The best high school and college tutors are just a click away, 24×7! Pick a subject, ask a question, and get a detailed, handwritten solution personalized for you in minutes. We cover Math, Physics, Chemistry & Biology.

Math
Basic MathPizarro's Pizzeria charges $12.50 for a large pizza. Each additional topping is $1.65.
Function: P(x) =
Slope/Meaning in context: m =
y-intercept/Meaning in Context: b =
What will the charge be for a pizza with 5 toppings?
If Pedro paid $24.05 for his pizza, how many toppings did he get?

Math
Basic MathThe count in a bacteria culture was 700 after 20 minutes and 1600 after 30 minutes. Assuming the count grows exponentially,
What was the initial size of the culture?
Find the doubling period.
Find the population after 65 minutes.
When will the population reach 10000. K

Math
Basic Math4) Write a system of equations to describe the situation below, solve using substitution, and
fill in the blanks,
A yoga studio offers memberships that cost $30 per month for unlimited classes. The
studio also accepts walk-ins, charging $10 per class. If someone attends enough classes in a month, the two options cost the same total amount. What is that total amount? How many
classes is that?
Each option costs $___if a person takes__classes,

Math
Basic MathTo find the distance from the house at A to the house at B, a surveyor measures the angle ACB, which is found to be 70°, and then walks off the distance to each house, 90 feet and 100 feet, respectively. How far apart are the houses?

Math
Basic MathOne of two complementary angles Is 8 degrees less than the other.
Which of the following systems of equations represents the word problem?
x+y=90 and 90 - x = 8
x+y=90 and y = 8 x
x+y=90 and x - y = 8

Math
Basic MathGiven f(x)= 16/ x+6 and g(x)=3√x, find the following expressions.
(a) (fog)(4) (b) (gof)(2) (c) (fof)(1) (d) (gog)(0)
(a) (fog)(4) =

Math
Quadratic equationsLet f(x) = 9x^4 - 6x³ +23x + 25. Use Descartes' Rule of Signs to answer the following questions.
(a) List the possible number of positive real zeros (counting multiplicities) of f(x):
(b) List the possible number of negative real zeros (counting multiplicities) of f(x):
(c) May anything definitive be said about the exact number of positive real zeros?
Select an answer
(d) May anything definitive be said about the exact number of negative real zeros?
Select an answer
There are no negative real zeros.
There are exactly two real negative zeros.
There is exactly one negative real zero.
No conclusion may be made about the exact number of negative real zeros.

Math
FunctionsCaptain Ahab needs $6000.00 worth of harpoons. He started with $1500.00 and has been saving $350 a week to buy the harpoons. Write a function that illustrates this situation.
Function: h(x)=
Slope/Meaning in context: m =
y-intercep/Meaning in context: b =
How much will Captain Ahab have saved after seven weeks?
How many weeks will it take the captain to buy the harpoons he needs? Write an equation to model this situation and solve.

Math
Basic Math25. What is the molarity of sodium chloride in solution that is 13.0% by mass sodium chloride and that has a density of 1.10
g/mL?
A) 143
B) 2.45
C) 2.56
D) 2.23
E) 1.43 x 10-2
B) 2.45
C) 2.56
E) 1.43 x 10-2
A) 143
D) 2.23

Math
Straight linesThe senior classes at High School A and High School B planned separate trips to the indoor climbing gym. The senior class at High School A rented and filled 12 vans and 9 buses with 435 students. High School B rented and filled 5 vans and 3 buses with 155 students. Every van had the same number of students in it as did the buses. How many students can a van carry? How many students can a bus carry?
![Consider the function f(x) = -1/2 x² + 3x + 9.
What is the overestimate of the area under the curve of f using rectangular approximations on the interval [-2, 3] with five equal subintervals?](https://media.kunduz.com/media/sug-question/raw/45615053-1658262023.156044.jpeg?w=256)
Math
AreaConsider the function f(x) = -1/2 x² + 3x + 9.
What is the overestimate of the area under the curve of f using rectangular approximations on the interval [-2, 3] with five equal subintervals?

Math
Quadratic equationsy=x² + 4x-5
Before we calculate important features of this quadratic function, identify a, b, &c. Remember, the standard form quadratic functions: y = ax^2 + bx + c.
a = |
b=
C=

Math
Basic MathLinus sells widgets at his store. Today he has sold 21 widgets to three customers. What is the cost per widget?
$2.00
$1.50
$3.00
$1.00

Math
Basic MathThe explicit formula for a certain geometric sequence is f(n) =525 (20)^n-1. What is the exponential function for the sequence? Write your answer in the form shown.
f(n)= a/r (r) ^n

Math
Sequences & SeriesSelect the correct answer.
Bacteria begins to grow on the water's surface in a non-operational swimming pool on September 20. The bacteria grows and covers the water's surface in such a way that the area covered with bacteria doubles every day. If It continues to grow in this way, the water's surface will be entirely covered with bacteria on September 28.
When will a quarter of the water's surface be covered?
A The water's surface will be covered a quarter of the way on September 24.
B. The water's surface will be covered a quarter of the way on September 27.
C. The water's surface will be covered a quarter the way on September 26.
D. The water's surface will be covered a quarter of the way on September 25.

Math
Sequences & SeriesMia baked a very large apple pie that she cuts into slices to share with her friends. The
smallest slice is cut first. The volume of each successive slice of pie forms a geometric
sequence.
The second smallest slice has a volume of 30cm³. The fifth smallest slice has a volume of
240cm³.
Find the common ratio of the sequence.
Find the volume of the smallest slice of pie.
The apple pie has a volume of 61425cm³.
Find the total number of slices Mia can cut from this pie.

Math
Complex numbersFind zw and z/w. Write each answer in polar form and in exponential form.
z=4( cos 2π/9 + i sin 2π/9)
w= 16 (cos π/9 + i sin π/9)

Math
Basic MathFind the x-intercepts of the polynomial function. State whether the graph crosses the x-axis, or touches the x-axis and turns around, at each intercept.
f(x) = 3x² - x³
A. 0, touches the x-axis and turns around;
3, crosses the x-axis
B. 0, touches the x-axis and turns around;
3, touches the x-axis and turns around
C. 0, touches the x-axis and turns around;
3, crosses the x-axis;
- 3, crosses the x-axis
D. 0, crosses the x-axis;
3, crosses the x-axis;
- 3, crosses the x-axis

Math
Basic MathA) The number of students using a certain university's online programs has been
increasingly exponentially each year since 2010. The number of students n who use
the university's online programs t years after 2010 can be modeled using the function
n (t) = 425(1.14)^t . Interpret the meaning of 1.14 in the function n (t) in the context
of this situation.
The total number of students who attend the university increases by 114% each year
since 2010.
The number of students who study online at the university increases by 14% each year
since 2010.
The number of students who study online at the university increases by 114% each
year since 2010.
The total number of students who attend the university increases by 14% each year
since 2010.

Math
Basic MathAn ecologist randomly samples 13 plants of a specific species and measures their heights. He finds that this sample has a mean of 16 cm and a standard deviation of 1 cm. If we assume that the height measurements are normally distributed, find a 95% confidence interval for the mean height of all plants of this species. Then find the lower limit and upper limit of the 95% confidence interval.

Math
Basic MathA random sample of 200 individuals working in a large city indicated that 70 are dissatisfied with their working conditions. Based upon this, compute a 95% confidence interval for the proportion of all individuals in this city who are dissatisfied with their working conditions. Then find the lower limit and upper limit of the 95% confidence interval.

Math
Quadratic equationsThe owner of a video store has determined that the cost C, in dollars, of operating the store is approximately given by C(x)=2x² - 22x+780, where x is the number of videos rented daily. Find the lowest cost to the nearest dollar.
A. $841
B. $659
C. $538
D. $720

Math
Basic MathThe length of the first loop of a spring is 20 inches. The length of the second loop is 9/10 the length of the first. The length of the third loop is 9/10 the length of the second, and so on. Suppose the spring had infinitely many loops find the length.

Math
Basic MathAn existing inventory for a test measuring self-esteem indicates that the scores have a standard deviation of 11. A psychologist gave the self-esteem test to a random sample of 150 individuals, and their mean score was 63. Construct a 95% confidence interval for the true mean of all test scores. Then give its lower limit and upper limit.
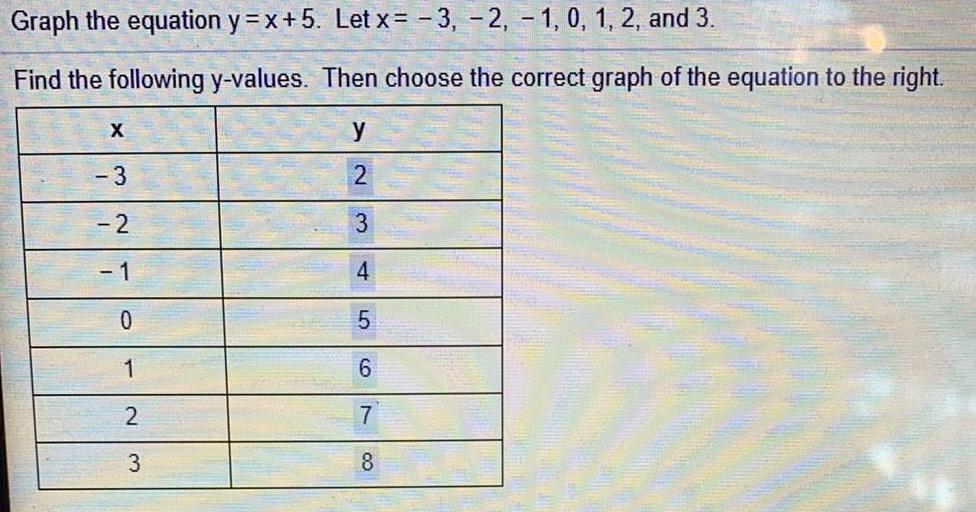
Math
FunctionsGraph the equation y=x+5. Let x = -3, -2, -1, 0, 1, 2, and 3.
Find the following y-values. Then choose the correct graph of the equation to the right.

Math
AreaSuppose an open box with a rectangular base is to be constructed from a rectangular piece of cardboard 12 inches wide and 24 inches long by cutting congruent squares from each corner and then bending up the sides.
What is the maximum possible volume of the box, in cubic inches?

Math
Basic MathOlivia's volleyball team is going on a trip to play in a tournament in Oregon. The 12 players all need to fundraise to pay for their trip. Each player needs to pay $20 for the bus plus some amount for food. The total cost of the entire trip for all 12 is $540. How much extra does each player need to fundraise for food? *
(3 Points)
1 Point: equation
1 Point: solution to written equation
1 Point: solution is labeled with context

Math
Basic MathA prescription medication has a half-life of 6 hours and the recommended dosage of the medicine is 2 pills. If each pill is 500 mg, how long will it take for the amount of the medicine to remain in the body to be 200 mg if a person takes the recommended dosage?

Math
Basic MathTwo factory plants are making TV panels. Yesterday, Plant A produced 8000 panels. Ten percent of the panels from Plant A and 3% of the panels from Plant B were defective. How many panels did Plant B produce, if the overall percentage of defective panels from the two plants was 7%?
Number of panels produced by Plant B:

Math
Basic MathDirections: Find each sum or difference. Answers must be in standard form.
11. (x²-4x + 3) + (3x²-3x - 5)
12. (8r^2 -12r + 4)-(3r²+5r-1)
13. (2m-3+ 7m²)-(3-9m² -2m)
14. (7p² + 3p)-(5p² + 4)
15. (3a²-a + 3) + (4a²-5)
16. (5w³-w+ 2u² + 4) + (3w²+1-4w)
17. (2x² +3y^2-2z^2) — (x² − y² − z²) + (4x² − 3y²)
18. (12+ 8k³ + 3k-4k²) + (5k³ + 15-k + 2k²)
19. Find the sum of (2x² - 6x-2) and (x² + 4x).
20. Subtract (-a² - 5ab + 3b²) from (3a²- 2ab + 3b²).

Math
Trigonometric equationsFor each of the following, identify the amplitude, period, horizontal (phase) shift, vertical shift, domain, range, y-intercept and any x-intercepts.
f(x) = 4 - 2 sin 3 (x - 2π)
Amplitude:
Period:
Hor. Shift:
Vert Shift:
Domain:
Range:
y-int:
x-int:

Math
Basic MathA contractor finds that Crew A takes 6 1/2 - hours to construct a retaining wall and Crew B can do the same job in 5 1/2 hours. If Crew A and Crew B work together, how long will it take them to construct the retaining
wall?
a) Let h= the time to complete the job. Write the equation you would use to solve this problem,
b) Now solve your equation. Give your answer first as a reduced fraction, and then a decimal rounded to 3 places.
Working together, the two crews will construct the wall in which as a decimal is hours.
hours (fraction),

Math
Basic MathMaria read in the newspaper that 59% of voters in her city were voting "no" on a local initiative measure. The newspaper article stated that 772 people were originally polled.
What is the margin of error for this survey?
4.6%
2.6%
3.6%
1.6%

Math
TrigonometryFind the exact value of cos π/3, expressing your answer with a rational denominator.

Math
Basic MathIn testing their recording devices, a team of seismologists positioned two of the devices 2000 feet apart, with the device at point A to the west of the device at point B. At a point between the devices and 200 feet from point B, a small amount of explosive was detonated. A second explosion is to be carried out at a point directly north of point B. How far north should the site of the second explosion be chosen so it is directly north of point B?
Imagine what this would look like on the coordinate plane ... what do we know about the graph and/or equation of this hyperbola?

Math
ProbabilityOut of 75 students, 45 are taking Algebra 2 and 32 are taking Chemistry. Sixteen students are taking both Algebra 2 and Chemistry. If a student is chosen at random, what is the probability that the student is taking Algebra 2 but not Chemistry?

Math
Indefinite IntegrationEvaluate the integral using the fundamental theorem of calculus when appropriate (your answer should be exact. Make sure you use C if needed.):
f(x) = ∫(4x^2 –3x+4+3x-¹-x-^2) da

Math
Basic MathSam bought three folders and one notebook from the office store and spent $8.25. He went back the next day and bought two more folders and three more notebooks and spent $16. If the prices did not change between the days, find the cost of one folder.

Math
Basic MathMrs. Browne wants to start a college fund for her newest addition, Wade. She makes a payment of $50 at the end of each month into an ordinary annuity account paying a 3.5% annual interest rate, compounded monthly. How much will be in the account after 18 years and Wade is ready to go to college?
![Ith (x) = x² + 16x 10 on the interval [-4, 6]:
Apply the mean value theorem to find the value of C in the interval
(-4, 6) such that h' (c) equals the average rate of change of h(x) on
the interval.](https://media.kunduz.com/media/sug-question/raw/45481871-1658260653.3505087.jpeg?w=256)
Math
DifferentiationIth (x) = x² + 16x 10 on the interval [-4, 6]:
Apply the mean value theorem to find the value of C in the interval
(-4, 6) such that h' (c) equals the average rate of change of h(x) on
the interval.

Math
Basic MathIn 2017, the number of students enrolled in a high school was 232. It is estimated that the student population will increase by 3% every year. Estimate the number of students that will be enrolled in 2027.
Math
FunctionsA new dot.com business began with an initial stock offering of $3,000. At the end of the first year, the management conducted a complete top to bottom audit of the company's profits. The audit revealed that the company profit follows the graph of the function P (t) = t³ - ² +t+ 3,
in thousands of dollars where t is months.
During what month did the profit change at the same rate as the average rate of change of the profit during the first six months?
Note: Convert the month to its corresponding number when answering
(e.g., 1 for January, 2 for February, and so on). Round your answer up to the
nearest whole number.

Math
Application of derivativesFermium-257 has a half-life of 100 days. A sample has a mass of 60 mg initially.
I) What is the formula for finding the amount remaining after t days.
II) When will there only be 5 mg remaining?
A) y = 60(2) -t 100; 248 days
B) y = 60 e -t 100; 248 days
C) y = 60(2) -t 100; 358 days
D) y = 60e -t 100; 358 days

Math
Basic MathA 28 ft ladder leans against a wall so that the base of the ladder is 5 ft away from the base of the wall.
How far up the wall does the ladder reach?
Round to the nearest tenth.
Enter your answer, as a decimal, in the box.

Math
ProbabilityEvery day, Carmen walks to the bus stop and the amount of time she will have to wait for the bus is between 0 and 12 minutes, with all times being equally likely (i.e., a uniform distribution). This means that the mean wait time is 6 minutes, with a variance of 12 minutes.
What is the probability that her total wait time over the course of 60 days is less than 6.5 hours?

Math
Basic MathKristina needs to mix a 10% alcohol solution with a 60% alcohol solution to create 100 millileters of a 10% solution. How many millileters of each solution must Kristina use?
Answer: Kristina must mix
millileters of 10% solution and
millileters of 60% solution.

Math
ProbabilityMs. Kelly is organizing a board game day. She has 7 different board games. She needs to organize the order that the games are played that day.
S={Apples To Apples, Balderdash, Carcassonne, Dominion, Terraforming Mars,
Wingspan, Galaxy Trucker}
a) In how many ways can she play all 7 games?
b) In how many ways can she play just 3 of the games?
c) In how many ways can she play just 3 games if the last one must be Terraforming
Mars because that's everyone's favourite?
d) In how many ways can she play just 3 games if Terraforming Mars must be played at some point that day because that's everyone's favourite?
e) Apple to Apples and Balderdash are both considered "party" games, in how many
ways can all the games be played if Apples to Apples and Balderdash are not played
one right after the other?

Math
ProbabilityChoose one of the following questions to answer
Level 4-
A new supermarket was launched in Kitchener. On opening day customers came to the store and were asked how they heard about the grand opening. They were informed via the following methods:
- 119 received flyers in the mail
- 131 learned about the launch through the internet
- 140 heard about the launch through advertisements on television
- 41 were aware of the launch through flyers and the internet
- 35 came to know of it through flyers and television
- 16 heard about it from all three sources
a) Construct a Venn diagram.
b) Calculate n(S), what does that mean in the context of this question?
c) Calculate n(F ∪ T), what does that mean in the context of this question?
Level+4
A survey collects data from people about what pets they like. The choices were cats, dogs, and birds. The results were as follows:
- 95 like cats
- 165 like dogs
-93 like birds
- 75 like cats and dogs
-20 like dogs and birds
- 18 like cats and birds
-10 like none of those choices
There are 265 people surveyed
a) Construct a Venn diagram.
b) Calculate n(D ∩ C ∩ B), what does that mean in the context of this question?
c) Calculate n(C'), what does that mean in the context of this question?

Math
Basic MathHannah can paint a room in 20 hours. Destiny can paint the same room in 12 hours. How long does it take for both Hannah and Destiny to paint the room it they are working together.

Math
Basic MathA town's population has been growing linearly. In 2003, the population was 57000, and the population has been growing by 700 people each year.
Write an equation for the population years after 2003.
P(x) =