Math Questions

The amount of money that a car insurance company pays out in a given week on single-car accidents follows approximately a normal distribution with mean $45,000 and a standard deviation of $10,000. What is the probability that the insurance company will have to pay out more than $60,000 in a given week? 0.067 0.111 0.413 0.933

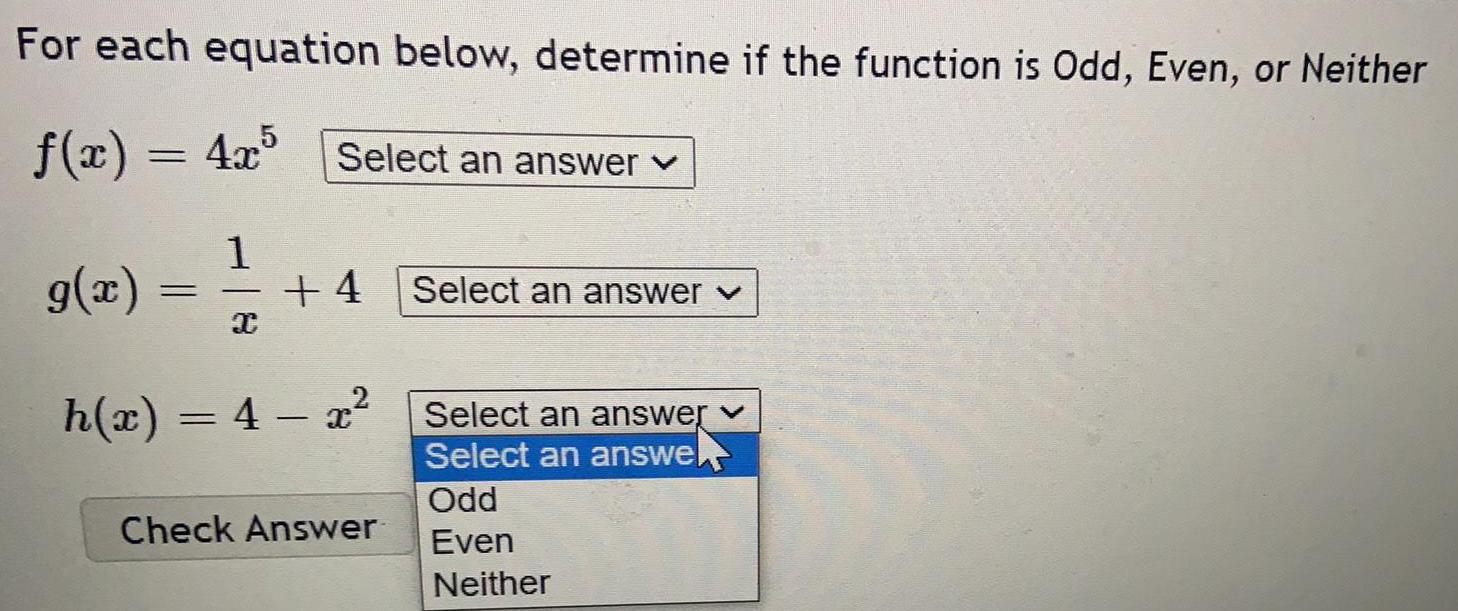
For each equation below, determine if the function is Odd, Even, or Neither f(x) = 4x^5 g(x) = 1/x + 4 h(x) = 4 - x²

A croissant, a cup of coffee, and a fruit bowl from Kelley's Coffee Cart cost a total of $5.25. Kelley posts a notice announcing that, effective next week, the price of a croissant will go up 25% and the price of coffee will go up 35%. After the increase, the total price of the purchase will be $5.74, and a fruit bowl will cost 3 times as much as a croissant. F

Mrs. Travis wants to have a clown deliver balloons to her secretary's office. Clowns R. Fun charges $1.25 per balloon and $6 delivery. Singing Balloons charges $1.95 per balloon and $2 for delivery. What is the minimum number of balloons Mrs. Travis needs to purchase in order for Clowns R Fun to have a lower price than Singing Balloons?

Which of the following most accurately describes the translation of the graph: y=(x-7)² + 16, from the origin (0, 0)? Select one: 7 units down, 16 units right 7 units up, 16 units right 16 units up, 7 units left 16 units up, 7 units right

The circumference of a circular field is 273.18 yards. What is the radius of the field?

Based on past experience, a building contractor sets the probability of winning a contract is 30%. The contract is worth $25,000 and the cost to prepare the contract proposal is $2,400. What is the expected value of the contract proposal? $5,100 $6,780 -$5,100 $22,600

The formula v= √2.5r models the safe maximum speed, v, in miles per hour, at which a car can travel on a curved road with radius of curvature, r, in feet. A highway crew measures the radius of curvature at an exit ramp as 810 feet. What is the maximum safe speed? A. 81 miles per hour B. 50 miles per hour C. 42 miles per hour D. 45 miles per hour

Use technology to find the P-value for the hypothesis test described below. The claim is that for 12 AM body temperatures, the mean is u> 98.6°F. The sample size is n=3 and the test statistic is t= 1.013. (Round to three decimal places as needed.) P-value=

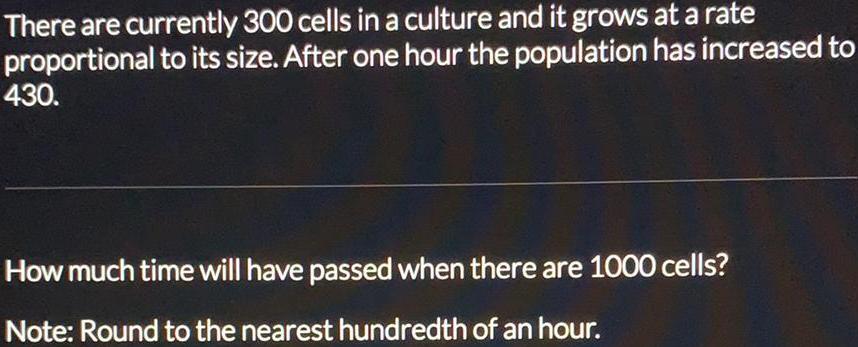
There are currently 300 cells in a culture and it grows at a rate proportional to its size. After one hour the population has increased to 430. How much time will have passed when there are 1000 cells? Note: Round to the nearest hundredth of an hour.

Delaney and Erin both leave the college at the same time, but in opposite directions. If Erin travels 5 mph faster than Delaney and after 7 hours they are 203 miles apart, how fast is each traveling?

Given cot A = 9/2 and that angle A is in Quadrant I, find the exact value of sin A in simplest radical form using a rational denominator.

The total profit function P(x) for a company producing x thousand units is given by P(x) = 2x² + 40x- 168. Find the values of x for which the company makes a profit. [Hint: The company makes a profit when P(x) > 0.] A. x is greater than 6 thousand units B. x is between 6 thousand units and 14 thousand units C. x is less than 6 thousand units or greater than 14 thousand units D. x is less than 14 thousand units

A random sample of 159 full-grown lobsters had a mean weight of 18 ounces; the standard deviation of weights of all lobsters is thought to be σ = 2.8 ounces. Use this data to construct a 98 percent confidence interval for the population mean m of weights of all such lobsters.. 16.9 <m< 18.0 18.1 <m< 20 17.48 <m < 18.517 17.48 <m < 19.52

Classify the hypothesis test as left-tailed, right- tailed, or two-tailed. At one school, the average amount of time that tenth-graders spend watching television each week is21.6 hours. The principal introduces a campaign to encourage the students to watch less television. One year later, the principal wants to perform a hypothesis test to determine whether the average amount of time spent watching television per week has decreased from the previous mean of 21.6 hours. Right-tailed Two-tailed Left-tailed

The quadratic function R(p) = -5.2p² +65p - 75 gives the amount of revenue R(p) in dollars generated by a product priced at p dollars. Question: At what price will the maximum revenue be generated?

Alice Coopersmith is moving to a new home. She can rent a 14-foot panel truck for $40.00 a day plus $0.26 a mile or an 18-foot truck for $55.00 a day plus $0.29 a mile. It would take 4 trips to make the move in the 14-foot truck, but only 3 trips in the larger truck. She estimates that gasoline would cost about $45.00 for either truck. A round-trip to her new home is 60 miles Regardless of the number of trips, she would need the truck for only 1 day. To save on expenses, which size truck should Coopersmith rent? How much would it cost per mile to rent each truck?

The function h(t) gives the height of a rock thrown off the Golden Gate Bridge in feet above the water at t seconds after it is thrown. h(t) = -16t² + 30t + 225 Question: How long after it's thrown will the rock hit the water?

If g(x) is the ƒ(x) = x after a vertical compression by 2/5 a shift right by 4, and a shift down by 2, a) write an equation for g(x): g(x) = b) The slope of this line is: c) The vertical intercept of this line is:

A college student launches a water balloon from the balcony of a building. The balloon's height off of the ground is a function of the amount of time after it is launched and is described by the equation h(t) = -16t² + 40t + 72 where t is time since launch in seconds and h(t) is the height in feet. Question: When will the balloon reach 60 feet above the ground? Enter your final answer rounded to the nearest tenth (one decimal place.) .Enter the correct units for your answer in the second box. IF there is no valid answer enter N/A in all blanks. Answer: The balloon reaches 60 feet after Why is there only one valid answer?

Use the Fundamental Counting Principle to determine the total number of outcomes. 3. Picking a card from a standard deck then rolling a standard die. 4. Choosing a random answer to the last five questions on a multiple- choice test if each question has four answer choices. 5. A mall has four escalators, one elevator, and three stairwells to travel from the second floor to the first floor. They have six doors on the first floor to exit the mall. If Jane is on the second floor, how many ways can she exit the mall?

Select the correct answer. Consider functions fand g. f(x)= x-1/x2+x-1 g(x) = 3² - 2 Using a table of values, what is the approximate solution to the equation f(x)=g(x) to the nearest quarter of a unit?

The ratio of shirts to pants in Sara's closet is 5:2. Which best explains what that means? Sara has 5 shirts for every 2 pairs of pants in her closet Sara only has 7 items of clothing in her closet Sara has 5 more shirts than pants in her closet Sara has 5 pairs of pants for every 2 shirts

Find an equation of the line that passes through the given point and has the indicated slope m. Point (-2,-5) Slope m= 4/5

Lighthouse Master Olivia is atop her 300 ft lighthouse and spots a boat in the distance at an angle of depression of 18º. Along the same line of sight, she spots another boat at an angle of depression of 62º. How far are the boats from other? Round your answer to the nearest tenth. distance = ft.

Ni Hua is an adventurous traveler. He skydives twice in every island he visits and skydives thrice in every peninsula he visits. In the last decade, Ni Hua went skydiving a total of 45 times in the 19 islands and peninsulas that he visited. How many islands and peninsulas did Ni Hua visit? Choose 1 answer: Ni Hua visited 12 islands and 7 peninsulas. Ni Hua visited 7 islands and 12 peninsulas. There is not enough information to determine the exact number of islands and peninsula Ni Hua visited. The given information describes an impossible situation.

How are chords and secants alike? How are they different? They are alike because they both intersect the circle___They are different because chords are____and__ secants are

A sandwich shop is ordering apples and grapes to make chicken salad. Apples cost $2.19 per pound and grapes cost $2.60 per pound. If they ordered a total of 20 pounds of apples and grapes and paid $35.80, how many pounds of grapes did they order?

In Exercises 1-4, find the indicated measure. 1. radius of a circle with a circumference of 42π meters 2. circumference of a circle with a radius of 27 feet 3. circumference of a circle with a diameter of 15 inches 4. diameter of a circle with circumference 39 centimeters

Given that a parabola has its vertex at (-2, 1). Which of the following can not be the function expression of the parabola? f(x) = -(x + 2)²+1 f(x)=(x-2)²+1 f(x)=(x + 2)²+1 f(x)=3(x+2)²+1

Two vessels are pulling a broken-down ship toward a boathouse with forces of 750 lb and 630 lb. The angle between the forces is 34.5°. Determine the magnitude of the equilibrant force. Show your work.

In 2016, 5,200 parking permits were issued in a city. The number of parking permits increases by 5% every year. Let y represent the number of parking permits issued xyears since 2016. Which type of sequence does the situation represent? A The situation represents an arithmetic sequence because the successive y-values have a common difference of 1.5. B. The situation represents a geometric sequence because the successive y-values have a common ratio of 1.5. C. The situation represents an arithmetic sequence because the successive y-values have a common difference of 1.05. D. The situation represents a geometric sequence because the successive y-values have a common ratio of 1.05.

In a Northwest Washington County, the speeding fines are determined by the formula: F(s) = 13(s 40) + 35 where F(s) is the cost, in dollars, of the fine if a person is caught driving at a speed of s miles per hour. If a fine comes to $308, how fast in mph was the person speeding? -

Let S be the universal set, where: S = {1,2,3,4,5,6,7,8,9,10} Let sets A and B be subsets of S, where: Set A={1,2,7,9,10} Set B= {1,2,4,5,6,7,8,10} Find the following: LIST the elements in the set (A'): (A) = { } Enter the elements as a list, separated by commas. If the result is the empty set, enter DNE LIST the elements in the set (B'): (B')={ } Enter the elements as a list, separated by commas. If the result is the empty set, enter DNE You may want to draw a Venn Diagram to help answer this question.

After a 40% reduction, you purchase a new stereo for $273. What was the price of the stereo before the reduction? A) First write an equation you can use to answer this question. Use x as your variable and express any percents in decimal form in the equation. The equation is B) Solve your equation in part [A] to find the original price of the stereo. Answer: The original price of the stereo was dollars.

Claire has received scores of 85, 88, 87, and 95 on her algebra tests. What score must she receive on the fifth test to have an overall test score average of at least 90? A. 95 or greater B. 96 or greater C. 94 or greater D. 93 or greater

The following equation: P = 139t + 9900 gives the total population, P, of a small city t years after 1971. Use the equation to determine when (what year) the population reached a population of 11707 people. Answer: The population reached a total of 11707 people in the year

The perimeter of a rectangle is 48 feet. Describe the possible lengths of a side if the area of the rectangle is to be greater than 95 square feet. A. The length of the rectangle must be greater than 19 ft or less than 5 ft B. The length of the rectangle must lie between 1 and 95 ft C. The length of the rectangle must lie between 5 and 19 ft D. The length of the rectangle must be greater than 19 ft

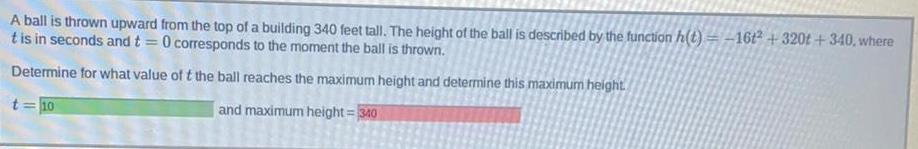
A ball is thrown upward from the top of a building 340 feet tall. The height of the ball is described by the function h(t) = -16t² +320t + 340, where t is in seconds and t = 0 corresponds to the moment the ball is thrown. Determine for what value of t the ball reaches the maximum height and determine this maximum height t=__ and maximum height=___

8) A cylindrical can without a top is to be made to contain 150 cubic centime- ters of liquid. Find the dimensions that will minimize the cost of the metal to make the can. (Recall that the volume formula for a cylinder is: V = r²h.)

Use the given sets below to find the new set. Enter each number separated by a comma. If there are no numbers in the resulting set, leave the answer blank. A = {-9, 2, 6} and B = {-3, 0, 4} AUB={

1. Mallory bought a computer system to start her business from home for $5,995. It is expected to depreciate at a rate of 8% per year. After how many years will the value of her home computer system depreciate to approximately $2,250. Hint: depreciate means decay

Determine whether the following are permutations or combinations The ways to form a three person committee of a chair, a treasurer, and a secretary from a group of eighteen people The ways to form a three 1. Permutations person committee from a group of eighteen people The ways to arrange the 2. Combinations letters Q, U, E, S, T, I, O, N The ways to get exactly three heads in ten tosses of a coin

A wire of length 15 is cut into two pieces which are then bent into the shape of a circle of radius r and a square of side a. Then the total area enclosed by the circle and square is the following function of sand r If we solve for s in terms of r. we can reexpress this area as the following function of r alone Thus we find that to obtain maximal area we should let r To obtain minimal area we should let r

A function f(z) and interval [a, b] are given. Check if the Mean Value Theorem can be applied to fon [a, b]. If so, find all values c in [a, b] guaranteed by the Mean Value Theorem Note, if the Mean Value Theorem does not apply, enter DNE for the c value. C= (Separate multiple answers by commas.) f(x) =z2-1/z²-16 on [0,8]

Geraldo earns $505 from his summer job and deposits it in a savings account that earns 4% simple interest. After 3 years, he moves all his money to another savings account at another ank that earns 6.5% simple interest. What formula below can be used to calculate Geraldo's total account balance after 7 years? (505 x 0.04 x 3) + (565 x 0.065 x 4) [(505 x 0.4 x 3) x 0.65 x 7] [(505 x 0.04 x 3 + 505) x 0.065 x 4]+ (505 x 0.04 x 3 +505) (505 x 0.065 x 7) + (505 x 0.04 x 3)

Alex is taking two courses; algebra and history. Student records indicate that the probability of passing algebra is 0.55, that of passing history is 0.4, and that of passing at least one of the two courses is 0.7. Find the probability that Alex will pass both courses. • Write the probability as a decimal For example, 0.22

The Franklins inherited $3,500, which they want to invest for the child's future college expenses. If they invest it at 8.25% with interest compounded monthly, determine the value of the account, in dollars, after 5 years. $5,270.61 $6,200.53 $3,200.47 $5,279.61

Points (4, 5) and (10, 5) are vertices of a rectangle. The length of the rectangle is twice the width. The other vertices are located at (4,) and (10,). What could these ordered pairs be?

A lumber yard has fixed costs of $7259.40 per day and variable costs of $0.07 per board-foot produced. Lumber sells for $1.87 per board-foot. How many board-feet must be produced and sold daily to break even?