Math Questions
The best high school and college tutors are just a click away, 24×7! Pick a subject, ask a question, and get a detailed, handwritten solution personalized for you in minutes. We cover Math, Physics, Chemistry & Biology.
Math
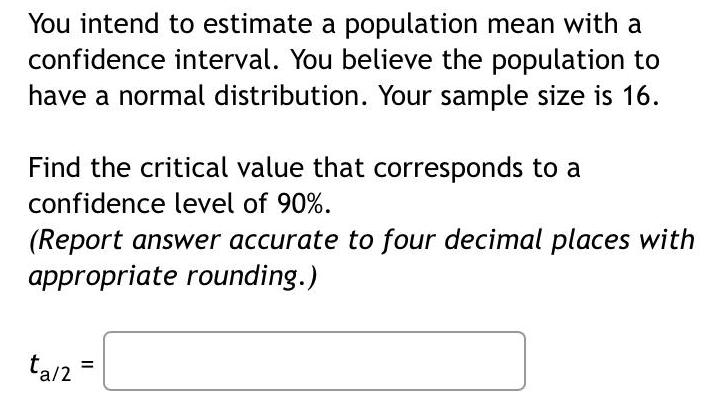
StatisticsYou intend to estimate a population mean with a confidence interval. You believe the population to have a normal distribution. Your sample size is 16. Find the critical value that corresponds to a confidence level of 90%.

Math
Basic MathA caravan of camels has a growth rate of 17%. If the initial population is 230 and we assume an exponential growth model, which of the following models the camel population?
(a) P(t) = 2300.17t
(b) P(t)=230e¹
(c) P(t) = 230(0.17)
(d) P(t) = 230(1.17)"

Math
Basic MathMark and Don are planning to sell each of their marble collections at a garage sale. If Don has 6 more than 3 times the number of marbles Mark has, how many does each boy have to sell if the total number of marbles is 98? Show your work and explain how you arrived at your answers. There are sample student explanations in the feedback to questions 1, 3, 5, 9, 11, and 20 that show the level of detail that is expected in your explanations.

Math
Basic MathSolve the compound inequality.
x< 1/1 orx>-1/3
Select the correct choice below and, if necessary, fill in the answer box to complete your choice.
A. The solution set is
B. The solution set is Ø.

Math
Basic MathSolve the compound inequality.
-5x≤ - 10 or 2x-222 - 12
Select the correct choice below and, if necessary, fill in the answer box to complete your choice.
A. The solution set is
B. The solution set is Ø.

Math
Basic MathThe savings in a bank account can be modeled using S=1250e045t, where t is the number of years the
money has been in the account. Determine, to the nearest tenth of a year, how long it will take for the
amount of savings to double from the initial amount deposited of $1250.
Math
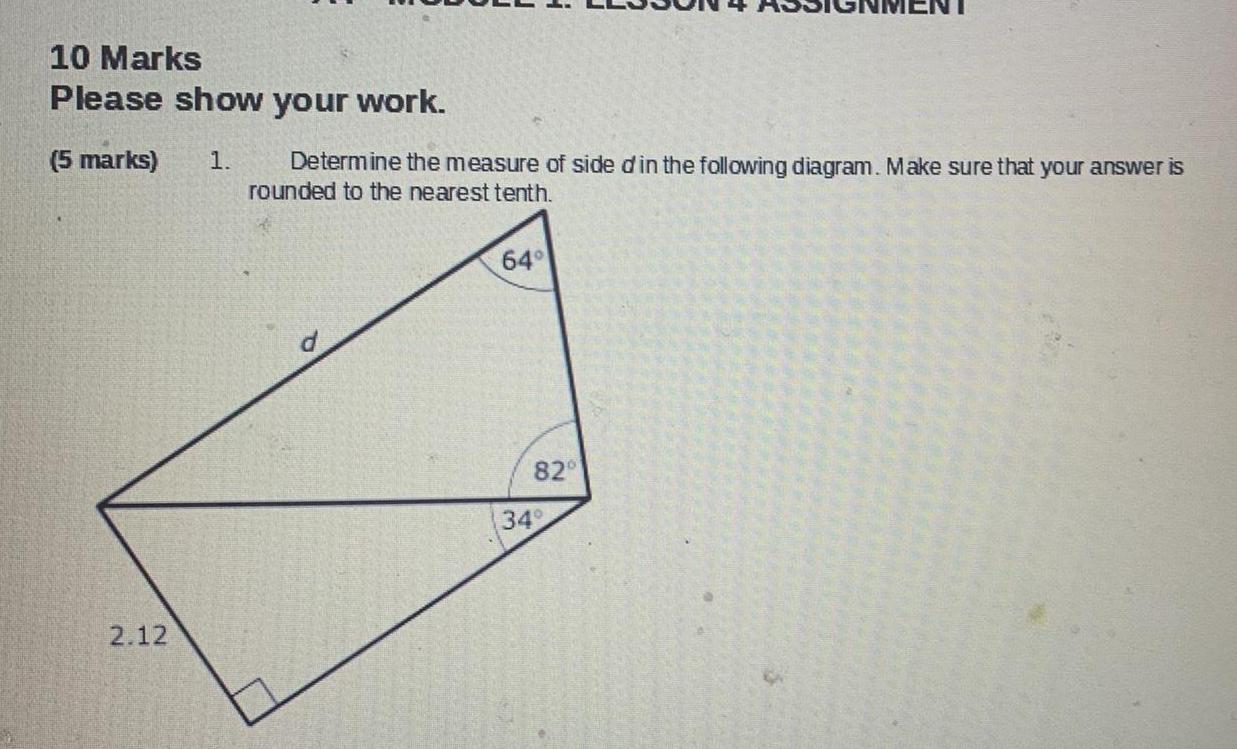
Solution of trianglesDetermine the measure of side d in the following diagram. Make sure that your answer is rounded to the nearest tenth.

Math
Mathematical ReasoningIn the year 2019, 14% of the college students said they didn't like their roommate. Suppose that several people took random samples of 150 college students from the population and computed the percent of students who didn't like their roommate. What would be the shape of the sampling distribution? skewed left uniform approximately normal skewed right
Math
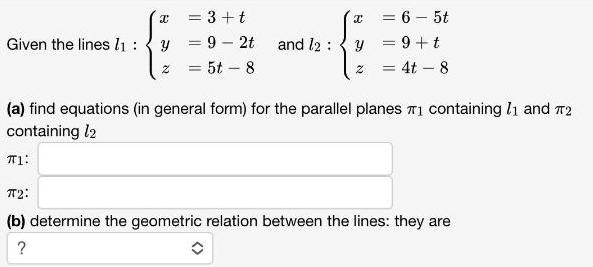
Basic MathGiven the lines I₁:
x = 3+t
y = 9-2t
z = 5t - 8
and I2:
x = 6-5t
y = 9+t
z = 4t - 8
(a) find equations (in general form) for the parallel planes π1 containing I1 and π2
containing I2
π2 :
π1 :
(b) determine the geometric relation between the lines: they are ?
Math
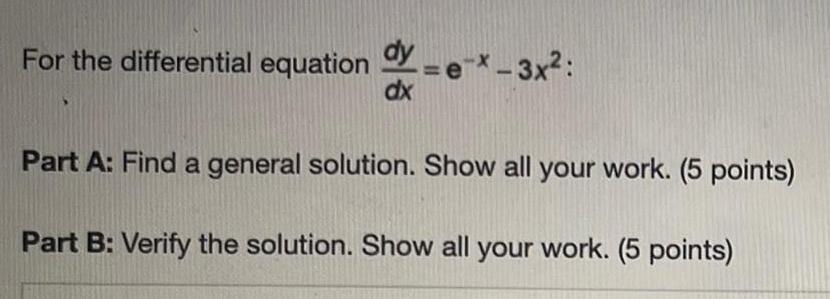
Differential equationsFor the differential equation dy/dx =e-x-3x²:
Part A: Find a general solution. Show all your work.
Part B: Verify the solution. Show all your work.
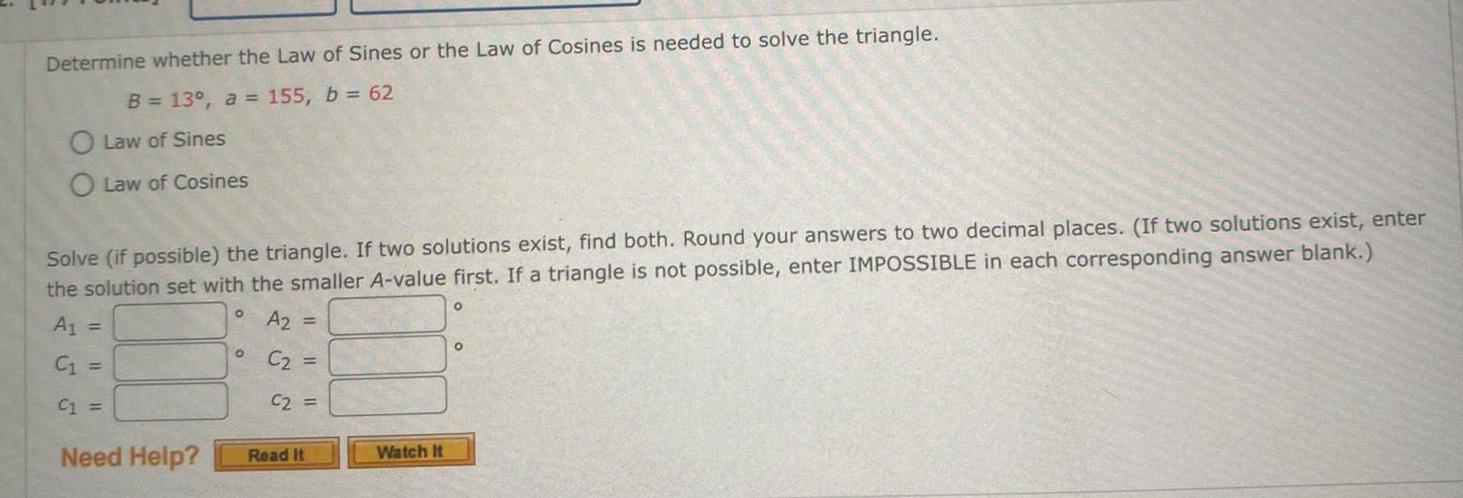
Math
Solution of trianglesDetermine whether the Law of Sines or the Law of Cosines is needed to solve the triangle.
B = 13°, a = 155, b = 62
Law of Sines
Law of Cosines
Solve (if possible) the triangle. If two solutions exist, find both. Round your answers to two decimal places. (If two solutions exist, enter
the solution set with the smaller A-value first. If a triangle is not possible, enter IMPOSSIBLE in each corresponding answer blank.)

Math
Sets and RelationsIf A = {x | x is an odd integer), B = {x|x is an even integer), C= {2, 3, 4, 5), and D={10, 11, 12, 13), list the element(s) of the following set.

Math
Basic MathA bank operates both a drive-up facility and a walk-up window. On a randomly selected day, let X be the proportion of time that the drive-up facility is in use (at least one customer is being served or waiting to be served) and Y be the proportion of time that the walk- up window is in use. The joint PDF is What is the probability that neither window is busy more than one-quarter of the time?

Math
Mathematical InductionFlu is spreading exponentially at a school. The number of new flu patients can be modeled using the equation F = 10e0.12d, where d represents the number of days since 10 students had the flu. (a) How many days will it take for the number of new flu patients to equal 50? Determine your answer algebraically using the natural logarithm. Round your answer to the nearest day.
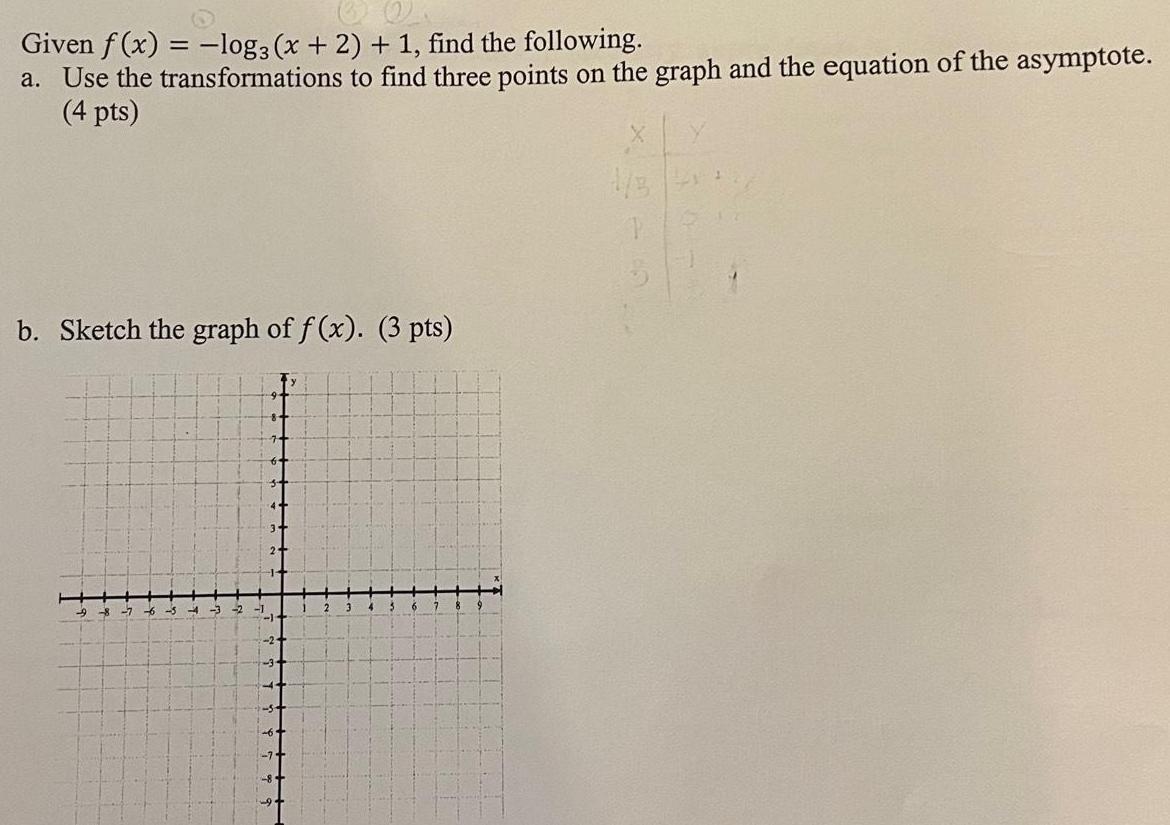
Math
FunctionsGiven f(x) = -log3 (x + 2) + 1, find the following. a. Use the transformations to find three points on the graph and the equation of the asymptote. b. Sketch the graph of f(x).

Math
Basic MathThe area of the region that lies to the right of the y-axis and to the left of the parabola x = 2y - y2 (the shaded region in the figure) is given by the integral f (2y - y2) dy. (Turn your head clockwise and think of the region as lying below the curve x = 2y = y² from y 0 to y = 2.) Find the area of the region.
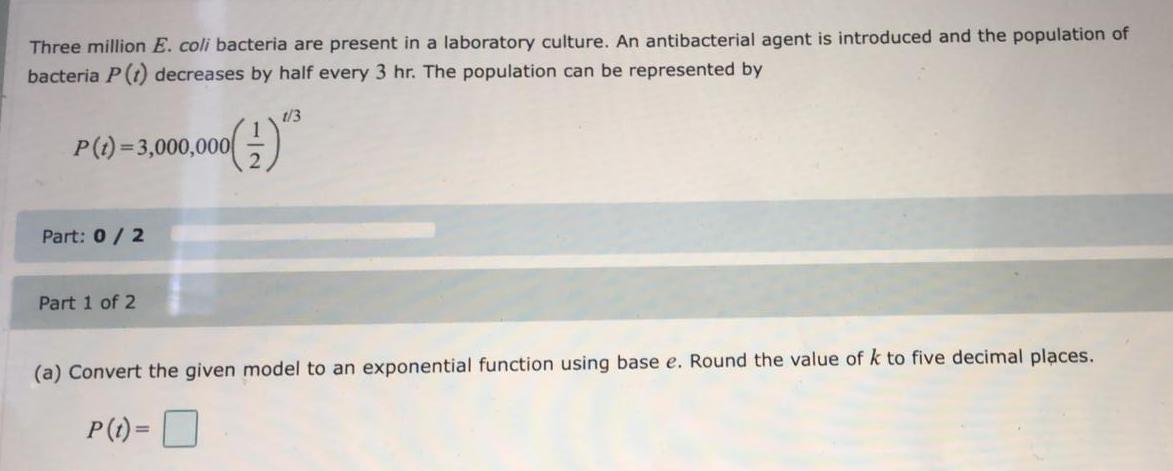
Math
Basic MathThree million E. coli bacteria are present in a laboratory culture. An antibacterial agent is introduced and the population of bacteria P (1) decreases by half every 3 hr. The population can be represented by (a) Convert the given model to an exponential function using base e. Round the value of k to five decimal places.
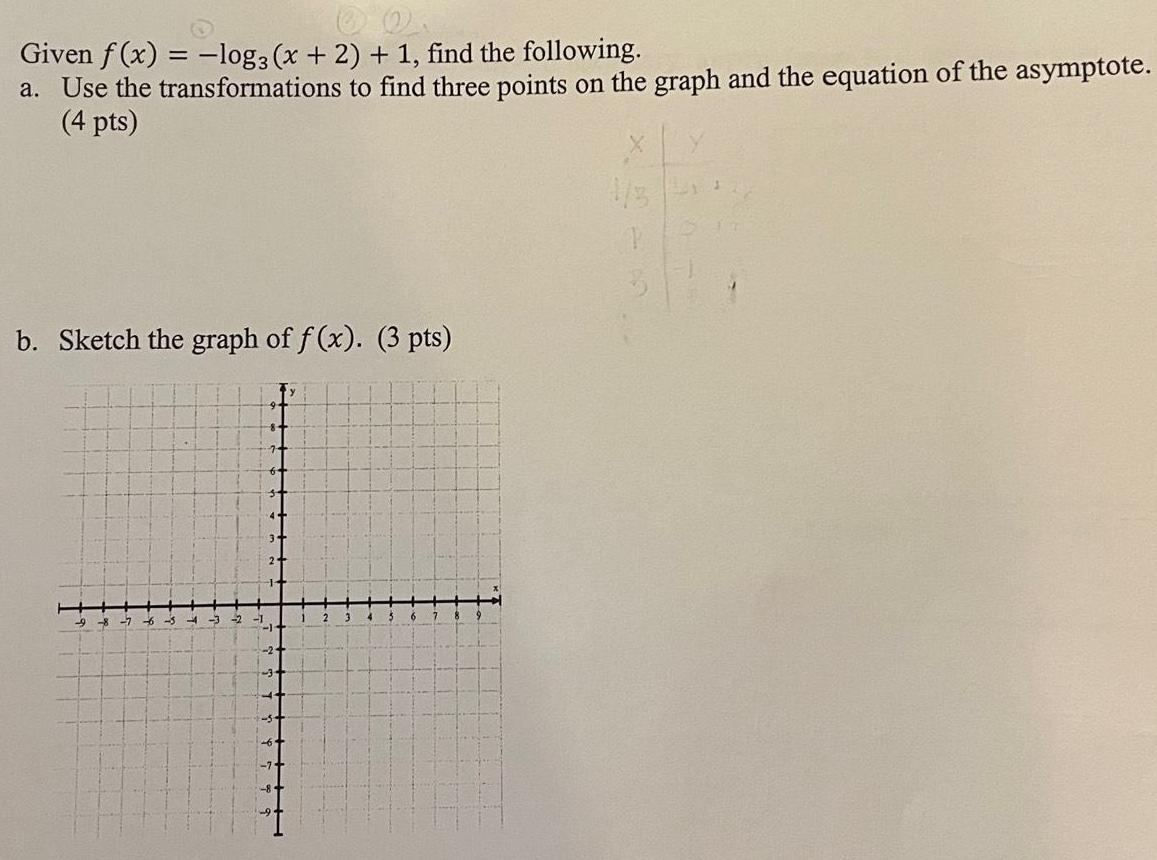
Math
LogarithmsGiven f(x) = -log3 (x + 2) + 1, find the following.
a. Use the transformations to find three points on the graph and the equation of the asymptote.
b. Sketch the graph of f(x).
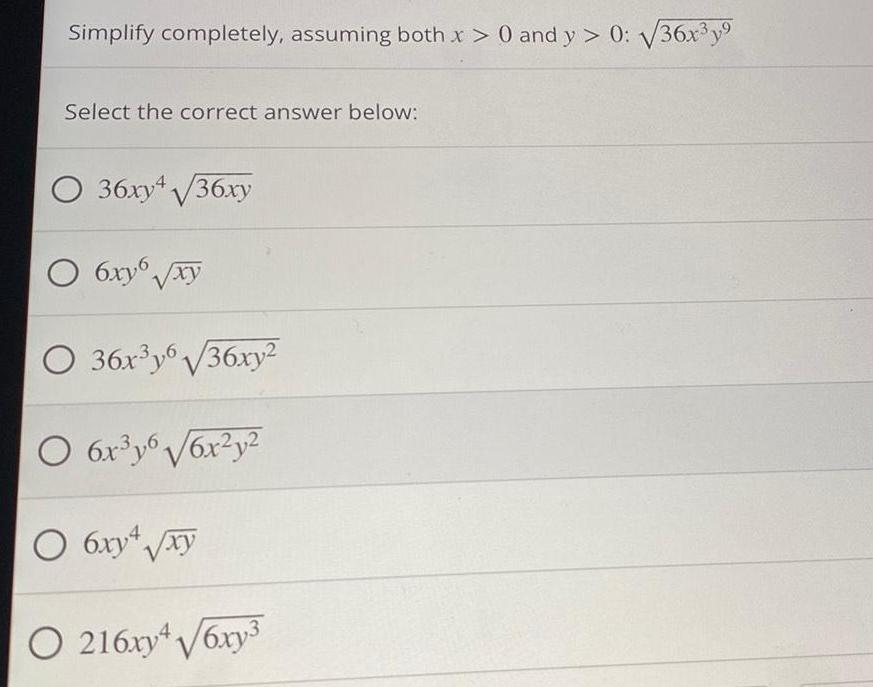
Math
Basic MathSimplify completely, assuming both x > 0 and y> 0: √36x³y⁹
Select the correct answer below:
36xy4√36xy
6xy √xy
36x³y6√36xy2
6x³y6 √6x²y²
6xy √√xy
216xy4√6xy3

![Let f(x) = ²-3t + 2 dt. We'll take the domain of ƒ to be a 20.
(a) Find the intervals of increase or decrease of f(x) and any local extrema.
(b) Find the intervals of concavity of f(x) and any points of inflection.
(c) Find the absolute max and min of f(x) on the interval [0,3].](https://media.kunduz.com/media/sug-question/raw/77564311-1660484956.3946884.jpeg?w=256)
Math
FunctionsLet f(x) = ²-3t + 2 dt. We'll take the domain of ƒ to be a 20.
(a) Find the intervals of increase or decrease of f(x) and any local extrema.
(b) Find the intervals of concavity of f(x) and any points of inflection.
(c) Find the absolute max and min of f(x) on the interval [0,3].


Math
StatisticsIn a 2018 study, research finds that 45% of people own an iPhone. It is hypothesised that every year that percentage gets higher. Therefore,in 2019 a research company completes a study and finds that 480 of 1023
people owned an iphone.
a) State the null and alternative hypothesis
b) Find the p value. (Round to the nearest tenth)
c) Using a 5% significance level, state a conclusion to either reject or fail to reject the null hypothesis.

Math
Basic MathCompound interest is given by the formula A P(1 + r). Where A is the balance of the account
after t years, and P is the starting principal invested at an annual percentage rate of r, expressed as a decimal. Erik invested $7200 in a savings account that pays 11% interest compounded annually and plans to leave it there for 20 years. Determine what Erik 's ending balance will be after 20 years.
After 20 years, Erik will have a balance of $ Round your answer to the nearest cent. in his savings account.
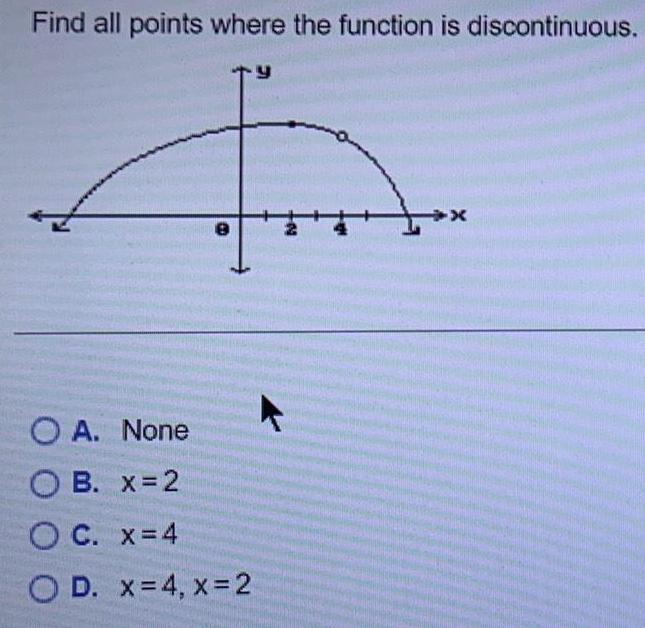
Math
Limits and ContinuityFind all points where the function is discontinuous.
A. None
B. x=2
C. x = 4
D. x = 4, x = 2
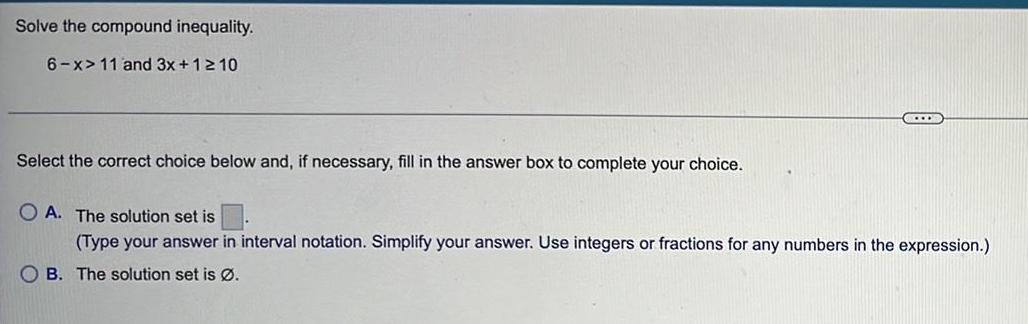
Math
Basic MathSolve the compound inequality.
6-x> 11 and 3x + 1 ≥10
Select the correct choice below and, if necessary, fill in the answer box to complete your choice.
A. The solution set is
B. The solution set is Ø.
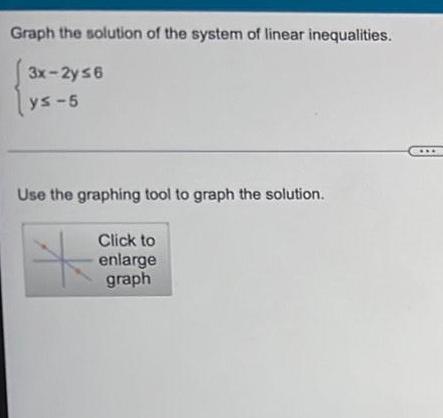
Math
Basic MathGraph the solution of the system of linear inequalities.
(3x-2y≤6
y≤-5
Use the graphing tool to graph the solution.
Click to enlarge graph

Math
Basic MathWe want to know what percent of people get their coffee from a gas station. A random sample of 50 people found that 20% had gotten their coffee from the gas station. All conditions for inference have been met.
a) What is the point estimate, the sample size and the critical z value for the sample?
a) Find a 90% confidence interval. Show your work.

Math
Quadratic equationsA jazz group on tour has been drawing average crowds of 1000 people. It is projected that for every $1 increase in the $17 ticket price, the average attendance will decrease by 60. What equation could be used to solve this problem and at what ticket price will nightly receipts be $16,400?
A) (17+x)(1000-60x)=16400;
$20
B) (17x)(1000x-60)=16400;
$20
C) (17+x)(1000-60x)=16400;
$19
D) (17-x)(1000+60x)=16400;

Math
Basic MathTogether, Kali and Geoff bake 10 pies for the bake sale in 2 hours. Then they make another 6 pies, but Kali works for 45 minutes while Geoff works for an hour and a half. Write and solve a system of equations to find the pie making speed of each person per hour.
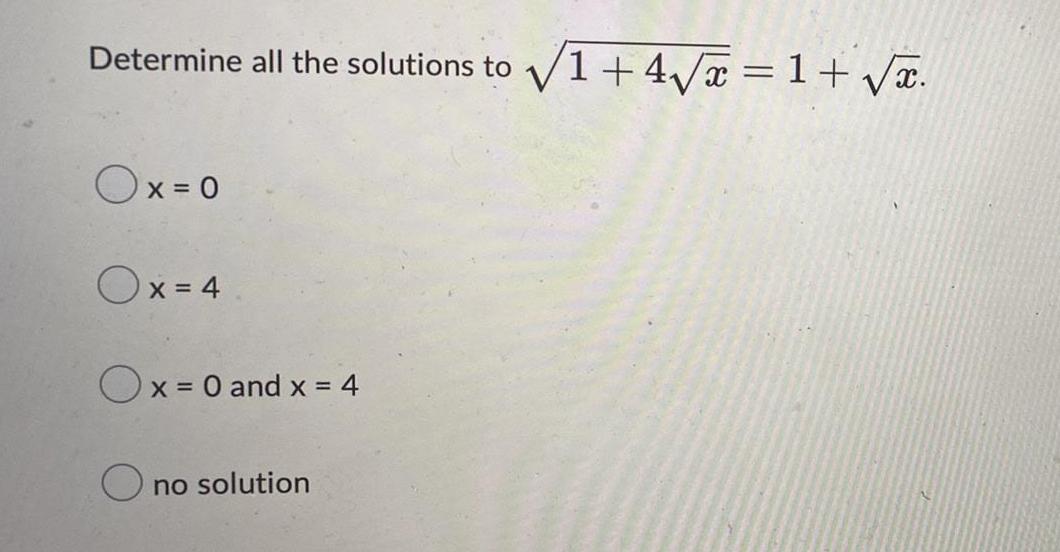

Math
Sets and RelationsIf A = {x|x is an odd integer), B = {x|x is an even integer), C = {2, 3, 4, 5), and D={15, 16, 17, 18), list the element(s) of the following set.
Math
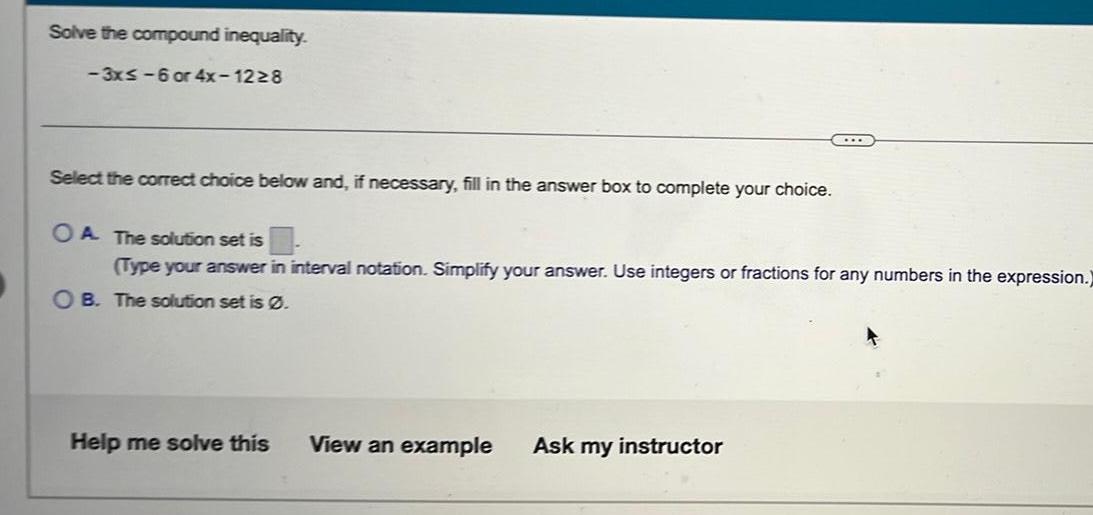
Sets and RelationsSolve the compound inequality.
-3x≤-6 or 4x-1228
Select the correct choice below and, if necessary, fill in the answer box to complete your choice.
A. The solution set is
B. The solution set is Ø.
Math
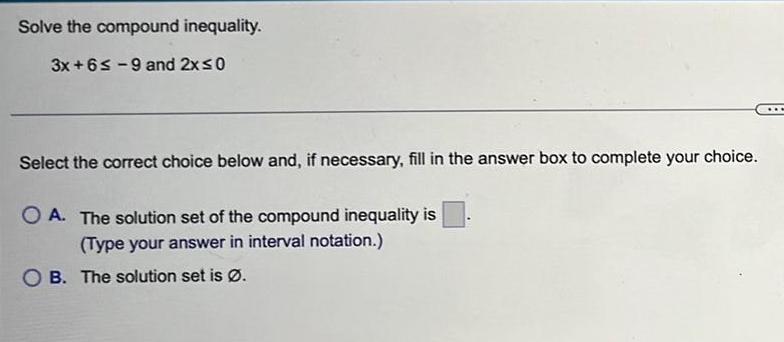
Basic MathSolve the compound inequality.
3x+6≤-9 and 2x≤0
Select the correct choice below and, if necessary, fill in the answer box to complete your choice.
A. The solution set of the compound inequality is
B. The solution set is Ø.
Math
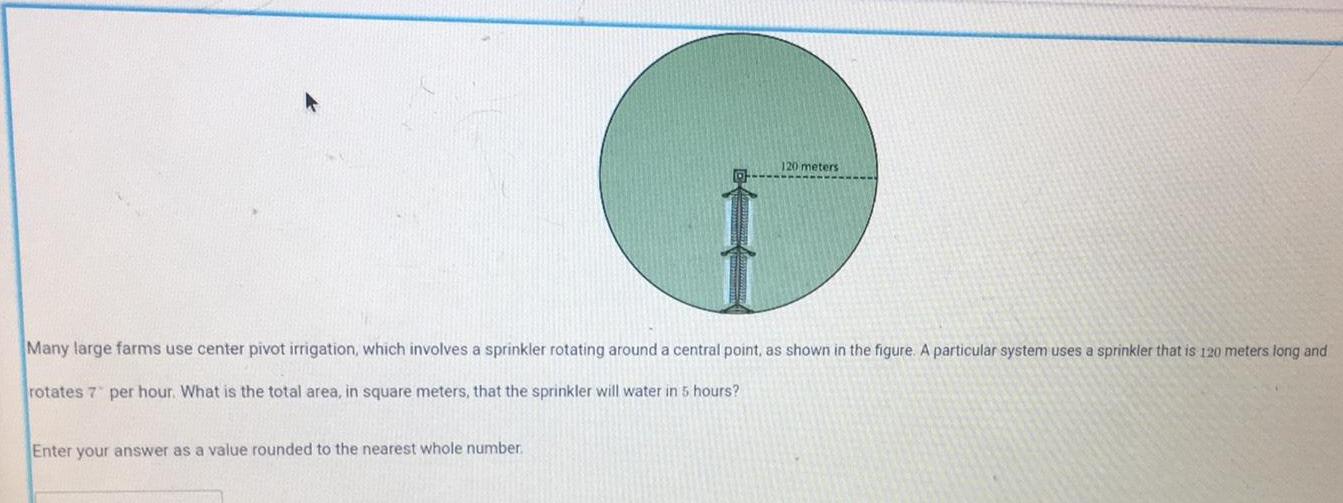
Basic MathMany large farms use center pivot irrigation, which involves a sprinkler rotating around a central point, as shown in the figure. A particular system uses a sprinkler that is 120 meters long and rotates 7 per hour. What is the total area, in square meters, that the sprinkler will water in 5 hours?
Enter your answer as a value rounded to the nearest whole number.

Math
VectorsDetermine whether u and v are equivalent. Explain.
Vector Initial Point Terminal Point
Interpret your results.
u and v have the same magnitude and direction so they are equivalent.
u and v have the same magnitude but not the same direction so they are not equivalent.
u and v have the same direction but not the same magnitude so they are not equivalent.
u and v do not have the same magnitude or direction so they are not equivalent.

![Consider the differential equation
dy/dx = x² (y + 1).
Sketch a slope field for the given differential equation on your paper for the domain [-3, 3] and the range [-3, 3].
Part A: List five coordinates and the corresponding slope of their segments.
Part B: Using the slope field that you drew, describe all points in the xy-plane for which the slopes are negative.](https://media.kunduz.com/media/sug-question/raw/77435227-1660484498.63686.jpeg?w=256)
Math
Application of derivativesConsider the differential equation
dy/dx = x² (y + 1).
Sketch a slope field for the given differential equation on your paper for the domain [-3, 3] and the range [-3, 3].
Part A: List five coordinates and the corresponding slope of their segments.
Part B: Using the slope field that you drew, describe all points in the xy-plane for which the slopes are negative.

Math
StatisticsBenji has $3,100 in a savings account which earns 7% interest compounded quarterly. He made no
additional withdrawals or deposits. What was the amount in the account after one quarter?
O $3,145.25
O $3,255.25
O $3,451.25
O $3,154.25
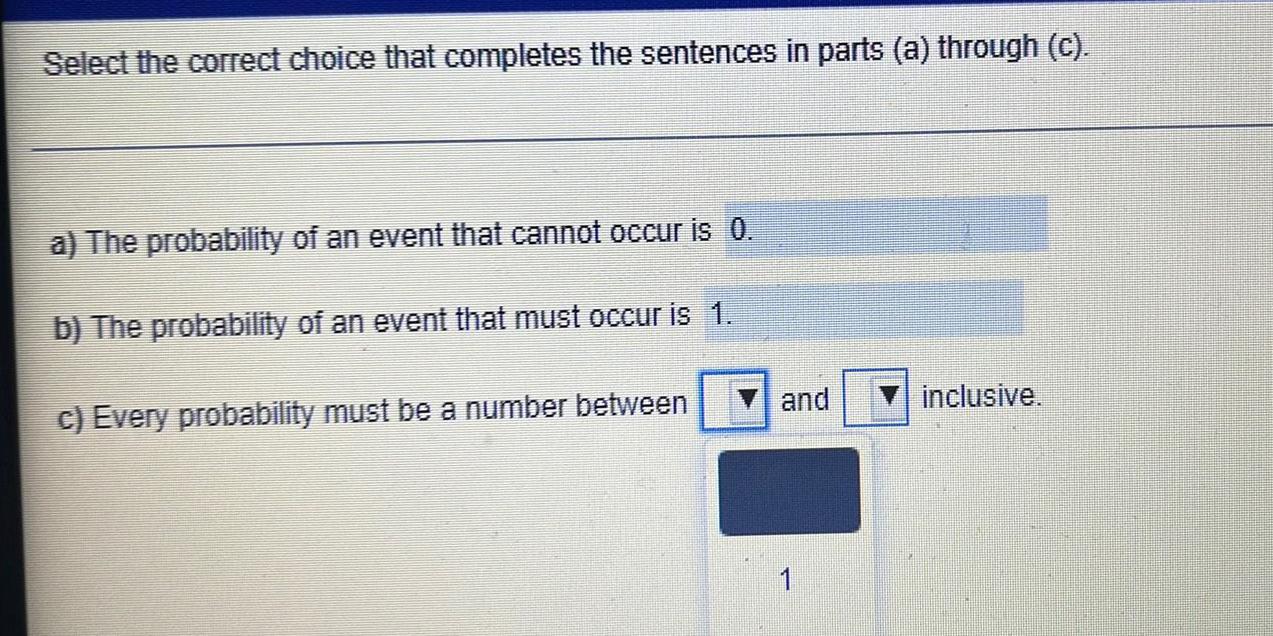
Math
ProbabilitySelect the correct choice that completes the sentences in parts (a) through (c).
a) The probability of an event that cannot occur is 0.
b) The probability of an event that must occur is 1.
c) Every probability must be a number between
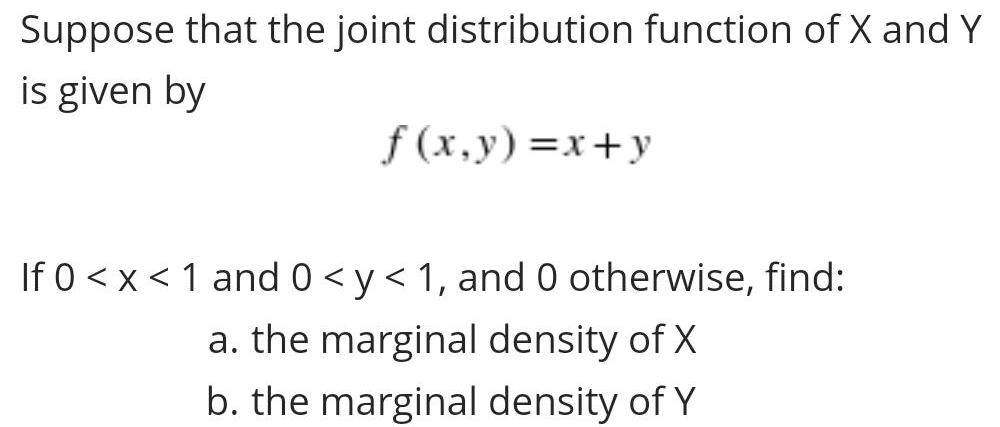
Math
StatisticsSuppose that the joint distribution function of X and Y
is given by
f(x,y)=x+y
If 0<x< 1 and 0 <y< 1, and 0 otherwise, find:
a. the marginal density of X
b. the marginal density of Y

Math
Basic MathA golden rectangle is said to be one of the most visually appealing of all geometric forms. The front of the Parthenon, built in Athens Greece in the 5th century B.C. is a golden rectangle. In a golden rectangle, the length I and the height h of the rectangle must satisfy the equation
l/h = h/l - h
If a rectangular billboard is to have a height of 10 feet, how long should it be if it is to form a golden rectangle? Round your answer to the nearest tenth of a foot.
A) 16.4 ft
B) 18.2 ft
C) 15.9 ft
D) 17.2 ft

Math
Differential equationsIf f(x) = 2² +1, use the limit definition of the derivative to compute f'(z). You can use derivative rules to check your answer, but answers calculated without using the definition of derivative will receive very little credit.

Math
Basic MathTrisha's car cost $10,900. She has enough money to make a down payment of $1,000. Her monthly payments are $250 for 48 months. True or False: The deferred price is $2,100 greater than the original price.
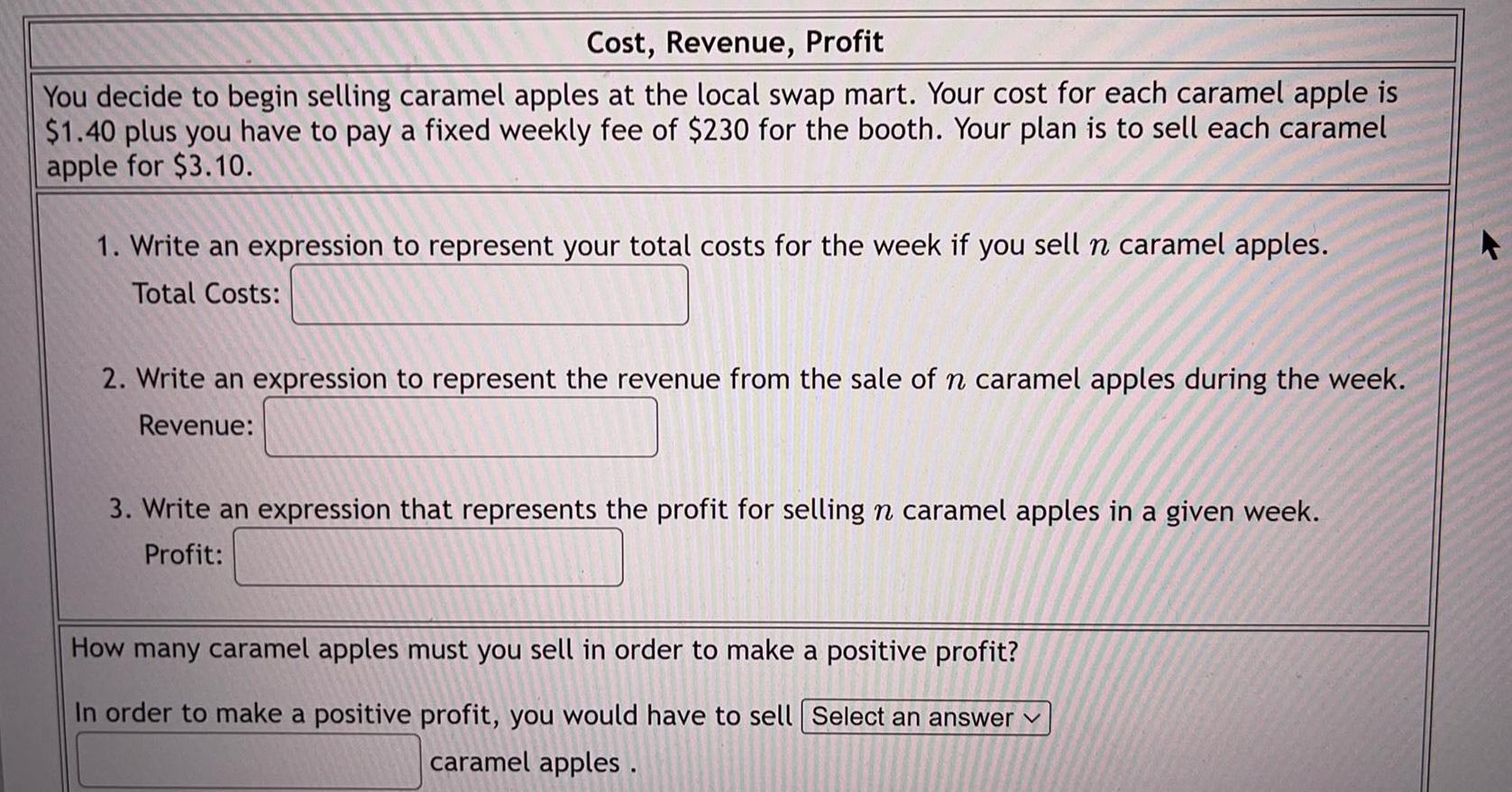
Math
Basic MathYou decide to begin selling caramel apples at the local swap mart. Your cost for each caramel apple is
$1.40 plus you have to pay a fixed weekly fee of $230 for the booth. Your plan is to sell each caramel
apple for $3.10.
1. Write an expression to represent your total costs for the week if you sell n caramel apples.
Total Costs:
2. Write an expression to represent the revenue from the sale of n caramel apples during the week.
Revenue:
3. Write an expression that represents the profit for selling n caramel apples in a given week.
How many caramel apples must you sell in order to make a positive profit?
In order to make a positive profit, you would have to sell.
Math
Basic MathAlice and Wyatt depart Alice's house at the same time. Alice drives due North at 30 mph and Wyatt drives due East at 40 mph. One hour later, Alice will be 30 miles due north of the house, and Wyatt will be 40 miles due east of the house. At what rate is the distance between Alice and Wyatt changing at this time? Diagrams are included below for your convenience. You are NOT required to use them.
Dynamic Diagram
Static Diagram
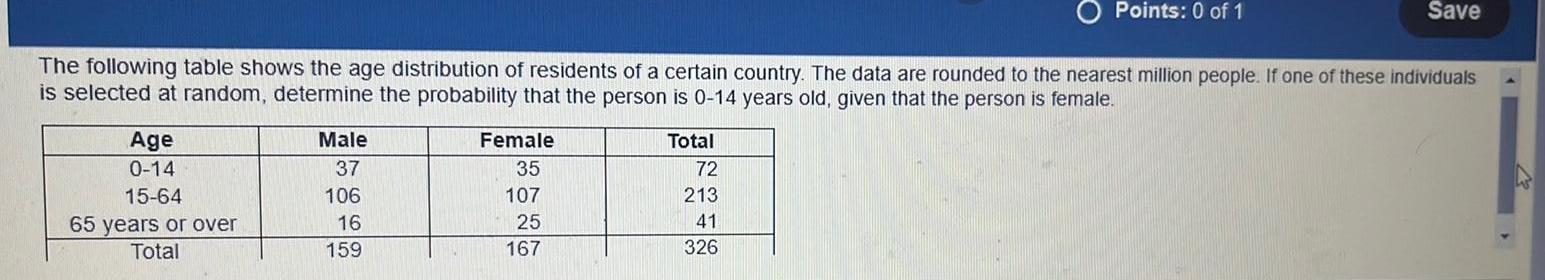
Math
ProbabilityThe following table shows the age distribution of residents of a certain country. The data are rounded to the nearest million people. If one of these individuals is selected at random, determine the probability that the person is 0-14 years old, given that the person is female.
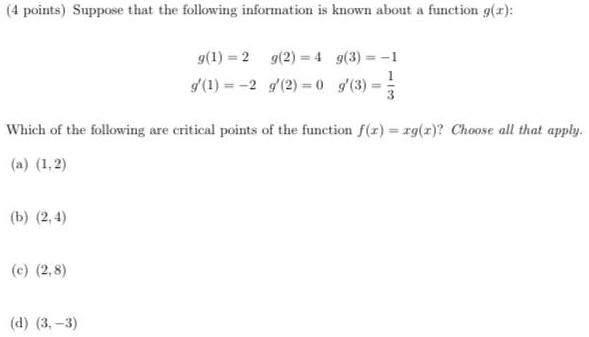
Math
Basic MathSuppose that the following information is known about a function g(x):
(b) (2,4)
(c) (2,8)
g(1)=2 g(2)=4 g(3) =-1
g'(1) -2 g'(2)=0 g'(3)=
Which of the following are critical points of the function f(x)=xg(r)? Choose all that apply.
(a) (1,2)
(d) (3,-3)
![Solve the inequality
x +4| < |x| Hint: use the fact that |x| = √² and x + 4 = √(x+4)² so x +4|< x implies √(x+4)² <√x² impying further that (x+4)² < x². Now you finish.
A) (-∞, - 2)
B) (-∞0, 2)
Og) (-4, ∞)
D) (-∞, -2]](https://media.kunduz.com/media/sug-question/raw/77434021-1660484147.8869233.jpeg?w=256)
Math
Basic MathSolve the inequality
x +4| < |x| Hint: use the fact that |x| = √² and x + 4 = √(x+4)² so x +4|< x implies √(x+4)² <√x² impying further that (x+4)² < x². Now you finish.
A) (-∞, - 2)
B) (-∞0, 2)
Og) (-4, ∞)
D) (-∞, -2]
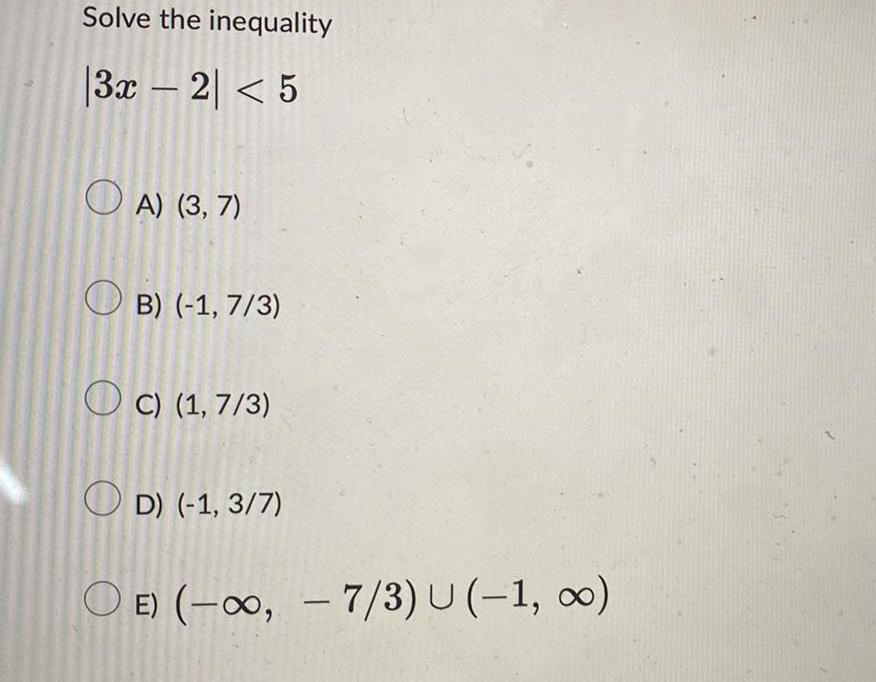
Math
Basic MathSolve the inequality
|3x2| < 5
A) (3,7)
B) (-1,7/3)
C) (1,7/3)
D) (-1,3/7)
E) (-∞, -7/3) U (-1, ∞)