Math Questions
The best high school and college tutors are just a click away, 24×7! Pick a subject, ask a question, and get a detailed, handwritten solution personalized for you in minutes. We cover Math, Physics, Chemistry & Biology.

Math
FunctionsSolve each exponential equation. Use a calculator to write the answer in decimal form (to at least 4 decimal places).
a. 3^x = 4
x=
b. 4^x = 3

Math
Basic MathA company's lawn seed mixtures contain three types of seeds: bluegrass, rye, and Bermuda. The costs per pound of the three types are 13 cents, 18 cents, and 7 cents, respectively. In each batch there must be at least 20% bluegrass seed and the amount of rye must be at least two-thirds the amount of Bermuda. To fill current
orders the company must make at least 9000 pounds of the mixture. How much of each kind of seed should be used to minimize cost?
The minimum cost of $ occurs with a mixture of pounds of bluegrass seed, pounds of rye seed, and pounds of Bermuda seed.
(Simplify your answers. Do not include the $ symbol in your answers.)
Math
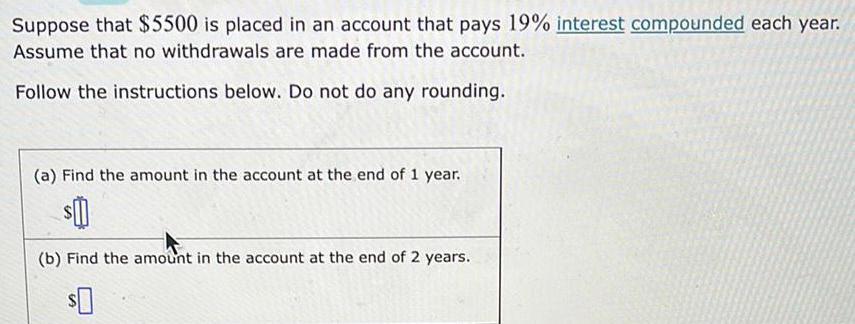
Basic MathSuppose that $5500 is placed in an account that pays 19% interest compounded each year.
Assume that no withdrawals are made from the account.
Follow the instructions below. Do not do any rounding.
(a) Find the amount in the account at the end of 1 year.
(b) Find the amount in the account at the end of 2 years.
$

Math
StatisticsIf 15% of adults in a certain country work from home what is the probability that fewer than 30 out of a random sample of 250 adults will work from home? (Round your answer to 3 decimal places)

Math
StatisticsA researcher studying public opinion of proposed Social Security changes obtains a simple random sample of 50 adult Americans and asks them whether or not they
support the proposed changes. To say that the distribution of the sample proportion of adults who respond yes, is approximately normal, how many more adult
Americans does the researcher need to sample in the following cases?
(a) 15% of all adult Americans support the changes
(b) 20% of all adult Americans support the changes
(a) The researcher must ask___more American adults. (Round up to the nearest integer.)
Math
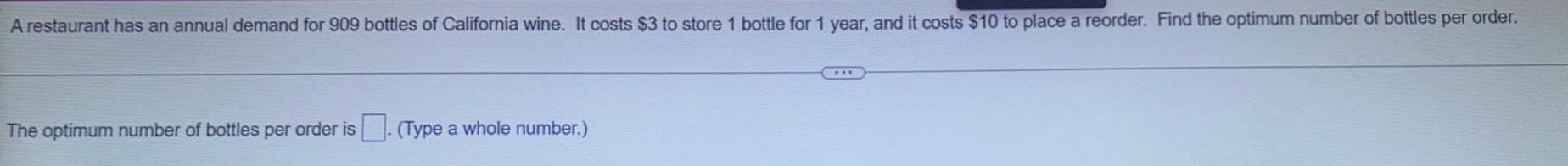
Basic MathA restaurant has an annual demand for 909 bottles of California wine. It costs $3 to store 1 bottle for 1 year, and it costs $10 to place a reorder. Find the optimum number of bottles per order.
The optimum number of bottles per order is. (Type a whole number.)
Math
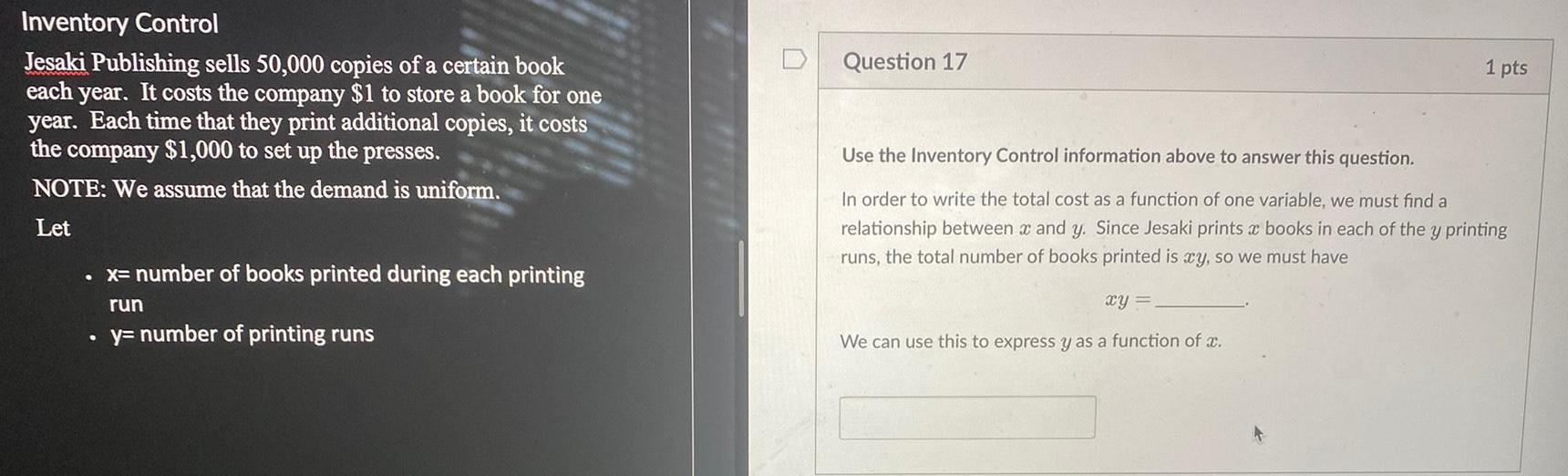
Basic MathInventory Control Jesaki Publishing sells 50,000 copies of a certain book each year. It costs the company $1 to store a book for one year. Each time that they print additional copies, it costs the company $1,000 to set up the presses. NOTE: We assume that the demand is uniform. Let x= number of books printed during each printing run y= number of printing runs Use the Inventory Control information above to answer this question. In order to write the total cost as a function of one variable, we must find a relationship between x and y. Since Jesaki prints a books in each of the y printing runs, the total number of books printed is ay, so we must have
xy=
We can use this to express y as a function of a.

Math
StatisticsA student satisfaction survey was completed with respect to the middle school
cafeteria. Two methods were discussed. Decide which method is better and why.
Method A: A survey of 65 students randomly selected from a list of all students in
the school was conducted. Sixty percent said they are satisfied with the food in the
cafeteria.
Method B: 40 students in two seventh-grade math classes were surveyed and 70%
stated they were satisfied with the food in the cafeteria.
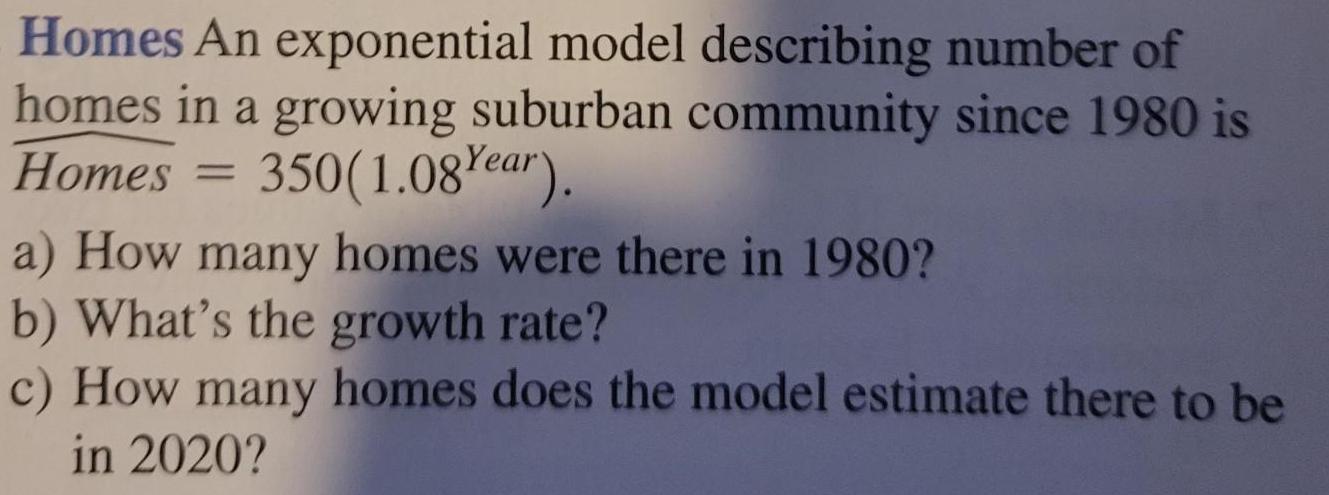
Math
Basic MathHomes An exponential model describing number of homes in a growing suburban community since 1980 is Homes = 350(1.08 Year).
a) How many homes were there in 1980?
b) What's the growth rate?
c) How many homes does the model estimate there to be in 2020?

Math
Sets and RelationsChoose which sets of ordered pairs satisfies an exponential function. Select two that apply
A {(-1,1), (0,0), (1,1), (2,4)}
B {(-1,-9), (1,9), (3,27), (5,45)}
C (-2,4), (-1,2), (0,1), (1,0.5)}
D (-1,1.5), (0,3), (1,6), (2,12)}
Math
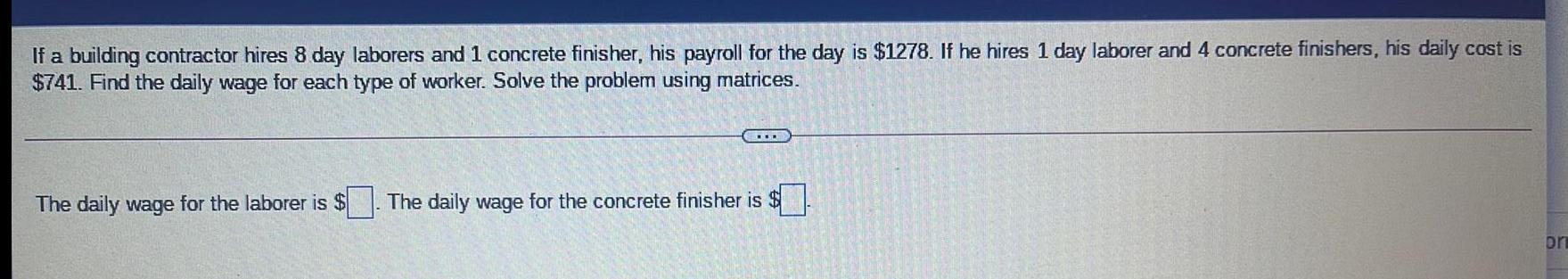
Basic MathIf a building contractor hires 8 day laborers and 1 concrete finisher, his payroll for the day is $1278. If he hires 1 day laborer and 4 concrete finishers, his daily cost is
$741. Find the daily wage for each type of worker. Solve the problem using matrices.
The daily wage for the laborer is $
The daily wage for the concrete finisher is $
Math
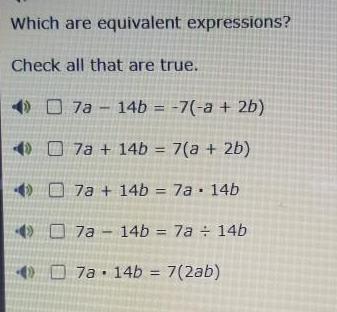
Basic MathWhich are equivalent expressions?
Check all that are true.
7a - 14b = -7(-a + 2b)
7a+14b = 7(a + 2b)
47a+ 14b = 7a 14b
7a - 14b = 7a /14b
7a- 14b = 7(2ab)

Math
Basic MathWhich of the following examples could correctly show the mean density of lunar soil?
3.24 cubic centimeter
3.24 grams per square centimeter
3.24 cubic centimeter per gram
3.24 grams per cubic centimeter

Math
Basic MathFill in each blank with the appropriate equation. (Select all that apply.)
The two notations for the inverse of y= sin x are__and___
y = arcsin x
y = sin-¹ x
y =csc x
y=1/sin x
y = -sin x

Math
Basic MathSuppose a product's revenue function is given by R(q) = 7q² + 300q
Find an expression for the marginal revenue function, simplify it, and record your result in the box below. Be sure to use the proper variable in your answer. (Use the preview button to check your syntax before submitting your answer.)
Math
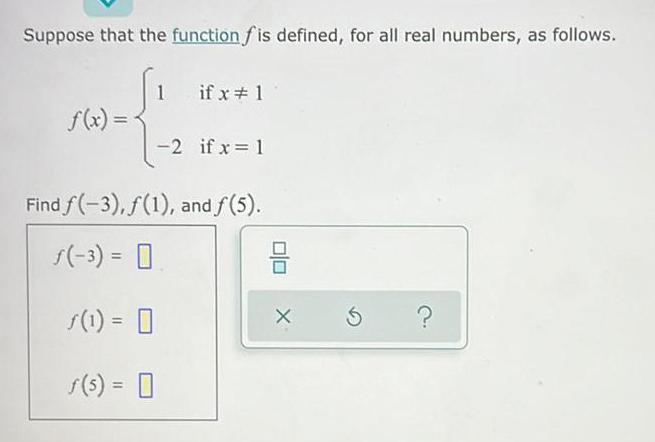
FunctionsSuppose that the function fis defined, for all real numbers, as follows.
f(x) = 1 if x ≠1
-2 if x = 1
Find f(-3), f(1), and ƒ (5).
ƒ(-3) =
f(1) =
ƒ(5) =

Math
ProbabilityIn Australia in 1995, of the 2907 indigenous people in prison 17 of them died. In that same year, of the 14501 non-indigenous people in prison 42 of them died ("Aboriginal deaths in," 2013).
a. Find the probability that an indigenous person in prison dies.
Enter your answer as a fraction.
Enter your answer rounded to 3 decimal places
b. Find the probability that a non-indigenous person in prison dies.
Enter your answer as a fraction.
Enter your answer rounded to 3 decimal places

Math
Basic MathThe length of time Joe takes to get to work varies inversely as the speed he travels. If he jogs at 6.6 feet per second it takes him 75 minutes to get to
work.
Let T = Time and V = speed:
The general equation for this variation problem is:
1) v=kT
2) T = kV
3) TV = k
4) k =v/T
(5) T=k/V

Math
Basic MathMalaya is making a collage of her birthday party photos and she wants to add an 8 x 10 inch. photo. Since there is not enough space left on the collage, she must trim the photo. If the scale factor from the original to the trimmed photo is 5:4, the new dimensions of the photo are
7x9 in
6.2 x 7.6
6.4 x 8 in
5.4 x 6.8


Math
Basic MathThe state fair is a popular field trip destination. This year the senior class at High School A and the senior class at High School B both planned trips there. The senior class at High School A rented and filled 5 vans and 1 bus with 74 students. High School B rented and filled 13 vans and 7 buses with 386 students. Each van and each bus carried the same number of students. How many students can a van carry? How many students can a bus carry?
Math
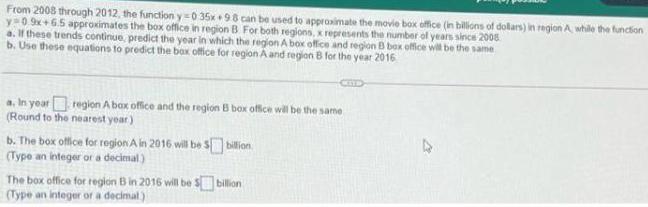
FunctionsFrom 2008 through 2012, the function y-035x +98 can be used to approximate the movie box office (in billions of dollars) in region A while the function
y =0.9x+6.5 approximates the box office in region B. For both regions, x represents the number of years since 2008
a. If these trends continue, predict the year in which the region A box office and region B box office will be the same
b. Use these equations to predict the box office for region A and region B for the year 2016
a. In year region A box office and the region B box office will be the same
(Round to the nearest year)
b. The box office for region A in 2016 will be $ billion
(Type an integer or a decimal)
The box office for region B in 2016 will be 5 billion
(Type an integer or a decimal)
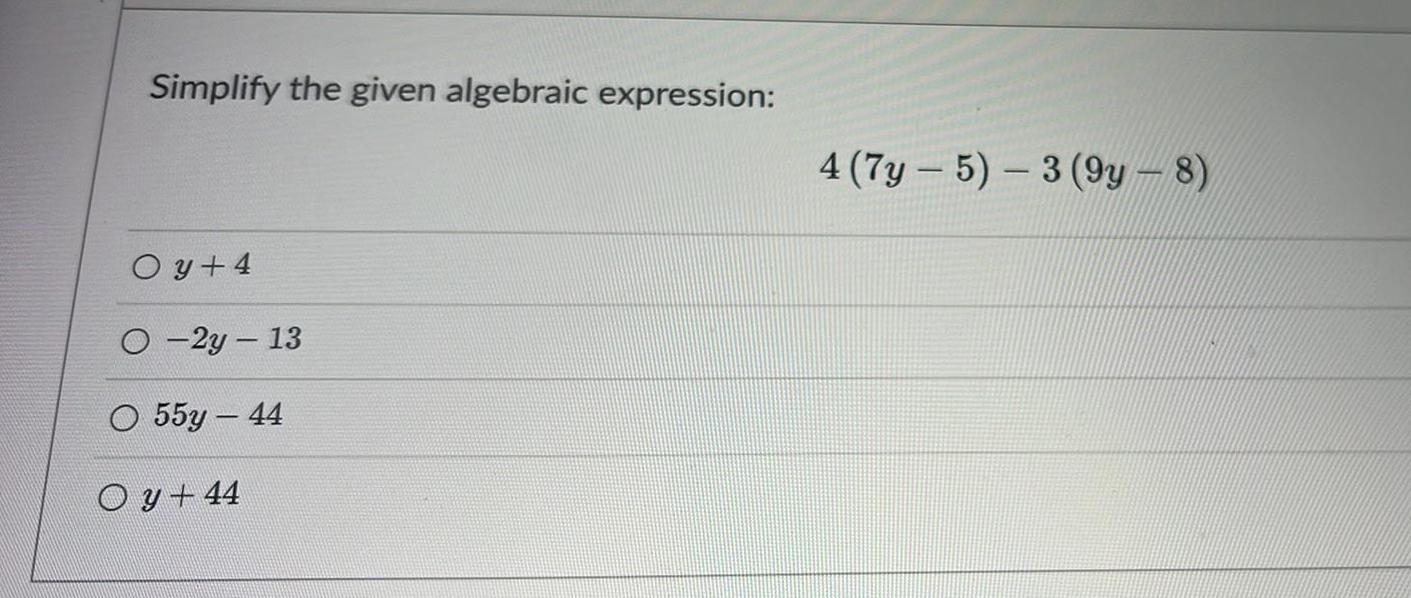
Math
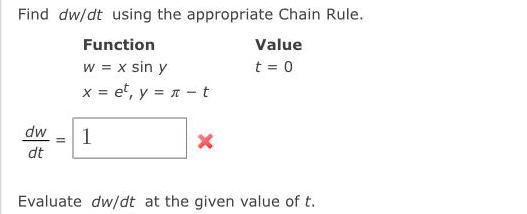
Application of derivativesFind dw/dt using the appropriate Chain Rule.
Function
w = x sin y
x = e^t, y = π- t
value t=0
dw/dt=
Evaluate dw/dt at the given value of t.

Math
Basic MathFind a formula for the exponential function V=h(t) that gives the value of an item initially worth $5000 that loses half its value every 8 years.
h(t) =
dollars. help (formulas) (Do not enter any commas in your formula.)
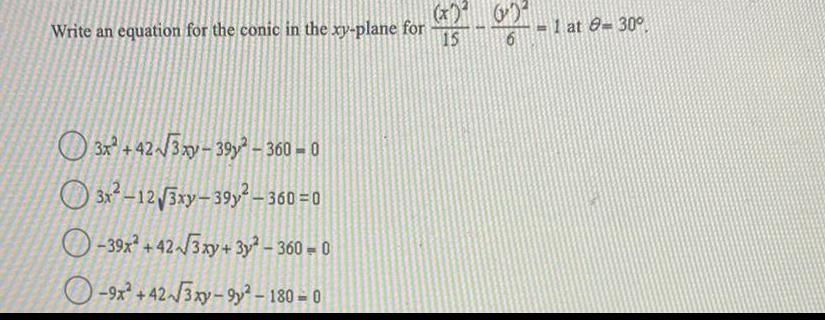
Math
Basic MathWrite an equation for the conic in the xy-plane for (x')2 /15 - (y')^2/6 =1 at θ=30
3x+42√3xy-39y²-360 = 0
3x²-12√3xy-39y²-360=0
-39x² +42-√3xy+3y² - 360 = 0
-9x² +42-√3xy-9y2² – 180 = 0

Math
Basic MathA radioactive element decays at a rate of 5% annually. There are 40 grams of the substance present.
How much of the substance remains after 30 years (to the nearest tenth)?
When will the amount of the substance drop to below 20 grams (to the nearest year)?

Math
Basic MathHow much time will be needed for $25,000 to grow to $26,015.10 if deposited at,,4% compounded quarterly? For calculating use the formula A = P(1+t/k)^kt
The amount $25,000 will grow to $26,015.10 in __ year(s).
(Do not round until the final answer. Then round to the nearest tenth as needed.)

Math
Binomial theoremUse the Binomial Theorem and Pascal's Triangle to find the expanded form of the binomial expression.
(2x+3y)
A. (2x) +6(2x)³ (3y)+15(2x)*(3y)² +20(2x)³ (3y)³ +15(2x)² (3y)* +6(2x)(3y)³ +(3y)
B. (2x)+7(2x)³ (3y)+21(2x)*(3y)² +35(2x)³ (3y)² +21(2x)²(3y)* +7(2x)(3y)³ +(3y)
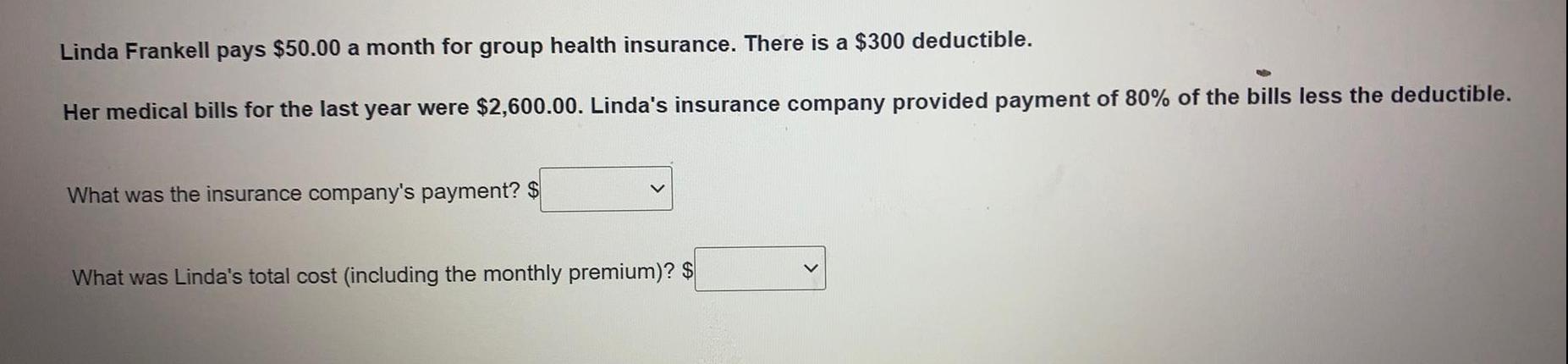
Math
Basic MathLinda Frankell pays $50.00 a month for group health insurance. There is a $300 deductible.
Her medical bills for the last year were $2,600.00. Linda's insurance company provided payment of 80% of the bills less the deductible.
What was the insurance company's payment? $
What was Linda's total cost (including the monthly premium)? $

Math
Straight linesGraph the image of A(1,-3) after the described glide reflection. Then determine the coordinate of the image (A').
Translation: (x,y) → (x,y+2)
Reflection: in the y-axis
![Which formula would be used to calculate the middle rectangle approximation of 0∫1 4xdx using five subintervals?
M5= (0.4)[4(0.1) + 4(0.3) + 4(0.5) + 4(0.7) + 4(0.9)]
M5 = (0.2) [4(0.1) + 4(0.3) + 4(0.5) + 4(0.7) + 4(0.9)]
M5 (0.2) [4(0.2) + 4(0.4) + 4(0.6) + 4(0.8) + 4(1.0)]
M5= (0.2)[4(0.0) + 4(0.2) + 4(0.4) + 4(0.6) + 4(0.8)]](https://media.kunduz.com/media/sug-question/raw/71067631-1659115226.4116735.jpeg?w=256)
Math
Basic MathWhich formula would be used to calculate the middle rectangle approximation of 0∫1 4xdx using five subintervals?
M5= (0.4)[4(0.1) + 4(0.3) + 4(0.5) + 4(0.7) + 4(0.9)]
M5 = (0.2) [4(0.1) + 4(0.3) + 4(0.5) + 4(0.7) + 4(0.9)]
M5 (0.2) [4(0.2) + 4(0.4) + 4(0.6) + 4(0.8) + 4(1.0)]
M5= (0.2)[4(0.0) + 4(0.2) + 4(0.4) + 4(0.6) + 4(0.8)]

Math
Basic Math(a) This compound statement is made up of three simple statements. Identify them and assign a letter to each.
Choose the correct choice of statements and assignment of letters.
p: You take the product weekly.
q: You cut your calorie intake by 10%.
r: You will lose at least 10 pounds in the next 4 months.
p: A new weight loss supplement claims.
q: You take the product daily and cut your calorie intake by 10%.
r: You will lose at least 10 pounds in the next 4 months.
p: You take the product daily.
q: You cut your calorie intake by 10%.
r: You will lose at least 10 pounds in the next 4 months.

Math
Straight linesCreate a line that is parallel to AB and passes through point C. You can use the tools available in GeoGebra to create parallel lines for this construction. Take a screenshot of your results, and paste it below.

Math
Basic MathQuadrilateral ABCD has the following angle measures:
m∠A = 72°
m∠B = 103°
m∠C= 10x + 9
m∠D = 7x + 8
Find x first, then find the measure of angle D. Choose the answer that represents the measure of angle D.
10
78
9.7
109

Math
Basic MathIn a certain lottery, 3 different numbers between 1 and 8 are drawn. These are the winning numbers. How many different selections are possible? Assume that the order in which the numbers are drawn is not important.

Math
Basic MathIn country A, about six times as many cars are manufactured per day than in country B. If the total number of these cars manufactured per day is 46,263, find the number manufactured in country B and the number manufactured in country A.
In country A,___cars are manufactured per day.
In country B,__cars are manufactured per day.

Math
Basic Math[5 pts) In a recent survey in 1050 UCLA students 175 out of the 650 female students said they preferred to drive a SUV to driving a compact car. Identify the population, sample, and variable
Population:
Sample:
Variable
is it categorical or numerical?
What percentage of female students in the study said they prefer to drive a SUV to driving a compact car? (Round answer to one decimal place)

Math
Definite IntegralsFor the regions bounded by y = 6cosx and y= 1/3x²-7
Part A: Find the limits of integration. (10 points)
Part B: Write an expression representing the total area. (10 points)
Part C: Solve for the total area. (10 points)
Math
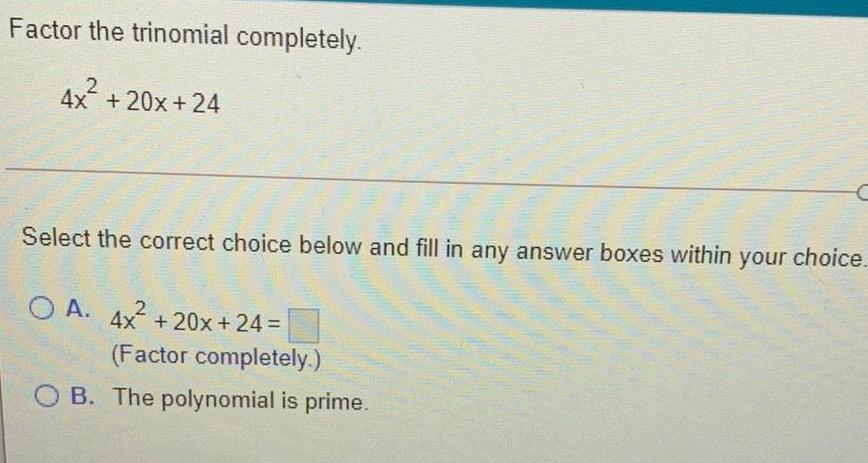
Basic MathFactor the trinomial completely.
4x² +20x+24
Select the correct choice below and fill in any answer boxes within your choice.
A. 4x² +20x + 24 =
(Factor completely.)
B. The polynomial is prime.

Math
Basic MathWater is leaking from a tank at a rate of R(t) gallons per hour, where t is time in hours. What is the meaning of R(t)dt = 2,430 in terms of the context? (1 point),
The tank is leaking at a rate of 2,430 gallons per hour from hours 2 to 4.
The tank leaked 2,430 gallons of water over the first 4 hours.
The tank leaked 2,430 gallons of water from t = 2 to t = 4.
The tank has 2,430 gallons of water left in the tank after the first 4 hours.
Math
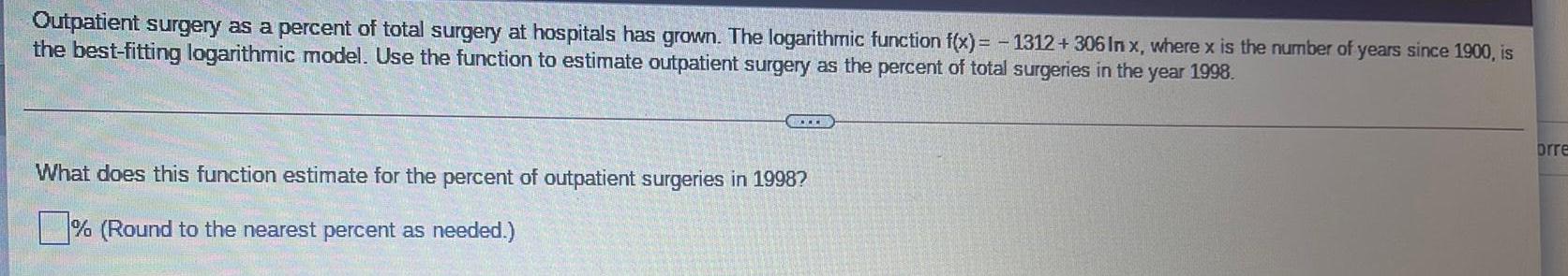
Basic MathOutpatient surgery as a percent of total surgery at hospitals has grown. The logarithmic function f(x) = -1312+ 306 In x, where x is the number of years since 1900, is
the best-fitting logarithmic model. Use the function to estimate outpatient surgery as the percent of total surgeries in the year 1998.
What does this function estimate for the percent of outpatient surgeries in 1998?
% (Round to the nearest percent as needed.)

Math
Basic MathA particular country's exports of goods are increasing exponentially. The value of the exports, t years after 2006, can be approximated by V(t) = 1.7 e0
corresponds to 2006 and V is in billions of dollars.
a) Estimate the value of the country's exports in 2006 and 2010.
b) What is the doubling time for the value of the country's exports?
a) The value of the country's exports in 2006 is $ 1.7 billion.
(Simplify your answer. Round to the nearest tenth as needed. Do not include the $ symbol in your answer.)
The value of the country's exports in 2010 is $ billion.
(Simplify your answer. Round to the nearest tenth as needed. Do not include the $ symbol in your answer.)

Math
Basic MathThe amount of medication, in milligrams, in a patient's bloodstream after t hours, can be represented by the following function:
M(t)=75/1+ e^x+0.5
What is the rate of change for the amount of medication in the patient's bloodstream after 2 hours?
5.6894 mg/hr
-0.4316 mg/hr
-5.2578 mg/hr
-5.6894 mg/hr

Math
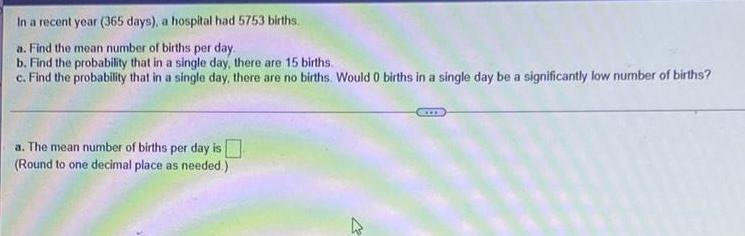
Basic MathIn a recent year (365 days), a hospital had 5753 births.
a. Find the mean number of births per day.
b. Find the probability that in a single day, there are 15 births
c. Find the probability that in a single day, there are no births. Would 0 births in a single day be a significantly low number of births?
a. The mean number of births per day is____
(Round to one decimal place as needed.)

Math
Basic MathWhich lists the possible types of roots for f(x) = 3x^4 + 7x³ + 2x² + x + 9?
One rational root and three irrational roots
Two irrational roots, one complex root, one real root
Three complex roots and one rational root
Two rational roots and two irrational roots
Math
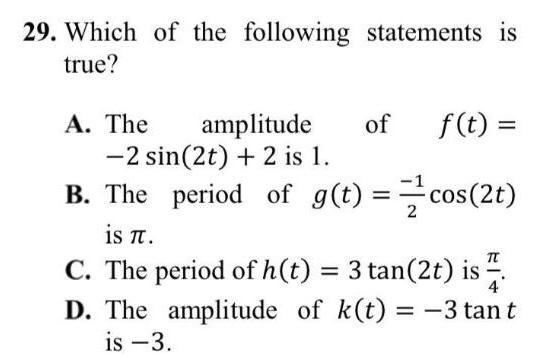
Basic MathWhich of the following statements is true?
A. The amplitude of f(t)= -2 sin(2t) + 2 is 1.
B. The period of g(t) =-1/2 cos(2t) is π
C. The period of h(t) = 3 tan(2t) is π/4
D. The amplitude of k(t) = -3 tan t is -3.

Math
Basic MathFor the planer curve represented by the parametric equations x = 3t and y = t^2 + 5, what is the rectangular equation of the curve?
9x² - y + 5 = 0
3y²-x+15=0
x² + y²-25= 0
x² - 9y + 45 = 0