Math Questions
The best high school and college tutors are just a click away, 24×7! Pick a subject, ask a question, and get a detailed, handwritten solution personalized for you in minutes. We cover Math, Physics, Chemistry & Biology.

Math
Basic MathKelly bought 4 shirts and 3 skirts for her doll and paid $11.40 total. After she did a doll fashion show for her best friend, Caitlyn wanted to know how much one of the skirts cost. Kelly remembered that each skirt cost $0.30 more than each shirt. How much did one skirt cost?
A. $1.50
B. $1.60
C. $1.65
D. $1.75
E. $1.80

Math
Basic MathThe square of a number is equal to 10 less than 7 times that number. What are the two possible solutions?
Which of the following equations is used in the process of solving this problem?
x² - 7x + 10 = 0
x² - 7x+70 = 0
x² + 7 x-10 = 0

Math
Sets and RelationsIf set A contains seven distinct numbers and set B contains three distinct letters, how many elements are in (A u B)?

Math
Basic MathSolve for the variable in each of the following equations.
Write your answers as whole numbers, proper fractions, Improper fractions, or mixed numbers in simplest form.
x + 9 = 14 x=
17z = 7 z=
c+ 17 = 20 c=
16d = 6 d=
b-9 = 14 b=
19r = 9 r=
n - 14 = 16 n=
8y = 10 y=

Math
StatisticsIn a large city, a transportation engineer wants to estimate the proportion of residents who use the bus system regularly. Out of a random sample of 2000 residents, 1220 use the bus regularly. Construct and interpret the 99% confidence interval.
(P-hat) = 1220 / 2000 = .61
(Q-hat) 1-.61 = .39
Standard Error = .010906
with a Z score of 2.5758 for the 99% confidence interval.
.61 +2.5758 (.010906) = .6380
.612.5758 (.010906) = .5819
(.5819, .6380)
So with a confidence interval of 99%, the true proportion of residents who use the bus system regularly is between 58.19% and 63.8%

Math
Basic MathFind the probability of winning the Jackpot in a lottary if you buy just one ticket. In this lottary, a typical ticket is Formed by selecting 5 different numbers from the range {1,2,...,38}

Math
Solution of trianglesTriangle ABC is transformed to create AMNand APQR as described.
Triangle MNrepresents the translation 2 units left and 2 units down of AABC.
Triangle PQR represents the dilation of AABC by a scale factor of 2.
Which of these is correct?
The transformation of triangle ABC to triangle MNO preserves the angle measures but not the side lengths of the triangle.
The transformation of triangle ABC to triangle MNO preserves both the side lengths and angle measures of the triangle.
The transformation of triangle ABC to triangle PQR preserves both the side lengths and angle measures of the triangle.
The transformation of triangle ABC to triangle PQR preserves the side lengths but not the angle measures of the triangle.

Math
Basic MathYou had the following readings on your water meter:
December 1: 7,500 cubic feet
January 1: 7,585 cubic feet
February 1: 7,671 cubic feet
March 1: 7,749 cubic feet
What is the number of cubic feet consumed in January?

Math
Basic MathCash equivalents by definition
a. are a comparison of cash and liabilities.
b. are expected to be converted to cash within three months.
c. will be converted to cash within one year.
d. are long-term investments.
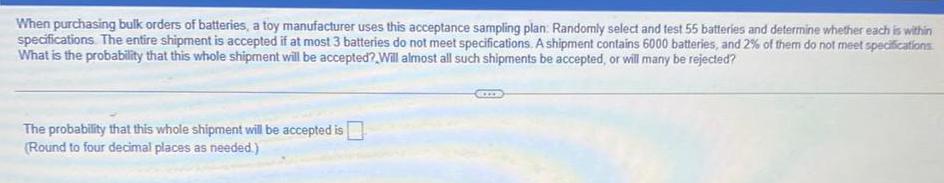
Math
ProbabilityWhen purchasing bulk orders of batteries, a toy manufacturer uses this acceptance sampling plan: Randomly select and test 55 batteries and determine whether each is within
specifications. The entire shipment is accepted if at most 3 batteries do not meet specifications. A shipment contains 6000 batteries, and 2% of them do not meet specifications
What is the probability that this whole shipment will be accepted? Will almost all such shipments be accepted, or will many be rejected?
The probability that this whole shipment will be accepted is
(Round to four decimal places as needed.)
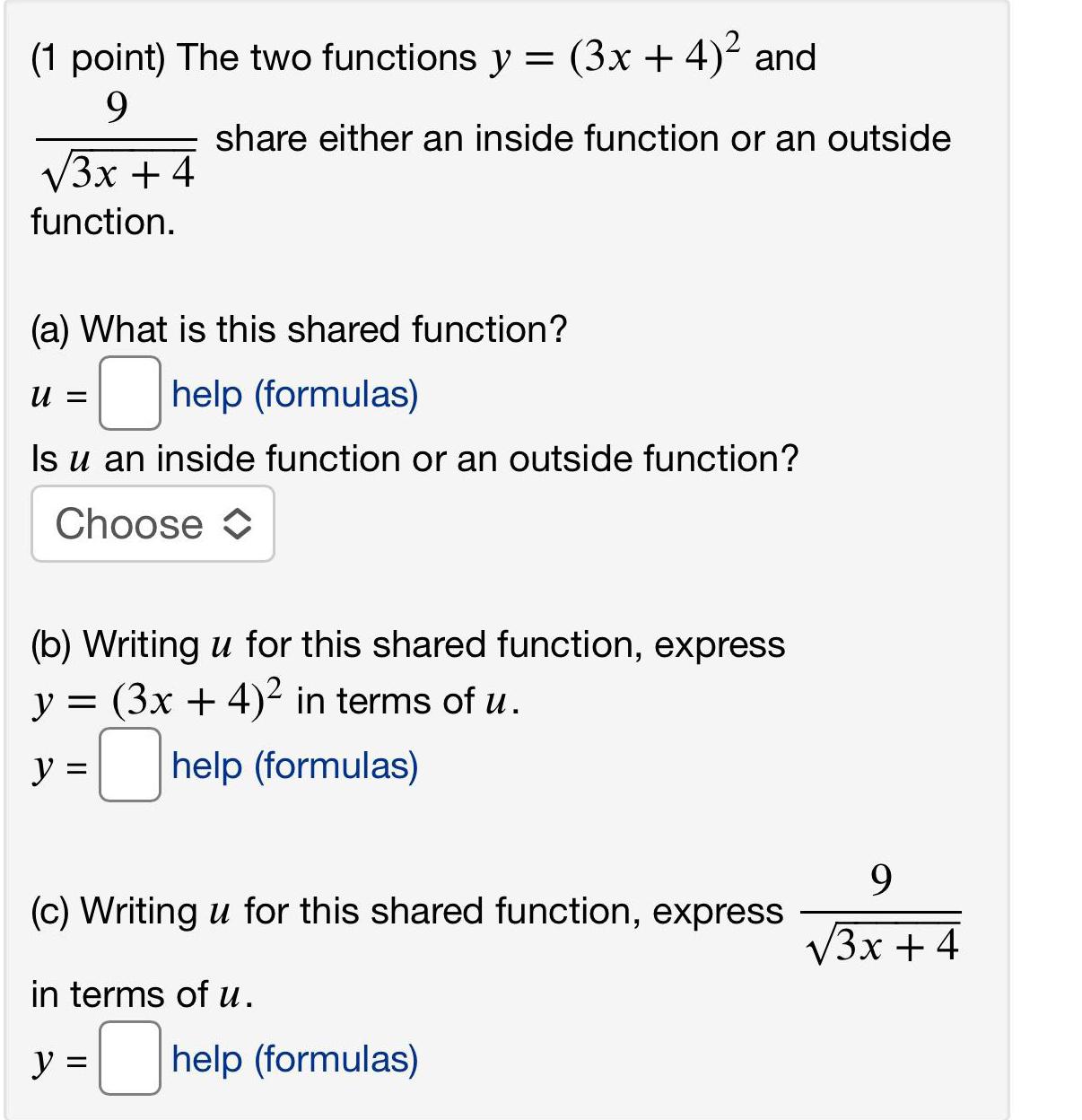
Math
FunctionsThe two functions y = (3x + 4)² and 9/√3x + 4 share either an inside function or an outside function.
(a) What is this shared function?
u = help (formulas)
Is u an inside function or an outside function?
(b) Writing u for this shared function, express y = (3x + 4)² in terms of u.
y = help (formulas)
(c) Writing u for this shared function, express 9/√3x + 4 in terms of u.
y = help (formulas)

Math
TrigonometryWhich expression is equivalent to tan(x) + cot(x)?
cos x/sec x
cos(x) sin(x)
1/sin(x) cos(x)
2 tan(x)
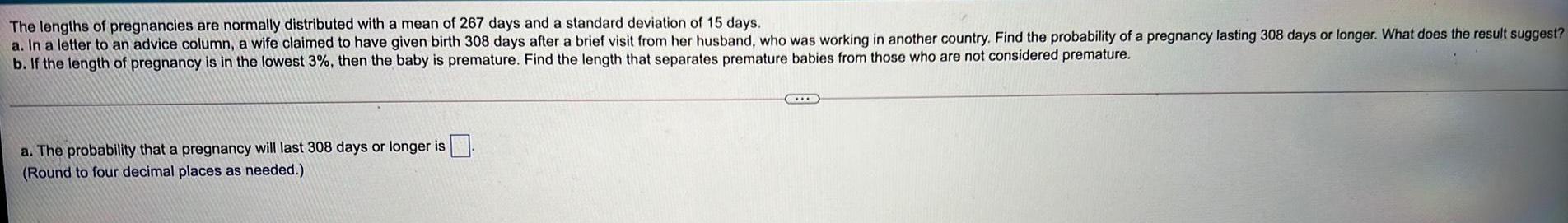
Math
ProbabilityThe lengths of pregnancies are normally distributed with a mean of 267 days and a standard deviation of 15 days.
a. In a letter to an advice column, a wife claimed to have given birth 308 days after a brief visit from her husband, who was working in another country. Find the probability of a pregnancy lasting 308 days or longer. What does the result suggest?
b. If the length of pregnancy is in the lowest 3%, then the baby is premature. Find the length that separates premature babies from those who are not considered premature.
a. The probability that a pregnancy will last 308 days or longer is
(Round to four decimal places as needed.)

Math
Basic MathSelect the correct answer.
What is the variable being tested in an experiment?
A. control variable
B. response variable
C. placebo
D. explanatory variable

Math
FunctionsFor the piecewise function below, it is known that the function is always continuous,
f(x) = 3x - 1, x < -2
f(x) = px² + qx, -2 ≤ x < 4
f(x)=√x, x ≥ 4
A. Find the values of p and q
B. Explain if there is a value such that f(x) = 5 in the interval of -2 ≤ x < 4.

Math
ProbabilityIf a candy jar has 21 green jelly beans and 12 black jelly beans, and 19 red jelly beans, what is the probability of eating a green and then a red jelly bean? Express the probability to the nearest hundredth.

Math
Coordinate systemGive the coordinates of the point obtained from each reflection.
(a) Reflect the point (0, 4) across the y-axis:
(b) Reflect the point (0, 4) across the X-axis:

Math
StatisticsAssume that the readings at freezing on a bundle of thermometers are normally distributed with a mean of 0°C and a standard deviation of 1.00°C. A single thermometer is randomly selected and tested. Find the probability of obtaining a reading less than -1.775°C.
P(Z < -1.775) =
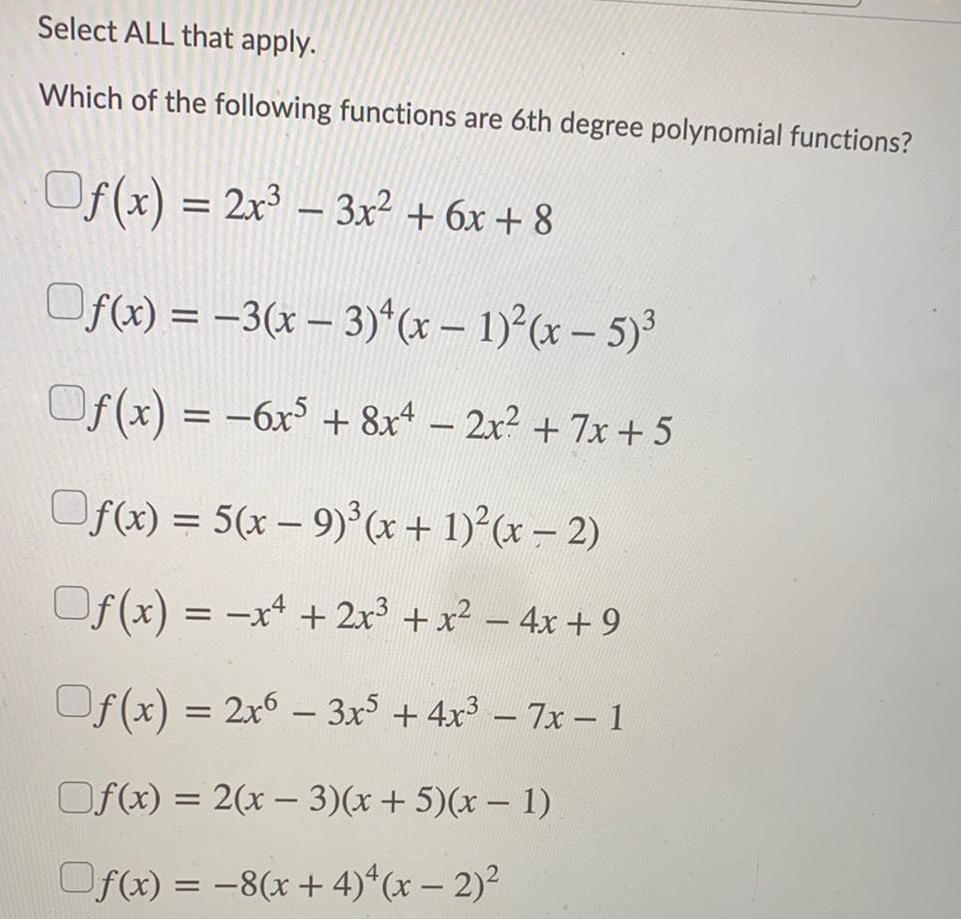
Math
FunctionsSelect ALL that apply.
Which of the following functions are 6th degree polynomial functions?
f(x) = 2x³ − 3x² + 6x +8
f(x) = -3(x-3)(x - 1)²(x - 5)³
ƒ (x) = −6x^5 + 8x^4 − 2x² + 7x + 5
f(x) = 5(x - 9)³ (x + 1)²(x − 2)
f(x) = -x² + 2x³ + x² - 4x +9
f(x) = 2x^6 - 3x³ + 4x³ - 7x - 1
f(x) = 2(x − 3)(x + 5)(x - 1)
f(x) = -8(x+4)^4 (x - 2)²
Math
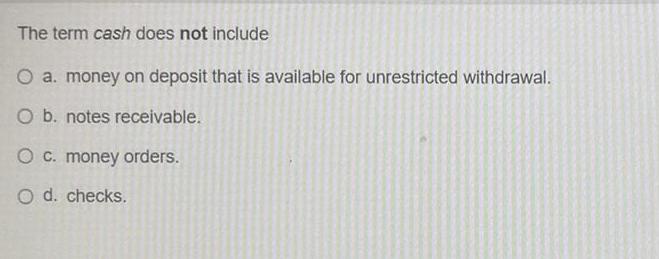
Mathematical ReasoningThe term cash does not include
a. money on deposit that is available for unrestricted withdrawal.
b. notes receivable.
c. money orders.
d. checks.
Math
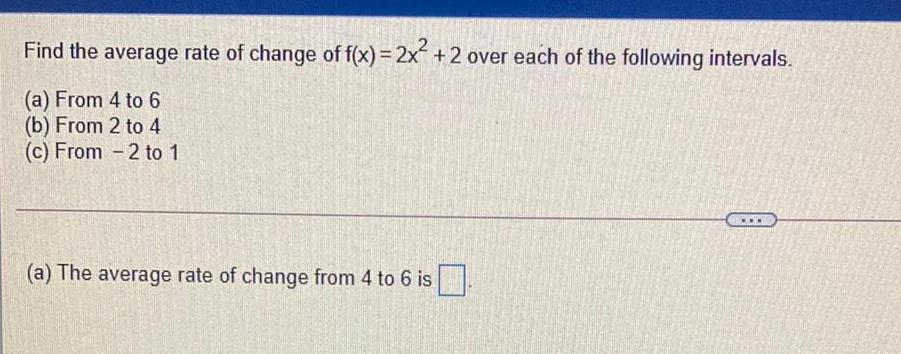
Basic MathFind the average rate of change of f(x)=2x² +2 over each of the following intervals.
(a) From 4 to 6
(b) From 2 to 4
(c) From 2 to 1
(a) The average rate of change from 4 to 6 is

Math
Basic MathThe thermal plot of a gaming system during play time is defined by 10ln(15t³) - 2T+100=T + 10t for 0<t≤ 5, where t represents time in hours and T represents temperature in °C. For which condition does the system reach a possible maximum or minimum temperature? (1 point)
30-10t=0
450t² - 10 = 0
6-90t³ = 0
15t²-10=0

Math
Basic MathThe heat loss of a glass window varies jointly as the window's area and the difference between the outside and inside temperatures. A window 2 feet by 5 feet loses 3000 Btu per hour when the temperature outside is 30° colder than the temperature inside. Find the heat loss through a glass window that is 8 feet by 15 feet when the temperature outside is 15° colder than the temperature inside.
The heat loss is ____Btu per hour.
(Type an integer or a decimal.)

Math
Basic MathStrontium-90 is a radioactive material that decays according to the function
A(t) = A e ^-0.0244t, where Ao is the initial amount present and A is the amount present at time t (in years). Assume that a scientist has a sample of 400 grams of strontium-90.
(a) What is the decay rate of strontium-90?
(b) How much strontium-90 is left after 10 years?
(c) When will only 100 grams of strontium-90 be left?
(d) What is the half-life of strontium-90?
(a) The decay rate of strontium-90 is
(Type an integer or a decimal. Include the negative sign for the decay rate.)
(b) Approximately ___grams of strontium-90 left after 10 years.
(Do not round until the final answer. Then round to the nearest whole number as needed.)
(c) Only 100 grams of strontium-90 will be left in about ____years.
(Do not round until the final answer. Then round to the nearest tenth as needed.)

Math
Basic MathFind f(g(x)) and g(f(x)) and determine whether the pair of functions f and g are inverses of each other.
f(x)=7x-4 and g(x)= x+7/4
a. f(g(x)) =
(Simplify your answer. Use integers or fractions for any numbers in the expression.)

Math
FunctionsFind the horizontal asymptote, if any, of the graph of the rational function.
g(x) =5x2-9x-8/3x² - 4x + 2
y = 0
y = 5/3
y=9/4
no horizontal asymptote

Math
TrigonometrySolve the equation on the interval [0, 2TT).
cos x - 2 cos x sin x = 0
π/6,5π/6
π/6,π/2,5π/6,3π/2
π/6,5π/6,2π
0,π/6,5π/6,3π/2

Math
Basic MathUsing a table of values, what is an approximate solution of the given equation to the nearest quarter of a unit?
|x + 3 |= 3x + 1
A.x≈1.25
B.x≈-3.75
C.x ≈ -1.75
D.x≈1.50
Math
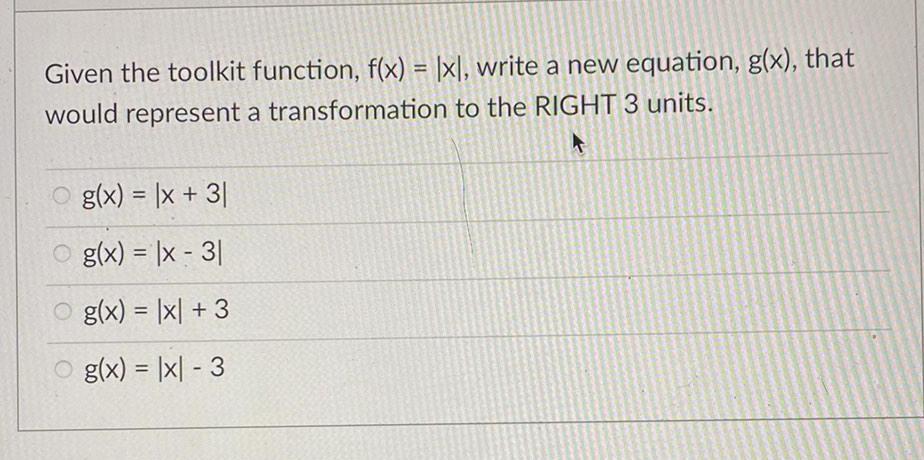
FunctionsGiven the toolkit function, f(x) = |x|, write a new equation, g(x), that
would represent a transformation to the RIGHT 3 units.
g(x) = |x + 3|
g(x) = |x - 3|
g(x) = |x| +3
g(x) = |x - 3

Math
Basic MathWhich methods can be used to expand (x+3)³?
multiply 2 by (2+3), then multiply the product by 3
apply patterns from the third row of Pascal's Triangle
find the third power of both 2 and 3, and then add the terms
in vertical form, multiply (2+3) three times, then add like terms
use horizontal format to find (x+3)², then multiply the product by (x+3)

Math
ProbabilityBased on historical data, your manager believes that 31% of the company's orders come from first-time customers. A random sample of 100 orders will be used to estimate the proportion of first-time-customers.
What is the probability that the sample proportion is less than 0.27

Math
Basic MathThe weight M of an object on the moon varies directly as its weight E on earth. A person who weighs 157.18 lb on earth weighs 26.72 lb on the moon. How much would a 228.24-lb person weigh on the moon?

Math
Basic MathLet a curve be defined as siny = x + y. Determine which of the following statement(s) is/are true. (1 point)
I. The curve has a vertical tangent when cosy - 1 = 0.
II. The curve has no horizontal tangents because cosy - 1 * 0.
III. The curve has no horizontal tangents because dy/dx≠0.
I only
III only
I and III
I and II

Math
Basic MathA rope from the top of a mast on a sailboat is attached to a point 19 feet from the mast. If the rope is 28 feet long, how tall is the mast? Round to the nearest tenth of a foot.

Math
FunctionsA pharmaceutical corporation has two plants that produce the same over-the-counter medicine. If x₁ and x₂ are the numbers of units produced at plant 1 and plant 2, respectively, then the total revenue for the product is given by
R = 160x₁ + 160x₂ - 2x₁² - 4x₁x₂ - 2x₂². When x₁ = 11 and x₂ = 7, find the marginal revenue for plant 1, ƏR/0x₁, and the marginal revenue for plant 2, 3R/3x2.
(a) Find the marginal revenue for plant 1, 3R/3x₁.
(b) Find the marginal revenue for plant 2, ƏR/0x₂.
Math
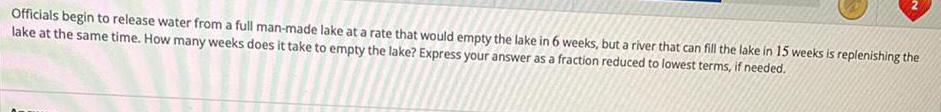
Basic MathOfficials begin to release water from a full man-made lake at a rate that would empty the lake in 6 weeks, but a river that can fill the lake in 15 weeks is replenishing the lake at the same time. How many weeks does it take to empty the lake? Express your answer as a fraction reduced to lowest terms, if needed.

Math
Basic MathDuring the past six months, 73.2 percent of U.S. households purchased sugar. Assume that these expenditures are approximately normally distributed with a mean of $8.22 and a standard deviation of $1.10. Find the probability that a household spent more than $10.00 on sugar.
Multiple Choice
.7320
.9474
.0528
.2680

Math
StatisticsIn a random sample of 12 dental assistants, the mean annual earnings was $31,721. The population is normally distributed with a standard deviation of $5260. Construct a 95% confidence interval for the population mean annual earnings for dental assistants.
Select the correct answer below:
With 95% confidence, you can say that the population mean annual earnings is between $29,942 and $32,991.
With 95% confidence, you can say that the population mean annual earnings is between $28,745 and $34,697.
With 95% confidence, you can say that the population mean annual earnings is between $29,734 and $35,007.
With 95% confidence, you can say that the population mean annual earnings is between $28,877 and $34,598.

Math
Basic MathThe Pool Fun Company has learned that, by pricing a newly released Fun Noodle at $3, sales will reach 9000 Fun Noodles per day during the summer. Raising the price to $4 will cause the sales to fall to 5000 Fun Noodles per day.
a. Assume that the relationship between sales price, x, and number of Fun Noodles sold, y, is linear. Write an equation in slope-intercept form describing this relationship. Use ordered pairs of the
form (sales price, number sold).
The equation is y=-4000x+21000. (Type your answer in slope-intercept form.)
b. Predict the daily sales of Fun Noodles if the price is $3.50.
sales

Math
Basic MathCompany XYZ has 34 employees in the Finance Department, 45 technicians, and 32 in the Engineering Department. The HR Department received 20 complaints from the whole company. There were 5 complaints from the technicians and 3 from the Finance Department. There were 7 complaints from the Engineering Department.
What percent of complaints came from either the technicians or the Finance Department?

Math
Differential equationsIn drawing the slope field for the differential equation dy/dx= x+3y-4, I would draw a short line segment for different points on the x-y plane.
What slope should I draw the line segment at the point (0,-2)?
Your Answer:

Math
Application of derivativesFor the function, find the points on the graph at which the tangent line is horizontal. If none exist, state that fact.
f(x) = 5x² - 2x+5
Select the correct choice below and, if necessary, fill in the answer box within your choice.
A. The point(s) at which the tangent line is horizontal is (are)
(Simplify your answer. Type an ordered pair. Use a comma to separate answers as needed.)
B. There are no points on the graph where the tangent line is horizontal.
C. The tangent line is horizontal at all points of the graph.

Math
Mathematical InductionA 75 L gas tank has a leak. After t hours, the remaining volume, V, in litres is V(t) = 75 (1 - t/24) ²,0≤ t ≤ 24. Use the product rule to determine how quickly the gas is leaking from the tank when the tank is 60% full of gas.
Math
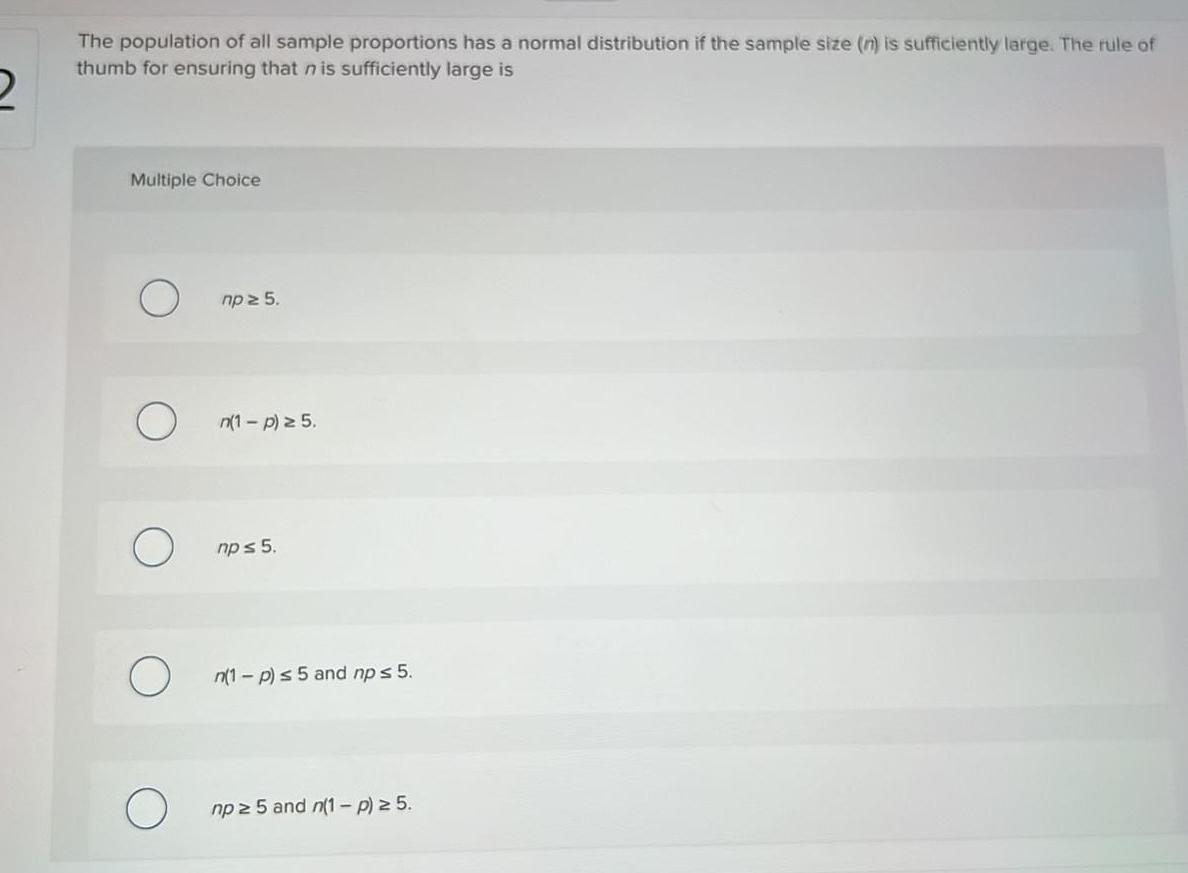
Straight linesThe population of all sample proportions has a normal distribution if the sample size (n) is sufficiently large. The rule of thumb for ensuring that n is sufficiently large is
Multiple Choice
O пp≥5.
O n(1-P)≥5.
O np≤5.
O n(1-p)≤5 and np≤5.
O np≥5 and n(1-P)≥5.
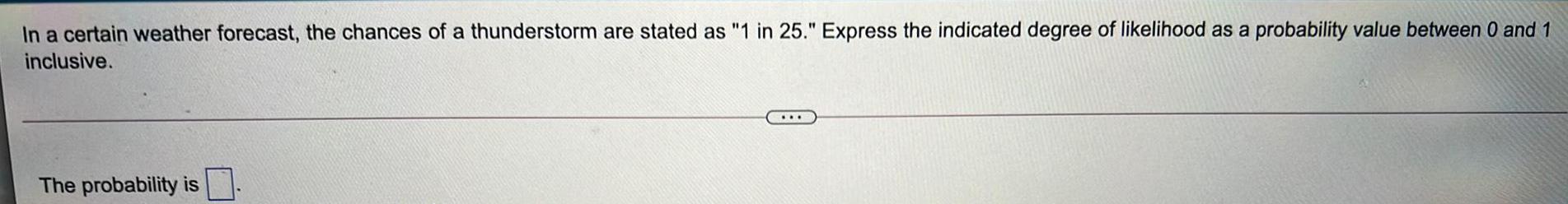
Math
ProbabilityIn a certain weather forecast, the chances of a thunderstorm are stated as "1 in 25." Express the indicated degree of likelihood as a probability value between 0 and 1
inclusive.
The probability is____
![A manufacturer of personal computers tests competing brands and finds that the amount of energy they require is normally distributed with a mean of 285 kwh and a standard deviation of 9.1 kwh. If the lowest 25 percent and the highest 30 percent are not included in a second round of tests, what are the upper and lower limits for the energy amounts of the remaining computers?
Multiple Choice
[269.76, 300.24]
[278.86, 289.78]
[280.22, 289.78]
[280.22, 300.24]](https://media.kunduz.com/media/sug-question/raw/68680640-1659101641.4983642.jpeg?w=256)
Math
Basic MathA manufacturer of personal computers tests competing brands and finds that the amount of energy they require is normally distributed with a mean of 285 kwh and a standard deviation of 9.1 kwh. If the lowest 25 percent and the highest 30 percent are not included in a second round of tests, what are the upper and lower limits for the energy amounts of the remaining computers?
Multiple Choice
[269.76, 300.24]
[278.86, 289.78]
[280.22, 289.78]
[280.22, 300.24]
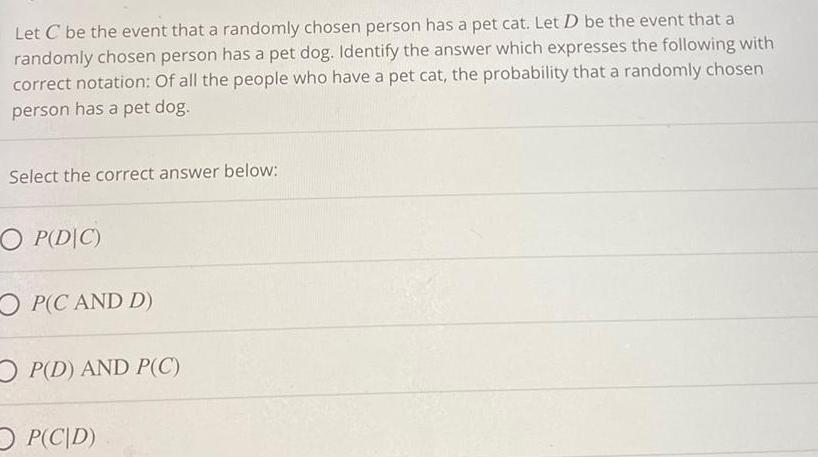
Math
ProbabilityLet C be the event that a randomly chosen person has a pet cat. Let D be the event that a randomly chosen person has a pet dog. Identify the answer which expresses the following with correct notation: Of all the people who have a pet cat, the probability that a randomly chosen person has a pet dog.
Select the correct answer below:
O P(DIC)
O P(C AND D)
O P(D) AND P(C)
O P(CID)
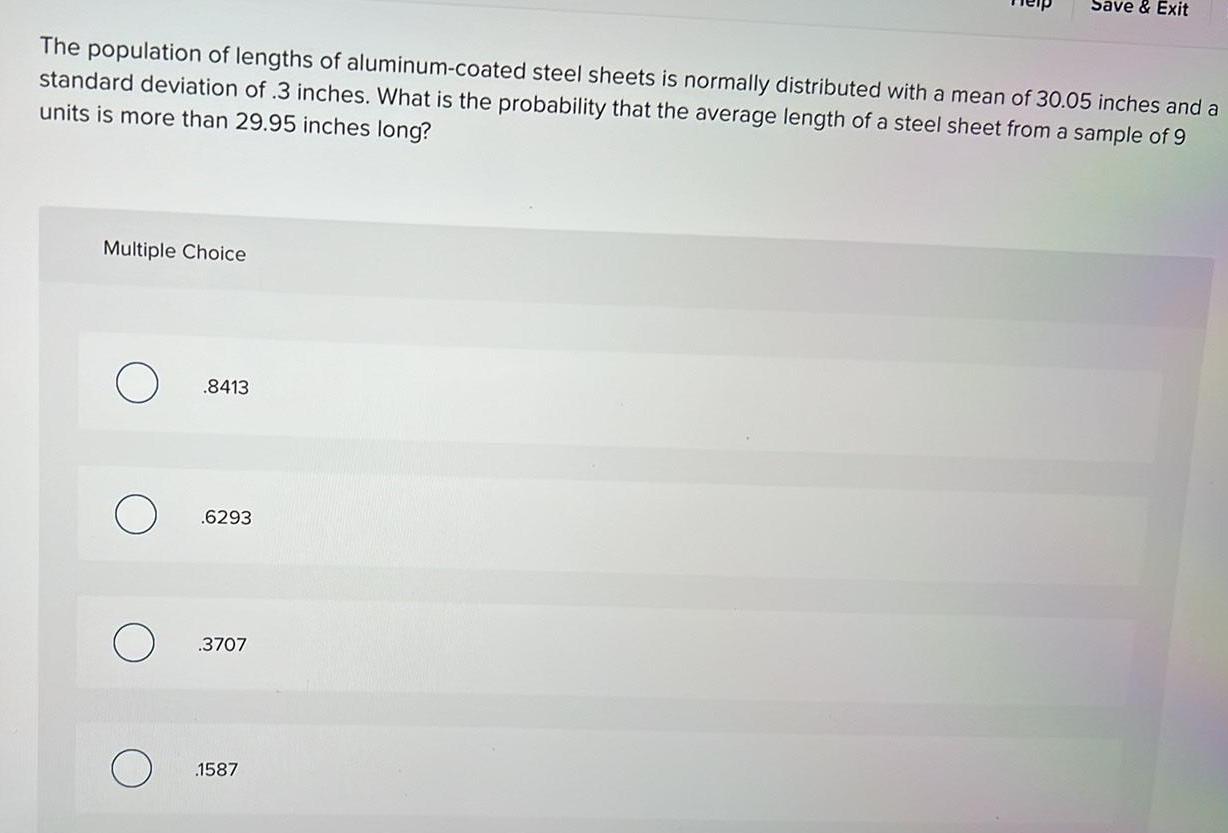
Math
StatisticsThe population of lengths of aluminum-coated steel sheets is normally distributed with a mean of 30.05 inches and a standard deviation of .3 inches. What is the probability that the average length of a steel sheet from a sample of 9 units is more than 29.95 inches long?
Multiple Choice
.8413
.6293
.3707
.1587
Math
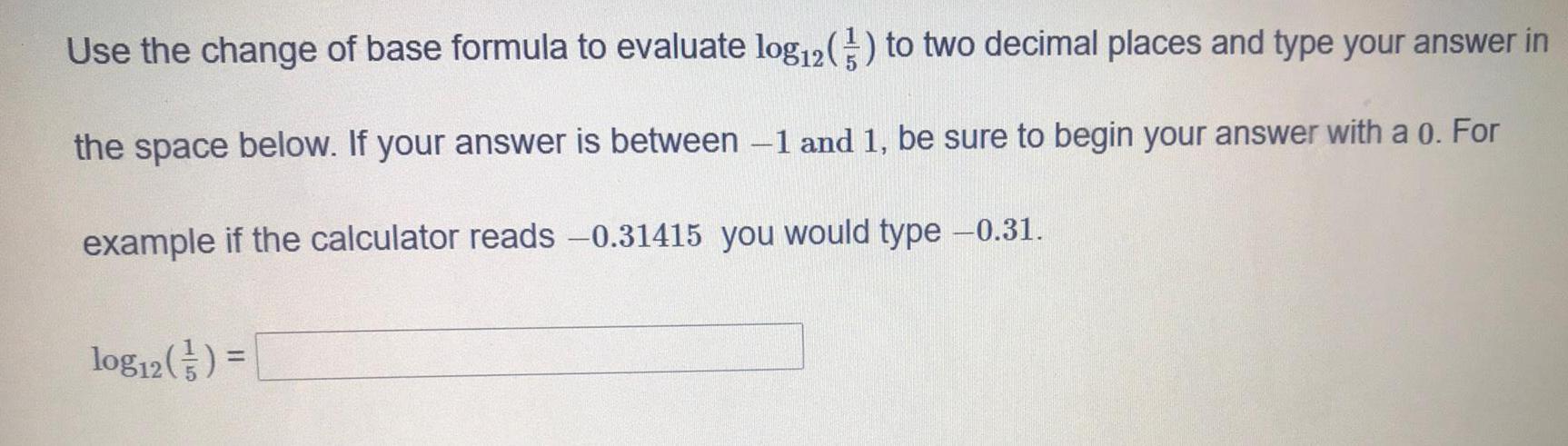
Basic MathUse the change of base formula to evaluate log12(1/5) to two decimal places and type your answer in the space below. If your answer is between -1 and 1, be sure to begin your answer with a0. For example if the calculator reads -0.31415 you would type -0.31.
log12(1/5)=___

Math
Basic MathAssume that a rectangle with sides x and y is expanding with time. Let y=2x and
x(t)=3t+1. What is the formula for the rate of change of the area with respect to time?
dA/dt=12(3t-1)
dA/dt=(3t+1)
dA/dt=4(3t - 1)
dA/dt=12(3t+1)