Math Questions
The best high school and college tutors are just a click away, 24×7! Pick a subject, ask a question, and get a detailed, handwritten solution personalized for you in minutes. We cover Math, Physics, Chemistry & Biology.
Math
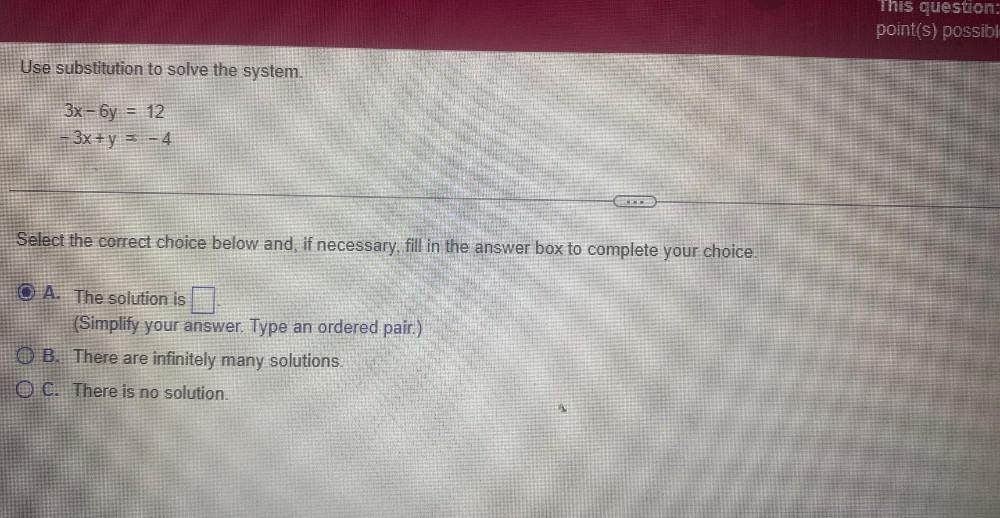
Basic MathUse substitution to solve the system.
3x - 6y = 12
- 3x + y = -4
Select the correct choice below and, if necessary, fill in the answer box to complete your choice.
○ A. The solution is ▢
(Simplify your answer. Type an ordered pair.)
○ B. There are infinitely many solutions.
○ C. There is no solution.
Math
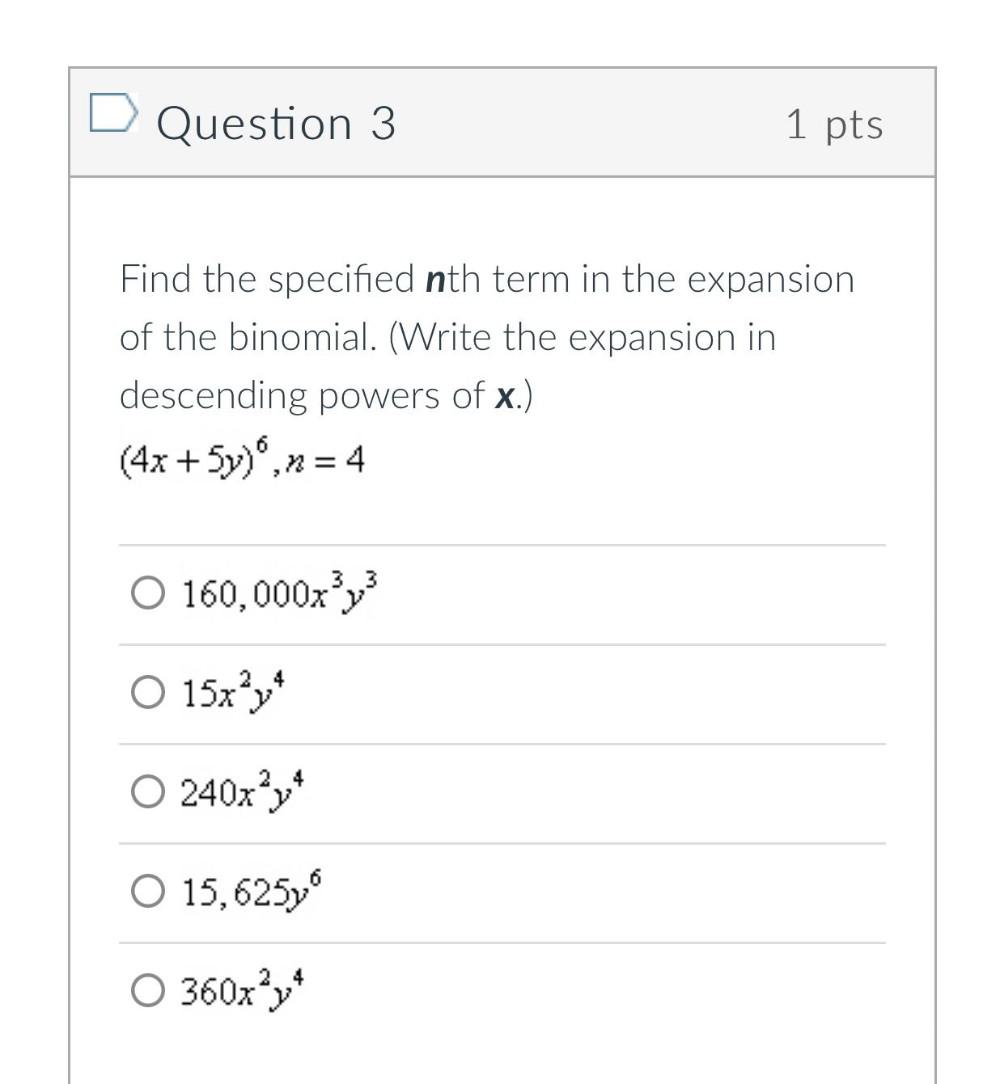
StatisticsFind the specified nth term in the expansion of the binomial. (Write the expansion in descending powers of x.)
(4x + 5y)^6 ,n=4
a)160,000x^3y^3
b)15x^2y^4
c)240x^2y^4
d)15,625y^6
e)360x^2y^4

Math
DifferentiationA ball is thrown into the air from a tree house and eventually lands on the ground. The equation below shows the distance in feet the ball is from the ground seconds after it is thrown. How high will the ball go? Show your work on paper. d(t) = -t^2 +4t +4
Math
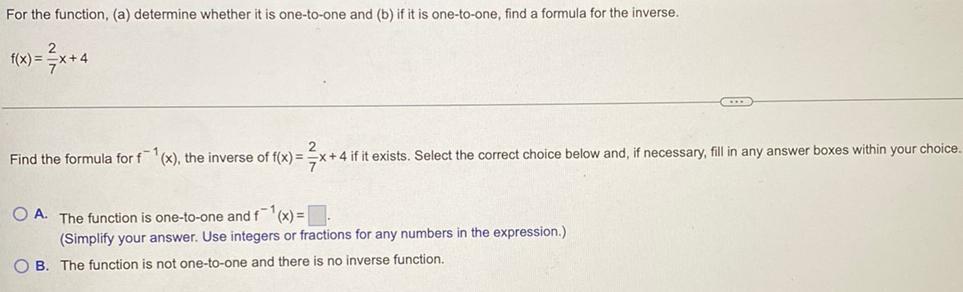
FunctionsFor the function, (a) determine whether it is one-to-one and (b) if it is one-to-one, find a formula for the inverse.
f(x) = 2/7x+4
Find the formula for f⁻¹(x), the inverse of f(x) = 2/7x+4 if it exists. Select the correct choice below and, if necessary, fill in any answer boxes within your choice.
A. The function is one-to-one and f⁻¹(x) = ▢.
(Simplify your answer. Use integers or fractions for any numbers in the expression.)
B. The function is not one-to-one and there is no inverse function.
Math
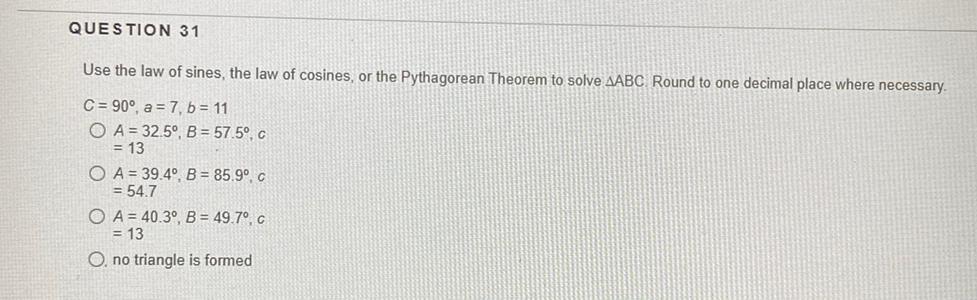
Basic MathUse the law of sines, the law of cosines, or the Pythagorean Theorem to solve ∆ABC Round to one decimal place where necessary.
(A) C = 90°, a = 7, b = 11
(B) A = 32.5°, B = 57.5°, c = 13
(C) A = 39.4°, B = 85.9°C = 54.7
(D) A = 40.3°, B = 49.7°C, c = 13
(E) no triangle is formed.
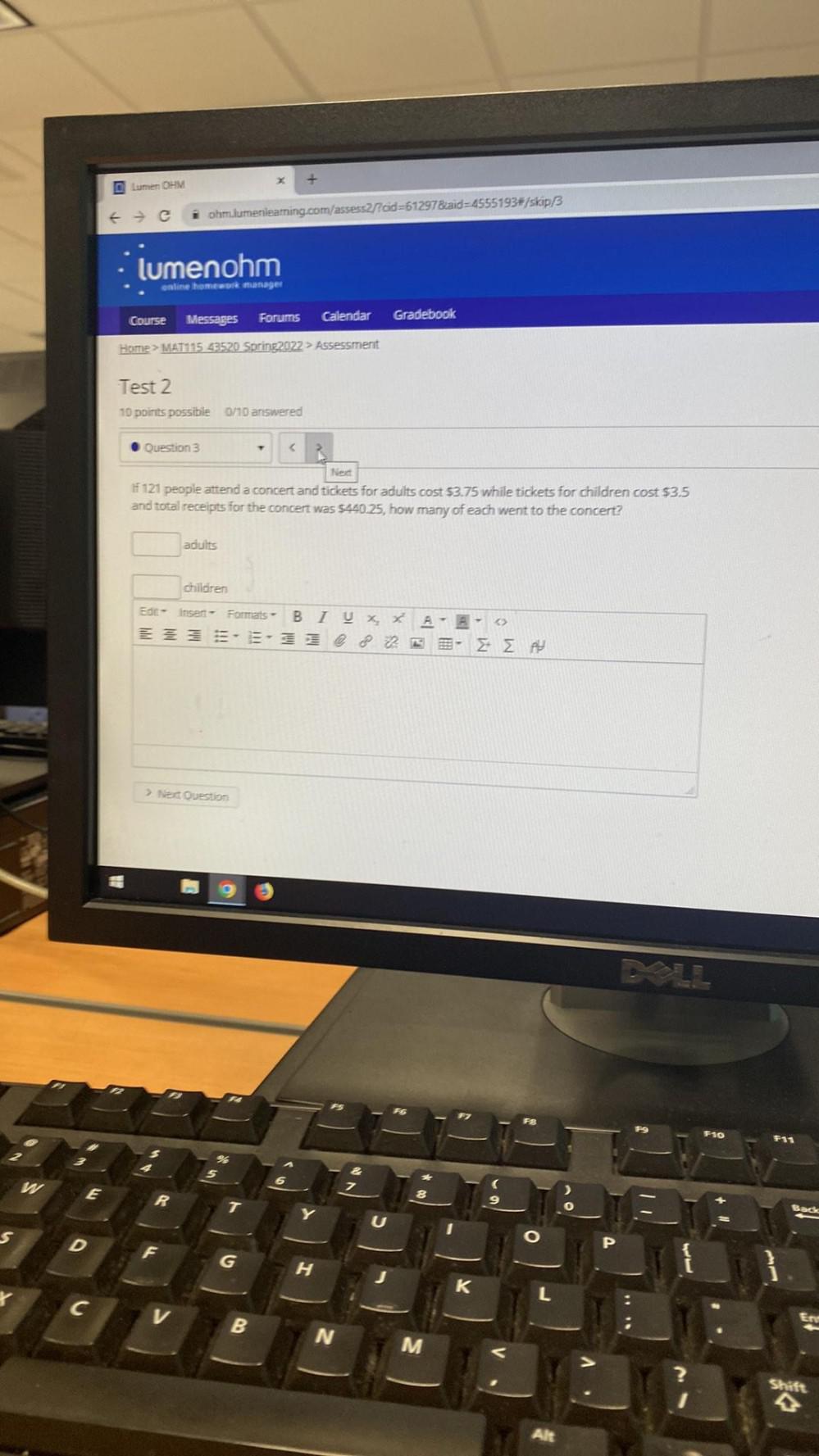
Math
Basic MathIf121 people attend a concert and tickets for adults cost $53.75 while tickets for children cost $3.5 and total receipts for the concert was $440.25, how many of each went to the concert?
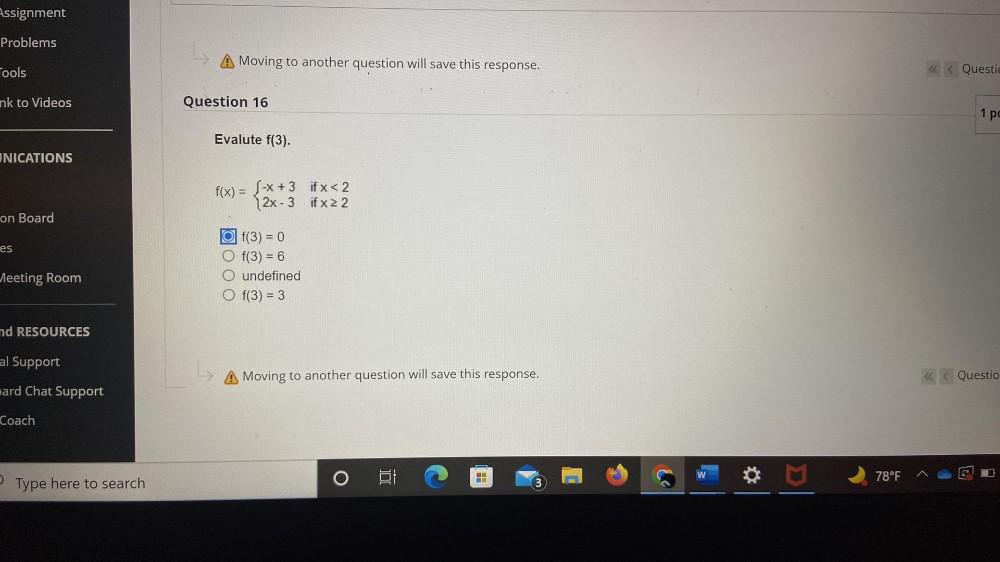
Math
FunctionsEvaluate f(3).
f(x) = -x+3 if x < 2
2x-3 if x ≥ 2
A) f(3) = 0
B) f(3) = 6
C) undefined
D) f(3) = 3

Math
Basic MathA football team sold raffle tickets to raise money for the upcoming season. They sold three different types of tickets: premium for $8, deluxe for $3, and regular for $1. The total number of tickets sold was 155, and the total amount of money from raffle tickets was $409. If 19 more deluxe tickets were sold than premium tickets, how many premium tickets were sold?
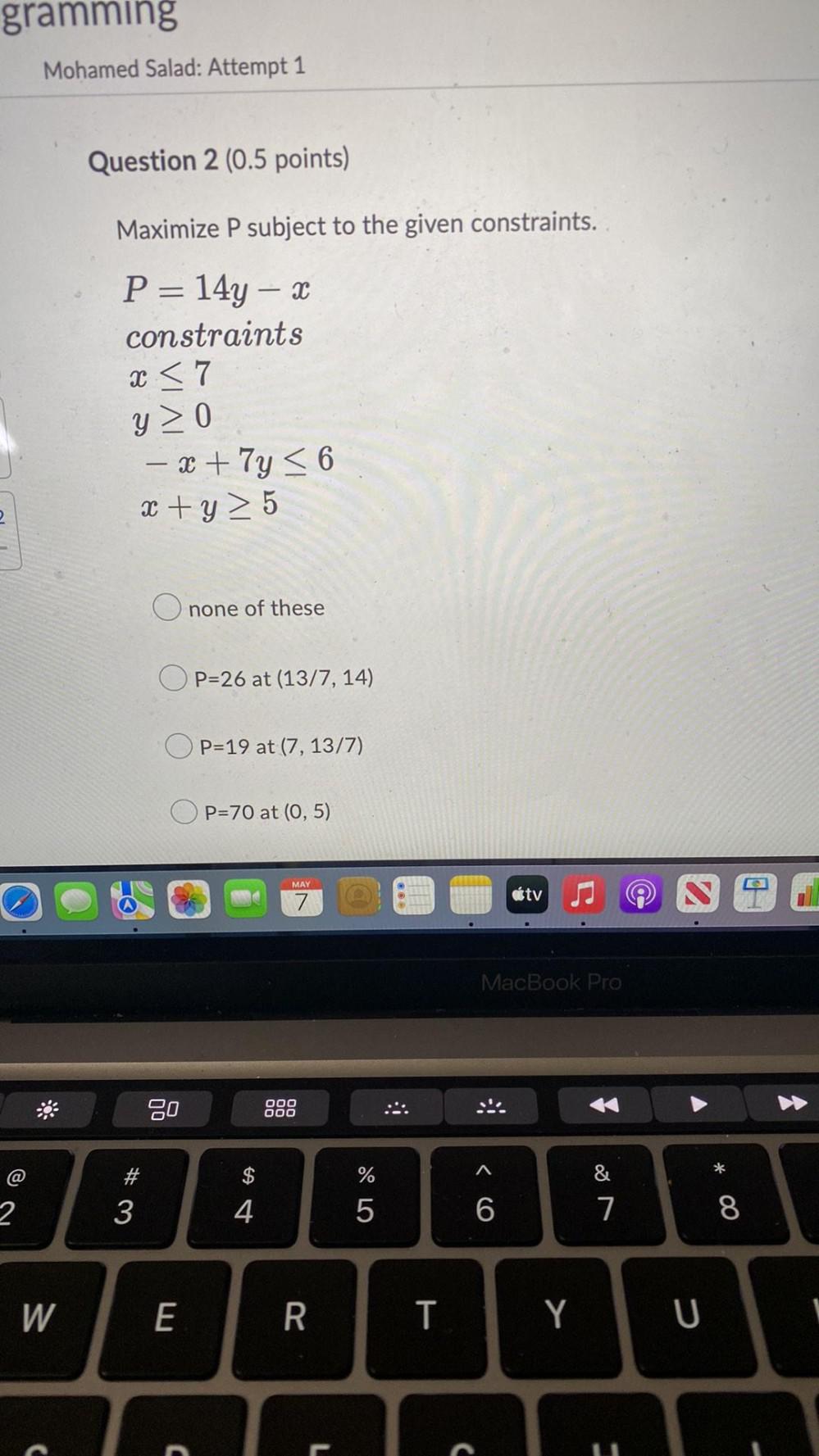
Math
Linear ProgrammingMaximize P subject to the given constraints. P= 14y – x constraints
x ≤ 7
y ≥ 0
-x + 7y ≤ 6
x +y ≥ 5
a. none of these
b. P = 26 at (13/7, 14)
c. P = 19 at (7, 13/7)
d. P = 70 at (0,5)
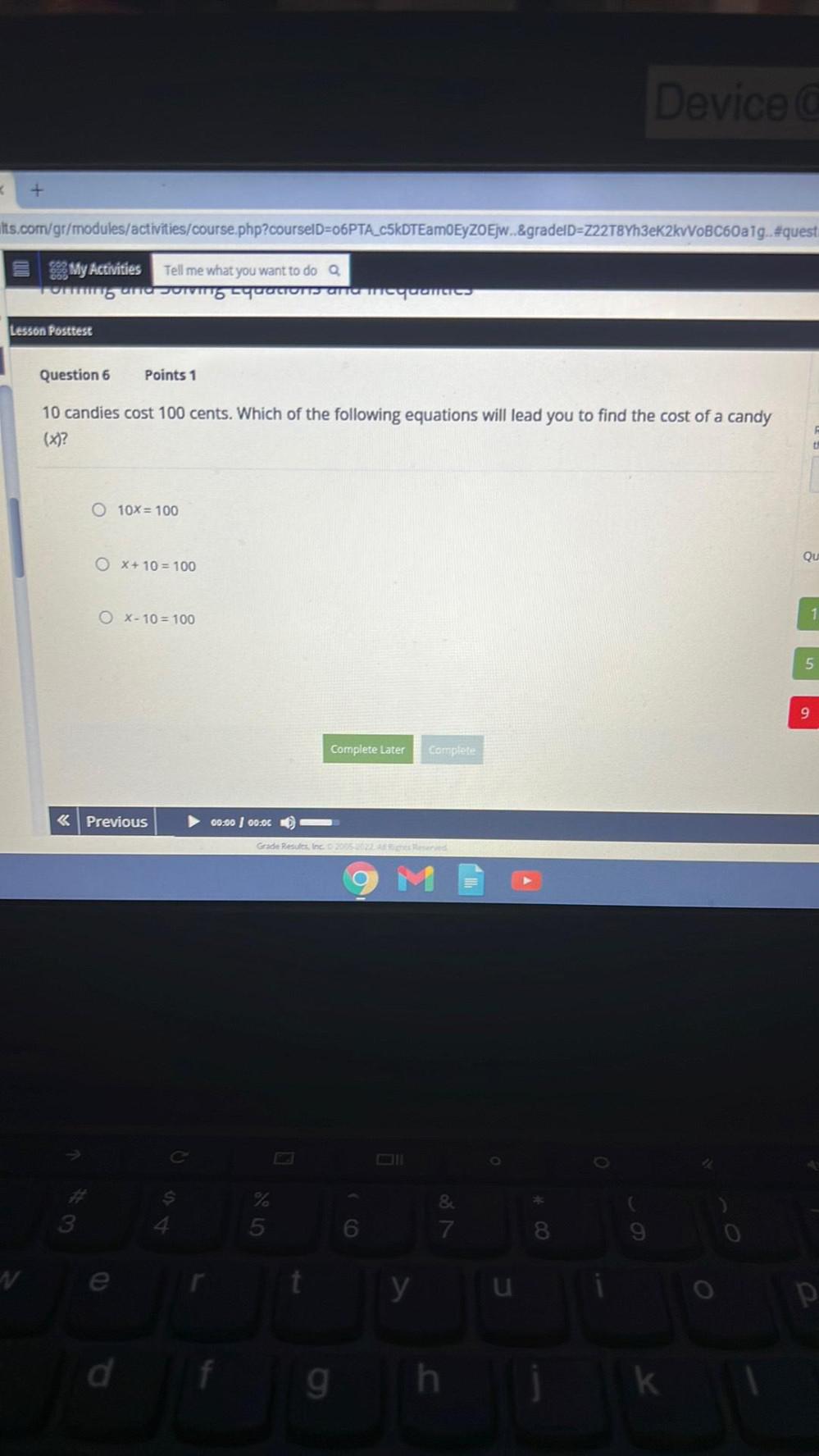
Math
Basic Math10 candies cost 100 cents. Which of the following equations will lead you to find the cost of a candy (x)?
O 10^x = 100
O x+10 = 100
O x-10 = 100
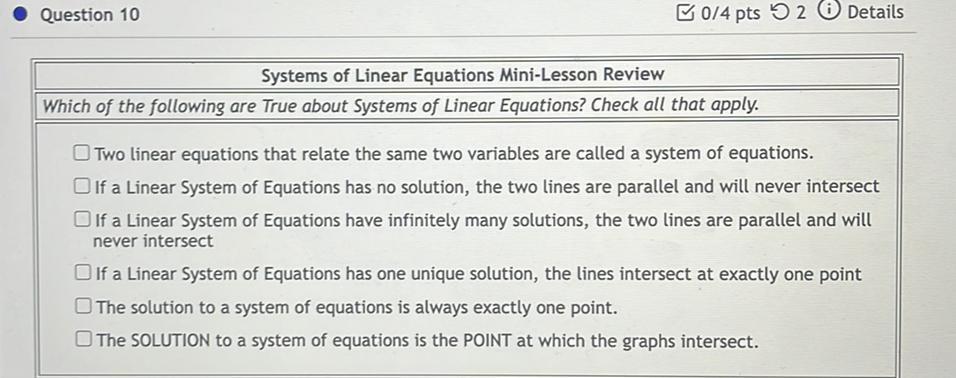
Math
Coordinate systemSystems of Linear Equations Mini-Lesson Review
Which of the following are True about Systems of Linear Equations? Check all that apply.
▢ Two linear equations that relate the same two variables are called a system of equations.
▢ lf a Linear System of Equations has no solution, the two lines are parallel and will never intersect
▢ If a Linear System of Equations have infinitely many solutions, the two lines are parallel and will never intersect
▢ If a Linear System of Equations has one unique solution, the lines intersect at exactly one point.
▢ The solution to a system of equations is always exactly one point.
▢ The SOLUTION to a system of equations is the POINT at which the graphs intersect.

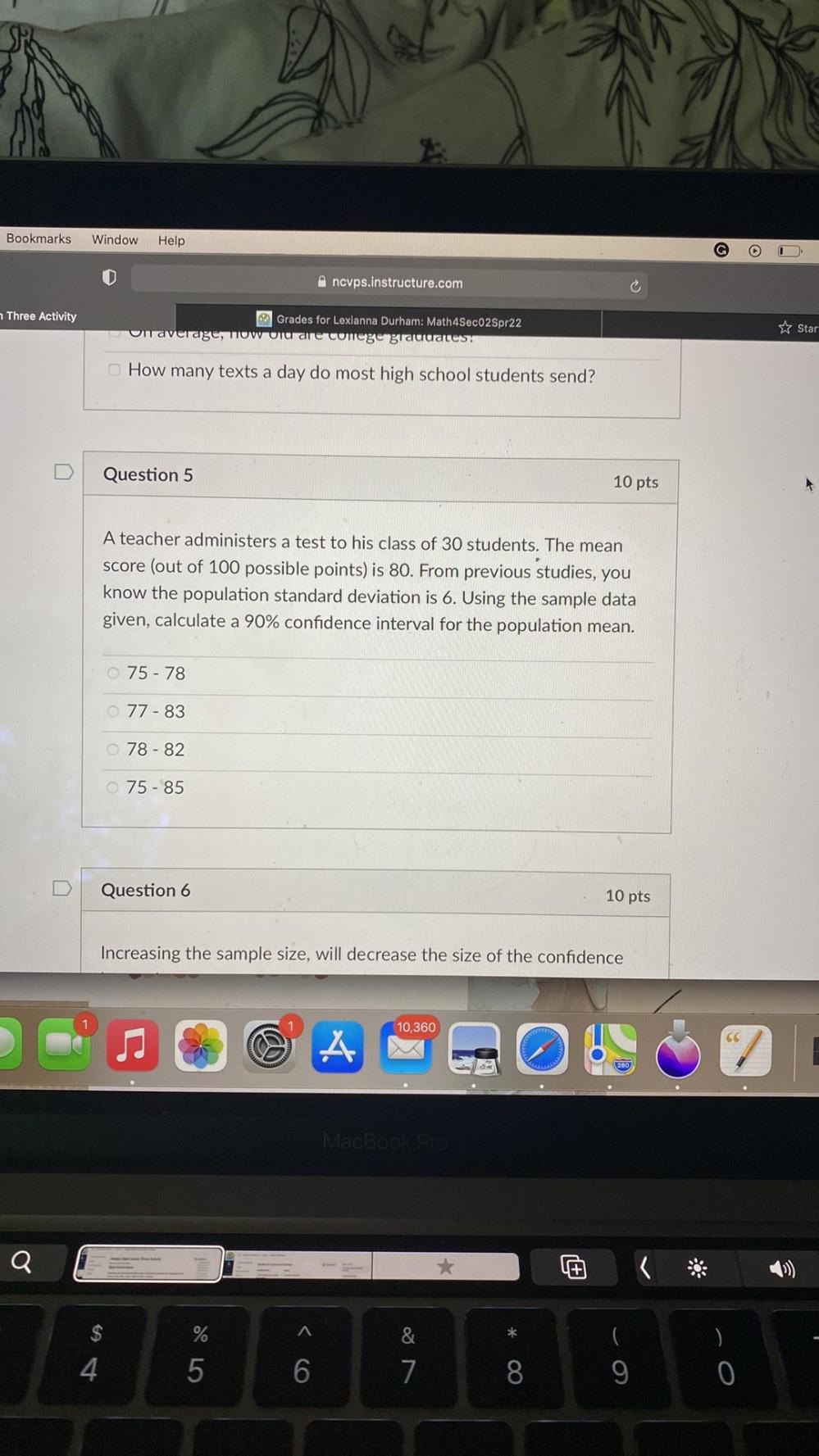
Math
Basic MathA teacher administers a test to his class of 30 students. The mean score (out of 100 possible points) is 80. From previous studies, you know the population standard deviation is 6. Using the sample data given, calculate a 90% confidence interval for the population mean.
(A) 75 - 78
(B) 77 - 83
(C) 78 - 82
(D) 75 - 85
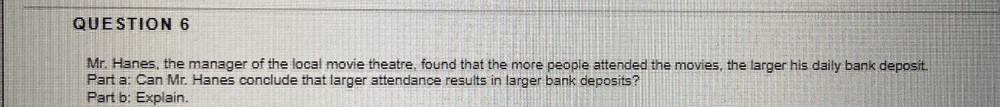
Math
Basic MathMr. Hanes, the manager of the local movie theatre, found that the more people attended the movies, the larger his daily bank deposit.
a): Can Mr. Hanes conclude that larger attendance results in larger bank deposits?
b): Explain.
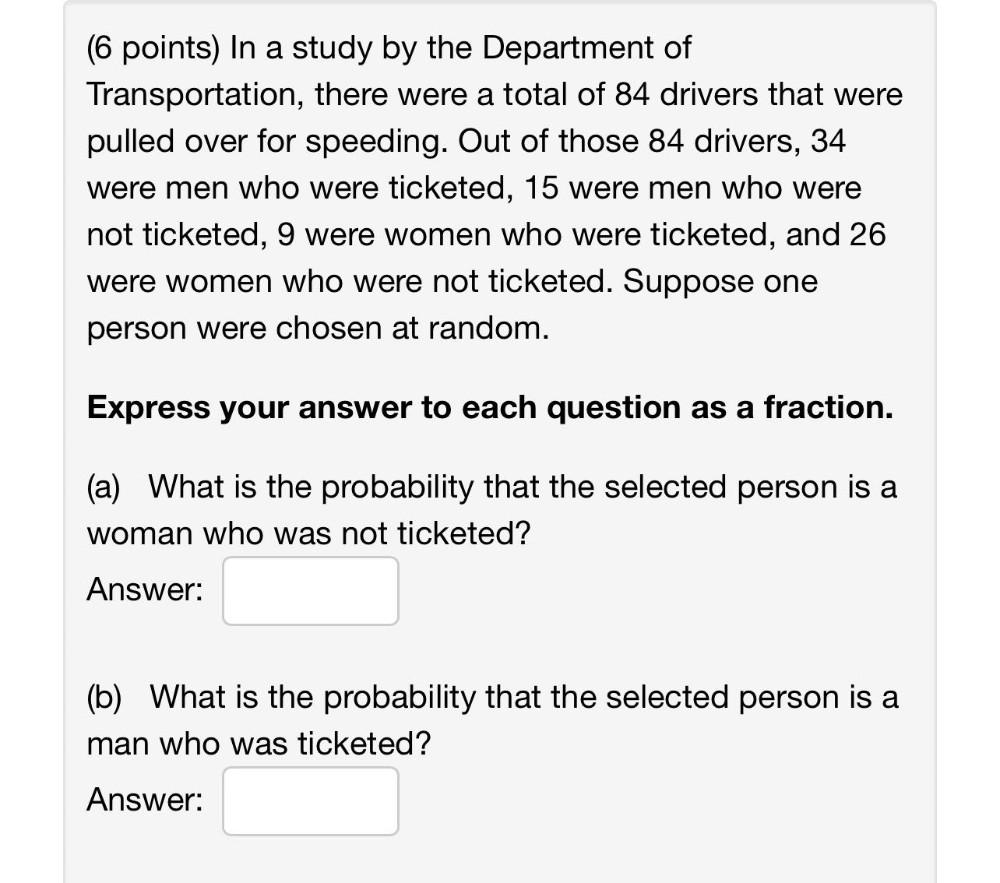
Math
ProbabilityIn a study by the Department of Transportation, there were a total of 84 drivers that were pulled over for speeding. Out of those 84 drivers, 34 were men who were ticketed, 15 were men who were not ticketed, 9 were women who were ticketed, and 26 were women who were not ticketed. Suppose one person were chosen at random.
Express your answer to each question as a fraction.
(a) What is the probability that the selected person is a
woman who was not ticketed?
Answer:
(b) What is the probability that the selected person is a
man who was ticketed?
Answer:

Math
Basic MathA water tank that holds 500 gallons and leaks 1/2 gallon of water every hour.
(A) What is the rate of change for the function that models the number of gallons in the tank for a given number of hours?
(B) Write an equation that represents this function.
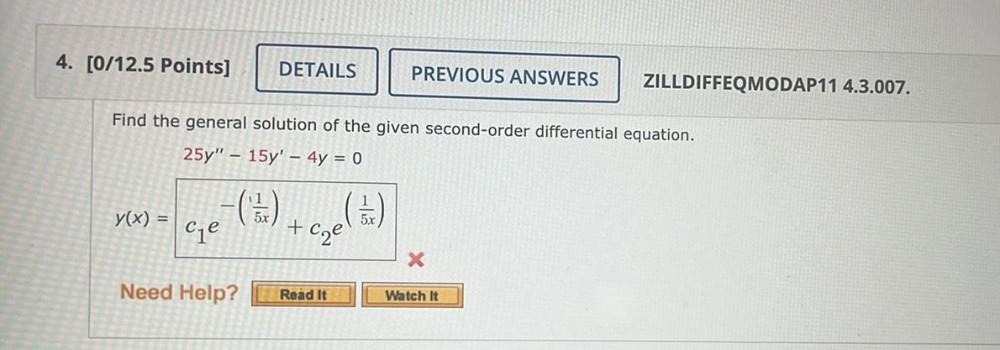
Math
Differential equationsFind the general solution of the given second-order differential equation.
25y" – 15y' - 4y = 0

Math
Permutations and CombinationsAt a high school cafeteria, diners can choose one vegetable from a choice of 5 vegetables, one meat from a choice of 2 meats, one serving of bread from among 2 breads, and a dessert from among 3 desserts. How many meal configurations are possible?
a) 20
b)4
c)60
d)12
d)12
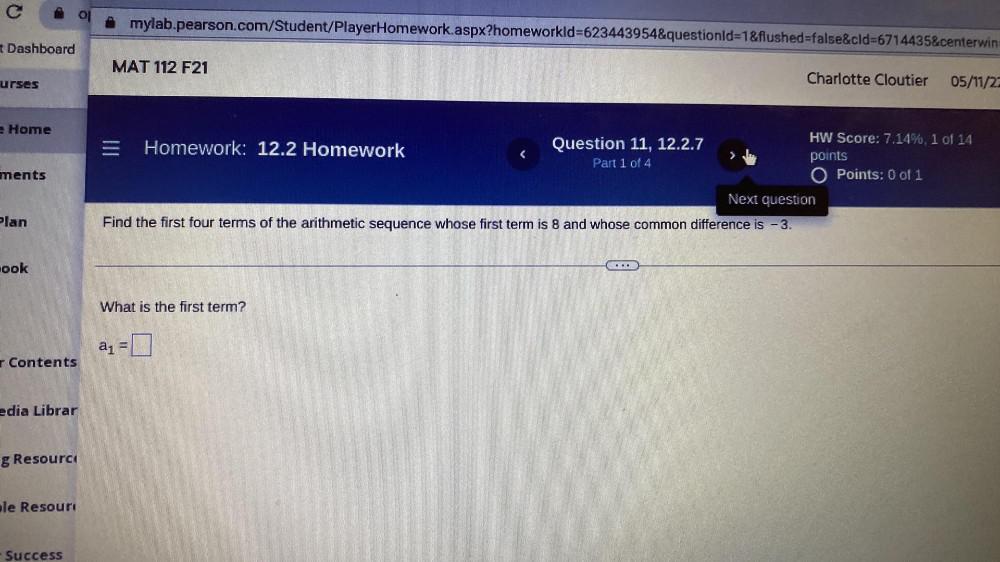
Math
Sequences & SeriesFind the first four terms of the arithmetic sequence whose first term is 8 and whose common difference is -3.
What is the first term?
a₁ = ▢
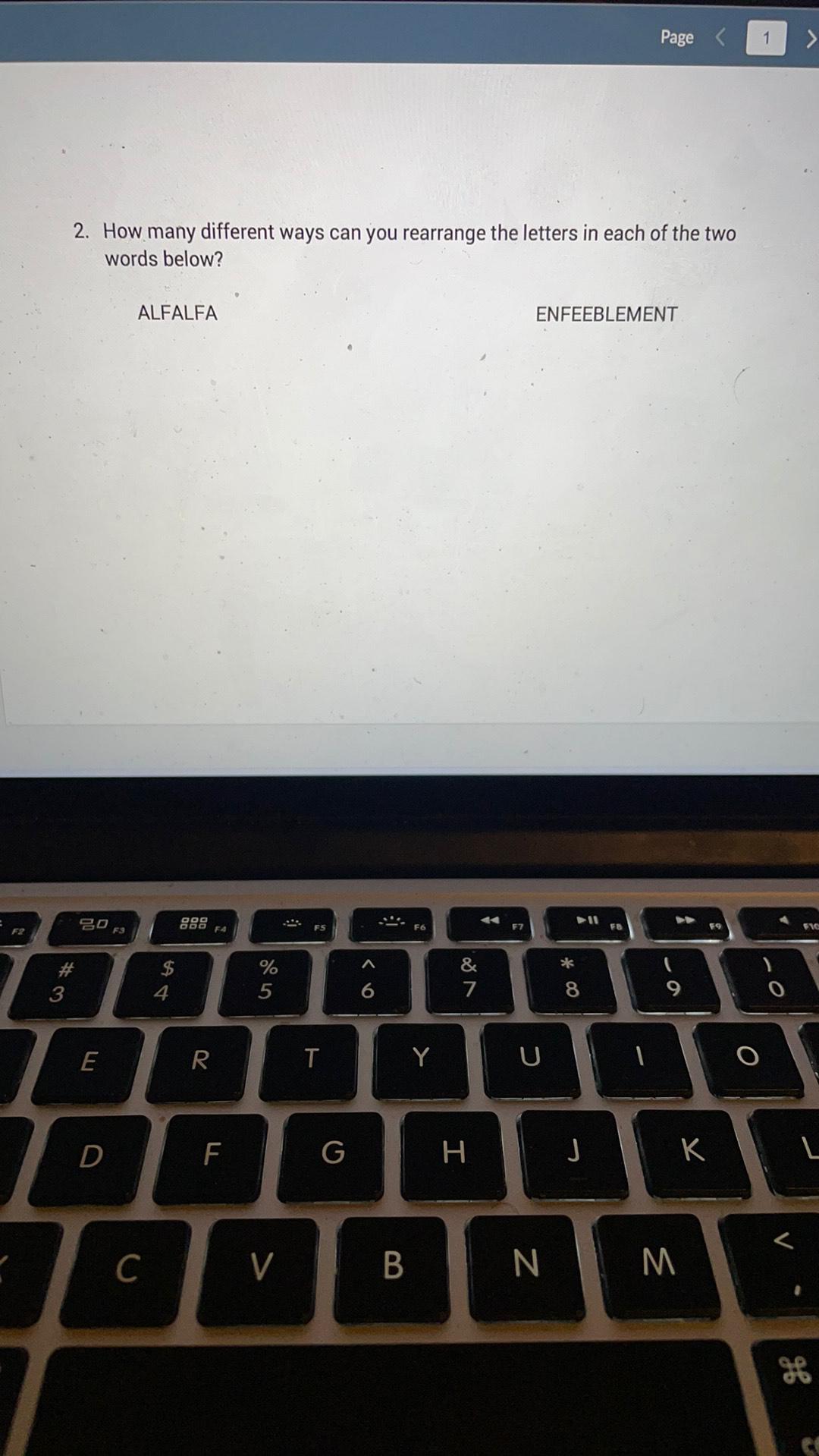
Math
Permutations and CombinationsHow many different ways can you rearrange the letters in each of the two
words below?
ALFALFA ENFEEBLEMENT

Math
Basic MathBlake is going to invest $34,000 and leave it in an account for 9 years. Assuming the interest is compounded annually, what interest rate, to the nearest tenth of a percent, would be required in order for Blake to end up with $50,000?

Math
Matrices & DeterminantsUse the inverse of the coefficient matrix to solve the system of equations.
x + 4y + 3z = - 26
x - 3y - 2z = 11
2x + 5y + 4z = -11
(x,y,z)= (__,__,__)
Math
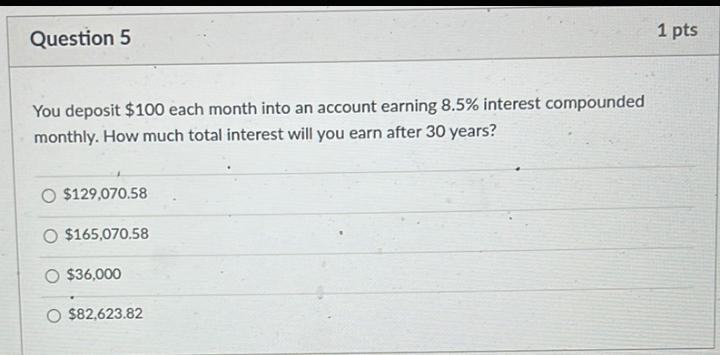
Basic MathYou deposit $100 each month into an account earning 8.5% interest compounded monthly. How much total interest will you earn after 30 years?
a)$129,070.58
b)$165,070.58
c)$36,000
d)$82,623.82

Math
Basic MathDaniel is going to invest $860 and leave it in an account for 14 years. Assuming the interest is compounded quarterly, what interest rate, to the nearest hundredth of a percent, would be required in order for Daniel to end up with $1,060?

Math
FunctionsSolve the equation by factoring: (x + 4)(x + 5) = 11x + 68
x=
Write your answers as a list of integers or reduced fractions, with your answers separated by (a) comma(s). For example, if you get 4 and-2/3 as your answers, then enter 4,-2/3 in the box.
Math
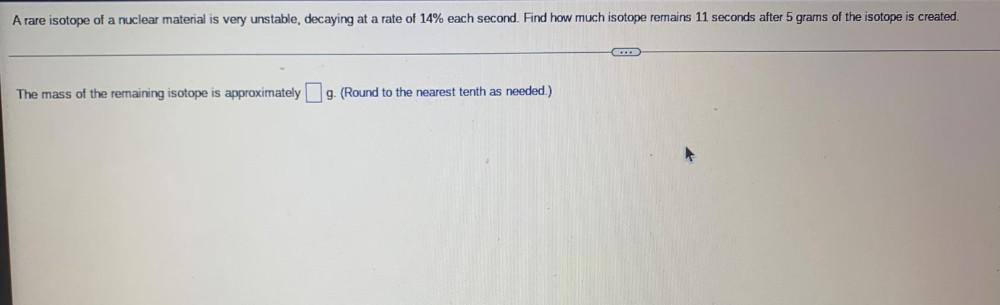
Basic MathA rare isotope of a nuclear material is very unstable, decaying at a rate of 14% each second. Find how much isotope remains 11 seconds after 5 grams of the isotope is created.

Math
ProbabilityFind the probability for the experiment of drawing two marbles (without replacement) from a bag containing 3 green, 5 yellow, and 2 red marbles such that both marbles are yellow.
○ 6/7
○ 1/3
○ 2/9
○ 1/8
○ 1/4
Math
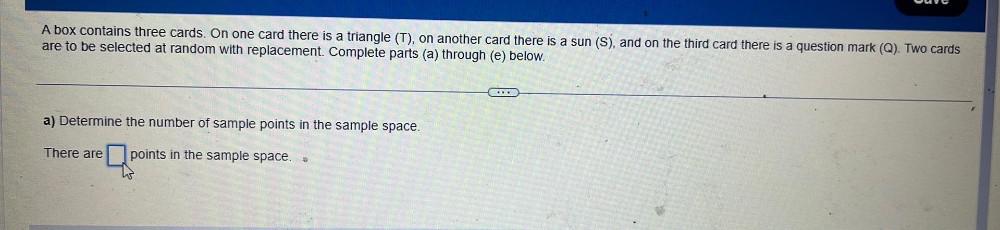
Permutations and CombinationsA box contains three cards. On one card there is a triangle (T), on another card there is a sun (S), and on the third card there is a question mark (Q). Two cards are to be selected at random with replacement. Complete parts (a) through (e) below.
a) Determine the number of sample points in the sample space.
Math
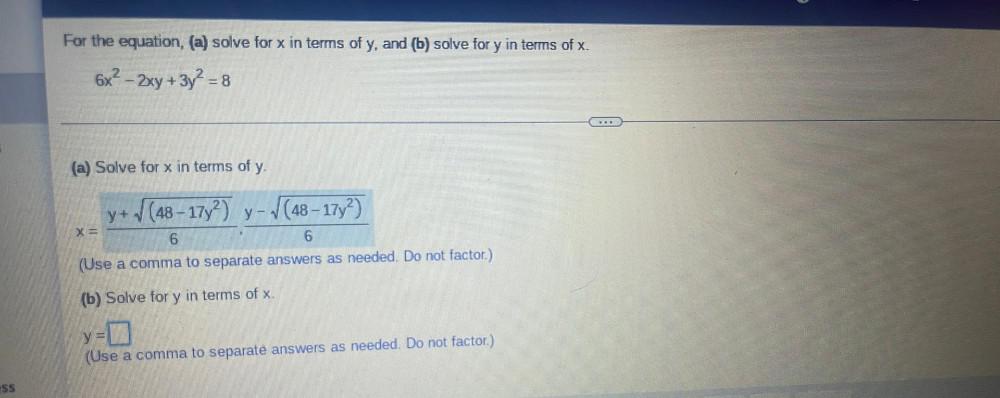
Quadratic equationsFor the equation, (a) solve for x in terms of y, and (b) solve for y in terms of x.
6x² - 2xy + 3y² = 8
(a) Solve for x in terms of y.
x = y+√(48 - 17y²)/6, y- √(48 - 17y²)/6
(Use a comma to separate answers as needed. Do not factor.)
(b) Solve for y in terms of x.
y= ▢
(Use a comma to separate answers as needed. Do not factor)
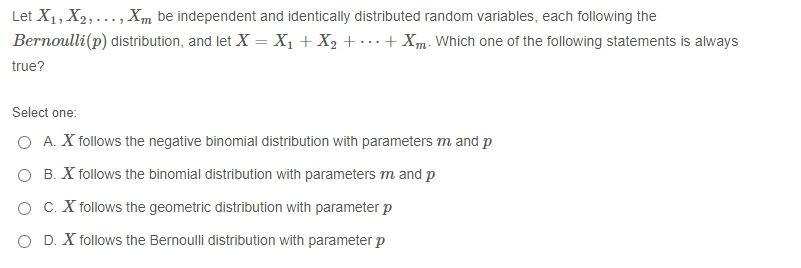
Math
ProbabilityLet X1, X2,..., Xm be independent and identically distributed random variables, each following the Bernoulli(p) distribution, and let X = X_1 + X_2 + ... + X_m.
Which one of the following statements is always true?
Select one:
A. X follows the negative binomial distribution with parameters m and p
B. X follows the binomial distribution with parameters m and p
C. X follows the geometric distribution with parameter p
D. X follows the Bernoulli distribution with parameter p
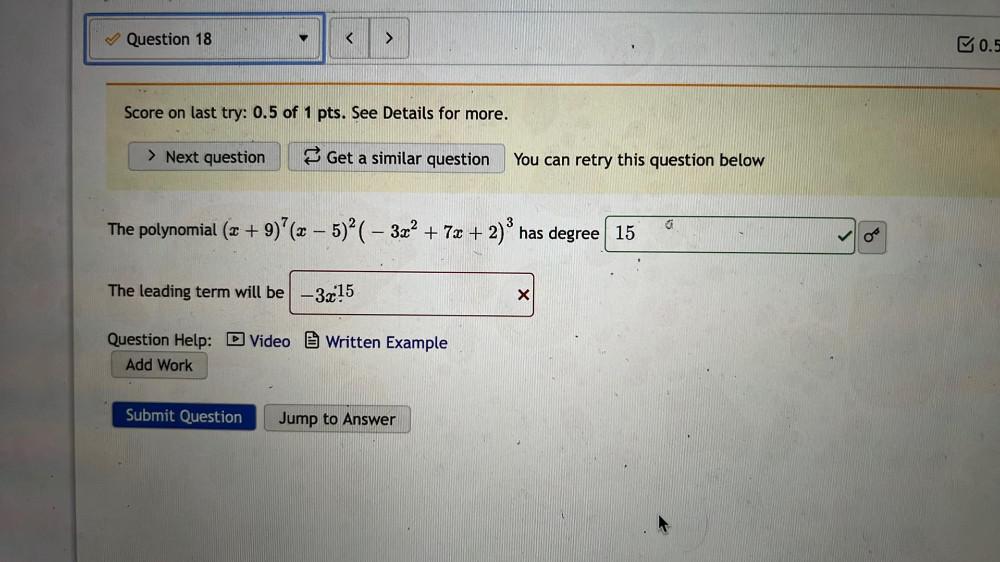
Math
Binomial theoremThe polynomial (x + 9)⁷ (x – 5)² (- 3x² + 7x + 2)³ has degree?
The leading term will be?
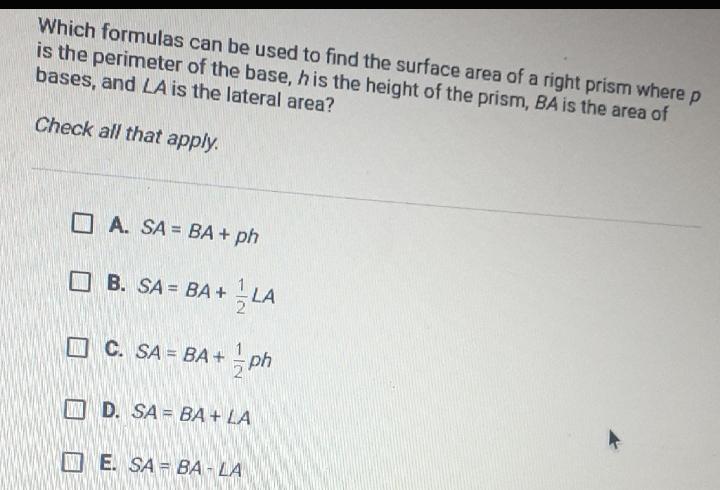
Math
AreaWhich formulas can be used to find the surface area of a right prism where p
is the perimeter of the base, h is the height of the prism, BA is the area of
bases, and LA is the lateral area? Check all that apply.
A. SA = BA + ph
B. SA = BA+ 1/2 LA
C. SA = BA + 1/2 ph
D. SA = BA + LA
E. SA - BA - LA
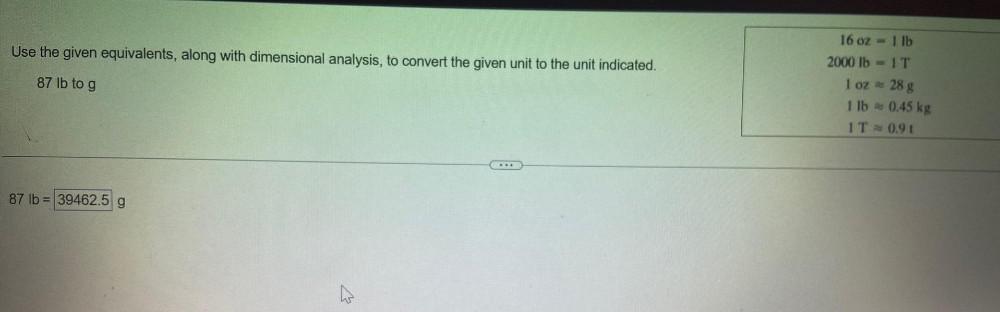
Math
Basic MathUse the given equivalents, along with dimensional analysis, to convert the given unit to the unit indicated.
87 lb to g

Math
Basic MathIn 2001, the mouse population in a park was measured to be 4,860. By 2009, the population was measured again and was found to be 5,980. Assume the population continues to change line
(a) Find a formula for the moose population, P, since 2001. (Let t represent the number of years since 2001.)
P(t)=_____
(b) What does your model predict the moose population to be in 2023?
_______moose

Math
ProbabilityHeights of fourth-graders are normally distributed with a mean of 52 inches and a standard deviation of 3.5 inches. Find the probability that a randomly selected fourth-grader is taller than 60 inches.
2.3%
5%
3%
1.1%
![Let f be a twice-differentiable function with derivative given by f'(x) = 4x³ - 24x².
(A) Find the x-coordinate of any possible critical points of f. Show your work.
(B) Find the x-coordinate of any possible inflection points of f. Show your work.
(C) Use the Second Derivative Test to determine any relative extrema and inflection points. Justify your answers.
(D) If f has only one critical point on the interval [5, 8], what is true about the function f on the interval [5, 8]? Justify your answers.](https://media.kunduz.com/media/sug-question/raw/77435682-1658836111.8369439.jpeg?w=256)
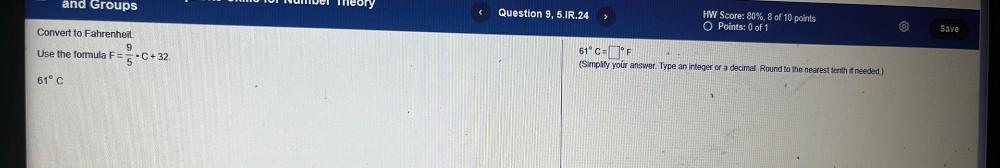
Math
DifferentiationLet f be a twice-differentiable function with derivative given by f'(x) = 4x³ - 24x².
(A) Find the x-coordinate of any possible critical points of f. Show your work.
(B) Find the x-coordinate of any possible inflection points of f. Show your work.
(C) Use the Second Derivative Test to determine any relative extrema and inflection points. Justify your answers.
(D) If f has only one critical point on the interval [5, 8], what is true about the function f on the interval [5, 8]? Justify your answers.
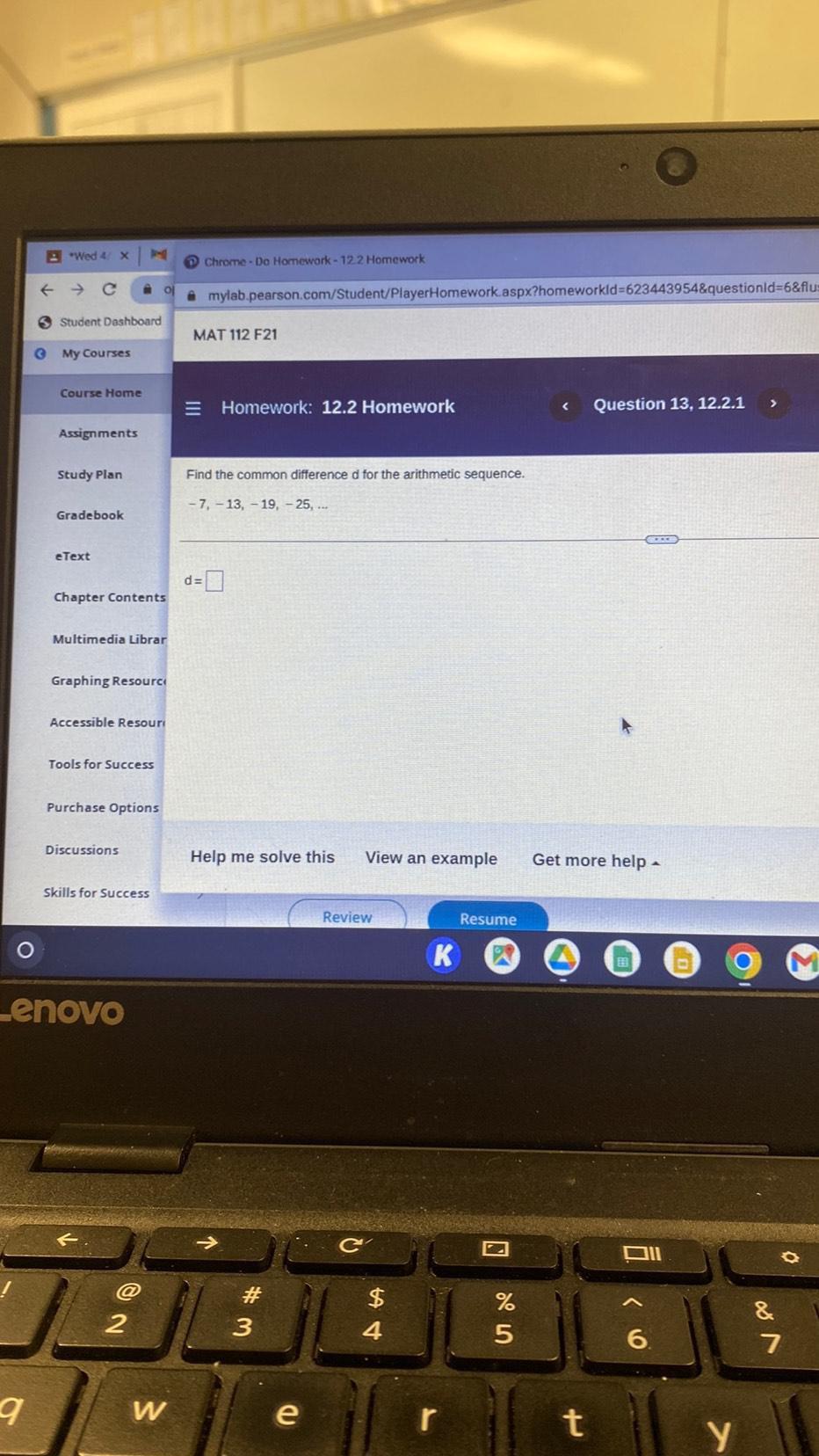
Math
Sequences & SeriesFind the common difference d for the arithmetic sequence.
-7, -13, -19, -25,...
d = ▢
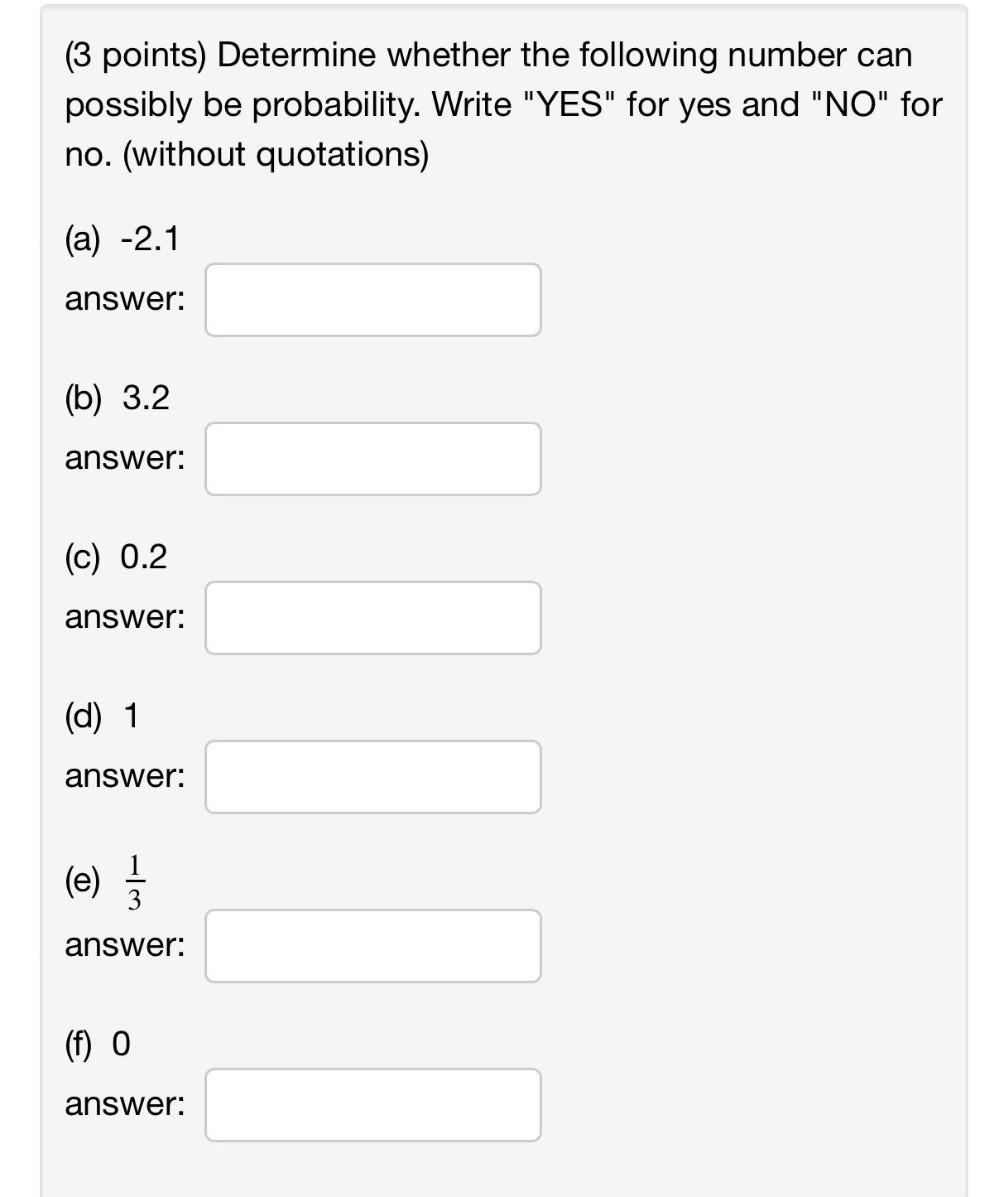
Math
ProbabilityDetermine whether the following number can possibly be probability. Write "YES" for yes and "NO" for no. (without quotations)
(a) -2.1
answer:
(b) 3.2
answer:
(c) 0.2
answer:
(d) 1
answer:
(e) 1/3
answer:
(f) 0
answer:
![Consider the differential equation dy/dx = x²(y + 1).
Sketch a slope field for the given differential equation on your paper for the domain [-3, 3] and the range [-3, 3].
(A) List five coordinates and the corresponding slope of their segments.
(B) Using the slope field that you drew, describe all points in the xy-plane for which the slopes are negative.](https://media.kunduz.com/media/sug-question/raw/77435223-1658835743.4339602.jpeg?w=256)
Math
Application of derivativesConsider the differential equation dy/dx = x²(y + 1).
Sketch a slope field for the given differential equation on your paper for the domain [-3, 3] and the range [-3, 3].
(A) List five coordinates and the corresponding slope of their segments.
(B) Using the slope field that you drew, describe all points in the xy-plane for which the slopes are negative.

Math
Basic MathFind the restricted values of x for the following rational expression. If there are no restricted values of x, indicate "No Restrictions"
(-3x^2 - 6)/6x
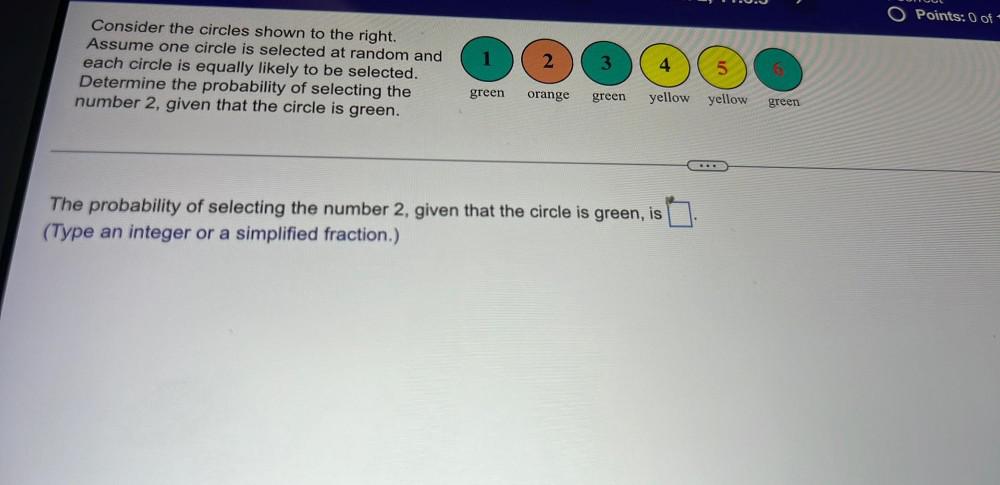
Math
Basic MathConsider the circles shown to the right. Assume one circle is selected at random and each circle is equally likely to be selected. Determine the probability of selecting the number 2, given that the circle is green.
1 2 4 5 6 7
green orange green yellow yellow green
The probability of selecting the number 2, given that the circle is green, is_______.
(Type an integer or a simplified fraction.)
Math
Basic MathAssume that a hat contains four bills: a $1, a $ 5, a $10, and a $20 bill. Two bills are to be selected at random with replacement. Find the probability that both bills are $20 if the first selected is a $20 bill?
The probability that both bills are $20 if the first selected is a $20 bill is
________.
(Simplify your answer. Type an integer or a fraction.)

Math
Coordinate systemA company recently found out that the new mechanical equipment it needs currently costs $42,000. In 10 years it will be out of date and thus can then be scrapped for $6,000. Create a line that shows the depreciation relationship of the cost (C) over time (t) in years. Graph the line. What is the slop of the line? What are the x and y intercepts of your line and what do they represent? How much would it be worth if it they decided to get rid of if early, after 7 years?
Math
ProbabilityPolygraph tests have an accuracy rate of about 85%. This means that it will detect 85% of people who lie and 85% of people who are telling the truth. A company of 5000 employees has everyone submit to a polygraph
test. It turns out there are 50 employees who will lie during their polygraph. (Hint: Tests Positive means they are lying!) Complete a table as in your course materials and use it to answer the given question.
If someone does pass their polygraph test, what is the probability they told the truth? (Round your probability to the nearest tenth of a percent!)
Express your answer rounded correctly to the nearest tenth of a percent.
Probability =
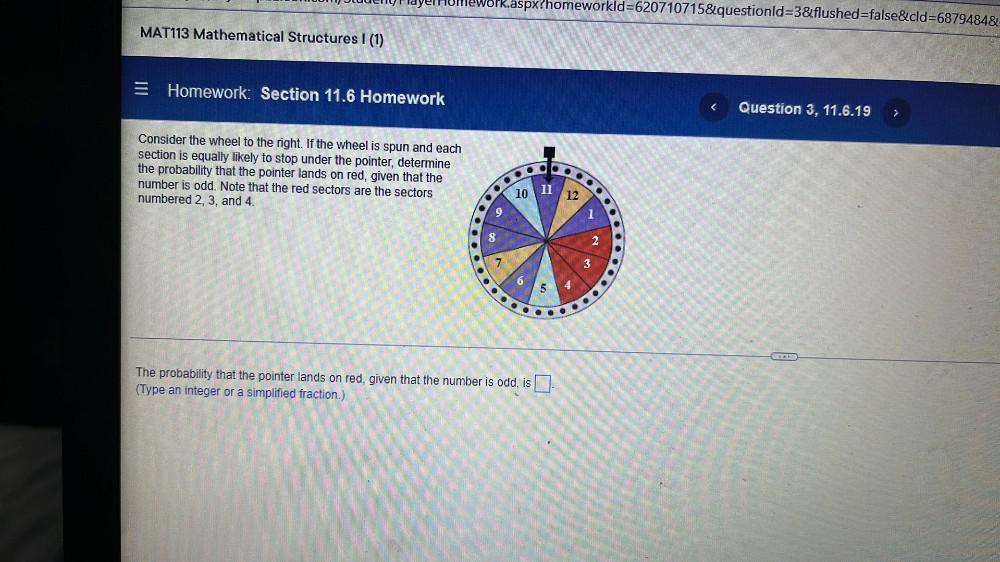
Math
ProbabilityConsider the wheel to the right. If the wheel is spun and each section is equally likely to stop under the pointer, determine the probability that the pointer lands on red, given that the number is odd. Note that the red sectors are the sectors
numbered 2, 3, and 4.
The probability that the pointer lands on red, given that the number is odd, is
(Type an integer or a simplified fraction.)

Math
StatisticsA researcher surveys 220 men and 190 women and finds that 207 of the men and 171 of the women wear their seatbelts. At alpha = 0.10, is there evidence to for the claim that men are more cautious based on their seat belt use?
What is the p-value?
A).1504
B).0828
C).0.3008
d).0.0618
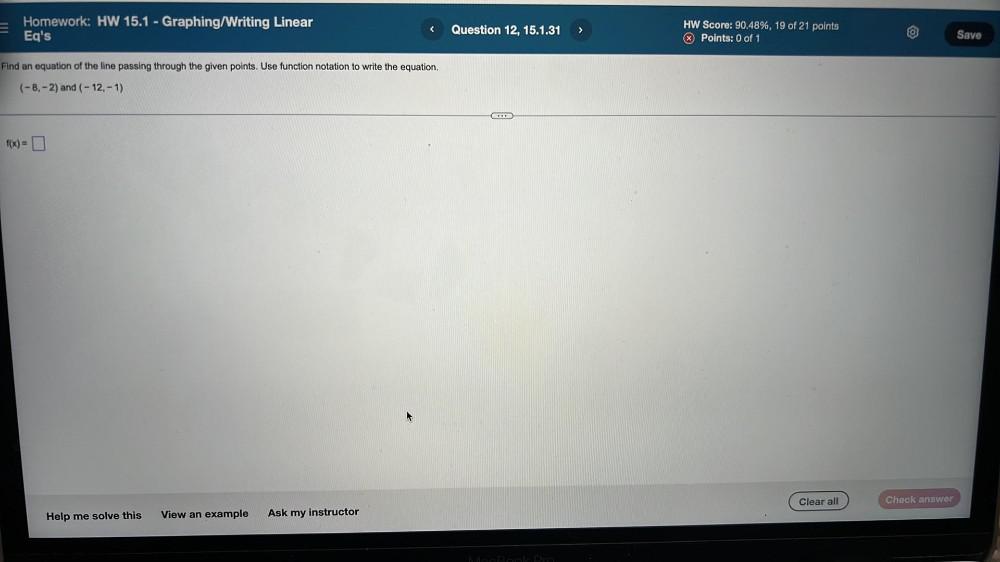
Math
Straight linesFind an equation of the line passing through the given points. Use function notation to write the equation (-8, -2) and (-12, -1).