Calculus Questions
The best high school and college tutors are just a click away, 24×7! Pick a subject, ask a question, and get a detailed, handwritten solution personalized for you in minutes. We cover Math, Physics, Chemistry & Biology.

Calculus
Definite Integrals1 The shaded regions R and R shown below are enclosed by the graphs of f x x and g x 2 R R I A Find the x and y coordinates of the three points of intersection of the graphs of f and g B Estimate the area of R to three reasonable places past the decimal C Find the EXACT area of R No calculator approximations
Calculus
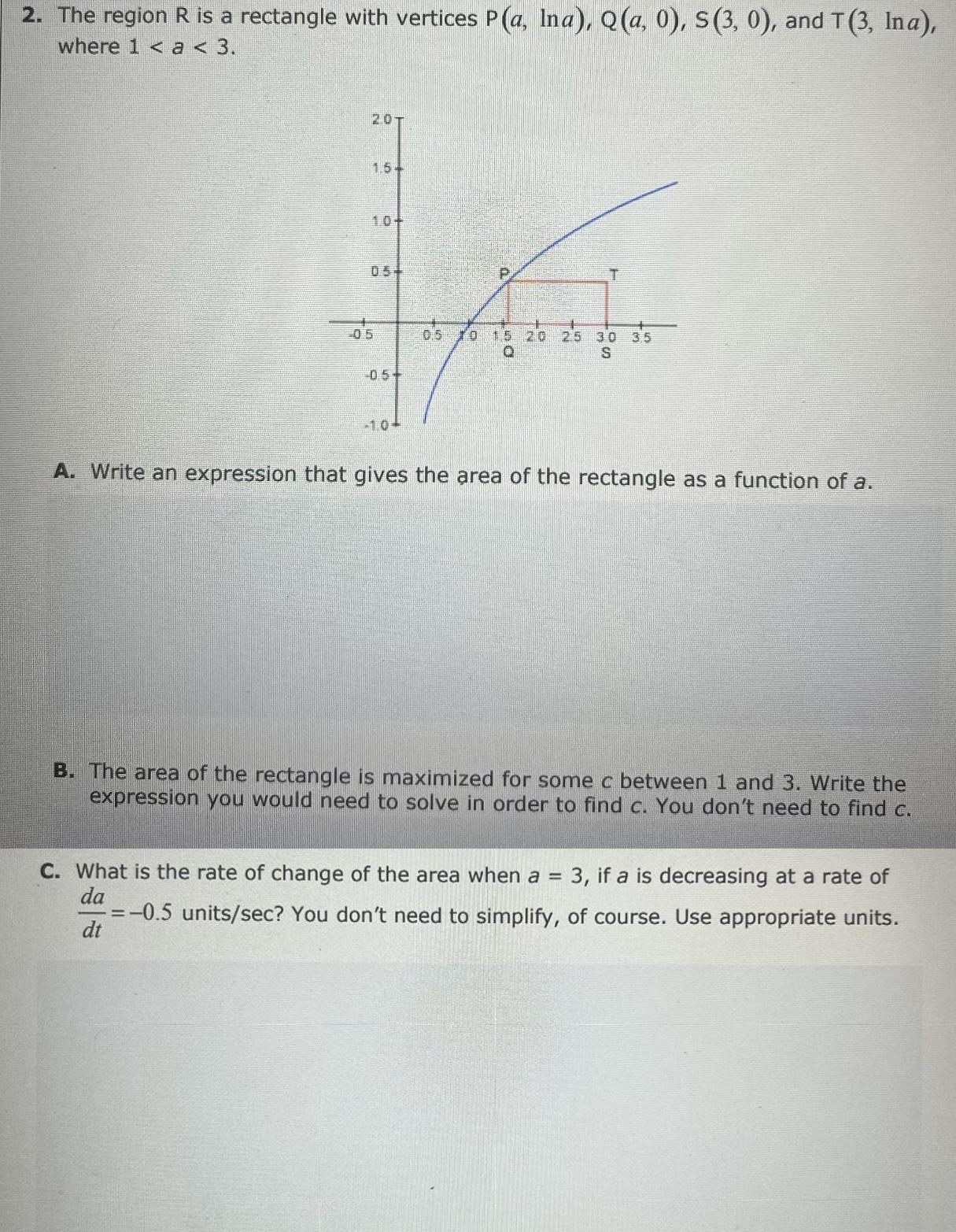
Definite Integrals2 The region R is a rectangle with vertices P a Ina Q a 0 S 3 0 and T 3 Ina where 1 a 3 201 1 54 05 1 0 P T 10 15 20 25 30 0 S A Write an expression that gives the area of the rectangle as a function of a B The area of the rectangle is maximized for some c between 1 and 3 Write the expression you would need to solve in order to find c You don t need to find c C What is the rate of change of the area when a 3 if a is decreasing at a rate of da 0 5 units sec You don t need to simplify of course Use appropriate units dt

Calculus
Indefinite IntegrationX O A B M dx 23 2 C 2 23 2 C C 223 23 2 C O D In 23 23 2 C O E x In 23 23 2 C

Calculus
Vector CalculusThe region in the first quadrant enclosed by the curves y 0 x 2 x 5and is rotated about the x axis The volume of the solid generated is 1 y 1 x O A In 5 O B T In O C T ln 2 O D In 10 OE E ln


Calculus
Differentiationg x x3 2x 1 What is the slope of the tangent line to the curve g x at the point 2 1 O A 1 OB 5 O C 8 OD 14 O E

Calculus
Application of derivativesThe position of an object moving vertically along a line is given by the function s t 4 9t2 26t 17 Find the average velocity of the object over the following intervals a 0 7 b 0 4 c 0 1 d 0 h where h 0 is a real number

Calculus
DifferentiationA 25 ft ladder is leaning against a vertical wall At what rate with respect to time is the angle between the ground and the ladder changing if the top of the ladder is sliding down the wall at the rate of rinches per second at the moment that the top of the ladder is h feet from the ground You re looking here for an equation in terms of h and 0 A 25 1 4 O A OB O C E 25 1 13 300 A 25 ft 12 1 4 1 25 1 4
Calculus
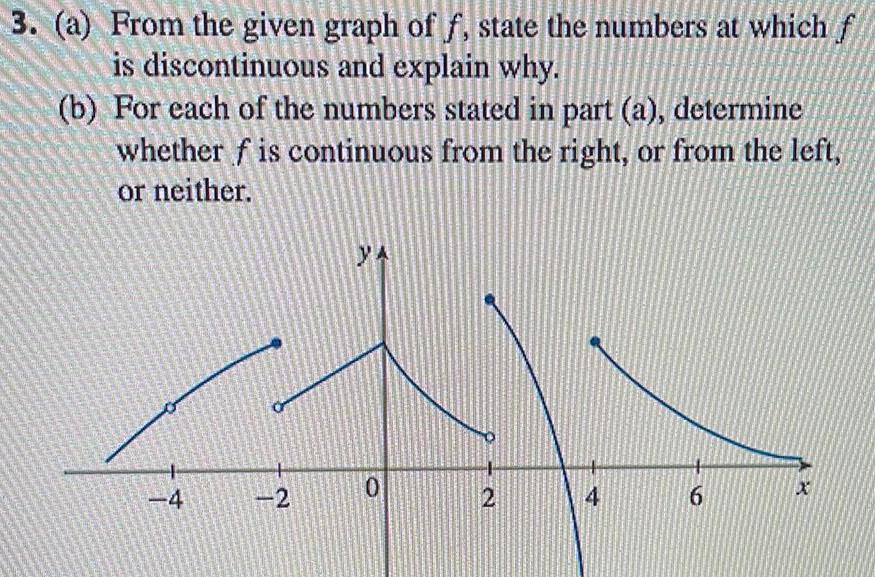
Vector Calculus3 a From the given graph of f state the numbers at which f is discontinuous and explain why b For each of the numbers stated in part a determine whether f is continuous from the right or from the left or neither 4 2 YA 0 2 4 6

Calculus
Application of derivativesO A 2 ln sin 0 2 4 C OB In sin 0 2 4 C O c 2 ln cos 0 2 4 C O D In cos 0 2 4 C OE None of these s

Calculus
Application of derivativesg x x 2x 1 What is the slope of the tangent line to the curve g x at the point 2 1 O A 1 OB 5 OC 8 OD 14 OE E

Calculus
Application of derivativesz 2 x y is an equation for the tangent plane to z f x y at the point 0 0 Which of the following is the linear approximation for f 0 02 0 01 O2 03 1 97 1 99 O2 01
Calculus
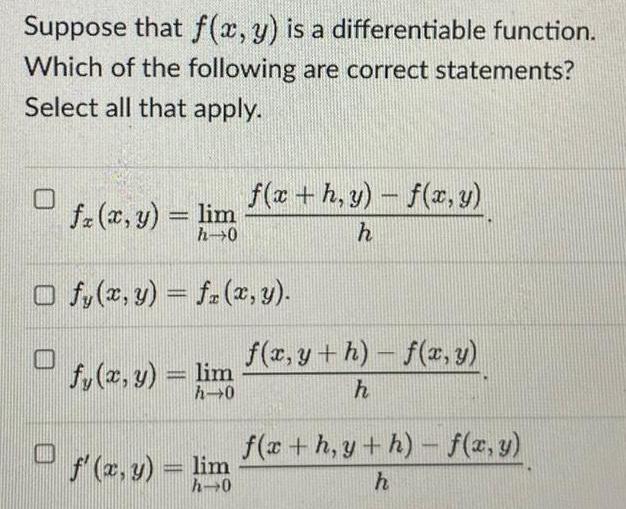
DifferentiationSuppose that f x y is a differentiable function Which of the following are correct statements Select all that apply fx x y lim h 0 O O fy x y f x y fy x y lim h 0 f x h y f x y h f x y lim f x y h f x y h f x h y h f x y h

Calculus
Application of derivativesSuppose z 1 2x 3y is an equation for the tangent plane to z f x y at 0 0 Then we also know that select all that apply Of 1 1 0 Ofz 0 0 2 Of 0 0 3 Of 0 0 1 The vector n 2 3 1 is orthogonal to the tangent plane

Calculus
Limits & Continuity25 x Find the points of discontinuity of f For each of them give the number Cat which the discontinuity occurs and the values of both one sided limits Enter INF for oo INF for DNE for does not exist CND for cannot determine If you find more than one point of discontinuity enter them in order from smallest to largest If there are answer boxes left unfilled fill each of them with an x Point 1 C lim f x x C lim f x x CH Point 2 C lim f x I C lim f x x C Point 3 C lim f x I C lim f x
Calculus
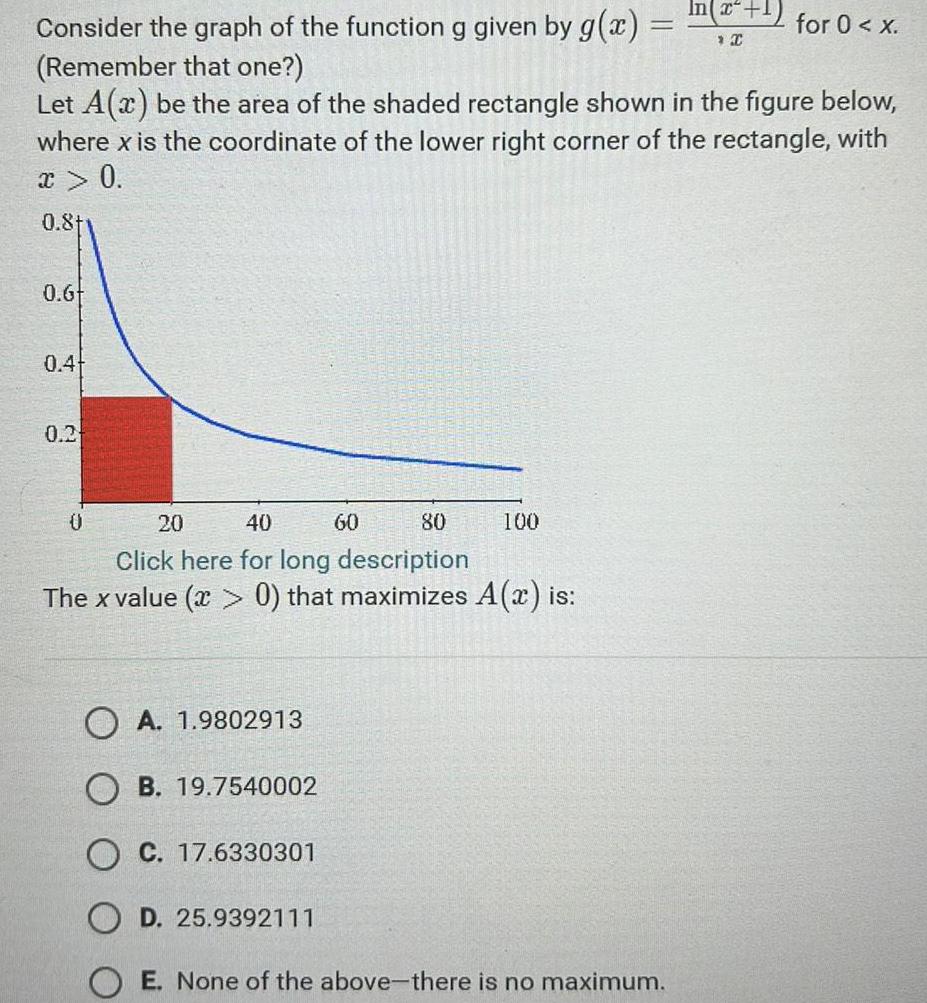
Vector CalculusConsider the graph of the function g given by g x Remember that one Let A x be the area of the shaded rectangle shown in the figure below where x is the coordinate of the lower right corner of the rectangle with x 0 0 81 0 6 0 4 0 2 20 40 80 Click here for long description The x value x 0 that maximizes A x is 0 OA 1 9802913 B 19 7540002 O C 17 6330301 D 25 9392111 60 100 In x 1 C OE None of the above there is no maximum for 0 x

Calculus
Indefinite IntegrationHint try the substitution u x O A arcsin x C OB B arcsin u C c arcsin x C O C D x arcsin x C O E 2 arcsin z C

Calculus
Limits & Continuitylim f x Find the indicated one sided limits of f and determine the continuity of f at the indicated point NOTE Type DNE if a limit does not exist You should also sketch a graph of y f x including hollow and solid circles in the appropriate places I 6 lim f x I 6 f x lim f x I 6 6 Is f continuous at x 6 YES NO 4 x x 6 5 x x 6
Calculus
Limits & ContinuityTHE RE HERE REFER MEREADERE WASSERANSE ABDGECACE CHARAC 2 If f is continuous on what can you say about its graph Er ARAPRAKINIM


Calculus
Application of derivativesda In 12x 4 dx 1 12x O A OB OC None of the above O D 12 12x 4 OE X 1 12x 4
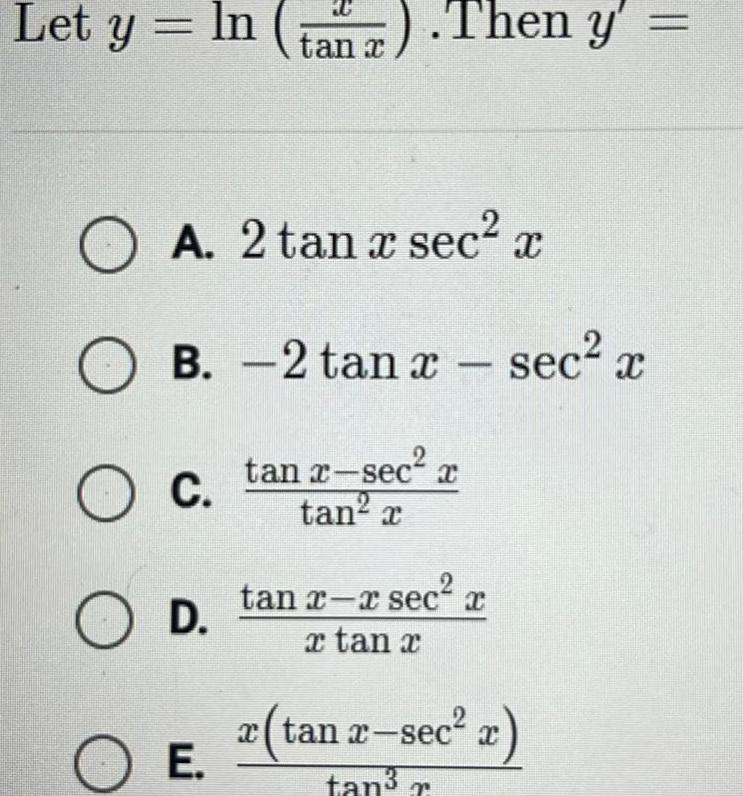
Calculus
Application of derivativesLet y In t tan a OA 2 tan x sec x O C B 2 tanx sec x tan x secr tan a O D OE Then y tan x x sec x a tan x sec x x tan x sec tan r

Calculus
Application of derivativesThe curve y x log3 x 5 has points of inflection at x OA In 3 5 B There are no points of inflection O c 10 O D D 10 In 3 OE 5

Calculus
Application of derivativesda A 2 In 2 In x 2 2 x In 2 In x 2 O B B O C In 2 2 X O D D 2 In 2 2 In x 2 X O E In 2 2 In x 2 X

Calculus
Application of derivativesLet f x 1 x if x 0 2x 1 if x 0 Evaluate f 3 and f 6 f 3 8 f 6 If you have had difficulty with these problems you should look at Sections 1 1 1 3 of y

Calculus
Application of derivativesO A 22 In 2 In x 2 B In 2 In x 2 In 2 2 I OB O C OD In 2 2 ln x 2 O E In 2 2 In x 2

Calculus
DifferentiationConsider the function f x 20x e on the domain U On its domain the curve y f x A attains its maximum value at x value at x 0 OB attains its maximum value at x minimum value O C attains its maximum value at x value at x 0 OD attains its maximum value at x value OE attains its maximum value at x minimum value and attains its minimum and does not have a and attains its minimum and does have a minimum and does not have a

Calculus
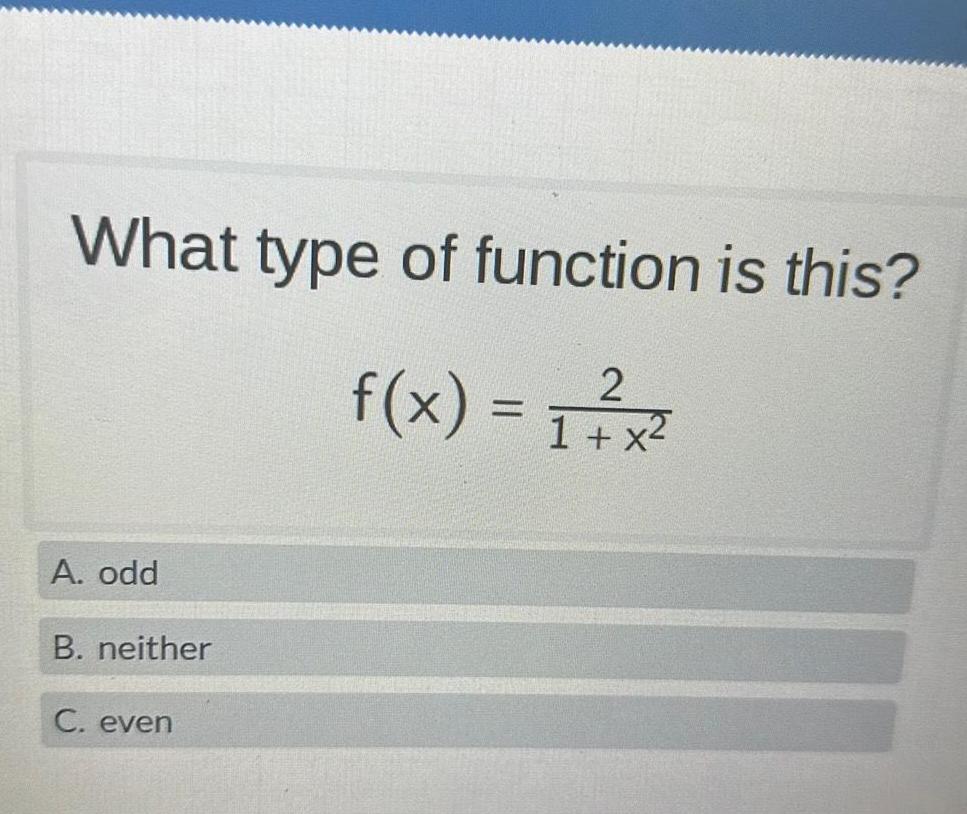
Vector CalculusFind the horizontal and vertical asymptotes for the function f x x 4 x 2 x 3 Vertical asymptotes x 2 x Horizontal asymptote y Enter


Calculus
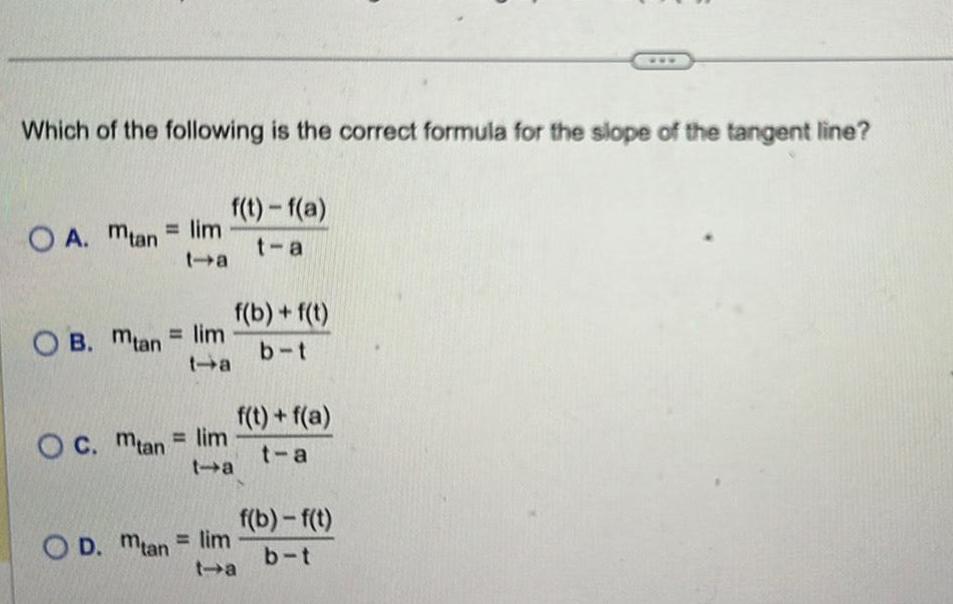
DifferentiationWhich of the following is the correct formula for the slope of the tangent line OA Mtan lim OB Mtan lim f t f a t a OD Mtan lim f b f t b t OC Mtan lim t a f t f a t a f b f t b t
Calculus
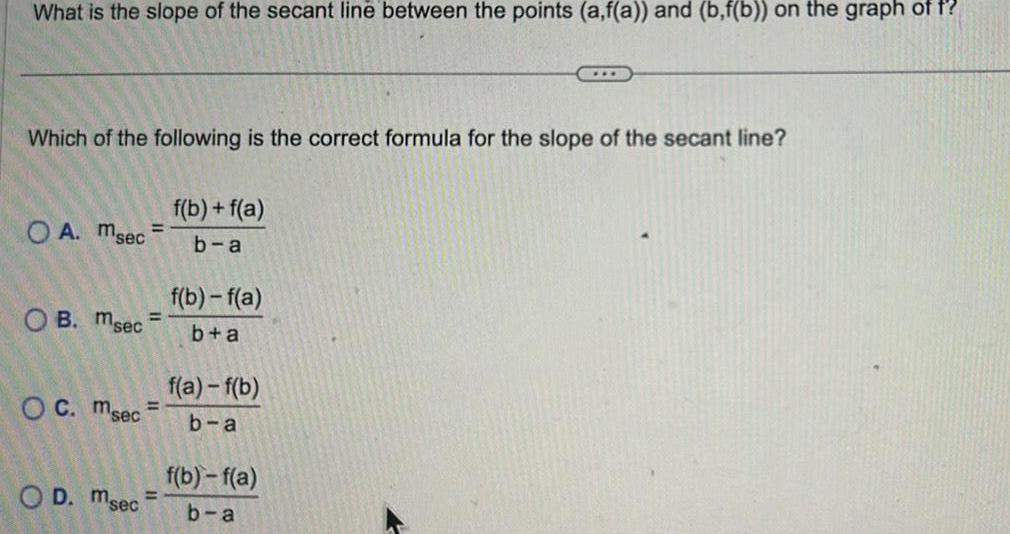
DifferentiationWhat is the slope of the secant line between the points a f a and b f b on the graph of f Which of the following is the correct formula for the slope of the secant line OA Msec OB Msec OC Msec OD Msec f b f a b a f b f a b a f a f b b a f b f a b a
Calculus
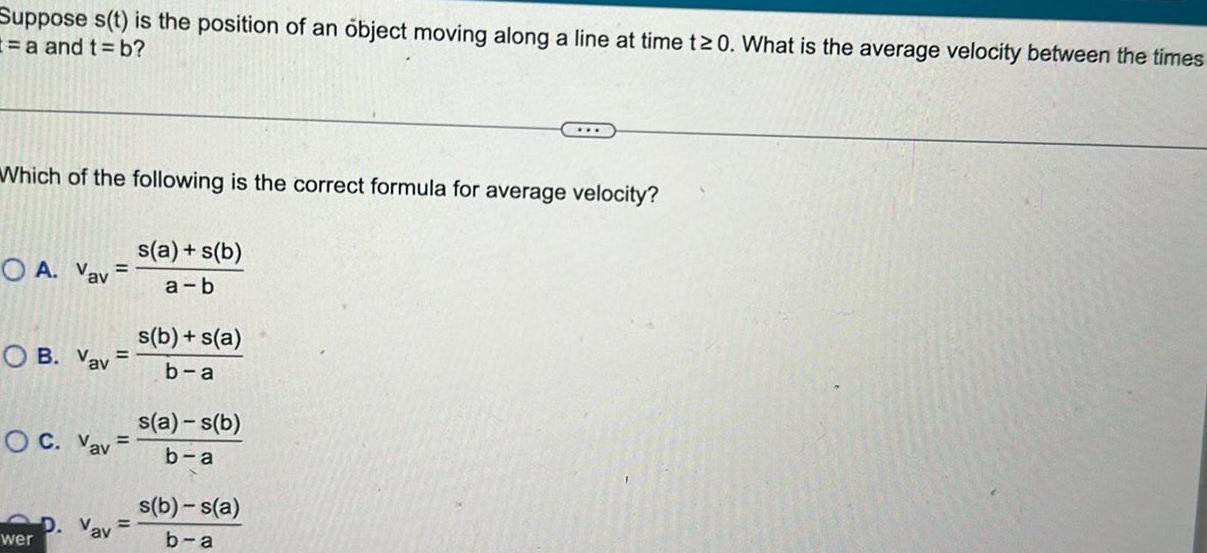
Definite IntegralsSuppose s t is the position of an object moving along a line at time t 0 What is the average velocity between the times t a and t b Which of the following is the correct formula for average velocity OA Vav OB Vav OC Vav wer Vav s a s b a b s b s a b a s a s b b a s b s a b a

Calculus
Application of derivatives3000 young trout are introduced into a large fish pond The number of trout still alive after tyears is modeled by the formula N t 3000 0 9 What is the rate of population decrease when the number of trout in the pond reaches 2000 A Approximately 520 trout per year B Approximately 211 trout per year C Approximately 2000 trout per year D Approximately 494 trout per year OE None of the above

Calculus
Application of derivativesFind intervals where this function is increasing decreasing or constant 3 f x x 3 10 5 1 1 2 3 4 5 6 7 5 10 A increasing from to 3 and decreasing from 3 to o B decreasing from to 3 and decreasing from 3 to o C increasing from t3 and decreasing from 3 to co
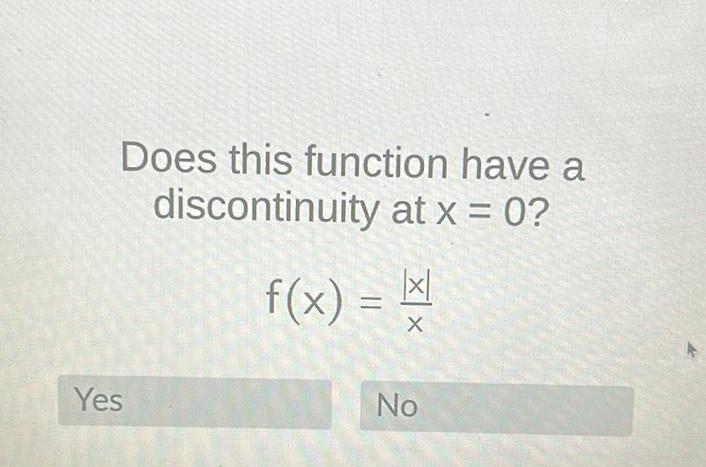

Calculus
Vector CalculusA Find the area of R x B Find the value of h such that the vertical line x h divides the region R into tw regions of equal area C Find the volume of the solid generated when R is revolved about the x axis
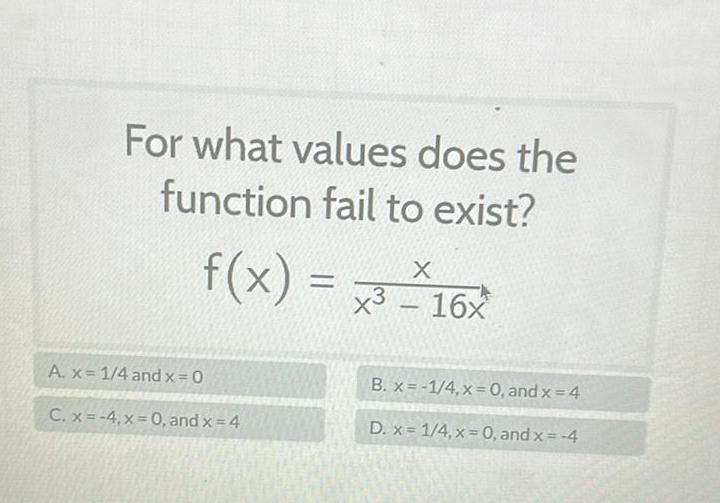
Calculus
Definite IntegralsFor what values does the function fail to exist f x A x 1 4 and x 0 C x 4 x 0 and x 4 X x 16x B x 1 4 x 0 and x 4 D x 1 4 x 0 and x 4
Calculus
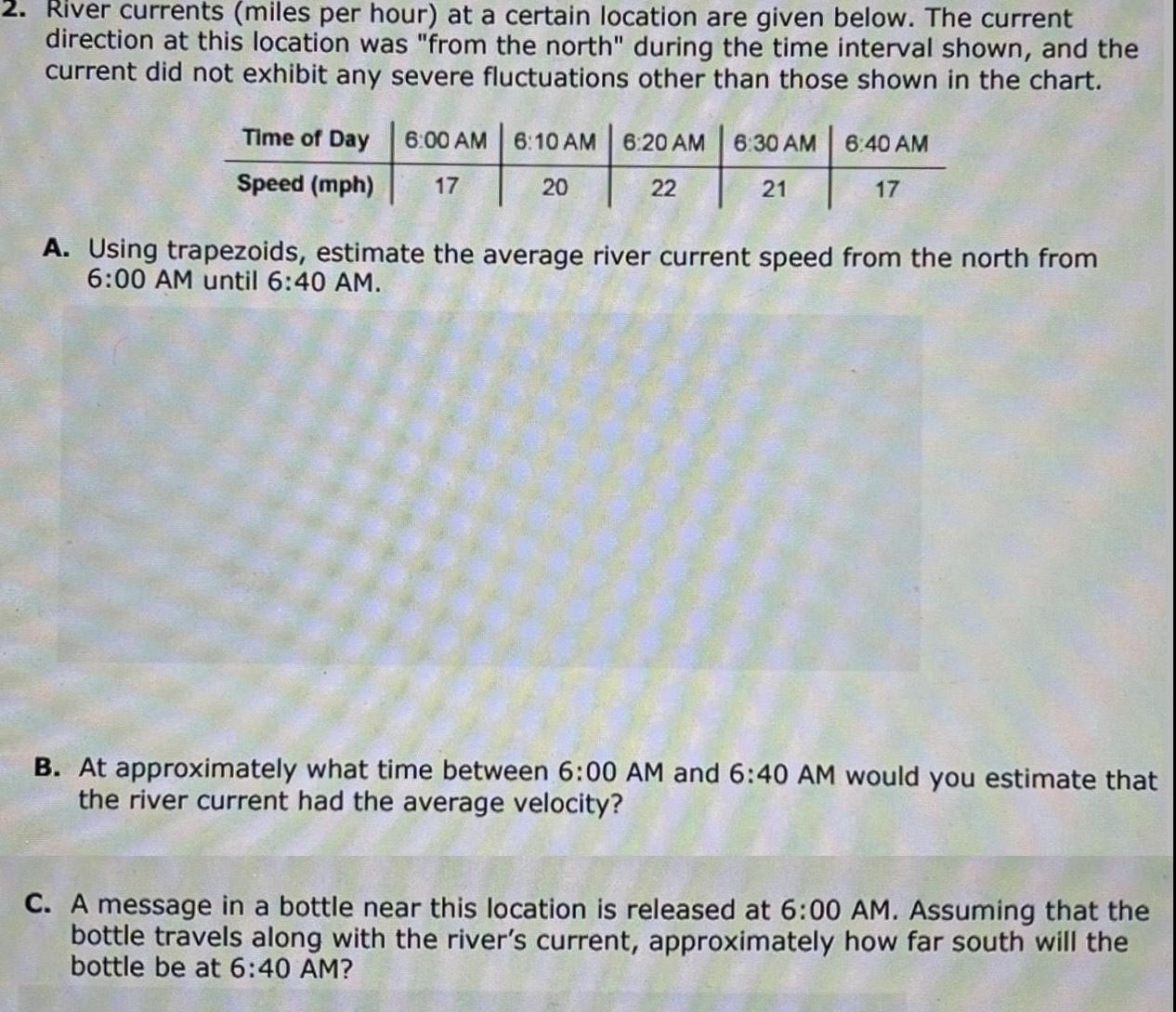
Vector Calculus2 River currents miles per hour at a certain location are given below The current direction at this location was from the north during the time interval shown and the current did not exhibit any severe fluctuations other than those shown in the chart Time of Day 6 00 AM 6 10 AM 6 20 AM Speed mph 17 20 22 6 30 AM 21 6 40 AM 17 A Using trapezoids estimate the average river current speed from the north from 6 00 AM until 6 40 AM B At approximately what time between 6 00 AM and 6 40 AM would you estimate that the river current had the average velocity C A message in a bottle near this location is released at 6 00 AM Assuming that the bottle travels along with the river s current approximately how far south will the bottle be at 6 40 AM
Calculus
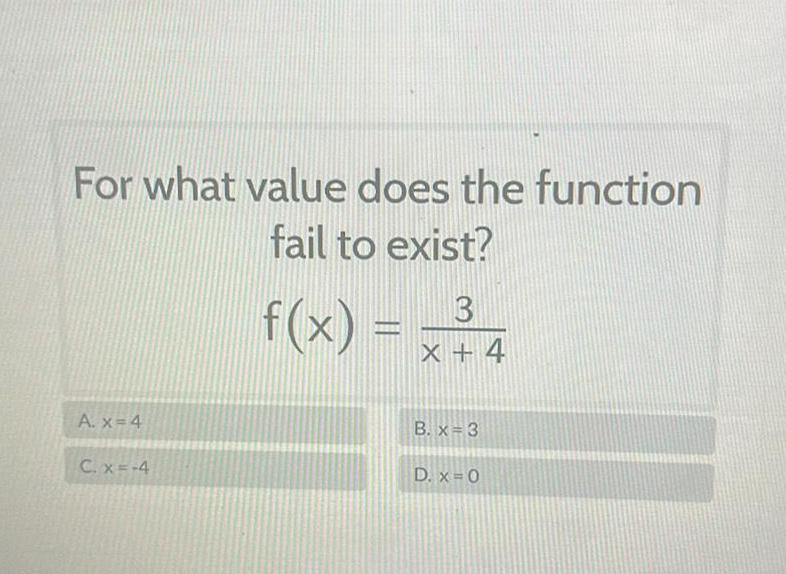
Differential equationsFor what value does the function fail to exist f x A x 4 C x 4 3 X 4 B x 3 D x 0

Calculus
DifferentiationThe position of a particle moving in a straight line is given by s t et cos 5t for t 0 where tis in seconds If the particle changes direction at time T seconds then Tmust satisfy the equation A 5e t sin 5t 0 OB tan 57 3 OC cos 5T 0 O D 5T arctan 5 E cot 57 5
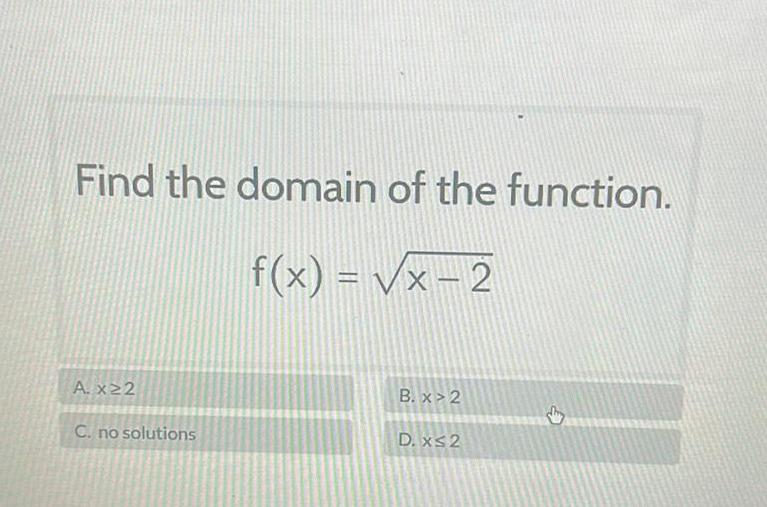
Calculus
Application of derivativesFind the domain of the function f x x 2 A x22 C no solutions B x 2 D x 2
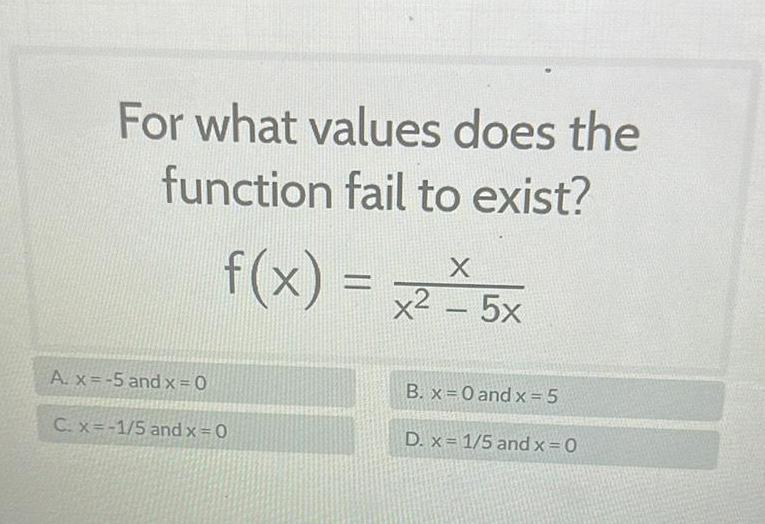
Calculus
Differential equationsFor what values does the function fail to exist A x 5 and x 0 f x C x 1 5 and x 0 X x2 5x B x 0 and x 5 D x 1 5 and x 0
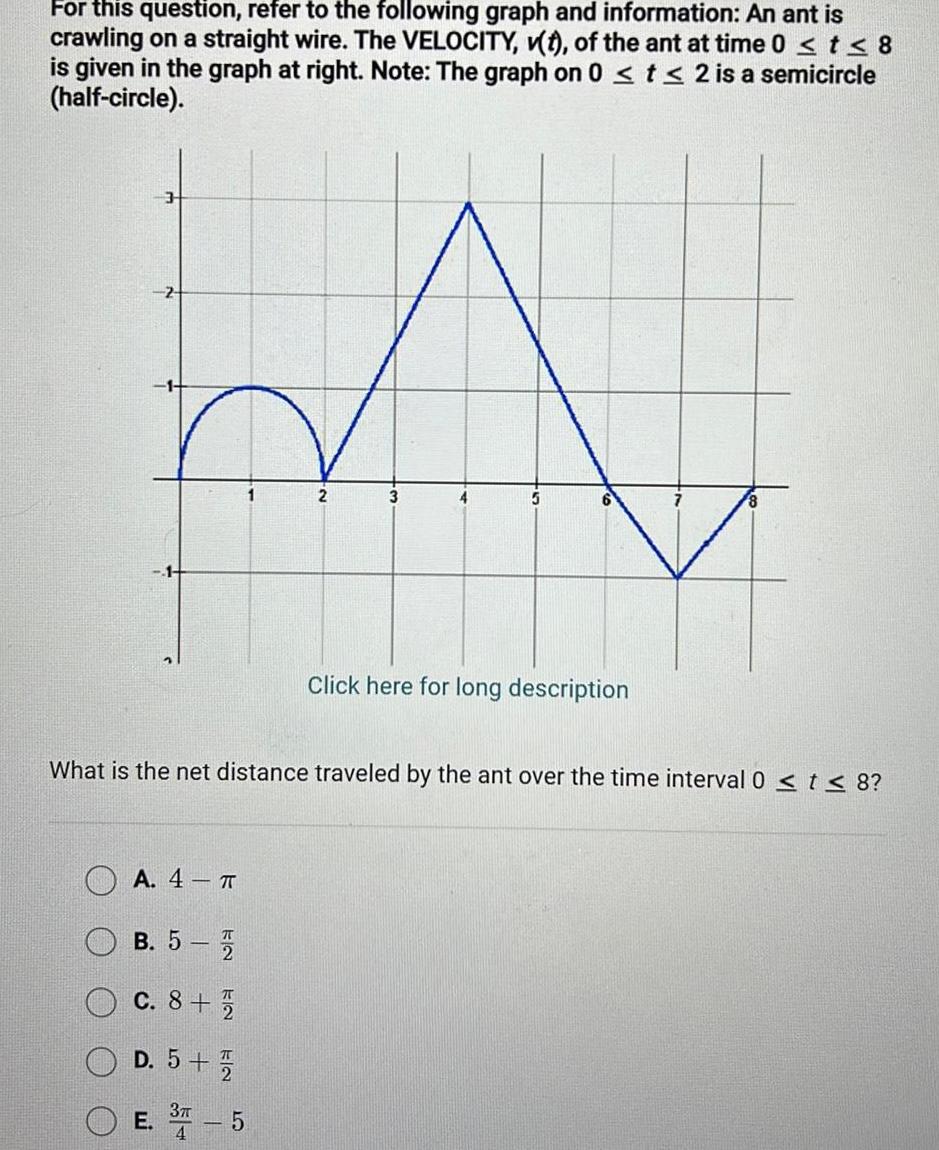
Calculus
Definite IntegralsFor this question refer to the following graph and information An ant is crawling on a straight wire The VELOCITY v t of the ant at time 0 t 8 is given in the graph at right Note The graph on 0 t 2 is a semicircle half circle A 4 2 B 5 7 C 8 1 2 D 5 1 2 E 37 5 3 What is the net distance traveled by the ant over the time interval 0 t 8 Click here for long description

Calculus
Application of derivativesda O A O O B O c x 3 x ln 2 1 3x O D None of the above 1 O E x In 2

Calculus
Definite IntegralsFind a definite integral for which 1 4 4 k 4 4 4 is a right rectangle approximation 5 O A x dx B x 4x dx c x 4x dx OD 21 6 5 O E x dx

Calculus
Application of derivativesdx O A OB B e3r 1 e z 1 2 2 1 e 1 x 1 2 O c 1 1 2 O D 3 D 2 1 2 E 3 1 x 1 2