Differentiation Questions and Answers

High School Calculus
Differentiation8 Wayne wants to plant a triangular garden in his backyard and he will enclose the garden with 204 feet of fencing a Give two possible sets of side lengths for the garden one making an acute triangle and one making an obtuse triangle b Find the semiperimeter of each garden c Find the area of each garden d Wayne decides that he will have one side length of the garden be 50 feet If another side length is x write an expression for the 3rd side length e Write an equation in terms of x for the area of the garden f Use technology to find the lengths of the sides of the garden that give the maximum area and state the maximum area
High School Calculus
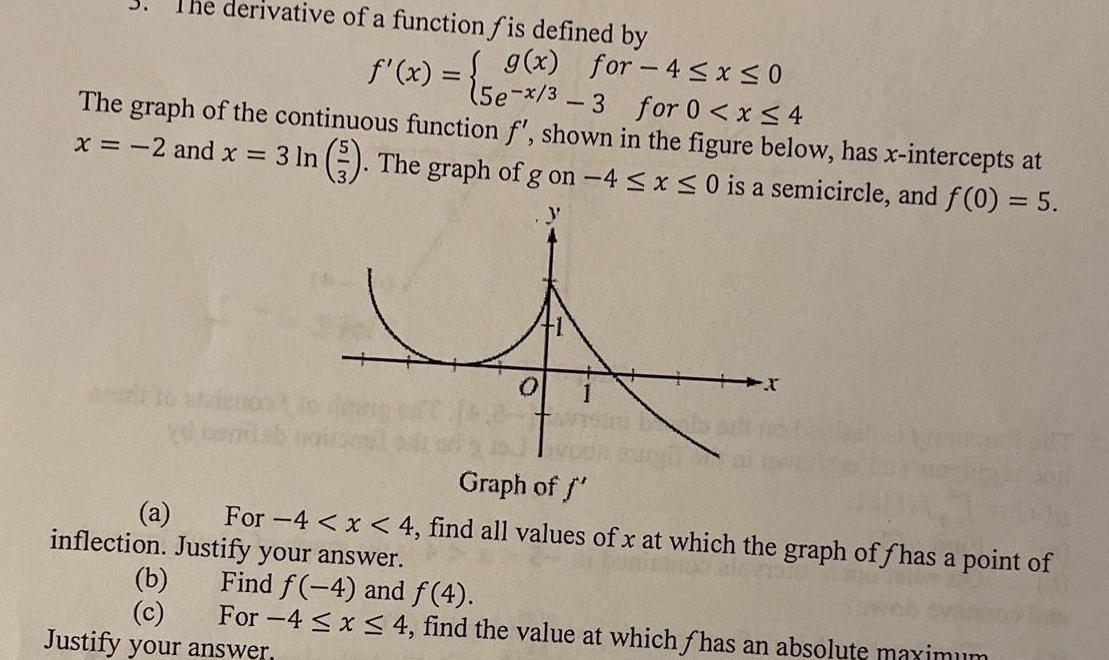
DifferentiationThe derivative of a function fis defined by f x g x for 4 x 0 15e x 3 3 for 0 x 4 The graph of the continuous function f shown in the figure below has x intercepts at 3 In 3 The graph of g on 4 x 0 is a semicircle and f 0 5 x 2 and x 0 Graph of f For 4 x 4 find all values of x at which the graph of fhas a point of inflection Justify your answer a b c Find f 4 and f 4 For 4x4 find the value at which fhas an absolute maximum Justify your answer
High School Calculus
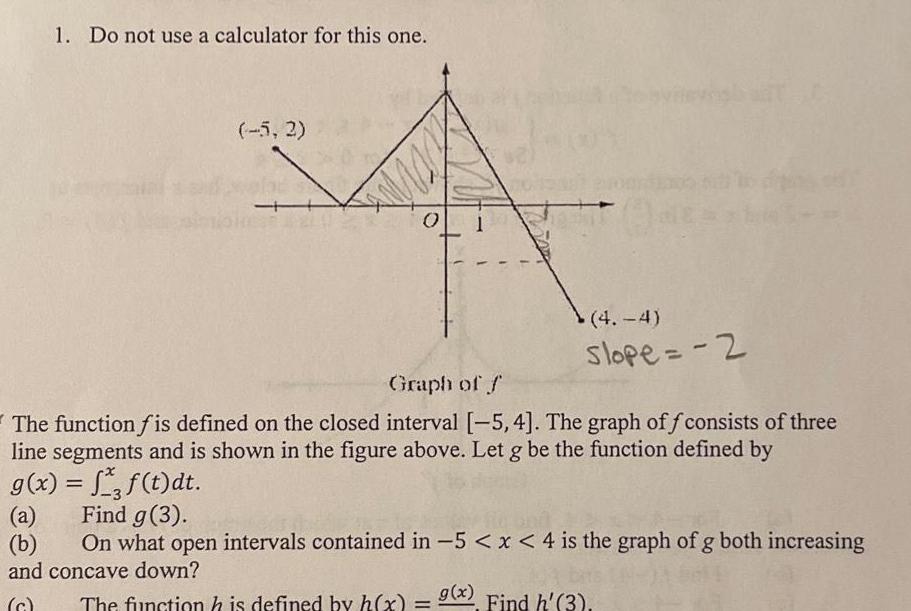
Differentiation1 Do not use a calculator for this one 5 2 Graph of f 4 4 slope 2 The function fis defined on the closed interval 5 4 The graph of consists of three line segments and is shown in the figure above Let g be the function defined by g x a b f t dt Find g 3 On what open intervals contained in 5 x 4 is the graph of g both increasing and concave down g x The function is defined by h x 9 Find h 3
High School Calculus
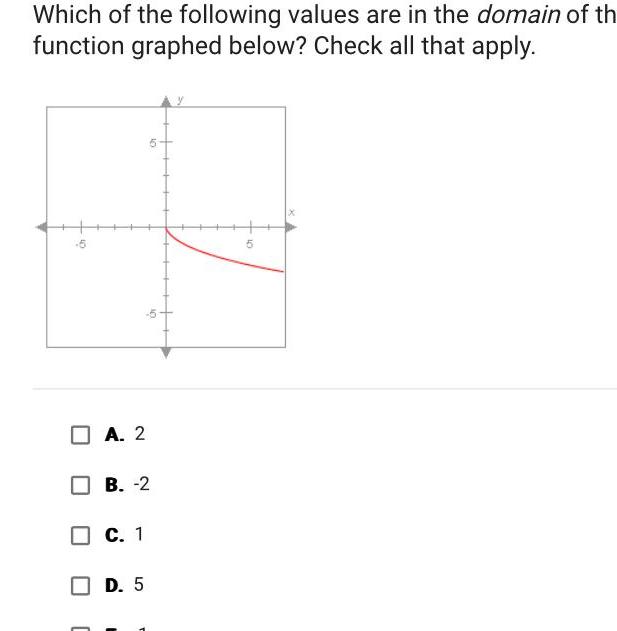
DifferentiationWhich of the following values are in the domain of th function graphed below Check all that apply A 2 B 2 C 1 D 5 5 5

High School Calculus
DifferentiationConvert each radian measure to degree measure Do not use a calculator a 11 12 b 7 4

High School Calculus
Differentiation5 Solve over the interval 0 0 2 2cos tane tane 6 Find ALL solutions to this equation in radians 2cos2x1 0
High School Calculus
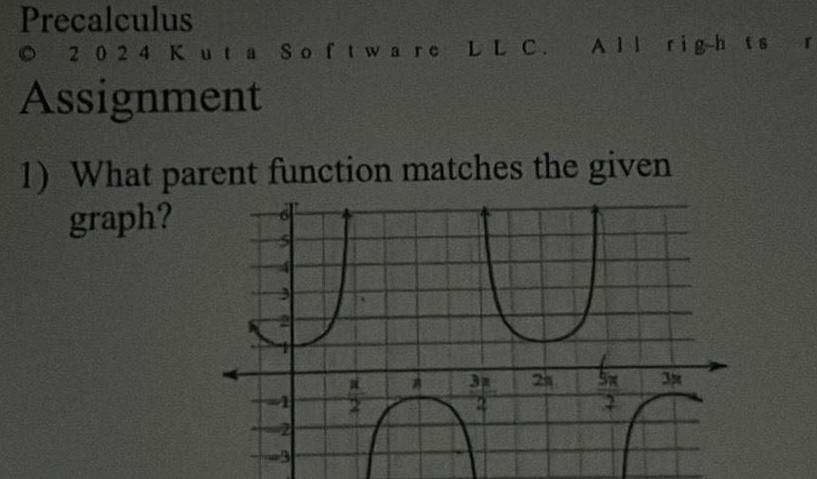
DifferentiationPrecalculus 2024 Kuta Software LLC All rights Assignment 1 What parent function matches the given graph 27 3M T
High School Calculus
DifferentiationIn ANOP p 870 cm m N 127 and m 0 30 Find the length of n to the nearest centimeter

High School Calculus
DifferentiationAnswer the following questions Be sure to show all work and justify when necessary 1 Given the function f x 4x3 3x2 17 over what intervals is f x decreasing
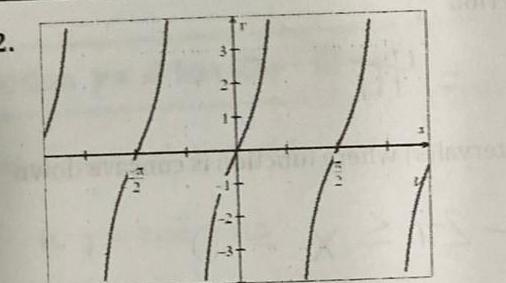
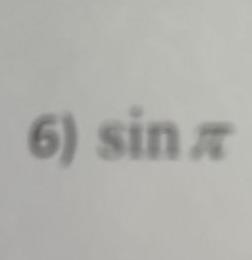

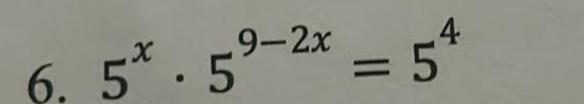
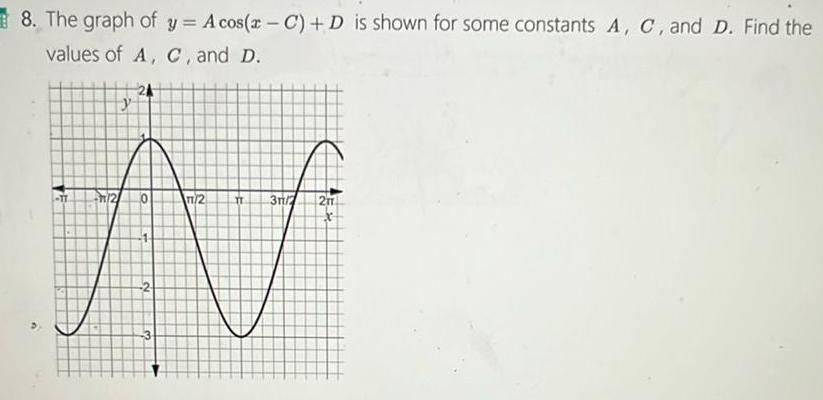
High School Calculus
Differentiation8 The graph of y A cos x C D is shown for some constants A C and D Find the values of A C and D 24 y TT 2 0 11 2 TT 31 2 211 M 2 3 5

High School Calculus
Differentiation1 A cylindrical soup can of volume 500 cm is to be constructed The material for the top costs 0 4e cm while the material for the bottom and the sides costs 0 2e cm Find the dimensions correct to one decimal place that will minimize the cost for producing the can Justify your answer Cylinder formulae Vrh and Total SA 2arh 2r 8 marks


High School Calculus
DifferentiationUse the table below with selected values of the twice differentlable function k Reach each explanation and decide whether you would apply IVT EVT or MVT X K x I 5 2 2 3 4 4 4 applies 5 3 6 2 7 O 6 Since k is differentiable it is also continuous Since k 6 2 and k 7 0 and since I is between 2 and O It follows by that K c 1 for some c between 6 and 7 7 Since k is differentiable and therefore also continuous and since 3 k 2 6 it follows by that k c 6 for some 3 2 c in the interval 2 3 8 There must be a minimum value for k at some r in 1 7 because k is differentiable and therefore also continuous Hence the q There must be some value a in 2 6 for which k a 0 because k 2 k 6 and since k is differentiable the applies 10 Since k is differentiable the guarantees some a in 4 5 for which k a 4 and also some b in 5 6 for which k b 1 Then since k is differentiable and therefore also continuous it follows by the applied to k that k c 0 for therefore in 46


High School Calculus
Differentiation3 Water is dripping through the bottom of a conical coffee filter 4 inches across and 6 inches deep Given that the filter loses half a cubic inch of coffee per minute how fast is the level of the liquid dropping when it is 3 inches deep

High School Calculus
Differentiation2 A 19 foot ladder is being pushed up a building at a constant rate of 2 feet per minute How fast is the base of the ladder moving toward the building when the base of the ladder is q feet from the building

High School Calculus
Differentiation1 A stone dropped into a sill pond sends out a circular ripple whose radius increases at a constant rate of 4 ft s How rapidly is the area enclosed by the ripple increasing when the radius is 2 feet
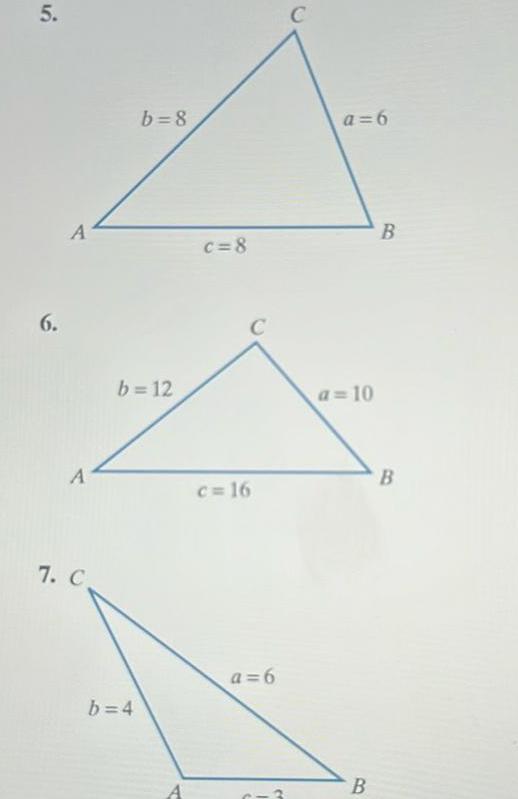

High School Calculus
Differentiationind the domain and range of the relation Use interval notation where appropriate 5 4 3 2 1 57 4 3 2 O 1 0 0 2 3 4 5 2 3 4 5 K

High School Calculus
DifferentiationLet f x x ax 2a x a such that f x has a point of inflection located at x 2 What is the value of A 3

High School Calculus
Differentiation2 Calculator active The rate of R t of a certain item produced in a factory is given by R t 4000 48 t 3 4 1 3 3 where t is the number of hours since the beginning of the workda 8 00 am At what time is the rate of production increasing most rapidly d 1 00 pm a 8 00 am b 10 00 am c 11 00 am

High School Calculus
DifferentiationT and a 9 Geraldine and Miranda were asked to write an equation of a cotangent function with an x intercept at x vertical asymptote at x Geraldine said there was more than one correct answer Miranda said there was only one correct answer Who is correct Explain your answer 4
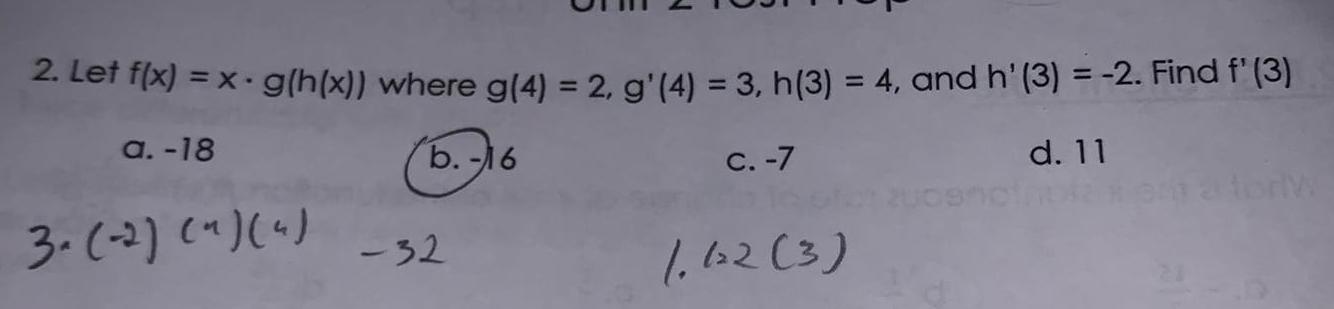
High School Calculus
Differentiation2 Let f x x g h x where g 4 2 g 4 3 h 3 4 and h 3 2 Find f 3 a 18 b 16 c 7 d 11 3 2 4 32 1 12 3

High School Calculus
Differentiation3 If f is a differentiable function such that f 2 5 and f 2 7 which of the following statements could be false a lim f x 5 b lim f x lim f x C lim f x 5 X 2 X 2 7 d lim f x 7 h 0

High School Calculus
Differentiationorodeoxyglucose is a derivative of glucose that contains the radionuclide fluorine 18 A patient is given a sample of this material containing 245 MbQ of fluorine 18 a megabecquerel is a unit of radioactivity The patient then undergoes a PET scan to detect areas of metabolic activity indicative of cancer After 138 minutes one third of the original dose remains in the body Use a function of the form Q t Qoe kt to model the radioactivity level of fluorine at a time t minutes after the initial dose Give the value of k to three decimal places Do not round intermediate calculations Your Answer
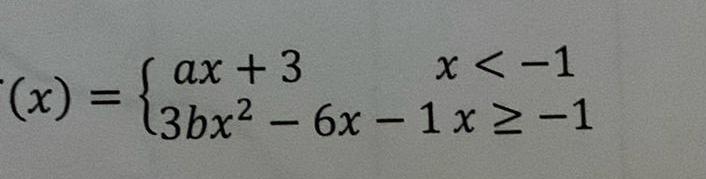

High School Calculus
DifferentiationFind the average rate of change on the given interval 1 s t t t 4 15

High School Calculus
Differentiation13 A landowner says his property is triangular with one side 500 m long and another side 350 m long The opposite angle to the 350 m side measures 20 Determine two possible lengths of the third side to the nearest metre 4 marks

High School Calculus
DifferentiationA 2010 estimate of the population of Mexico is 102 million people with a projected growth rate of 0 92 per year At this rate the population P t in millions can be approximated by P9t 102 1 0092 t where t is the time in years since 2010 Find the population in the 2 017 Round your answer to the nearest million Your Answer

High School Calculus
DifferentiationUse a calculator to evaluate the function at the indicated value of x Round your result to three decimal plac Value 67 f Function f x 1 2x X 7

High School Calculus
Differentiationof a race round all answers to the nearest tenth 10x 10 F t c Write a function representing the inverse of f x and interpret its meaning of x and y in context to the problem All work must be completed by hand and justified mathematically 6 points A Paragraph BI UV V Times New 12pt 7 15 All work must completed by hand and justified mathematically Type your work in using the time in seconds after the start V Fa 30 C

High School Calculus
DifferentiationUse the table to find the value of the derivatives of each function IL X 7 h x 5f x g x f x 5 f x 3 g x 2 g x 3
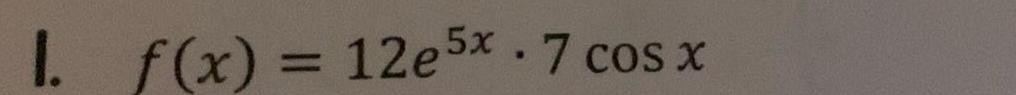

High School Calculus
DifferentiationNo Calculator No Calculator No Calculator No Calculator No Calculator 5 10 5 800 A g only C f g and h 10 The graphs of the derivatives of the functions f g and h are shown above Which of the functions f g or h have a relative maximum on the open interval a x b yux B fonly D and g only
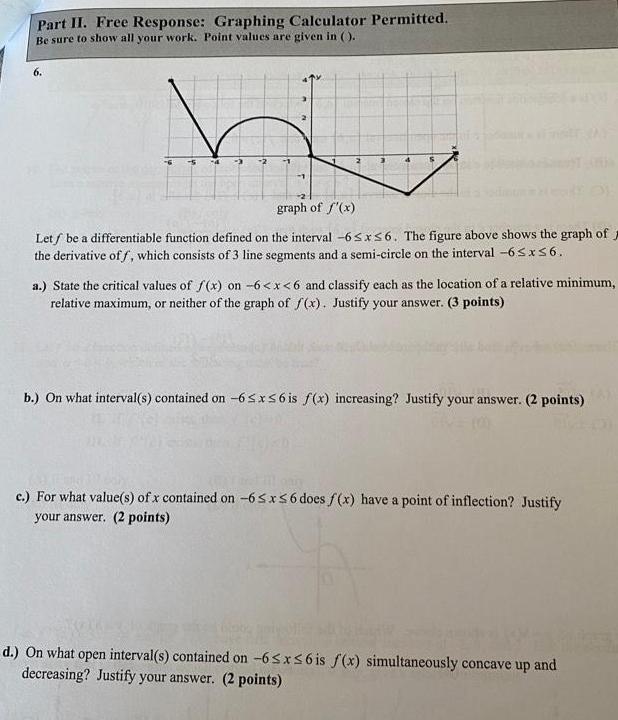
High School Calculus
DifferentiationPart II Free Response Graphing Calculator Permitted Be sure to show all your work Point values are given in 6 h graph of f x 5 Let be a differentiable function defined on the interval 6 x 6 The figure above shows the graph of the derivative off which consists of 3 line segments and a semi circle on the interval 6 x 6 a State the critical values of f x on 6 x 6 and classify each as the location of a relative minimum relative maximum or neither of the graph of f x Justify your answer 3 points b On what interval s contained on 6 x 6 is f x increasing Justify your answer 2 points c For what value s of x contained on 6 x 6 does f x have a point of inflection Justify your answer 2 points d On what open interval s contained on 6 x 6 is f x simultaneously concave up and decreasing Justify your answer 2 points


High School Calculus
Differentiation16 Use the table to the right to find the equation of the tangent line of the function r x 0 5f x 3g x at x 2 X f x f x g x 2 12 2 4 4 10 1 15
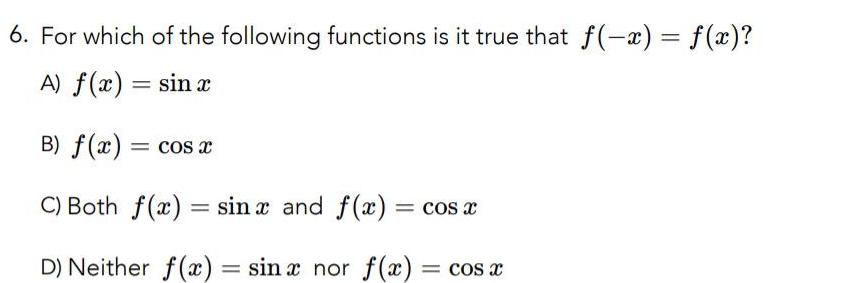
High School Calculus
Differentiation6 For which of the following functions is it true that f x f x A f x B f x cos x C Both f x sina and f x cos x D Neither f x sinx nor f x sin x

High School Calculus
DifferentiationPerform the operation s and write the result in standard form Simplify your answer completely 4 3i 4 3i
1