2D Geometry Questions and Answers
Geometry
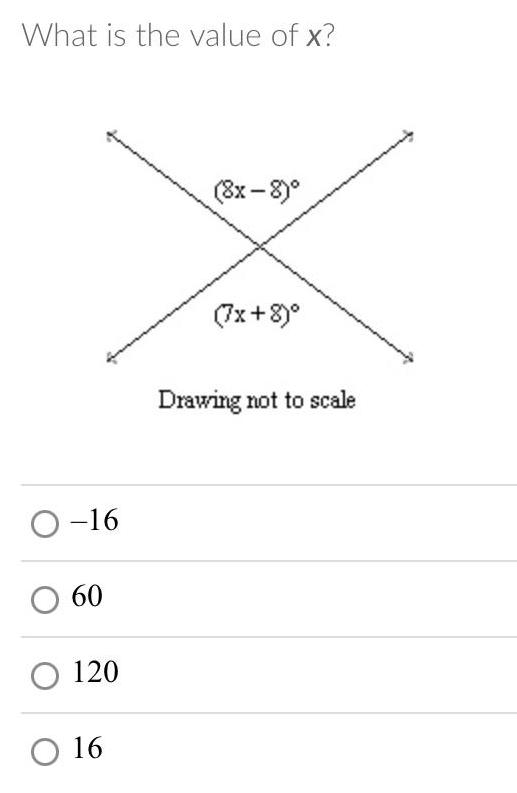
2D GeometryWhat is the value of x?
O-16
O 60
O 120
O 16
(8x-8)°
(7x+8)°
Drawing not to scale


Geometry
2D GeometryThe folding chair has different settings that change the angles formed by its parts. Suppose m/2 is 34 and m3 is 76. Find m21. The diagram is not to scale.
O 100
O 110
O 130
O 120

Geometry
2D GeometryEach tie on the railroad tracks is perpendicular to both of the tracks.
What is the relationship between the two tracks? Justify your answer
.The two tracks are parallel by the Converse of the PerpendicularTransversal Theorem.
The two tracks are perpendicular by the Perpendicular TransversalTheorem.
The two tracks are perpendicular by the definition of complementary angles.
The two tracks are parallel by the Same-Side Interior Angles Postulate.

Geometry
2D GeometryIf EG=25, and point F is 2/5 of the way between E and G, find the value FG. The drawing is not to scale.
E F G
O 12.5
O 10
O 15
O 20

Geometry
2D GeometryWhat are the names of the segments in the figure?
ZA B C
O The three segments are AB, BC, and AC
O The two segments are AB and BC
O The three segments are AR BC, and BA-
O The three segments are AB, CA, and AC

Geometry
2D GeometryFind the perimeter of the rectangle. The drawing is not to scale.
29 ft 95 feet
O 190 feet
O 161 feet
O 124 feet
O 66 ft

Geometry
2D GeometryIf EF = 6 and EG = 21, find the value of FG. The drawing is not to scale.
E F
O 14
O 15
O 17
0 6


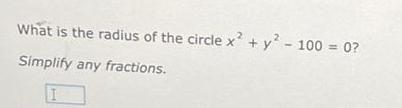


Geometry
2D GeometryFind the x-intercepts of the parabola with vertex (1,-9) and y-intercept at (0,-6).
A. (-1,0), (3,0)
B. (-0.73,0), (2.73,0)
C. (-1.48,0), (2.48,0)
D. (-4.67,0), (1.67,0)
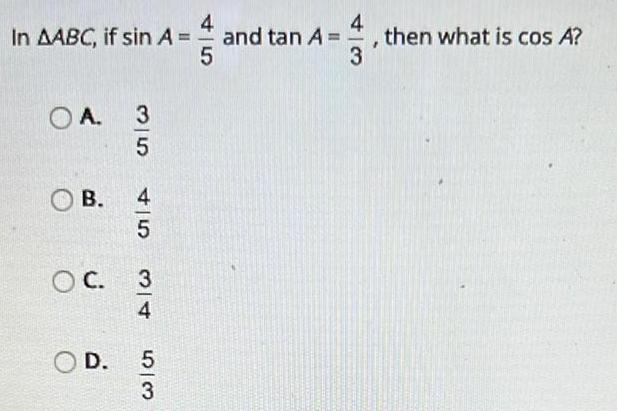
Geometry
2D GeometryIn ΔABC, if sin A =4/5 and tan A=4/3, then what is cos A?
A. 3/5
B.4/5
C.3/4
D.5/3

Geometry
2D GeometryWhat is the radius of the circle x² + y² - y - 30 = 0?
Write your answer in simplified, rationalized form.
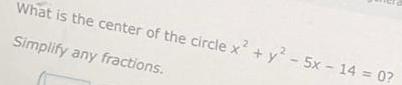

Geometry
2D GeometryRadius OA of circle O has a slope of 2.5. What is the slope of the line tangent to circle O at point A?
A. 0.8
B. 2.5
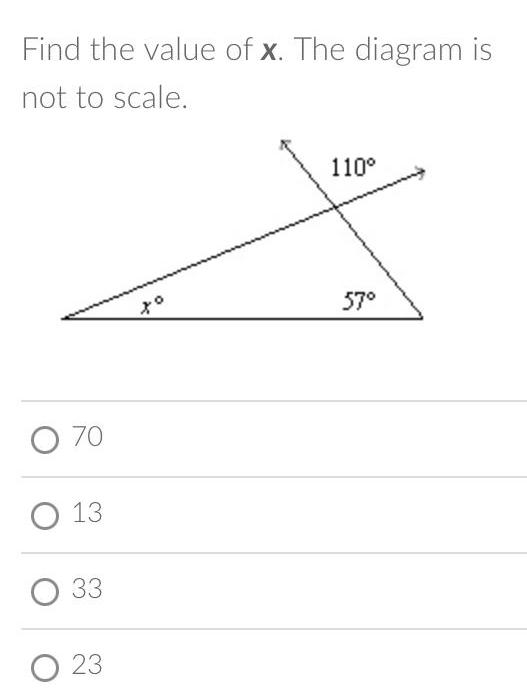
C. -0.4
D. -5

Geometry
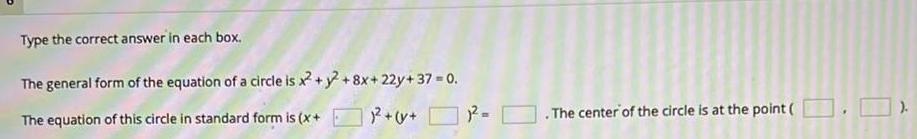
2D GeometryType the correct answer in each box.
The general form of the equation of a circle is x2 + y2 +8x+22y+37= 0.
The equation of this circle in standard form is (x+ )² + (y+ )² = The center of the circle is at the point
![35 Quadrilateral PQRS has vertices P(-2,3), Q(3,8), R(4,1), and S(-1,-4).
Prove that PQRS is a rhombus.
[The use of the set of axes on the next page is optional.]
Question 35 continued.
Prove that PQRS is not a square.
[The use of the set of axes below is optional.]](https://media.kunduz.com/media/sug-question/raw/81450667-1659790996.3345995.jpeg?w=256)
Geometry
2D Geometry35 Quadrilateral PQRS has vertices P(-2,3), Q(3,8), R(4,1), and S(-1,-4).
Prove that PQRS is a rhombus.
[The use of the set of axes on the next page is optional.]
Question 35 continued.
Prove that PQRS is not a square.
[The use of the set of axes below is optional.]
Geometry
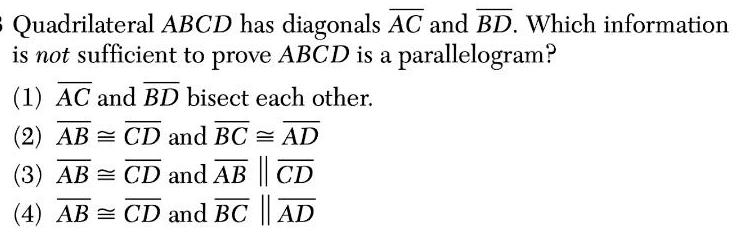
2D GeometryQuadrilateral ABCD has diagonals AC and BD. Which information is not sufficient to prove ABCD is a parallelogram?
(1) AC and BD bisect each other.
(2) AB CD and BC = AD
(3) AB = CD and AB || CD
(4) AB = CD and BC|| AD

Geometry
2D GeometrySuppose a circle is divided into congruent slices. Match each number of slices with the resulting central angle measure of each slice.
2
3
4
5
6
8

Geometry
2D GeometryIn a right trapezoid ABCD, <A = <D = 90deg, AB <CD, we know: AB = 9cm, CD = 12cm and AD = 4cm. Find the distance from B to AC and the tangent of the acute angle of the trapezoid.
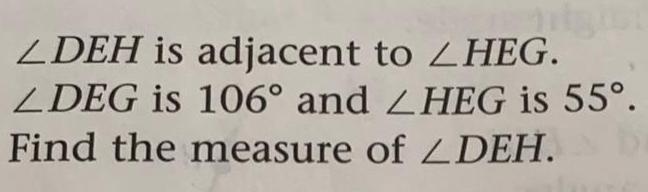
Geometry
2D Geometry∠DEH is adjacent to ∠HEG. ∠DEG is 106° and ∠HEG is 55°. Find the measure of ∠DEH.
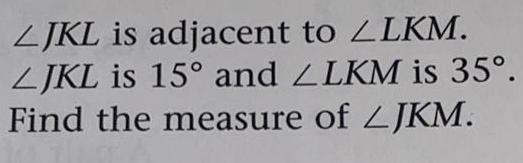
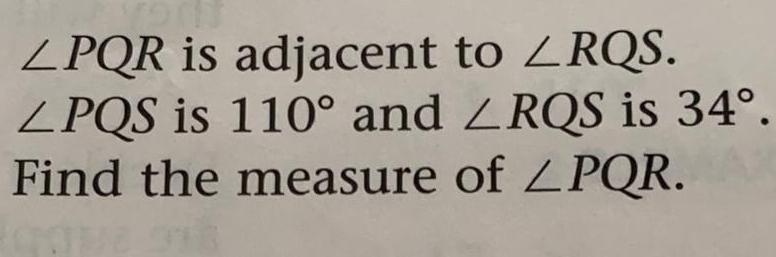
Geometry
2D Geometry∠PQR is adjacent to ∠RQS. ∠PQS is 110° and ∠RQS is 34°. Find the measure of ∠PQR.


Geometry
2D GeometryConsider a segment AB and two points O and O' on the same side of AB. Prove that the image of AB after two successive half-turns, first about O and then about O' is parallel to AB. Assume Euclidean.

Geometry
2D Geometry26. Two polygons are similar. The perimeter of the smaller
polygon is 66 m and ratio of their corresponding side
lengths is 3/4. Find the perimeter of the larger polygon.
(A) 33 m
(B) 49.5 m
(C) 132 m
(D) 88 m
![Solve for x.
2x³ = 18x
x = [?], [ ], [ ]](https://media.kunduz.com/media/sug-question/raw/80434922-1659731065.8852458.jpeg?w=256)

Geometry
2D GeometryDetermine if each statement must be true, could possibly be true, or definitely can't be true. Explain
or show your reasoning.
1. Congruent figures are similar.
2. Similar figures are congruent.

Geometry
2D GeometryA six-sided polygon is called
A. a nonagon
B. a quadrilateral
C. a heptagon
D. a hexagon
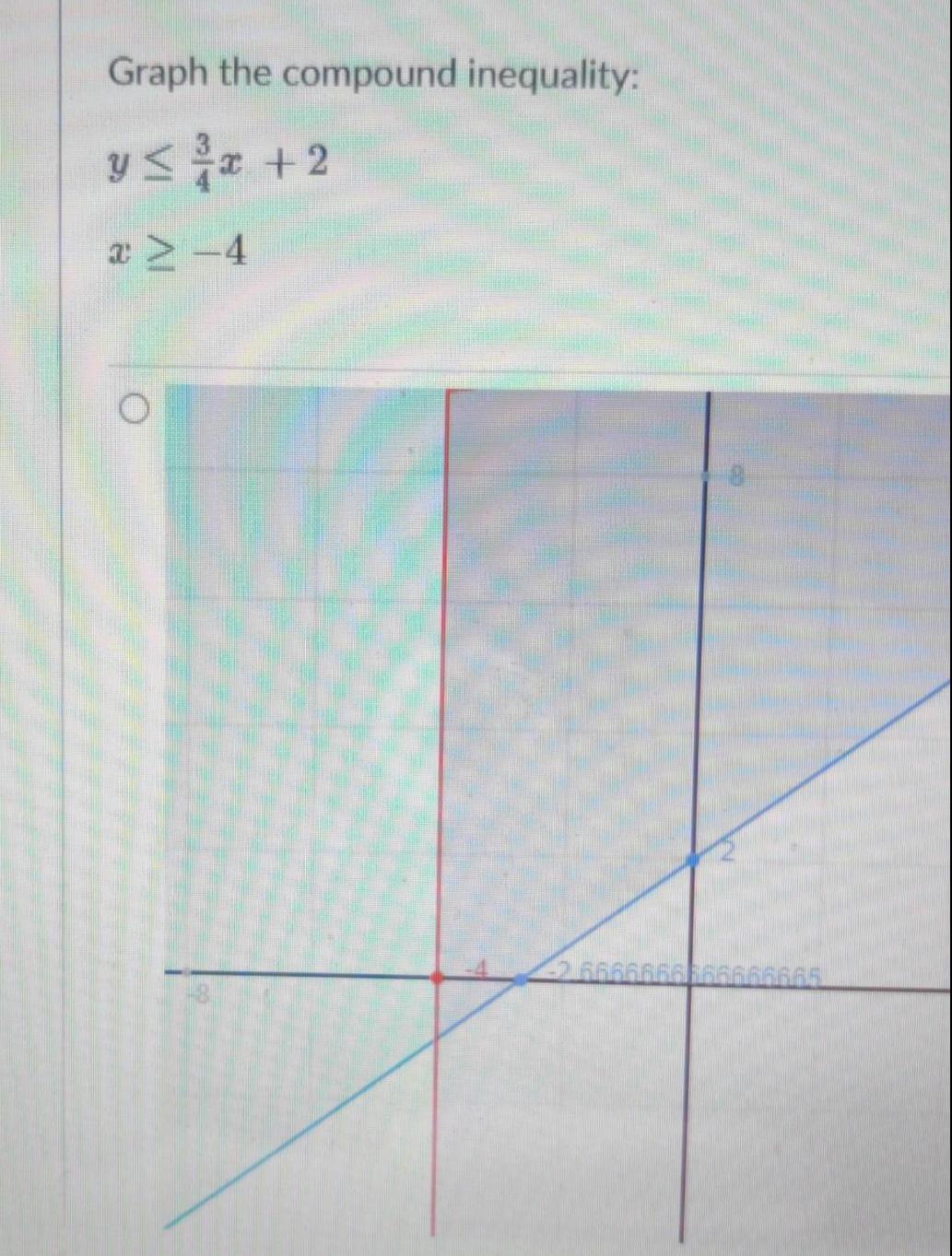
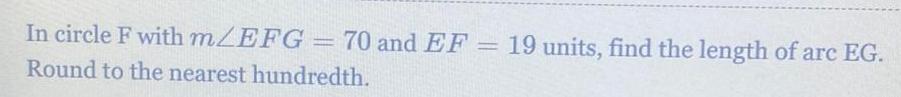
Geometry
2D GeometryIn circle F with m∠EFG = 70 and EF = 19 units, find the length of arc EG.
Round to the nearest hundredth.

Geometry
2D GeometryWhich is the best description of congruent line segments?
perpendicular line segments
line segments that are parallel
line segments that have the same length
line segments that share a vertex
u will need this Theorem to answer the next question.
The triangle angle bisector theorem states that in a triangle, the angle
Disector of any angle will divide the opposite side in the ratio of the sides
containing the angle. Consider the figure below:

Geometry
2D GeometryA square has a side length of 10 inches. What is the length of the diagonal of the square? You may draw a picture to help. Round your final answer to the nearest tenth.
diagonal length =___ inches

Geometry
2D GeometryParabola A and parabola B both have the x-axis as the directrix. Parabola A has its focus at (3, 2) and parabola B has its focus at (5, 4). Select all true statements.
A. Parabola A is wider than parabola B.
B. Parabola B is wider than parabola A.
C. The parabolas have the same line of symmetry.
D. The line of symmetry of parabola A is to the right of that of parabola B.
E. The line of symmetry of parabola B is to the right of that of parabola A.
![Find the area of quadrilateral ABCD. [Hint: the diagonal divides the quadrilateral into two triangles.]
A. 39.84 units²
B. 46.11 units²
C. 44.28 units²
D. 36.38 units2](https://media.kunduz.com/media/sug-question/raw/84812865-1659483288.270308.jpeg?w=256)
Geometry
2D GeometryFind the area of quadrilateral ABCD. [Hint: the diagonal divides the quadrilateral into two triangles.]
A. 39.84 units²
B. 46.11 units²
C. 44.28 units²
D. 36.38 units2

Geometry
2D GeometryFind the missing the side of the triangle.
A. 4√2 m
B. √130 m
C. √179 m
D. √211 m

Geometry
2D GeometryQuadrilatera ABCD is similiar to quadrilateral EFGH. The lengths of the three longest sides in
quadrilateral ABCD are 24 feet, 16 feet, and 12 feet long. If the two shortest sides of quadrilateral EFGH are 9 feet long and 18 feet long, how long is the 2nd longest side on quadrilateral EFGH?
A. 24 feet
B. 16 feet
C. 20 feet
D. 36 feet

Geometry
2D GeometryFind the distance between the points (0, 10) and (-9, 1).
A. 12.73
B. 14.21
C. 16.23
D. 20.22

Geometry
2D GeometryWhat type of triangle has side lengths 4, 4√15, and 16?
A. acute
B. not a triangle
C. obtuse
D. right

Geometry
2D GeometryIf the length of one leg of a right triangle is 3 and the hypotenuse is √34, what is the length of the other leg?
A. 8
B. 5
C. 34
D. √43

Geometry
2D GeometryWhich of the following is the rule for rotating the point with coordinates (x,y). 180° clockwise about the origin?
A. (x,y) → (y,x)
B. (x,y) → (-y, -x)
C. (x,y) → (-x, y)
D. (x,y) → (y, -x)
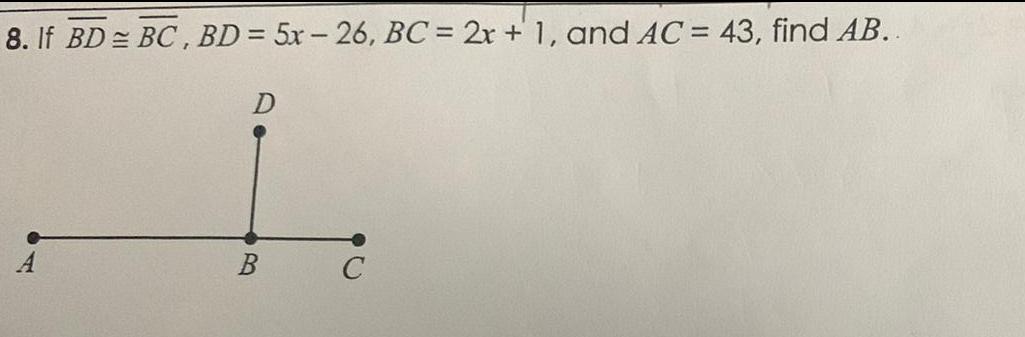

Geometry
2D GeometryGiven: Parallelogram ABCD with diagonal A
Prove: ∠D congruent to ∠B
You may not use the theorem stating that opposite angles of a parallelogram are congruent.

Geometry
2D GeometrySimilar Triangles Theorem 128. For triangles T and T', the following are equivalent; that is, each one implies the other two.
(i) T is similar to T', that is, T is congruent to a dilation of T'
(ii) Each angle of T is congruent to the corresponding angle of T'
(iii) The sides of T are proportional to the sides of T'.
![Theorem 111. Dilations preserve angle measure. Specifically, if noncollinear points A, B and C go to (necessarily) non-collinear points A', B' and C' under a dilation, then ∠ABC = ∠A'B'C'.
[Draw a diagram and apply what you know.]
The definition of a dilation says that the length of each segment coming from the center O is multiplied by the scaling factor k, that is, L(OP')=kL(OP). We would like to find out if the same relationship holds for every segment.](https://media.kunduz.com/media/sug-question/raw/84752680-1659186341.0978599.jpeg?w=256)
Geometry
2D GeometryTheorem 111. Dilations preserve angle measure. Specifically, if noncollinear points A, B and C go to (necessarily) non-collinear points A', B' and C' under a dilation, then ∠ABC = ∠A'B'C'.
[Draw a diagram and apply what you know.]
The definition of a dilation says that the length of each segment coming from the center O is multiplied by the scaling factor k, that is, L(OP')=kL(OP). We would like to find out if the same relationship holds for every segment.
Geometry
2D GeometryGiven the points C(-1,-5) and D(-7, 11), find the coordinates of point E on CD such that the ratio of CE to ED is 5:3.