2D Geometry Questions and Answers
Geometry
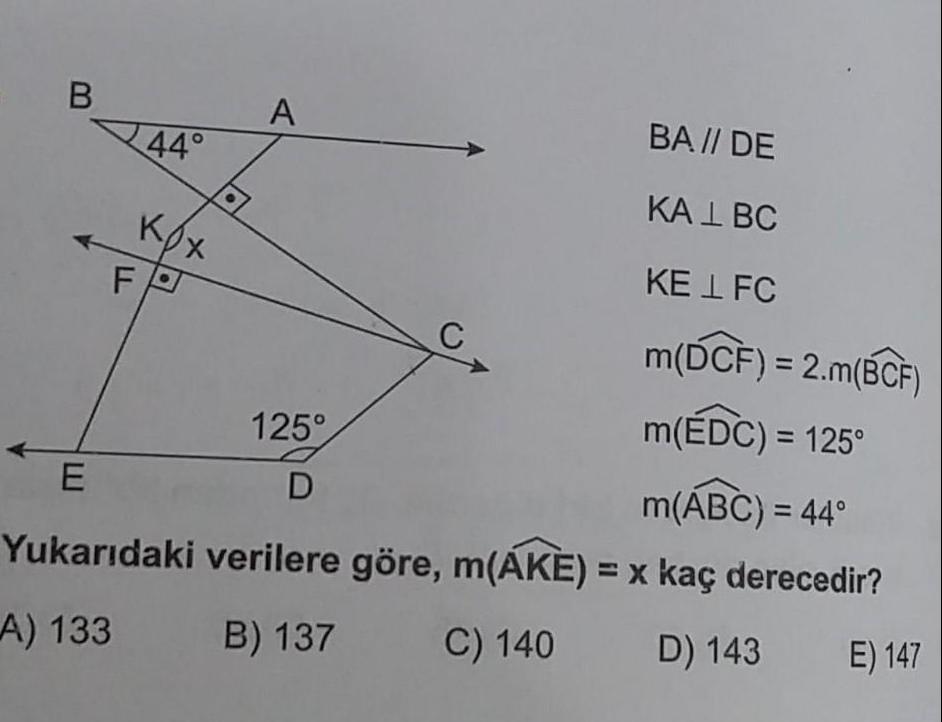
2D GeometryB BA DE KAL BC KE LFC m DCF 2 m BCF m EDC 125 E m ABC 44 Yukar daki verilere g re m AKE x ka derecedir A 133 B 137 C 140 D 143 44 Kox FO A 125 D E 147

Geometry
2D GeometryIn triangle ABC angle A is 60 and C is a right angle The length of side bis 9 as shown Find the lengths of the other sides and c Give the exact answers but you may not use sin cos or tan Use sqrt 3 or 3 5 for 3

Geometry
2D GeometryWhich of the following represent deductive reasoning Select all that apply The measures of the two angles in a linear pair add to 180 ZNTR and ZMKG form a linear pair so the measures of NTR and ZMKG add to 180 Birds fly south for the winter A penguin is a bird so it will fly south in the winter The sum of the measures of the interior angles of any triangle is 180 Polygon QAG is a triangle so the sum of the measures of its interior angles must be 180 Traffic is always busy on Friday afternoons at 4pm It is Friday at 4pm so traffic will be busy All rectangles are quadrilaterals A square is a rectangle so a square must be a quadrilateral
Geometry
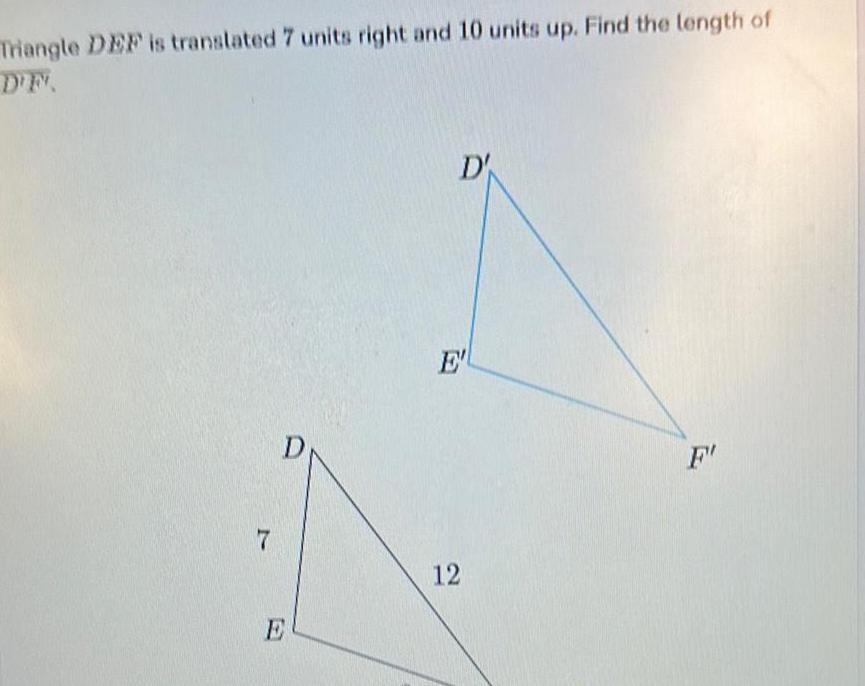
2D GeometryTriangle DEF is translated 7 units right and 10 units up Find the length of D F 7 D E 12 F

Geometry
2D Geometryraph the equation 40 y2 2x Ay 43 4 2 2 2 2 4x Evaluate the expression 41 2x 3y 6 4 2 41 2 2 4 2 4x

Geometry
2D Geometryopen top rectangular box is to be made from a piece of cardboard 26 inches long and 8 inches wide by cutting out identical squares from the four corners and turning up the sides In the image below you can adjust the location of the point to visualize different possible boxes Let x be the side length of each of the removed squares from the corners Express the volume V of the resulting box as a function of x V x powered by desmos What is the domain of this function Use interval notation Find the dimensions of the box that yields a maximum volume Round your answers to two decimal places

Geometry
2D Geometrysin 0 tan 0 csc 0 sec 8 cot 8 Need Help Submit Answer 3 5 Points sin 0 cos 0 Find the values of the trigonometric functions of 0 from the information given csc 0 4 0 in Quadrant I tan 0 Read It sec 0 DETAILS Watch It
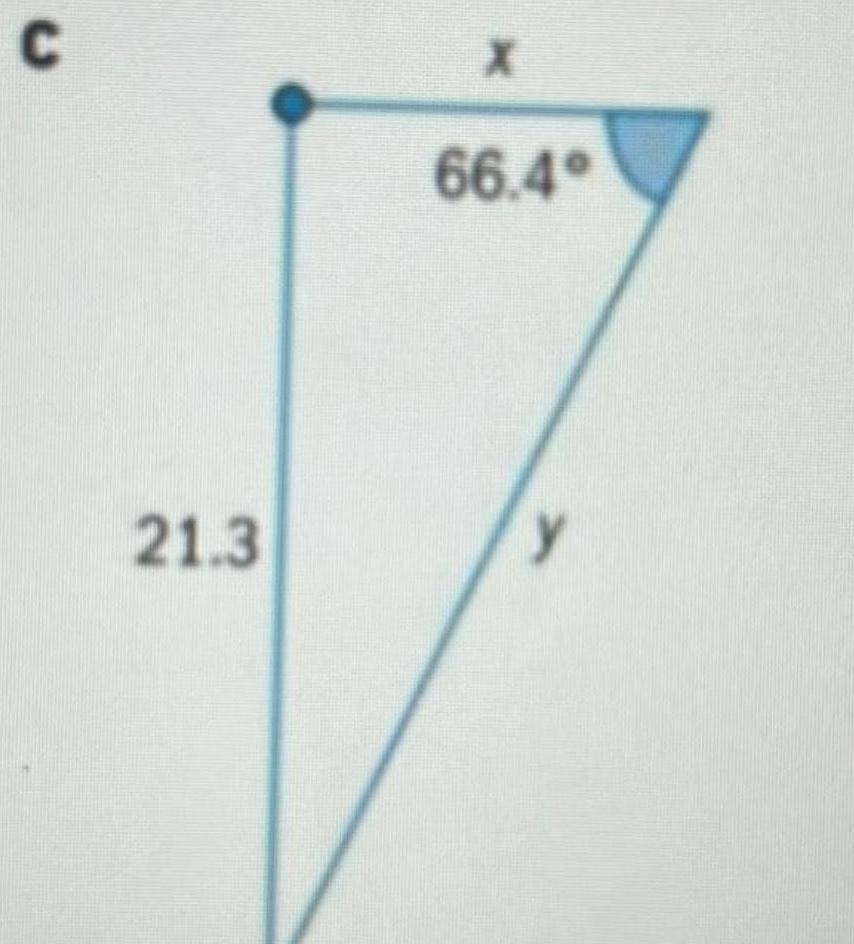
Geometry
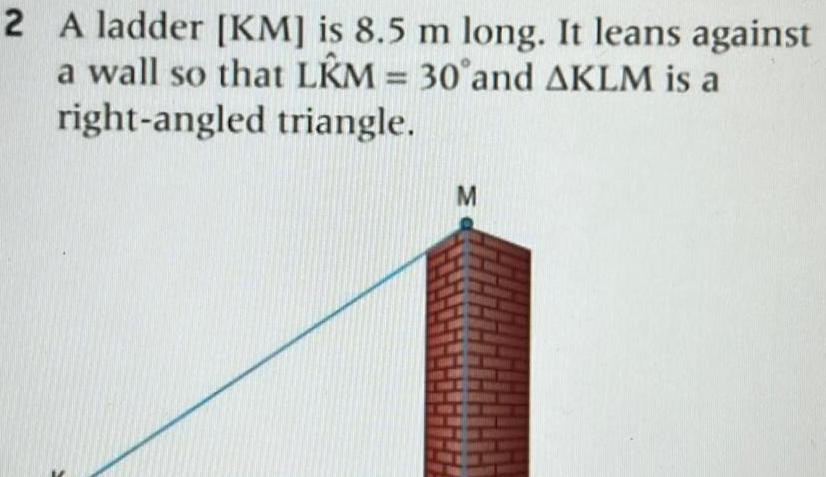
2D Geometry2 A ladder KM is 8 5 m long It leans against a wall so that L M 30 and AKLM is a right angled triangle M

Geometry
2D Geometry1 The angle of depression from the top of a cliff to a boat at sea is 17 The boat is 450 m from the shore Draw a diagram representing the situation Find the height of the cliff giving your answer rounded to the nearest metre 2 A family wants to buy an awning for a French window that will be long enough to keep out the sun when it is at its highest point in the sky The height of the French window is 2 80 m The angle of elevation of the sun from this point to the bottom of the window is 70 The awning will be 90 to the window Find how long the awning should be Write down your answer correct to 2 dp a b
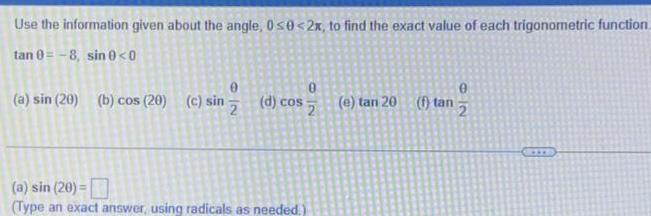
Geometry
2D GeometryUse the information given about the angle 0 0 2x to find the exact value of each trigonometric function tan 0 8 sin 0 0 0 a sin 20 b cos 20 c sin d cos 02 a sin 20 Type an exact answer using radicals as needed e tan 20 f tan 0 2

Geometry
2D Geometry3 A hiker whose eye is 1 6 metres above ground stands 50 metres from the base of a vertical cliff The angle between the line connecting her eye and the top of the cliff and a horizontal line is 58 a Draw a diagram representing the situation b Find the height of the cliff

Geometry
2D Geometryangles with the following sides are right angled triangles a 9cm 40 cm 41 cm b 10 m 24 m 26 m c 10 10 200 d 11 2 7 5 8 3 3 The spiral in the figure is made by starting with a right angled triangle with both legs of length 1 unit 1 1 1 11 1 a Find the hypotenuse of the first triangle Then the second right angled triangle is built with one leg measuring 1 unit and the other leg being the hypotenuse of the first triangle b Find the hypotenuse of this second triangle A third right angled triangle is built on the second triangle s hypotenuse again with the other leg measuring 1 unit c Find the hypotenuse of the third triangle The process is continued in the same fashion and the hypotenuse of the final triangle is denoted by x d Find the length of x 4 A right angled triangle has a hypotenuse of 8 2 cm and another side length of 4 3 cm Draw a diagram Calculate the area of the triangle Give your answer correct to 1 dp

Geometry
2D GeometryA door with width 4 20 m has an arc as shown in the diagram Find a b The radius of the arc to the nearest cm The length of the arc to the nearest cm 225 4 20 m


Geometry
2D Geometry3 The image of a speck of dust viewed through an electron microscope is 1 2 x 10 millimetres wide The image is 5 x 102 times as large as the actual size Determine the width in millimetres of the actual speck of dust
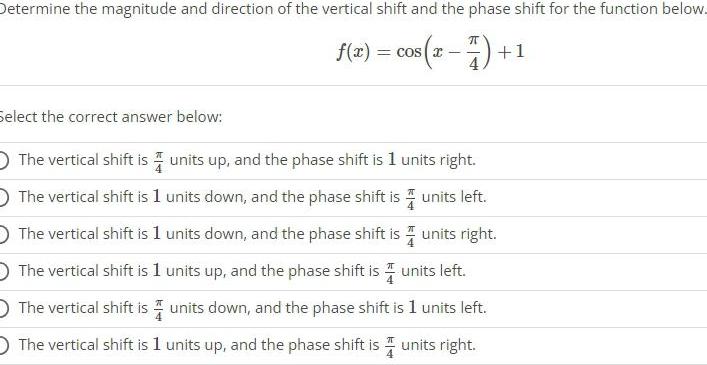
Geometry
2D GeometryDetermine the magnitude and direction of the vertical shift and the phase shift for the function below f x cos x 7 Select the correct answer below The vertical shift is units up and the phase shift is 1 units right The vertical shift is 1 units down and the phase shift is The vertical shift is 1 units down and the phase shift is The vertical shift is 1 units up and the phase shift is units left O The vertical shift is units down and the phase shift is 1 units left The vertical shift is 1 units up and the phase shift is units right 1 units left units right

Geometry
2D GeometryFind the range of possible values for the following measurements which were rounded to the nearest mm tenth of m and hundredth of kg respectively b 3 2 m c 1 75 kg a 24 mm
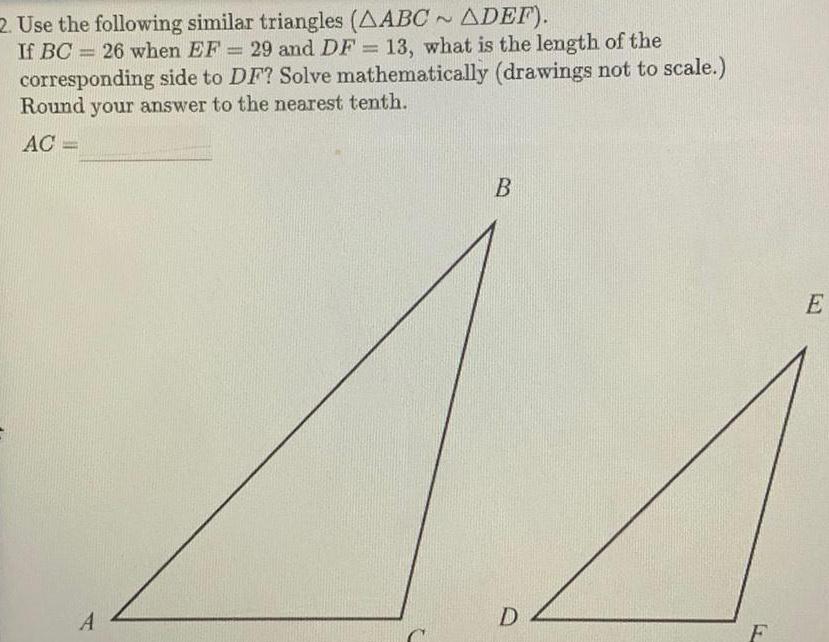
Geometry
2D Geometry2 Use the following similar triangles AABC ADEF If BC 26 when EF 29 and DF 13 what is the length of the corresponding side to DF Solve mathematically drawings not to scale Round your answer to the nearest tenth AC A B D F E
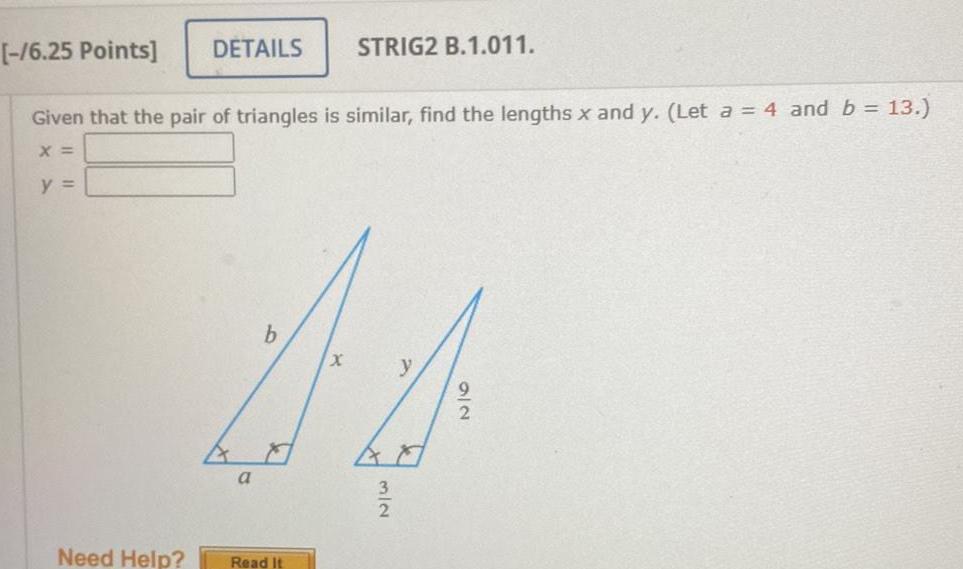
Geometry
2D Geometryy 6 25 Points Given that the pair of triangles is similar find the lengths x and y Let a 4 and b 13 X DETAILS Need Help a b STRIG2 B 1 011 Read It 3 2 y 2 2

Geometry
2D GeometryW 1 Volume of cubes prisms and pyramids JUB What is the volume of this triangular pyramid 6 m Submit 9 m 10 m cubic meters

Geometry
2D GeometryUse the graph to answer the question Y T S Q 2 4 2 N 2 R 29 Which ordered pair corresponds to point U 30 Which ordered pair corresponds to point S 31 Which point is located in Quadrant II
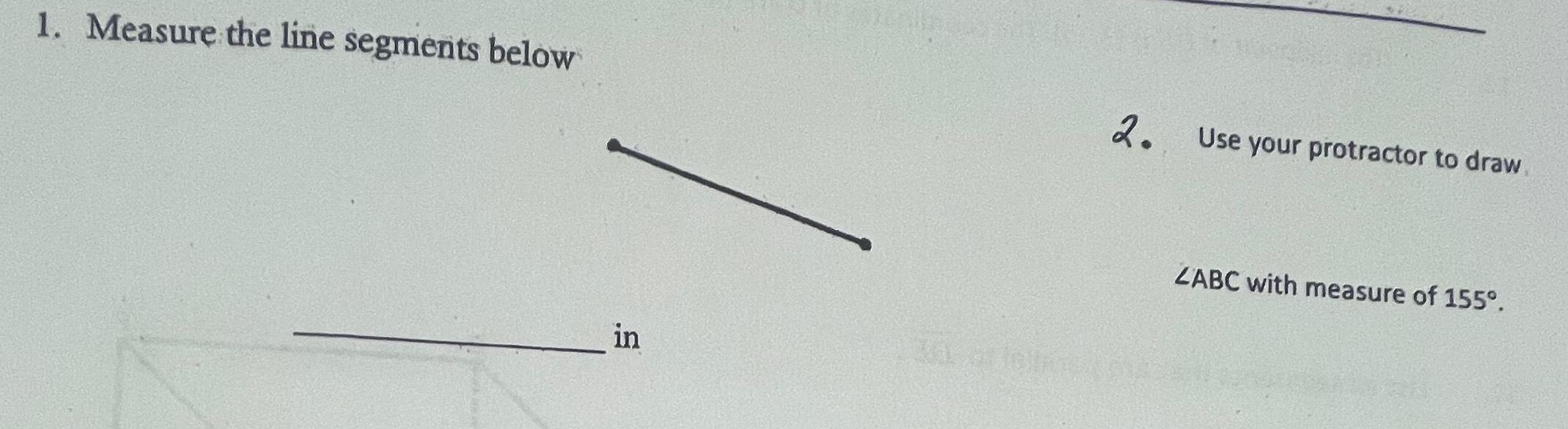
Geometry
2D Geometry1 Measure the line segments below in 2 Use your protractor to draw ZABC with measure of 155

Geometry
2D Geometry3 4 Name the plane that represents the bottom of the box Find the intersection of DC and CF Find the intersection of planes EDCF and BGFC Name the two planes that intersect at EF Shado plano CBG 0144 D A sipas telleme E H C B F

Geometry
2D Geometry2 3 of DE is 3 5 The coordinates of D are 1 5 What are the coordinates of E GADS PIRE JEAS List all segments that are parallel to DE List one segment that is skew to AB xod sids D E F G
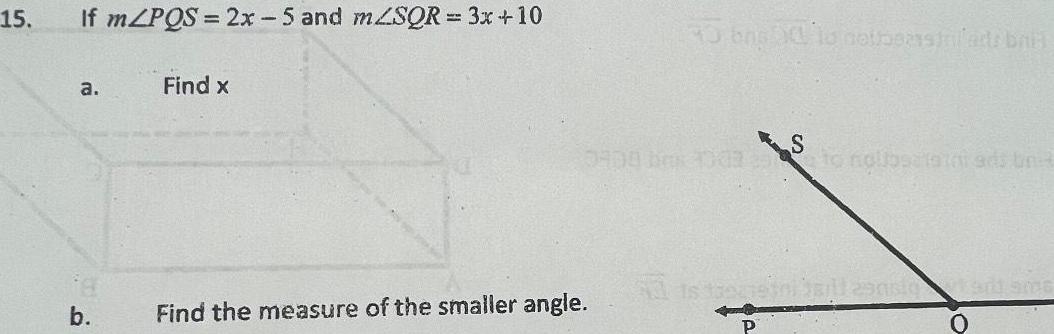
Geometry
2D Geometry15 If mZPOS 2x 5 and mZSQR 3x 10 a b Find x to engad to notrpensinilants bali S 0909 10 to nolasetain ads unit Find the measure of the smaller angle Al is there in baill anstadt ame P
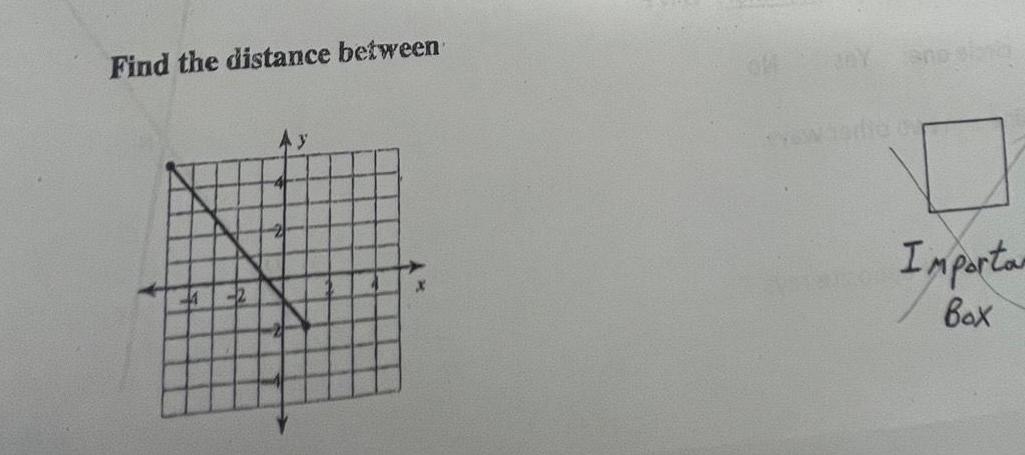
Geometry
2D GeometryMatch the following number sequences to the formulas 5 10 20 40 5 10 15 20 4 8 16 32 4 8 12 16 DRAG DROP THE ANSW a 5 5n an 4 2 1 an 4 4 n 1 an 5 5 n 1 an 5 2
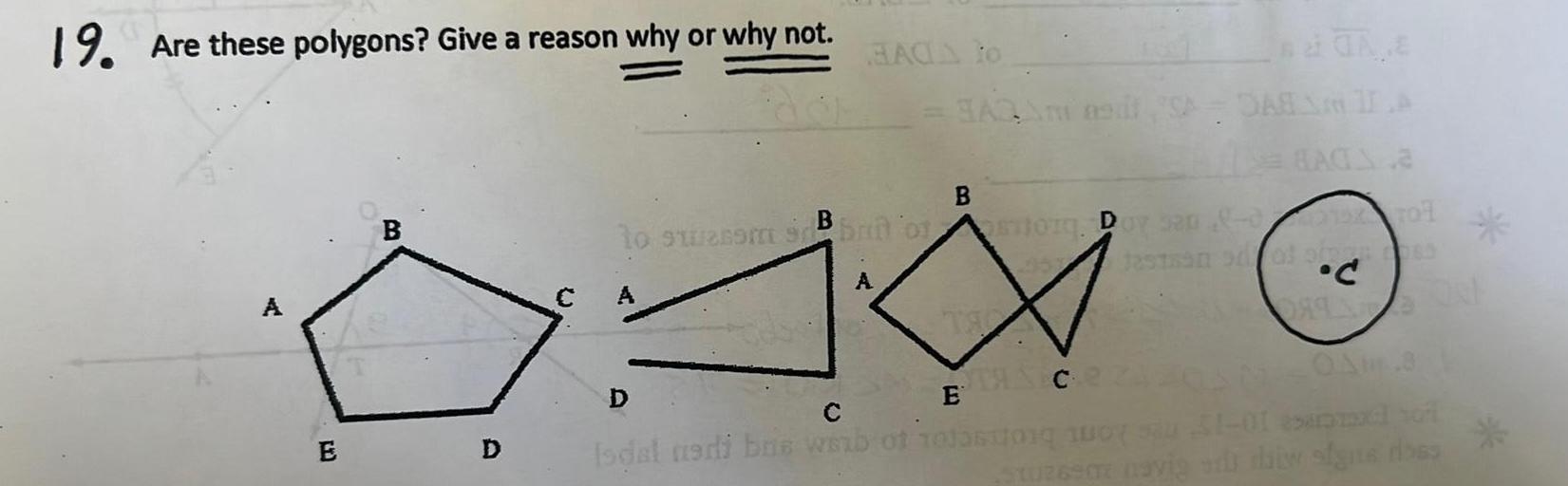
Geometry
2D Geometry19 Are these polygons Give a reason why or why not BAC to A od D E B B to swesom sa brit of torq Day 320 30 C SASA DAS A HACS 2 A E HACE Isdal nedi bus w of two fastsan 9000f om oss C 08935 001 ON 8 stuz6em navig adi daw signe ross

Geometry
2D GeometryClassify 7 Sides 09 4 sides the PolygoN 12 sides please 9 Sides Smal sielamos of trigins surgit sri sau 2 1 292075 207 BASS to ABS te

Geometry
2D GeometryK For Exercises 1 5 use the figure at right to complete each statement of ZBAE 1 A is the Mid Paint 2 AD is the Ray 3 AD is a 4 If mLBAC 42 then mLCAE 5 LDAB DAE of LBAE of LDAE no vriw no Seasure of D

Geometry
2D GeometryFor Exercises 10 12 use your protractor to draw and then label each angle with the given measure 10 mLMNO 15 11 m RIG 90 12 mLz 160

Geometry
2D GeometryPeas are measured by diameter Determine the area of a 6 inch personal pizza Round your answer to the nearest hundredth AN of square inches Determine the area of a 14 inch personal pizza Round your answer to the nearest hundredth 18394 osquare inches A6 inch personal pizza has 580 calories Determine the number of calories in the 14 inch poza Round your answer to the nearest calorie There are calories in the 14 inch pizza A 14h plas cut into 8 slices Estimate the number of calories in one slice of a 14 inch plesa Round your answer to the nearest calorie

Geometry
2D GeometryIf carpet costs 16 65 per square yard and is available in whole square yards only find the cost of carpeting the three bedroom floors in the accompanying floor plan Click the icon to view the floor plan It will cost to carpet the three bedroom floors www

Geometry
2D GeometryIn the diagram M is the midpoint of the segment Find the indicated length Find LN L X 9 M 4x N

Geometry
2D GeometryFind MidPoint 1 9 10 10 2 2 1 3 3 1 M x x x x 2 Find Distance between the follow 2 points 3 2 1 4 3 4 3 5 7 1 Distance Formula x x x Y d


Geometry
2D GeometryComplete part a and choose another part to complete for credit Show all of your work Use geometric relationships to find angle measures Start by finding a special relationship the the bolded words on the handout between some of the sides or angles and use that relationship to write an equation Solve the equation for the variable then use that variable value to answer the original question a Find m MNP N 152 J B c Find m DBC Af 2x 4 M D x 7 C b Find m FGH K G 4x 3x 2 F x 28 4 P IN d Find m LPQ and m LP H M

Geometry
2D Geometry4 5 17m 11m O D Terminal Angle sin cos tan 8 Terminal Angle sin 8 cos 8 tan 8 Terminal Angle sin 8 cos 0 tan 0 csc sec 8 cot csc 8 sec cot 8 csc 8 sec 8 cot 8 Radians Radians Radians


Geometry
2D GeometryPeriod The Distance Formula Find the distance between each pair of points Round your answer to the nearest tenth if necessary 2 1 3 5 7 2 3 7 7 9 5 9 7 7 11 10 7 8 1 4 Name 6 y 8 2 9 1 4 10 8 5 1 3 X 12 6 10 2 10

Geometry
2D Geometry11 The diagram shows the shape of one hole at a mini golf course The course is being renovated and the grass surface replaced Find how many square feet of grass will be needed to cover this area If the wooden railing along the outside also needs to be replaced how many feet of railing will be needed 12 The mini golf course has several holes with the same shape just a different size Write polynomials that can be used to calculate the amount of grass and railing needed for any course that shape 13 The mini golf course has another generic shape used for several holes write a polynomial for the area and perimeter of this shape as well 3x 7 ft 14 7 ft 2x 7 ft 14 ft x ft ft x ft 7 ft DE x ft 2x ft x ft x ft 2x f
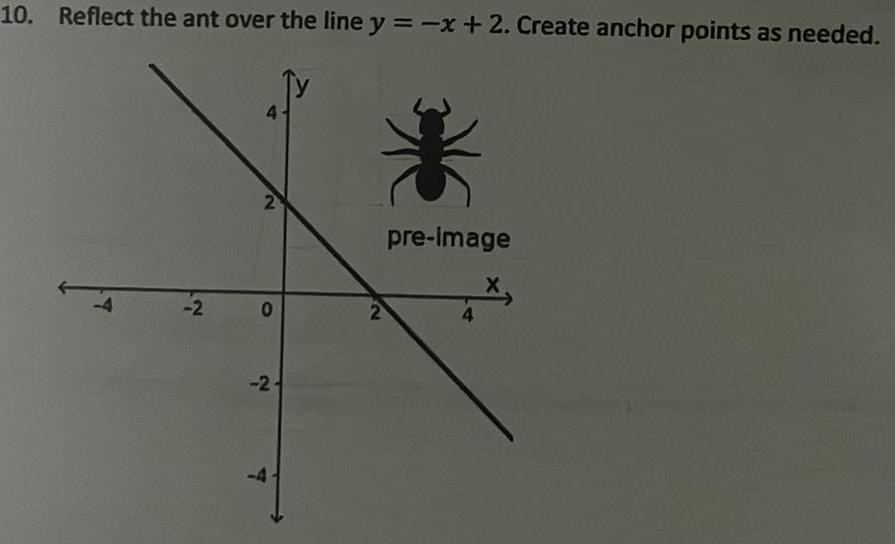
Geometry
2D Geometry10 Reflect the ant over the line y x 2 Create anchor points as needed 2 4 2 0 2 4 2 pre image

Geometry
2D Geometry12 g x 2x 3 5 0 5 5 13 State at least one similarity and one difference between the functions for
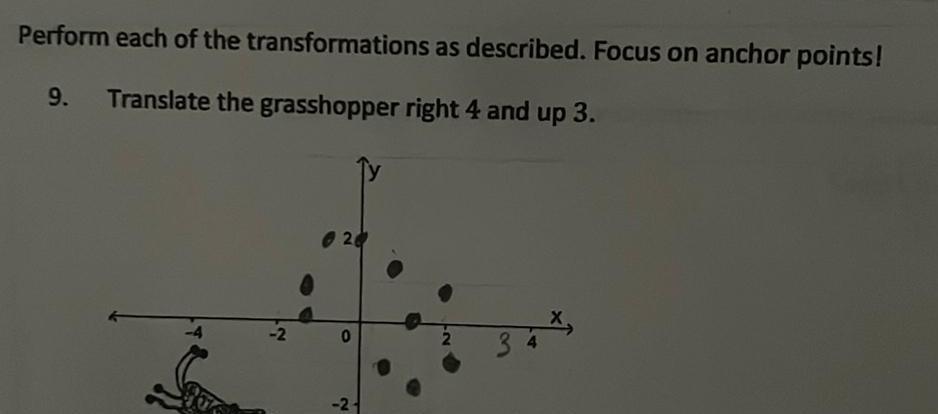
Geometry
2D GeometryPerform each of the transformations as described Focus on anchor points 9 Translate the grasshopper right 4 and up 3 2 20 0 2 2 3

Geometry
2D Geometry20 Construct compass and straightedge AEFG F G Please don t answer jost try to explay

Geometry
2D GeometryR 4x 3 X M 14 a What is the value of x 6x 12 b What is the length of RM 2X 15 c What is the length os RS 66 3x 1 X N 15 a What is the value of x M b What is the lenghth of MY c What is the lenghth of XY is Ray 5x48 8x 24 M 16 a What is the value of x b What is the length of XM c What is the length of XY 9x 12
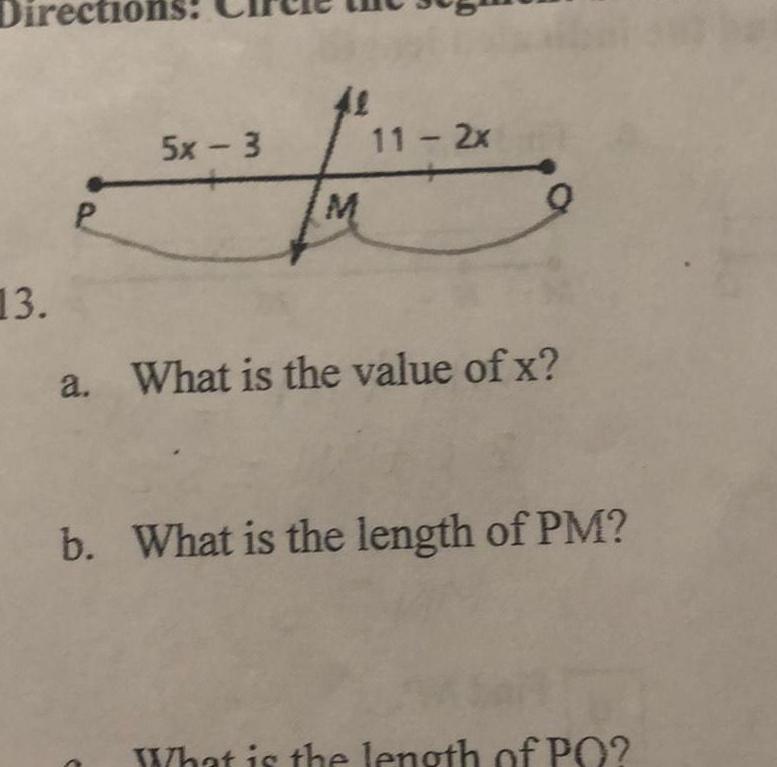
Geometry
2D GeometryDir 13 P 5x 3 M 11 2x Q a What is the value of x b What is the length of PM What is the length of PO

Geometry
2D GeometryCONNECTING CONCEPTS Point S is between points R and I on RT Use the information to write an equation in terms of x Then solve the equation and find RS ST and RT a RS 2x 10 ST x 4 RT 21 6 Equation X b RS x 9 ST 19 RT r 14 Equation X A CONNECTING CONCEPTS In the diagram AB BC AC CD and AD 12 Find the lengths of all segments in the diagram AB BC RS ST BD a AC RS ST RT B D
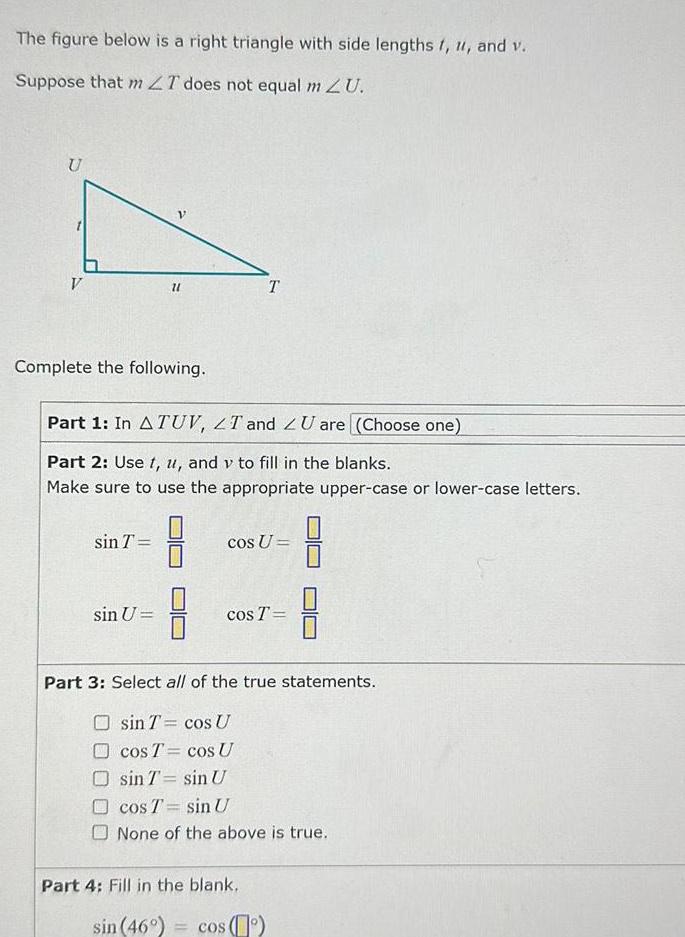
Geometry
2D GeometryThe figure below is a right triangle with side lengths t u and v Suppose that m LT does not equal m ZU U V Complete the following 21 sin T Part 1 In A TUV LT and ZU are Choose one Part 2 Use t u and v to fill in the blanks Make sure to use the appropriate upper case or lower case letters sin U 8 T cos U cos T Part 3 Select all of the true statements sin T cos U cos T cos U sin T sin U cos T sin U None of the above is true Part 4 Fill in the blank sin 46 cos