Complex numbers Questions and Answers



Algebra
Complex numbersHow did the Fourteenth Amendment become significant in certain Supreme Court decisions O This amendment limited rights for all citizens excluding land owning citizens O The due process clause addresses certain rights given to the states and not to the people O The due process clause prohibits states from depriving citizens of their natural rights O This amendment establishes that individual rights do not apply in certain state laws
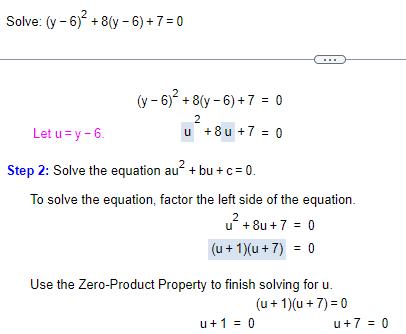
Algebra
Complex numbersSolve y 6 8 y 6 7 0 y 6 8 y 6 7 0 2 u 8u 7 0 Let u y 6 Step 2 Solve the equation au bu c 0 To solve the equation factor the left side of the equation u 8u 7 0 u 1 u 7 0 Use the Zero Product Property to finish solving for u u 1 u 7 0 u 1 0 u 7 0

Algebra
Complex numbers5 A high school principal wants to rate student experience on the school bus He stops the first fifteen students who come into the school one morning and asks them to rate their school bus experience on a scale of 1 worst to 10 best Is the principal s method of surveying students valid A Yes it is valid because it was a scaled rating B Yes it is valid since the students were chosen randomly C No it is invalid because the bus driver should have conducted the survey D No it is invalid since the students were chosen by convenience
Algebra
Complex numbersAn equation that is quadratic in form is solved by a substitution u x 6 and it has solutions u 3 and u 5 What are the values of x that solve the original equation The values of x that solve the original equation are Type integers or a simplified fractions Use a comma to separate answers as needed

Algebra
Complex numbersof the six trigonometric functions for if 7 12 is a point on the terminal side of 8 Reduce fractions and simplify radicals Do not use decimals Answers for the six trigonometric values cos 8 sin 0 tan 0 Remember decimals are NOT allowed sec 0 csc 0 cot 0
Algebra
Complex numbers4 7 Determine the exact value of sec 0 if is in Quadrant IV and tan 0 6 6 A sec 0 85 85 B sec 0 85 7 C sec 0 85 D sec 0 85 6 85 E sec 0 6
Algebra
Complex numbersA ball is thrown from a height of 65 meters with an initial downward velocity of 10 m s The ball s height h in meters after t seconds is given by the following h 65 101 57 How long after the ball is thrown does it hit the ground Round your answer s to the nearest hundredth If there is more than one answer use the or button ground seconds or X

Algebra
Complex numbersUse the ALEKS graphing calculator to find the x intercept s and vertex for the quadratic function f x 4x 2x 3 Round to the nearest hundredth if necessary If there is more than one x intercept separate them with commas If applicable click on None 0 x intercept s 0 0 None

Algebra
Complex numbersThe length of a rectangle is 5 m more than twice the width and the area of the rectangle is 63 m Find the dimensions of the rectangle

Algebra
Complex numbersFind the equation of the line Use exact numbers y x 9 8 7 6 5 4 3 2 1866 9 7 2345 6 5 4 3 2 1 Y ST 4 78 6 7 8 9 O 1 2 3 4 5 6 7 8 9

Algebra
Complex numbersin a triangle one leg measures 14 km and the other leg measures 48 km what is the length of the hypotenuse
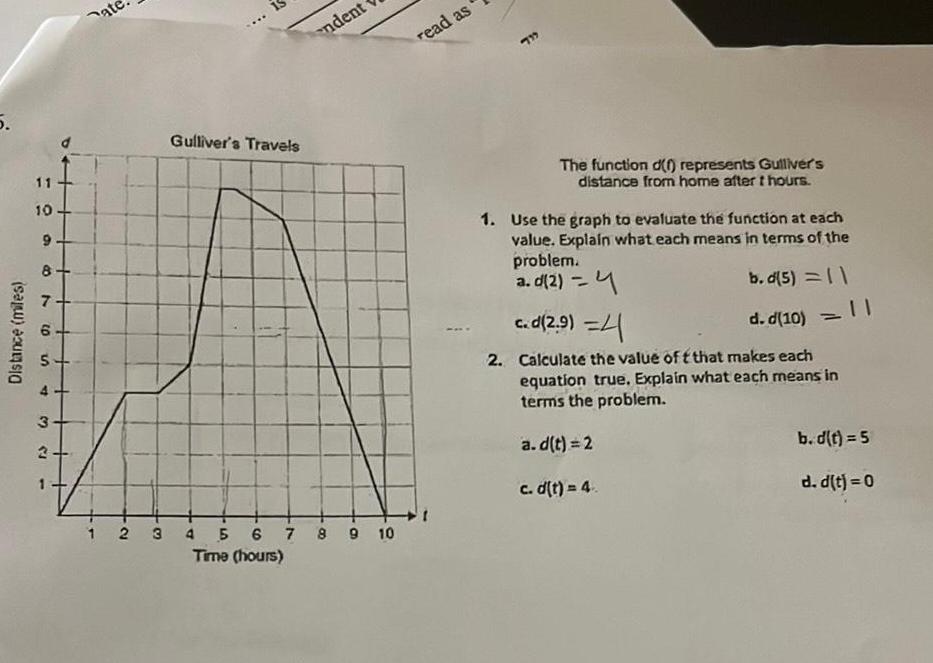
Algebra
Complex numbers5 Distance miles 0 10 9 8 765 3 1 3 ate 2 3 19 Gulliver s Travels 4 5 6 Time hours 7 endent 8 9 10 read as 711 The function d represents Gulliver s distance from home after t hours 1 Use the graph to evaluate the function at each value Explain what each means in terms of the problem a d 2 4 b d 5 11 c d 2 9 4 d d 10 2 Calculate the value of t that makes each equation true Explain what each means in terms the problem a d t 2 c d t 4 b d t 5 d d t 0

Algebra
Complex numbers3 Using the table of values find the following 9 4 X f x a f 3 c f 8 0 1 e If f x 9 what is x g If f x 0 what is x 8 4 P A 3 2 9 3 2 b f 20 d f 1 f If f x 4 what is x h If f x 5 what is x 5 8 20 0
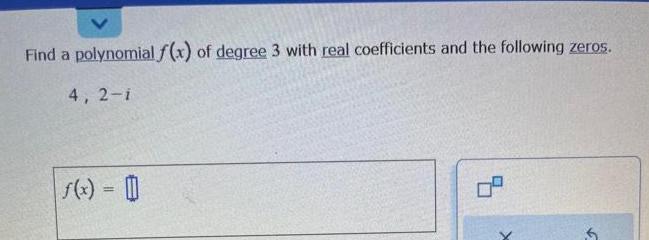
Algebra
Complex numbersFind a polynomial f x of degree 3 with real coefficients and the following zeros 4 2 i f x
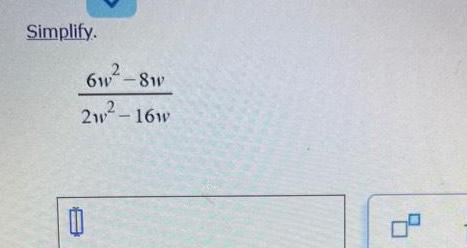
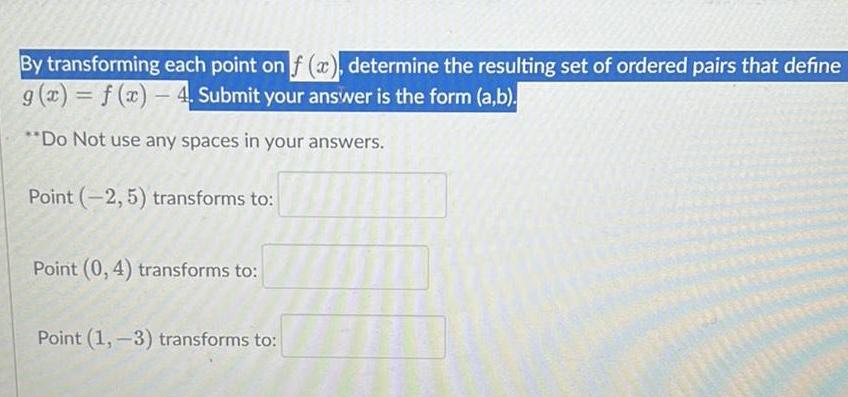
Algebra
Complex numbersBy transforming each point on f x determine the resulting set of ordered pairs that define g x f x 4 Submit your answer is the form a b Do Not use any spaces in your answers Point 2 5 transforms to Point 0 4 transforms to Point 1 3 transforms to
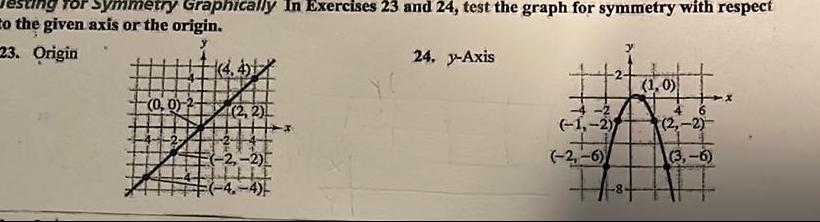
Algebra
Complex numberssting for Symmetry Graphically In Exercises 23 and 24 test the graph for symmetry with respect to the given axis or the origin 23 Origin 0 0 14 412 2 2 244 2 2 4 4 24 y Axis 4 2 1 2 2 6 1 0 4 6 2 2 3 6

Algebra
Complex numbersTony is going to invest to help with a down payment on a home How much would he have to invest to have 36 800 after 10 years assuming an interest rate of 1 61 compounded monthly Do not round any intermediate computations and round your final answer to the nearest dollar If necessary refer to the list of financial formulas
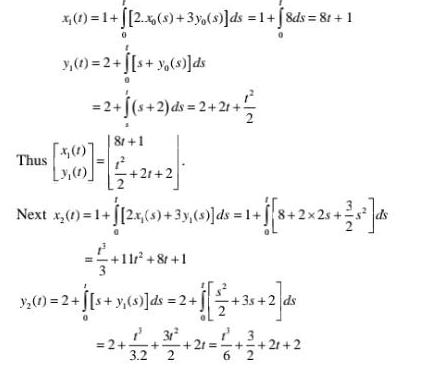
Algebra
Complex numbersThus 1 1 2 s 3yo s ds 1 8ds 81 1 y 1 2 s y s ds 2 f s 2 ds 2 2t 2 x 1 L3 1 8r 1 21 2 Next x 1 1 2x s 3y s ds 1 8 11 8 1 Y 1 2 f s y s ds 2 2 1 3 1 2 21 3 2 2 1 8 2 25 2 3 ds dr 3 2 21 2 6 2

Algebra
Complex numbersTo solve inequalities involving subtraction use the Addition Property of Inequality homi Solve the inequality Check to justify your answer Then write the solution set S using words 1 n 4 10 n 4 4 10 4 n 6 Check 6 4 10 10 10 S numbers less than or equal to 6 4 3 h 31 2 9 b 45 5 13 k 8 Combine terms to solve Check to justify your answer Then write the solution set S using words 7 8 2 t 8 8 6 5 g 1 6 t 8 6 6 t 8 6 t 2 S numbers greater than 2 10 4 p 2 1 11 22 t 3 1 3 x 4 11 6 24 f 6 9 5 j 2 4 12 1 b 10 2

Algebra
Complex numbers1 The point 15 8 is on the graph of y f x Find the corresponding point on the graph of y g x where g x f x The corresponding point on the graph of y g x is Simplify your answer Type an ordered pair
Algebra
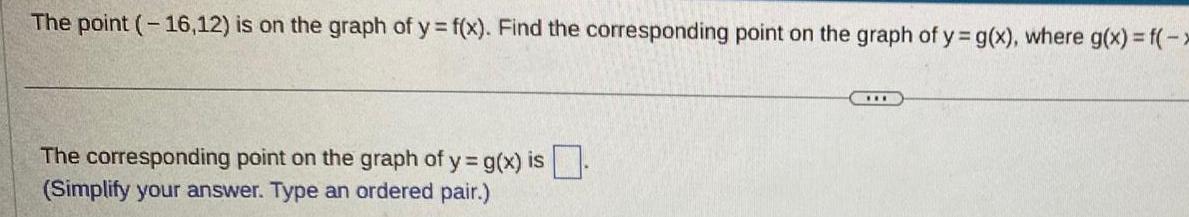
Complex numbersThe point 16 12 is on the graph of y f x Find the corresponding point on the graph of y g x where g x f x The corresponding point on the graph of y g x is Simplify your answer Type an ordered pair
Algebra
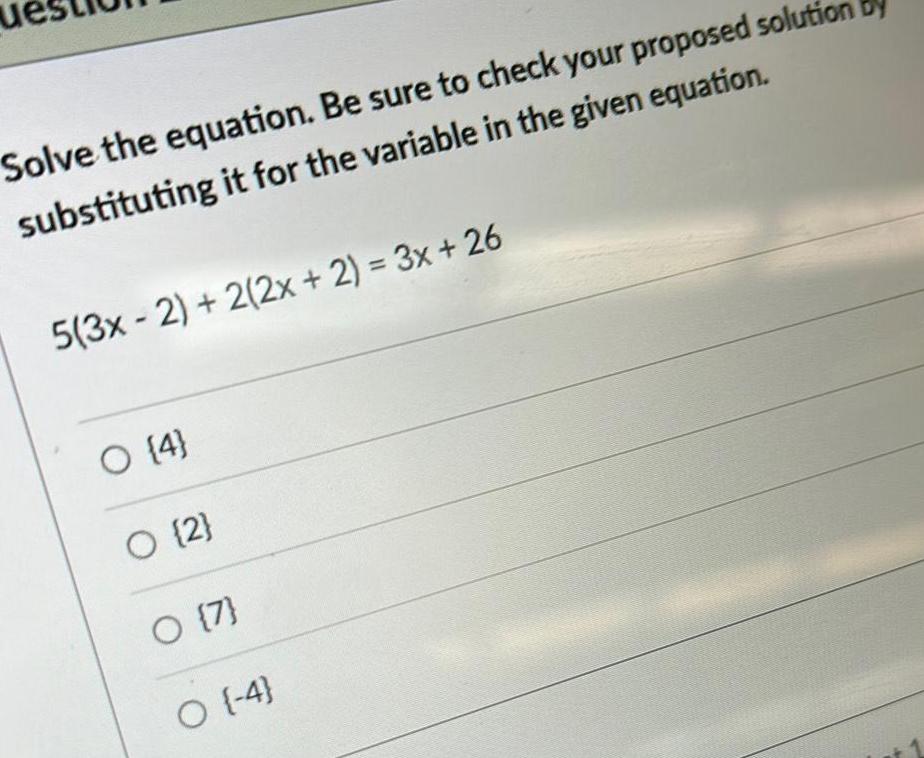
Complex numbersSolve the equation Be sure to check your proposed soluti substituting it for the variable in the given equation 5 3x 2 2 2x 2 3x 26 O 4 O 2 O 7 O 4 by
Algebra
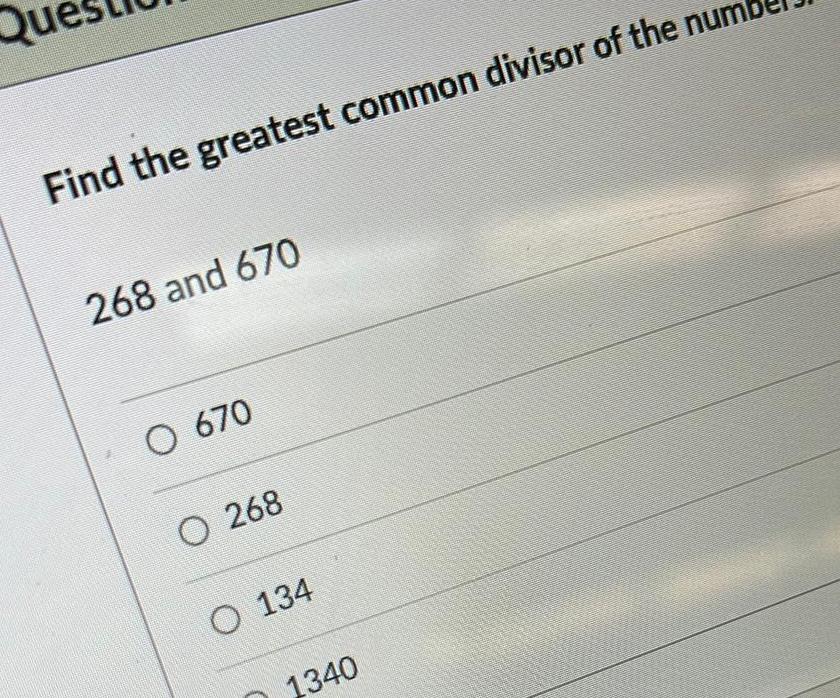
Complex numbersFind the greatest common divisor of the num 268 and 670 O 670 O 268 O 134 1340
Algebra
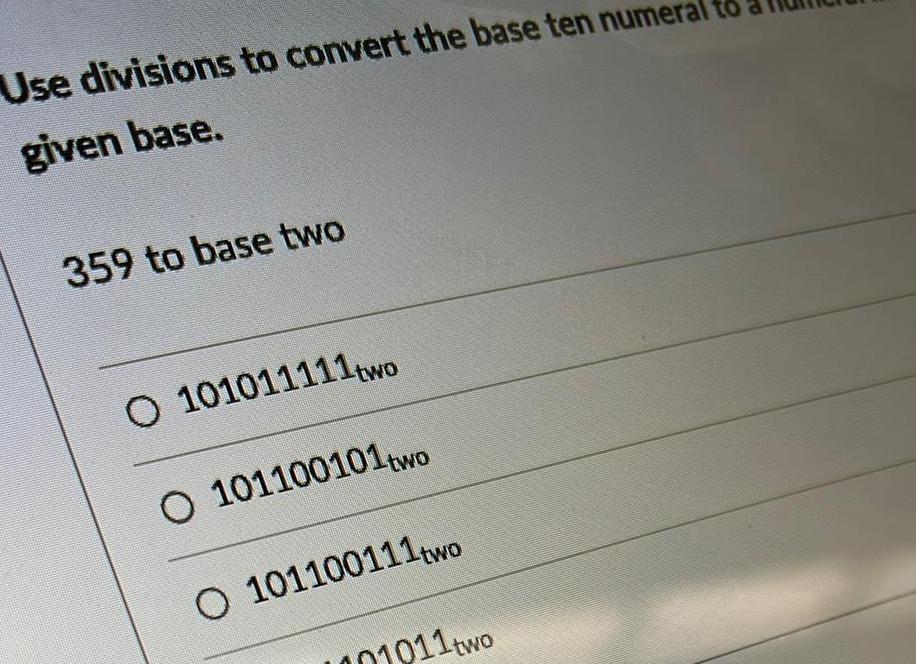
Complex numbersUse divisions to convert the base ten numeral to given base 359 to base two O 101011111two O 101100101two O 101100111two 1011two
Algebra
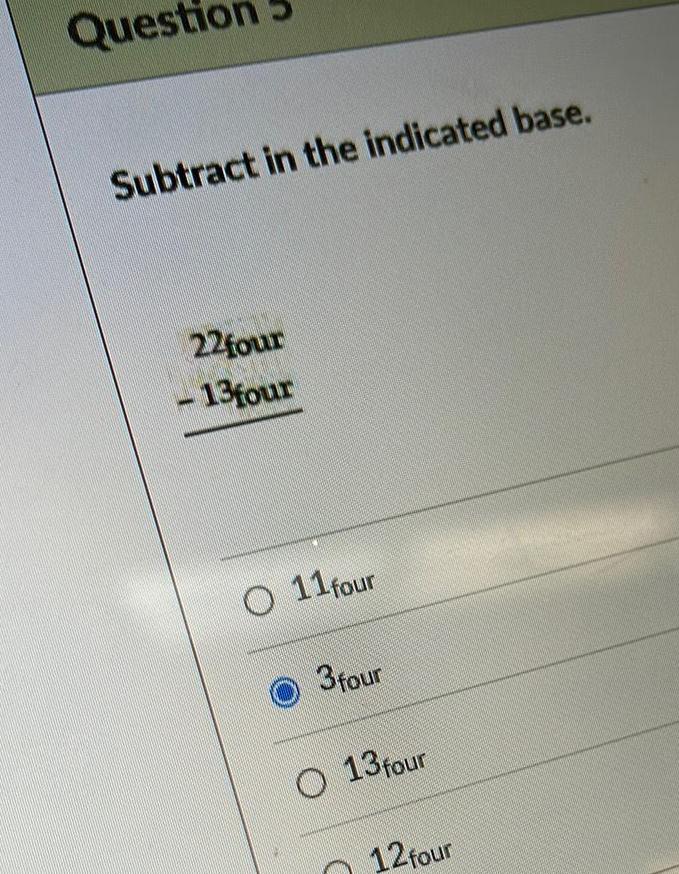
Complex numbersQuestio Subtract in the indicated base 22four 13 four O 11 four 3 four O 13 four 12 four

Algebra
Complex numbersFind the prime factorization of the composite number 252 024 x 7 O 22 x 32 x 7 O 34 x 7 2 x 3 x 7

Algebra
Complex numbersCalculate the slope of the line passing through the given undefined so state Then indicate whether the line rises falls is horizontal vertical 6 1 8 8 07 21 rises O 7 rises 07 2 falls 09 14 rises

Algebra
Complex numbers3 Daniel s Print Shop purchased a new printer for 35 000 Each year it depreciates at a rate of 5 Use an exponential function to find its approximate value after 8 years

Algebra
Complex numbersthen x t y t for all 1 JOJ Proof Recall that both x and y being solutions of the initial value problem satisfy the integral equations on their domain intervals x 1 x f s x s ds y t x f s y s ds Therefore x 1 y t s x s f s y s ds which implies x 1 y 1 0 S s x s s y s 0 Kx s y s for all 12 Applying Gronwalls result with A 0 we get 0 x 1 y 1 0 for all t t This gives the desired equality x 1 y 1 for all 1JJ Towards the uniqueness of the solution of the initial value problem 3 we consider all the solutions of the initial value problem 3 Let the totality of them be denoted by x J XEA the solutions x being thus indexed by a suitable indexing set A Above we have verified that any two solutions say x and x are equal on the overlap JJ of their domains Therefore we patch together all the solutions to get a maximal solution is the solution defined on the largest open interval It is obtained as follows Let J UJ A clearly Jis an open sub internal of I with XJ and all the solutions x patch up to get a solution x J of the initial value problem 3
Algebra
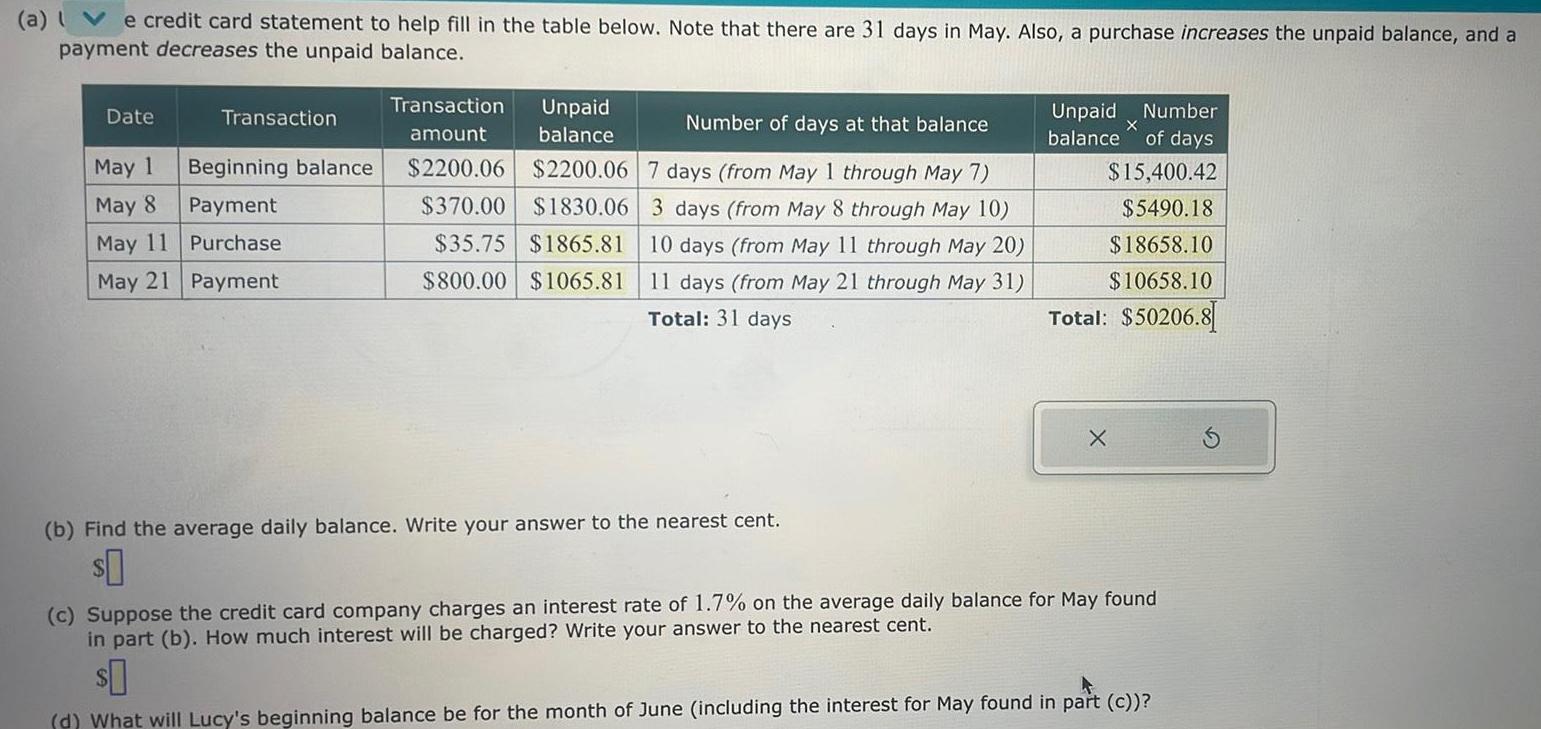
Complex numbersa e credit card statement to help fill in the table below Note that there are 31 days in May Also a purchase increases the unpaid balance and a payment decreases the unpaid balance Date Transaction May 1 May 8 May 11 May 21 Payment Beginning balance Payment Purchase Transaction amount Unpaid Number of days at that balance balance 2200 06 2200 06 7 days from May 1 through May 7 370 00 35 75 1865 81 10 days from May 11 through May 20 800 00 1065 81 11 days from May 21 through May 31 Total 31 days 1830 06 3 days from May 8 through May 10 b Find the average daily balance Write your answer to the nearest cent 0 Unpaid Number balance of days X 15 400 42 5490 18 18658 10 10658 10 Total 50206 8 X c Suppose the credit card company charges an interest rate of 1 7 on the average daily balance for May found in part b How much interest will be charged Write your answer to the nearest cent 0 d What will Lucy s beginning balance be for the month of June including the interest for May found in part c
Algebra
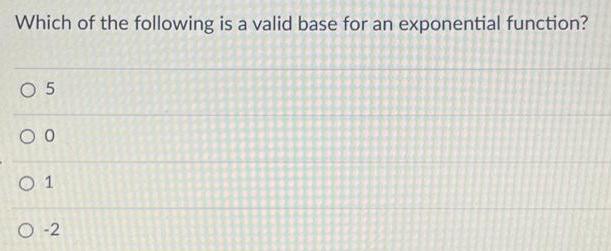
Complex numbersWhich of the following is a valid base for an exponential function 05 00 0 1 O 2

Algebra
Complex numbers1 Solve for x X 2 0 3 4 3x 1 x 5 If there is more than one solution separate them with commas If there is no solution click on No solution 8 5 X 0 0 No solution S 6
Algebra
Complex numbersTo offer scholarships to children of employees a company invests 13 000 at the end of every three months in an annuity that pays 10 compounded quarterly a How much will the company have in scholarship funds at the end of ten years b Find the interest i Click the icon to view some finance formulas a The company will have in scholarship funds Do not round until the final answer Then round to the nearest dollar as needed

Algebra
Complex numbersProof Let IxJ be arbitrary Using openness of IxJ choose 8 0 such that 1 8 4 8 x 8 x 8 IXJ Now the function is continuous on IxJ and therefore it is x bounded on the compact subset 10 8 0 8 x8 x 8 We consider any constant k 0 with the property of 1 3 K K for all t 1 8 8 and for all x x 8 x 8 Finally let 1 1 8 1 8 x y in 8 x 8 be arbitrary By the mean value theorem of differential calculus we have Of ft y f t x y x x for some z between x and y Therefore of fty f0 x 5 x 10 5K yx 1 2 K Ox Since 1 2 1 8 1 8 x 8 x 8 of 1 2z K K This proves the locally Lipschitz property of f Theorem 1 Emil Picard If f 1xJ R is locally Lipschitz then for any 1 EI x EJ the initial value problem df f 1 x x to xo dx has a solution x 6 8 16 8J for some 8 0 and therefore Proof Choose 8 0 such that 1 8 1 8 1 8 x 8 J and there exists K 0 for which f1 x f t y Kx y holds for all 1 8 8 x 8 x 8 We choose M 0 such that f 1 x M for all te t 8 8 xe x 8 x 8 Using the constants 8 0 K 0 M 0 chosen above we choose one more constant 77 satisfying 0 n min We define a sequence of functions KM no 1 R kEZ recursively as follows
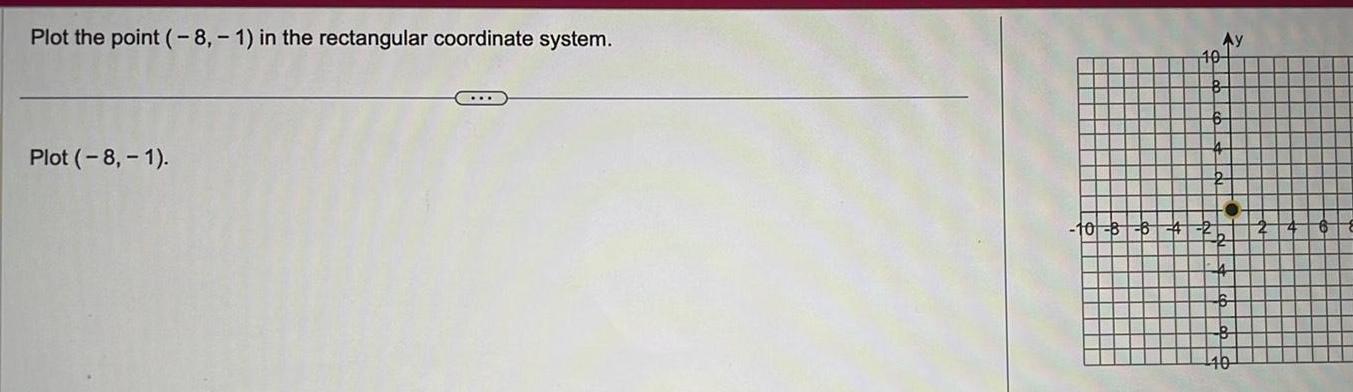
Algebra
Complex numbersPlot the point 8 1 in the rectangular coordinate system Plot 8 1 10 B 6 10 B 2 Ay 6 40

Algebra
Complex numbersAbout the sequence x keZ we have the following a x 1 EB xo b for each te to b to b MKk b x x te t b t b k20 k 1 c xk Z is uniformly Cauchy on t b t b verification of these properties is left for the reader We consider the uniform limit of the sequence x t 8 to 8 B x b C which is given by x t lim x 1 1E1 b t b Thus x 1 lim x f s x s ds Finally the validity of this integral equation has the following two implications 1 x lim ff s x s ds x S lim f s x s ds x f f s lim x s ds x f f s x s ds k 400 Thus the function tx 1 satisfying the integral equation x 1 x f s x s ds for all t 1 b t b 2 X 1 x dx dt s x s ds s ds x 0 xo 0 as ff s x s ds f t X 1 This now proves that the curve X 1 8 to 82 thus obtained is a solution of the initial value problem

Algebra
Complex numbers1 x 1 the right hand sides are independent of t and therefore 1 1 Ouniformly on 1 1 1 as k p being arbitrary This completes the proof of our claim that the sequence x k of functions is uniformly Cauchy on to to n j2k Using this last mentioned property of the sequence xk Z we define a function x 10 11 10 11 8 8 by putting x t lim x t t to to n The function x thus defined k x is the uniform limit of the sequence x KEN Therefore we have x t lim x 1 lim x f s X s ds 61 10 x limff s x s ds S lim f s x s ds ff s lim x s ds x f s x s ds All the above equations being valid because of the uniform convergence of of x fox on to 14 1 Finally the identity x t x f f s x s ds t t 1 1 derived above has the following two consequences 1 Differentiation of the identity implies
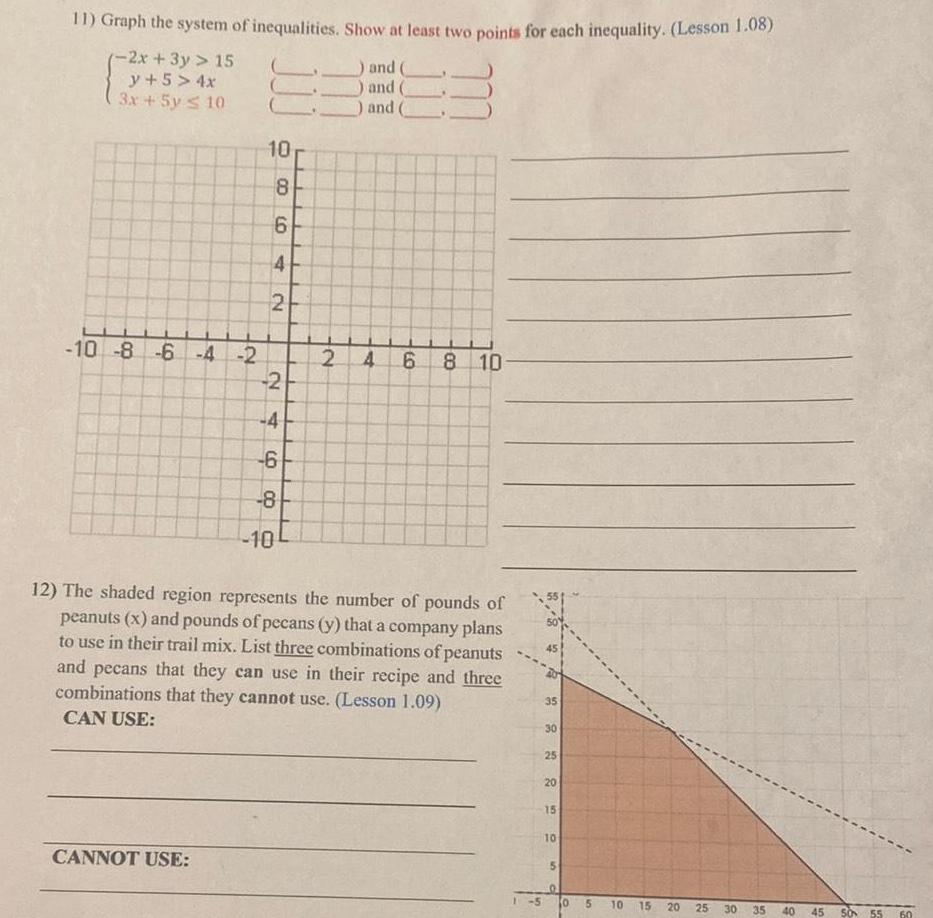
Algebra
Complex numbers11 Graph the system of inequalities Show at least two points for each inequality Lesson 1 08 2x 3y 15 y 5 4x 3x 5y 10 E 10 8 6 4 2 10 8 CANNOT USE 6 4 2 246 8 10 2 and and and 4 777 6 8 10 12 The shaded region represents the number of pounds of peanuts x and pounds of pecans y that a company plans to use in their trail mix List three combinations of peanuts and pecans that they can use in their recipe and three combinations that they cannot use Lesson 1 09 CAN USE 422222 45 35 30 25 20 15 10 5 0 5 0 5 10 15 20 25 30 35 40 45 50 55 60

Algebra
Complex numbersWhat is the degree of the polynomial 5pq2 3p6 5p6q 6p q5 Enter your answer in the box


Algebra
Complex numbersWhat is a y intercept of a graph Choose the correct answer below OA A y intercept of a graph is the y coordinate of a point where the graph intersects the y axis OB A y intercept of a graph is the y coordinate of a point where the graph intersects the x axis OC A y intercept of a graph is the x coordinate of a point where the graph intersects the x axis OD A y intercept of a graph is the x coordinate of a point where the graph intersects the y axis
Algebra
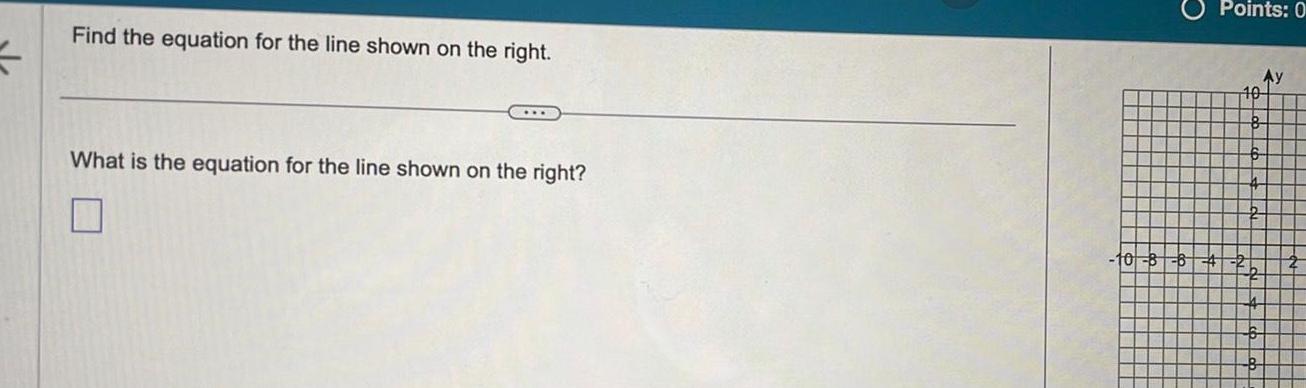
Complex numbers5 Find the equation for the line shown on the right What is the equation for the line shown on the right Points 0 Ay 10 10 8 84 2

Algebra
Complex numbersEvaluate 4x 5 y 1 when x is 2 less than the quotient of y and 4 and y 16 4x 5 y 1 Simplify your answer