Complex numbers Questions and Answers

Algebra
Complex numbersGive the slope and the y-intercept of the line y = x + 7. Make sure the y-intercept is written as an
ordered pair.
Slope =
y-intercept =
Algebra
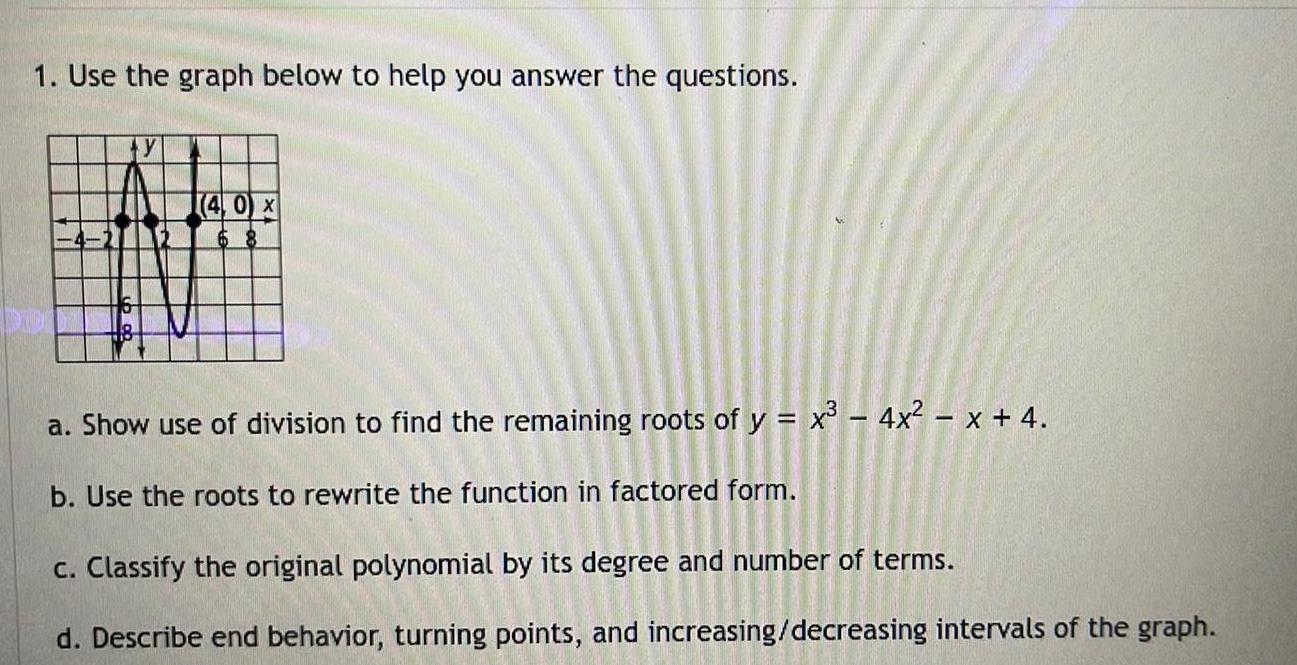
Complex numbersUse the graph below to help you answer the questions.
a. Show use of division to find the remaining roots of y = x³ - 4x² - x + 4.
b. Use the roots to rewrite the function in factored form.
c. Classify the original polynomial by its degree and number of terms.
d. Describe end behavior, turning points, and increasing/decreasing intervals of the graph.

Algebra
Complex numbersMaurice says: "Every linear function has exactly one zero. It follows from the Fundamental Theorem of Algebra." Cheryl disagrees. "What about the linear function y = 2?" she asks. Whose reasoning is correct?
Select one:
a. Maurice
b. Cheryl
c. not enough information

Algebra
Complex numbersChoose the property illustrated by the following statement.
0 +6=6
A. additive inverse property
B. associative property of addition
C. commutative property of addition
D. identity element of addition


Algebra
Complex numbersFind all points having a y-coordinate of - 6
whose distance from the point 11, 22 is 17.
(a) By using the Pythagorean Theorem.
(b) By using the distance formula.

Algebra
Complex numbersWhich value is NOT a solution to the equation? Choose all that apply.
27= -x4 - 12x²
Select one or more:
a. 0
b. 3i
c. İ√3
d. 3
Algebra
Complex numbersPadraig is a financial advisor. He earned a salary of $80,000 last year and sold stocks for $5,000. Which of the following types of income did Padraig have?
I earned income
Il passive income
III capital gains income
a. I and II
b. I and III
c. II and III
d. III

Algebra
Complex numbersUse a calculator to identify the following for the parabola: y = x² + 2x + 1
a. vertex
b. axis of symmetery
c. maximum or minimum value
d. domain
e. range
Algebra
Complex numbersEnter the appropriate word or phrase in each blank. (Use^ to denote an exponent. Do not enter spaces in your answer. Enter the
simplified expressions in parts b and d.)
a. The expression (a + b)² is called the
Write your response here... of a binomial.
b. When simplified, (a + b)² is equivalent to the expression
c. This resulting trinomial is called a
d. For example, (x+4)²-

Algebra
Complex numbersFind the inverse for each of the following one-to-one functions.
(a) f(x) = 2x + 7
f-¹(x) =
(b) f(x) = -x-4/4x + 1
f-¹(x) =
(c) f(x)=√x-1, x ≥ 1.
f-¹(x) = x ≥

Algebra
Complex numbersTo measure the area of a quadrangle, two students decided to walk along two sides of the rectangular lot, starting at the same corner at the same time. If student A walks at 2 paces per second and student B walks at 3 paces per 2 seconds, how far are they separating from each other after 8 seconds?

Algebra
Complex numbersConsider sec(-(2 + 3x)) = √2.
The principle solution is x =
We now determine all solutions for this problem.
What is the period of secant?
List all values of 0 in the interval [0, 2x) such that sec(0) = √2.
(Notice the relationship between the period and the interval here.)
(List all values in this answer box separated by a comma. Depending on the trig function and value, it is possible that there will only be one entry.)
Thus, using the above work, all solutions are given by
x=_ where k € Z.
(There will be a separate formula for each value you listed in the previous answer box. List each formula in this answer
box separated by a comma. Remember to use k as appropriate.)
Algebra
Complex numbersGive all transformations that occur to the function y=x that produce the function y=2|x|-3. Give both
the transformations and the order in which they occur.


Algebra
Complex numbersWrite the expression in the standard form a + b i.
(5+3i)-(9-8i)
(5+3i)-(9-8i) = (Simplify your answer.)

Algebra
Complex numbersWrite the expression in the standard form a + bi.
(4-8 i) + (9+3i)
(4-8 i) + (9 +3i) = (Simplify your answer.)


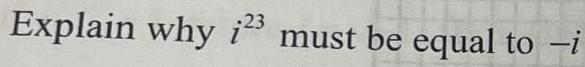

![Let L1 be the line passing through the point
P1=(-10, 5, 11) with direction vector →d1=
[−2, 3, 2]T, and let L2 be the line passing
through the point P2=(-34, 13, -3) with
direction vector →d2=[2, -5, 0]T. Find the
shortest distance d between these two
lines, and find a point Q1 on L1 and a point
Q2 on L2 so that d(Q1,Q2) = d. Use the
square root symbol 'V' where needed to
give an exact value for your answer.
d=
Q1=
Q2=
d = 4√33
Q₁=(-4,-4, 5)
Q2 = (-24, -12, -3)
Above is the answer, however, I am not
sure how to get to these solutions.](https://media.kunduz.com/media/sug-question/raw/83028704-1660232868.8074205.jpeg?w=256)
Algebra
Complex numbersLet L1 be the line passing through the point
P1=(-10, 5, 11) with direction vector →d1=
[−2, 3, 2]T, and let L2 be the line passing
through the point P2=(-34, 13, -3) with
direction vector →d2=[2, -5, 0]T. Find the
shortest distance d between these two
lines, and find a point Q1 on L1 and a point
Q2 on L2 so that d(Q1,Q2) = d. Use the
square root symbol 'V' where needed to
give an exact value for your answer.
d=
Q1=
Q2=
d = 4√33
Q₁=(-4,-4, 5)
Q2 = (-24, -12, -3)
Above is the answer, however, I am not
sure how to get to these solutions.

Algebra
Complex numbersFinancial Markets. The tock and bond markets are not the only
financial markets. Give two or three additional examples.
![Solve cos^2(x) = 3 sin(x) for all solutions x ∈ [0,2π].](https://media.kunduz.com/media/sug-question/raw/78724832-1660228671.6855006.jpeg?w=256)

Algebra
Complex numbersWhich of the following explains why f(x) = log4x does not have a y-intercept? Check all that apply.
There is no power of 4 that is equal to 0.
There is no power of 4 that is equal to 1.
Its inverse does not have any x-intercepts.
Its inverse does not have any y-intercepts.

Algebra
Complex numbersSolve for x and check for extranepus roots.
(a) √x-1=1
x =
(b) √x - 2 = x - 2
X =
(c) √x + 6 = x
X =
(d) √3x + 16 = −8+√4x + 116
x =
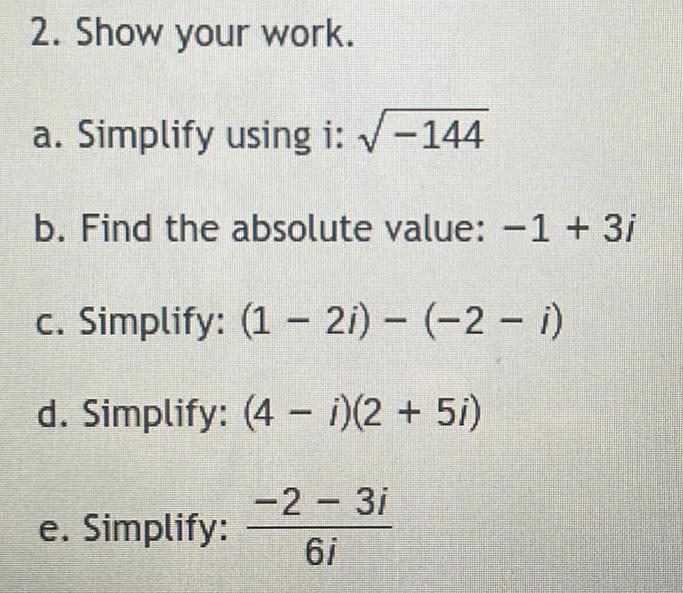
Algebra
Complex numbers2. Show your work.
a. Simplify using i: -144
b. Find the absolute value: -1 + 3/
c. Simplify: (1-21)-(-2-i)
d. Simplify: (4- i)(2 + 5i)
e. Simplify:
-2-3i/6i

Algebra
Complex numbersNewton's Law of Cooling states that for a cooling substance with initial temperature To, the temperature T after t minutes can be modeled 0' by T = (To-T)e+T, where T is the surrounding temperature and r is the cooling rate of the substance. You remove a baked potato from a 400°F oven and place it on the counter in your 67°F kitchen. After 20 minutes, you measure the temperature of the potato to be 128°F. What will the temperature of the potato be after 26 minutes?
about 104°F
about 69°F
about 113°F
about 37°F


Algebra
Complex numbersWhat does the notation Ry-3x+1(DAB) mean?
Reflection
Rotation
Clockwise
Counter-clockwise
Around the origin to the axis
Over the x-axis
Over the y-axis
Over y-3x+1
180°
90°
270°

Algebra
Complex numbersDivide and express the result in standard form.
5i/2-7i
5i/2-7i
(Simplify your answer. Type your answer in the form a +bi. Use integers or fractions for any numbers in the expression.)

Algebra
Complex numbers.Find a general solution to
y" - 2y + 1y =12.5elt/t² + 1
Use a and b for the constants of integration associated with the homogeneous solution.
y =

Algebra
Complex numbersGiven the function h(x)=x²-7x³ +14x² -38x-60 has a zero of 1+3i, find the remaining zeros of the function (using algebra!). Write the function in factored form over the complex number system.
Zeros:__________________
Factored:______________

Algebra
Complex numbersEnter the expression or number that is required to solve the equation. Do not enter any blank spaces in your answers.
2(1 – 5x) = 72
a. Apply the distributive property: Write your response here...
b. When the constant term is moved to the right side, the resulting equation is:
c. Solve: X =
d. Solution set:
Algebra
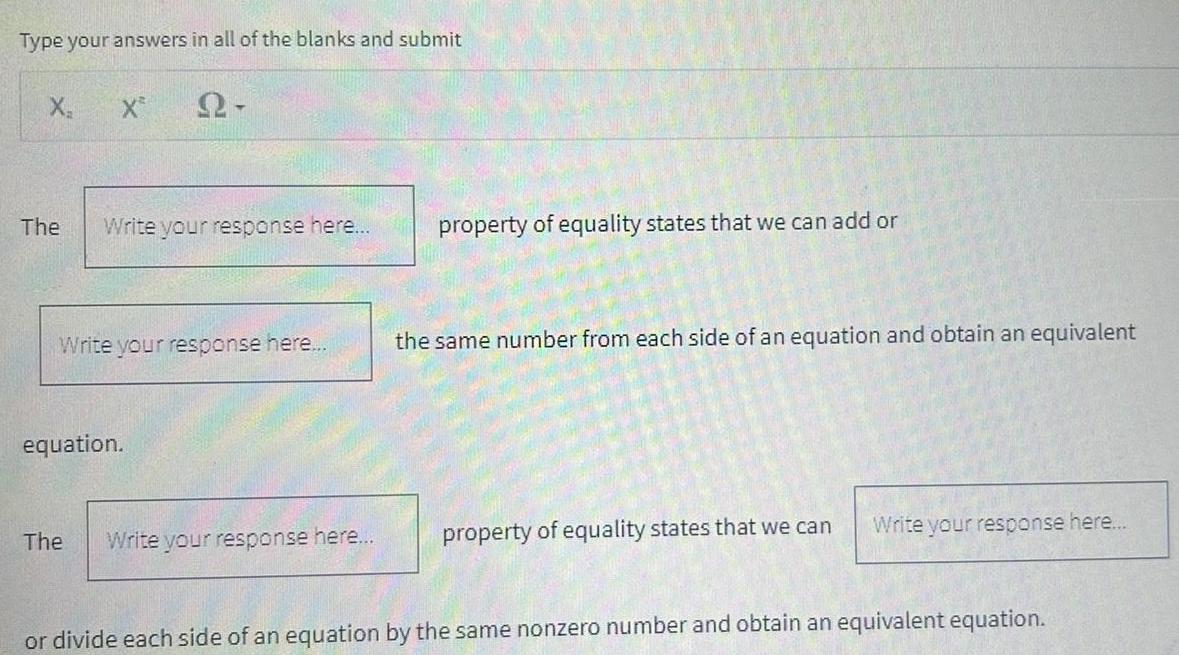
Complex numbersThe property of equality states that we can add or the same number from each side of an equation and obtain an equivalent equation.
The property of equality states that we can or divide each side of an equation by the same nonzero number and obtain an equivalent equation.

Algebra
Complex numbersSolve the equation. Enter the solution set below. Recall that solution set notation is written in the form {p}, where P is the solution of the equation. (For example, if 2 is a solution, the solution set is {2}.)
-4 = 38 + 6x

Algebra
Complex numbersQuestion 36 (1 point) At a sandwich shop, 42% of customers buy a drink with their sandwich. Only 28% of customers buy a drink and chips with their sandwich. What is the probability that a customer who buys a drink also buys chips?
a)70%
b)about 66.7%
c)14%
d)1.5%
Algebra
Complex numbersAndy and Cheyenne are planning to have their wedding and reception at a local venue. The venue charges a rental fee of $2030.
The menu they have selected costs $36.75 per person.
a. If they have $7,500 saved for their reception, what is the maximum number of guests that can attend the reception without going over budget?
b. If, in addition to the meal, the couple wants to provide a beer and wine selection at the reception, there is an additional charge of $19.50 per person. In this case, what is the maximum number of guests that can attend the reception without going over budget?

Algebra
Complex numbersIf a linear equation is an identity, what is its solution set?
Select an answer and submit. For keyboard navigation, use the up/down arrow keys to select an answer.

Algebra
Complex numbersLet f(x) = - 3x + 7. Find each of the following:
f(a) =
2f(a) =
f(2a) =
f(a + 2) =
f(a) + f(2)=

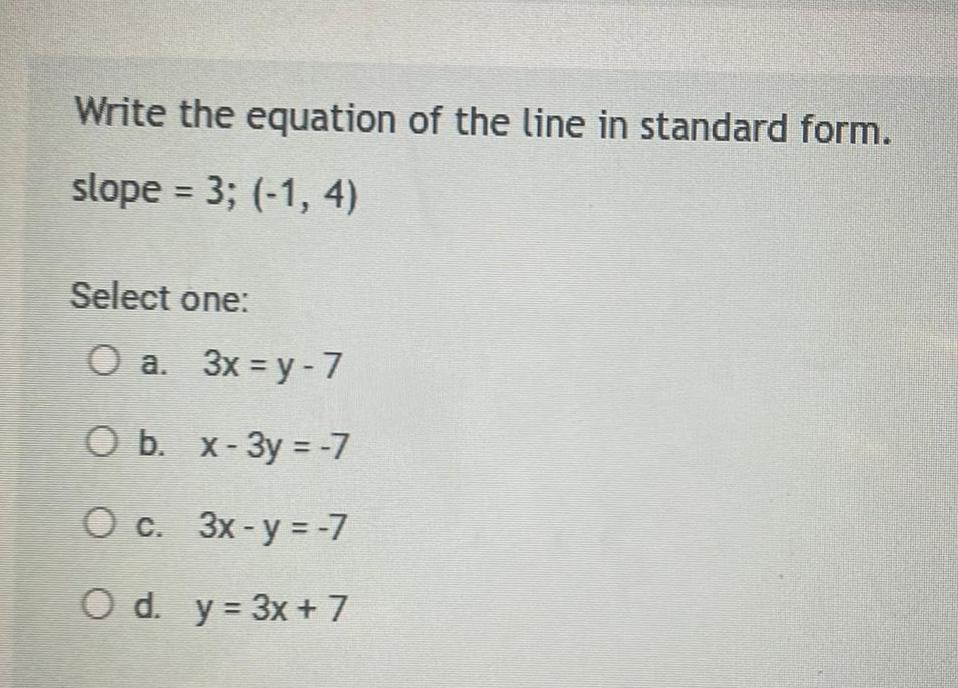
Algebra
Complex numbersWrite the equation of the line in standard form.
slope = 3; (-1, 4)
Select one:
a. 3x=y-7
b. x-3y = -7
c. 3x-y = -7
d. y = 3x +7

Algebra
Complex numbersDarnell invested a total of $52,000 in two accounts. The first account earned 13% after one year.
However, the second account suffered a 5% loss in the same time. At the end of one year, the total
amount of money gained was $2,620.
LA
at 13%
at 5%

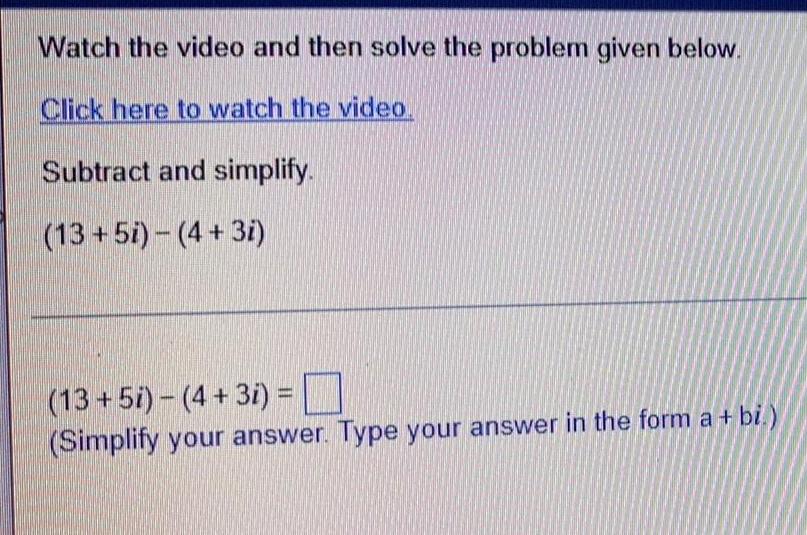
Algebra
Complex numbersSubtract and simplify.
(13+5i)-(4+3i)
(13+5i)-(4+3i) =
(Simplify your answer. Type your answer in the form a + bi.)
![Express 9[cos(60°) —isin(60°)] in the form a+ bi](https://media.kunduz.com/media/sug-question/raw/84973368-1660149287.5194259.jpeg?w=256)

Algebra
Complex numbersDetermine whether each of the following mapping is a homomorphism (justify your
answer). If yes, find its kernel.
i. Define : Z → R by p(x) = x. (where Z and R are both groups under addition).
ii. Let(G,*) be a group under a binary operation and let y: GG be defined by
y(m) = m² for all meg.
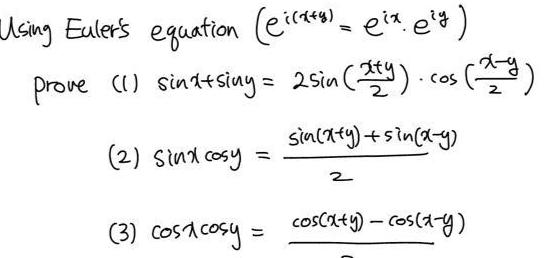
Algebra
Complex numbersUsing Euler's equation (eixes) eix e¹g)
prove (1) sini+sing = 2 Sin (x+y). cos (x-4)
sin(x+y)+sin(x-y)
(2) Sinx cosy
(3) cost cosy = =
cos(x+y) - cos(x-y)
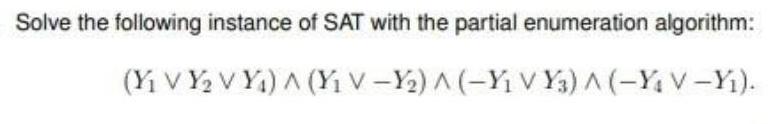
Algebra
Complex numbersSolve the following instance of SAT with the partial enumeration algorithm:
(Y₁ VY₂ VY₁) A (Y₁ V-Y₂) A(-Y₁ VY3) A(-Y₁ V-Y₁).