Permutations and Combinations Questions and Answers

Algebra
Permutations and CombinationsUse the table below to write the Mayan numeral as a Hindu Arabic numeral 5 10 15 6 11 16 O 19 O 10 7 O 14 12 17 3 8 13 18 g 14 19

Algebra
Permutations and Combinations5 Kathy plans to purchase a car that depreciates at a rate of 12 per year The initial value of the car is 21 000 Use an exponential function to find the value of the car after 3 years

Algebra
Permutations and CombinationsThus x t lim xo f s x s ds Finally the validity of this integral equation has the following two implications X 1 x f s x s ds x 0 xg 1 x lim ff s x s ds x S lim f s x s ds x f f s lim x s ds x ff s x s ds Thus the function rx 1 satisfying the integral equation x 1 x f f s x s ds for all 1 t b 1 b 2 dx dt 0 ff s x s ds f 1 X 1 This now proves that the curve X 1 8 82 thus obtained is a solution of the initial value problem

Algebra
Permutations and CombinationsChus 360 1 4 1 1 4 1 4 2 2 30 2 3 2 9 91 233723 2 1 3 3ds 3 3r 3 3t 1 5 1 2 x 1 300 2 6 8s gi 91 441 11 1 32 33 8 4 91 sds 1 3 91 44 19 44 2 3 62 3 2 22 3
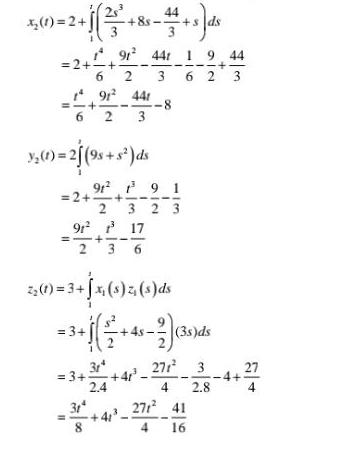
Algebra
Permutations and Combinationsx 1 2 2 2 3 6 2 191 441 62 3 3 3t 3 8 91 44 19 44 3 62 3 3 1 2 9s s ds 91 2 91 1 17 2 5 2 4 I 3 6 2 1 3 x s z s ds 41 191 32 3 I 44 4s 4 3s ds 27 3 4 2 8 271 41 4 16 4 27
Algebra
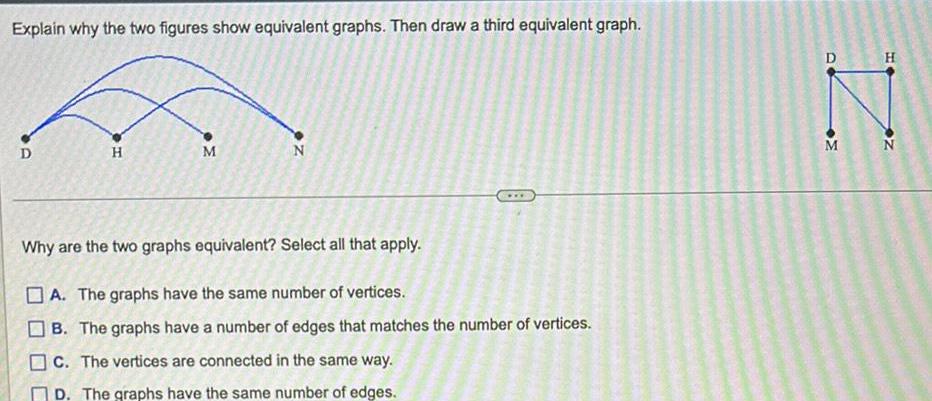
Permutations and CombinationsExplain why the two figures show equivalent graphs Then draw a third equivalent graph D H M M Why are the two graphs equivalent Select all that apply A The graphs have the same number of vertices B The graphs have a number of edges that matches the number of vertices c The vertices are connected in the same way D The graphs have the same number of edges D M H N
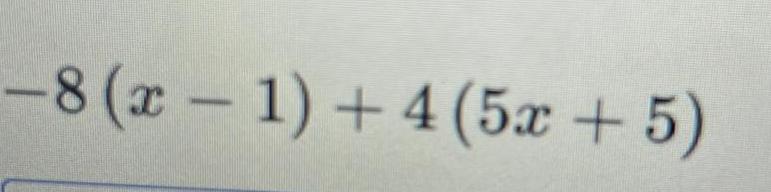
Algebra
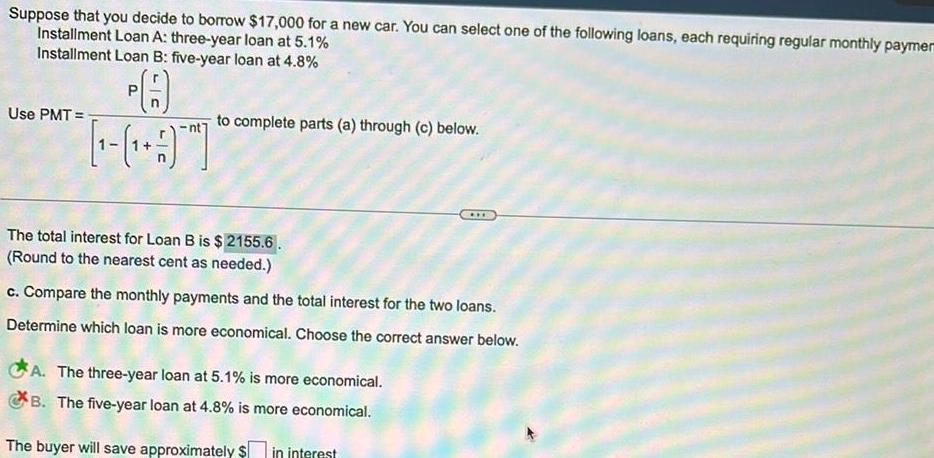
Permutations and CombinationsSuppose that you decide to borrow 17 000 for a new car You can select one of the following loans each requiring regular monthly paymen Installment Loan A three year loan at 5 1 Installment Loan B five year loan at 4 8 PA Use PMT to complete parts a through c below The total interest for Loan B is 2155 6 Round to the nearest cent as needed c Compare the monthly payments and the total interest for the two loans Determine which loan is more economical Choose the correct answer below A The three year loan at 5 1 is more economical B The five year loan at 4 8 is more economical The buyer will save approximately in interest

Algebra
Permutations and CombinationsConsequently the DE 3 is actually the following system of ODE in the functions t X t t X t t X t d dX dk X 1 X 5 X dik dt dk X dik 12 1 X 4 dk X di di 1 d x 1 1 x dx d dX dik 1 X 1 dX dx dt d X dik t At this stage we become more specific about the features of the ODE 3 or equivalently about the system 4 Let I be an open internal and let denote an open subset of R We consider the open sets IxNxR x xR there being k 1 copies of R in the above Cartesian product This set is being designated to accommodate the variable quantities dk X di 1 dX dt 4 Clearly the function f appearing on the right hand side of 3 must have this set as its domain of definition Choosing 1 1 x En and w w win R we form the initial condition fo W W w Now the initial value problem for the ODE 3 is the following pair dk X di 1 ff x ToX W W 1 5 By a solution of the initial value problem 5 we mean an at least k mously diffamanti 01
Algebra
Permutations and CombinationsTo begin with we reorganize the form 1 of the ODE in the following manner Unraveling it we separate the top order derivative and express it as a function of the remain variable quantities namely dX d X t X 1 that is we dt di form the equation ft X dX d x dt di d X di We regard the equation 3 as the standard form of an ODE of course the ODE has order k Note that the function X t X t is a vector valued function of the real variable t and as such it is a curve in R Each X t has n components X t X 1 X 1 X 1 and therefore all the derivative of it has n components d X 1 X 1 X dt X for 1 k Consequently the function f appearing on the right hand side of 3 has n components f f ff each f being a real valued function MATHEMATICS Consequently the DE 3 is actually the following system of ODE in the functions t X t t X X t da X dik 1 ft X d X dik f 1 X d X dik dX dt f t X dx t X t dt 1 d X dik dX dt dx dX dt dit 1 d X di 1 At this stage we become more specific about the features of the ODE 3 or equivalently about the system 4 dit 1 3 3 dk X dik 1 Let I be an open internal and let denote an open subset of R We consider the open sets IxNxR x XR there being k 1 copies of R in the above Cartesian product This set is being designated to accommodate the variable quantities 4 Clearly the function f appearing on the right hand side of 3 must have this set as its domain of definition dX dk X To W W 1 Choosing t 1 x En and www in R we form the initial condition o W WW Now the initial value problem for the ODE 3 is the following pair 5
Algebra
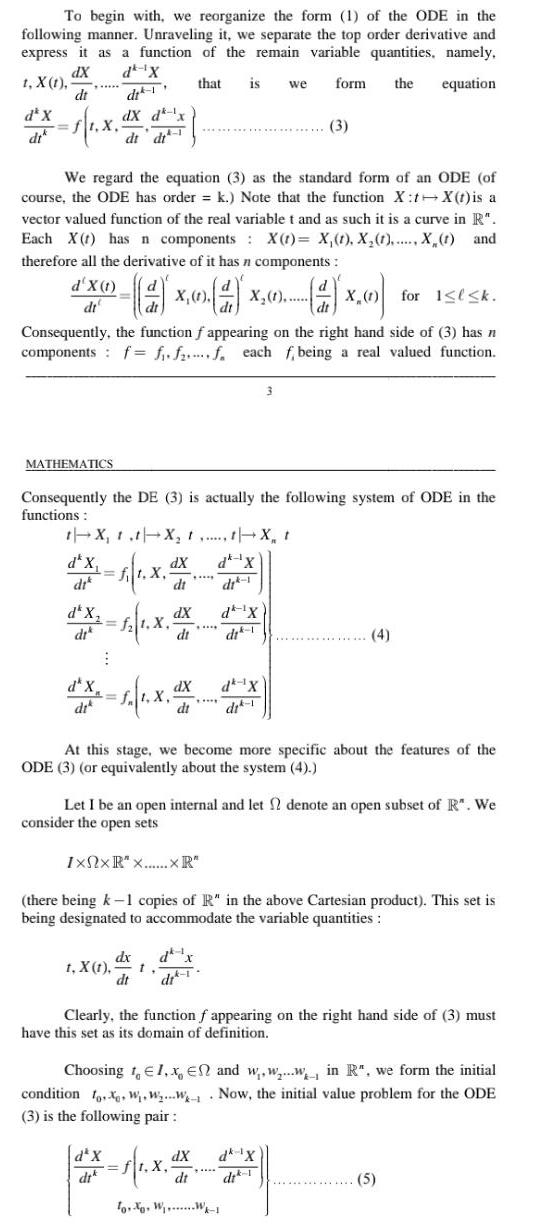
Permutations and CombinationsWhich description is correct for the polynomial 3x 4x 7 O cubic binomial O cubic trinomial O quartic trinomial O quadratic trinomial

Algebra
Permutations and CombinationsWe begin some more generalities related to first order ODE As in the preceding part I denoted an open interval and 2 an open subset of R We consider a function f Ixn R giving rise to the first order ODE dX dt f t x dX dt Note that for each 1 EI held fixed the map ft R x f 1 x is a vector field on Interpreting as the time variable we call the function 7 a time dependant vector field on n And often we call a solution X J of the ODE 8 an integral curve of the vector field f An initial condition for the ODE 8 consists of a pair too with to El x En and the associated initial value problem is f t X x t Xo X J N Finally recall that a solution of 9 is an at least once continuously differentiable curve J being an open interval with 1 EJ CI satisfying dX 1 dt 7 f t X t for all tEI and the initial condition X to x MATHEMATICS 8 In the context of the IVP 9 we consider yet another equation the following integral equation in an unknown function X J X 1 Xo f s x s ds tEJ 10 Proposition 1 Following result relates solutions of the IVP 9 and those of the integral equation 10 J s X s ds 9 X to x A continuously differentiable curve X J J being an open subinterval of I with 1 EJ is a solution of the IVP 9 if and only if it satisfies the integral equation 10 Proof I First suppose that the curve X J satisfies the integral equation 10 Putting 1 1 in 10 we get 0 X f s X s ds Thus X satisfies the initial condition Next differentiating 10 we get dX t d 0 f s X
Algebra
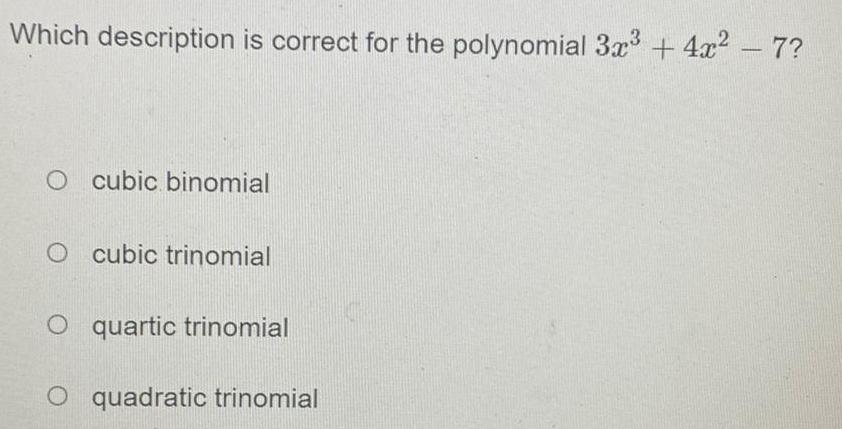
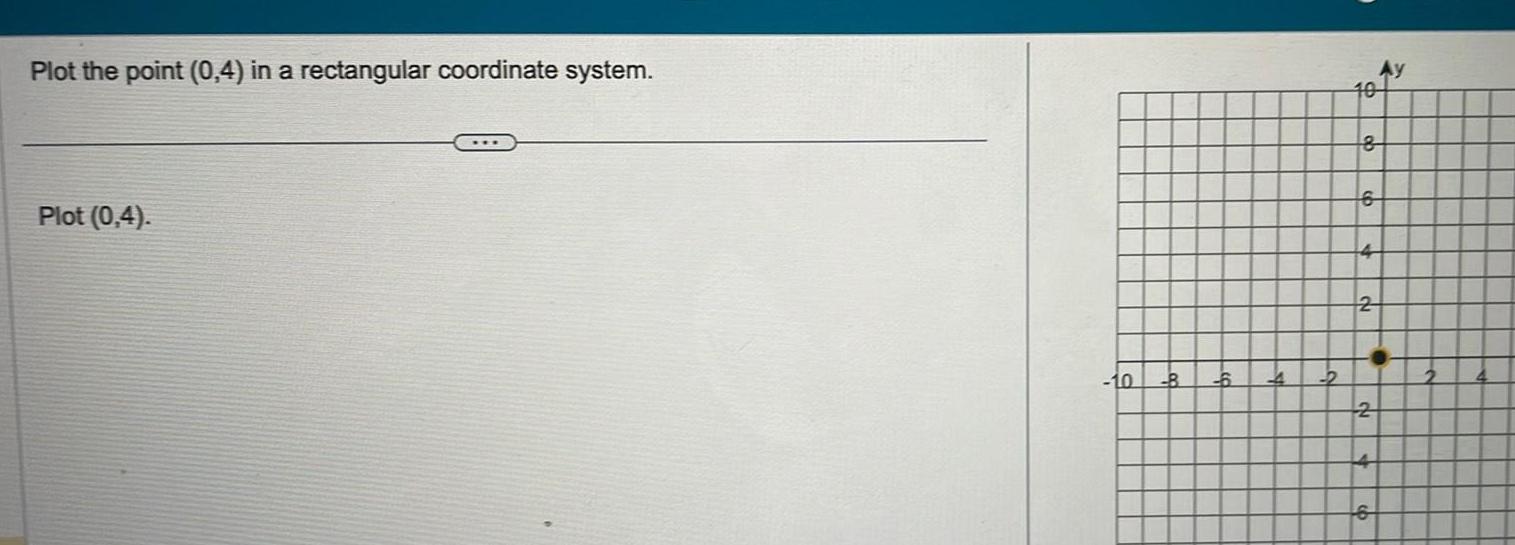
Permutations and CombinationsPlot the point 0 4 in a rectangular coordinate system Plot 0 4 10 6 2 10 8 6 4 2 2 Ay 6

Algebra
Permutations and Combinations1 5 1 2 x y oldu una A 7 1 2 g re x y toplam ka t r B 1 12 D 7 1 2 4 4 C 4 E 47
Algebra
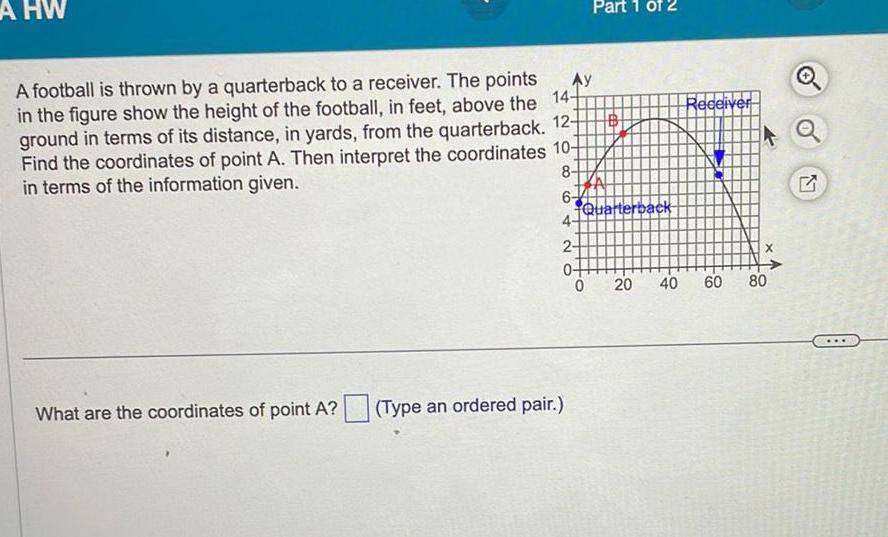
Permutations and CombinationsA HW A football is thrown by a quarterback to a receiver The points in the figure show the height of the football in feet above the ground in terms of its distance in yards from the quarterback 12 Find the coordinates of point A Then interpret the coordinates 10 in terms of the information given 14 8 What are the coordinates of point A Ay 6 4 Type an ordered pair Part 1 of 2 2 0 0 Quarterback Receiver 20 40 60 X 80 G

Algebra
Permutations and CombinationsPlot 4 3 on the coordinate axes Plot the point 4 3 on the graph to the right Next question 10 8 B 4 10 e 2 Ay
Algebra
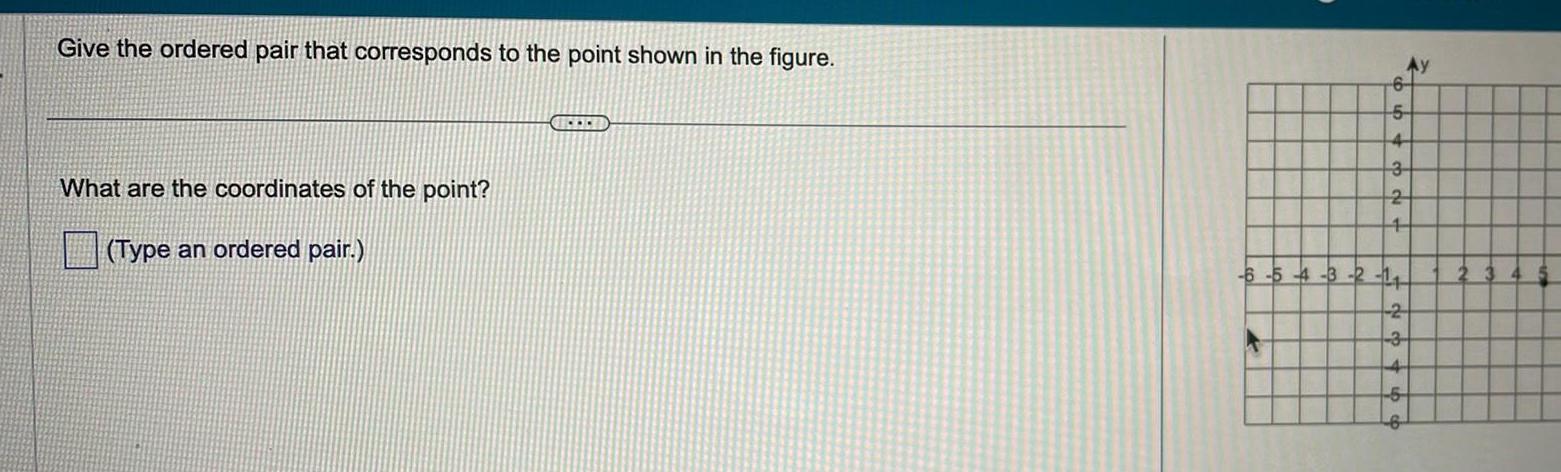
Permutations and CombinationsGive the ordered pair that corresponds to the point shown in the figure What are the coordinates of the point Type an ordered pair Z 6 5 4 3 2 1 6 5 4 3 2 2 3 4 6
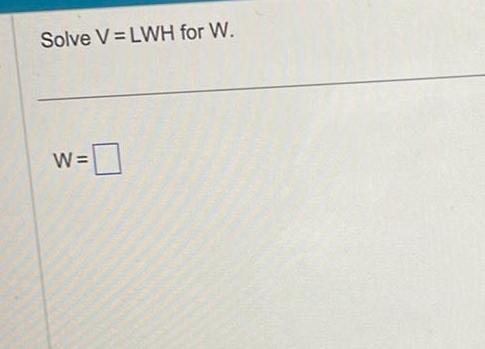
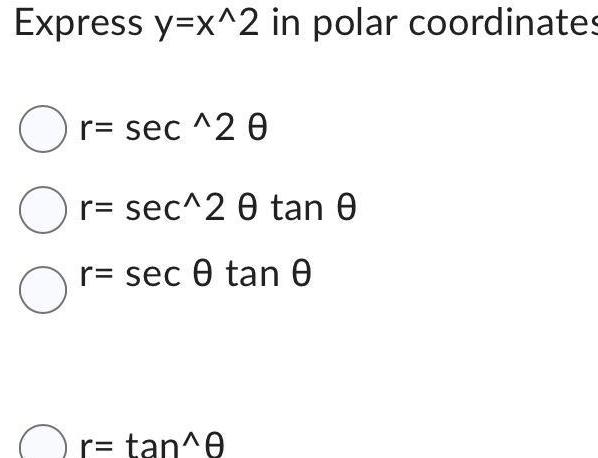
Algebra
Permutations and CombinationsExpress y x 2 in polar coordinates O r sec 2 0 Or sec 2 0 tan 0 r sec 0 tan 0 O r tan e

Algebra
Permutations and Combinationse Fiesch Reading Fase Test is a test that ranks the reading level of passagest Let W be the total number of words in the passage S the number of sentences and the number of syllables Then he Fiesch test calculates the score of the passage using the formula F 206 835 1015 846 a Calculate for the paragraph above the one that describes the Fleich Reading Ease Test Omit the formula itself Round your answer to the nearest whole number We count 3 sentences 51 words and 71 sylables b for the rest of this exerse we consider passages that have 10 sentences and 400 sylaties Find a formula expressing as a function of w for such passages 846 400 206 835 1015 10 Assage thought to be easily understandable by 13 to 15 year old students of the resch score rounded to one decimar place is 60 or higher For the passages considered in part b how many words should be in the passage to make it easily understood bv 13 to 15 year old students Suppestion First use a table increment of 20 to get an estimate of the answer Then change to lable increment of L 1 What is the maximum number of words in the passages considered in part b Can there be more words than syllables 400 wants 4 Bear in mind your answer from part 0 and ortering

Algebra
Permutations and CombinationsConsider the equation P 2Q 3 This equation represents a supply Instructions Enter your answer as a whole number Use a negative sign if necessary This curve has a slope of Instructions Use the Line tool to draw the line by plotting the y intercept and the price when the quantity is 5 2 points total Price 15 14 13 12 11 10 9 43210 8 76543 curve 2 Tools Line

Algebra
Permutations and CombinationsThe perimeter of a college athletic field is 92 meters and the length is 18 m more than the width Find the length and width What is the width m o D

Algebra
Permutations and Combinations5 Extra Credit What is your favorite movie and why is it all three Lord of the Rings movies with the extended edition and cut content

Algebra
Permutations and Combinationsb Steven looks at the top of the lat pulldown machine with an angle of elevation being 50 degrees He is 2 feet away from the machine what is the height from his sight line to the handles

Algebra
Permutations and Combinations3 Let u and 7 be two unit vectors that are orthogonal Show that ar 7 7 are orthogonal too
Algebra
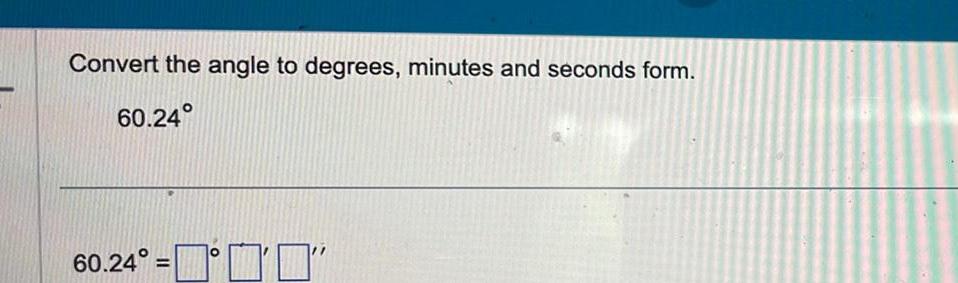
Permutations and CombinationsConvert the angle to degrees minutes and seconds form 60 24 60 24

Algebra
Permutations and Combinations15 Use your ruler and protractor to draw a triangle with one side 9 cm long and an adjacent angle measuring 45 Explain your method Can you draw a second triangle with the given measures that is not congruent to the first Sketch your results 16 Use your ruler and protractor to draw a triangle with one angle measuring 40 and an opposite side 10 cm long Explain your method Can you draw a second triangle with the given measures that is not congruent to the first Sketch your results

Algebra
Permutations and CombinationsThe midpoint of AB is M 0 2 If the coordinates of A are 1 3 what are the coordinates of B

Algebra
Permutations and CombinationsHow much water should be added to 10mL of 6 alcohol solution to reduce the concentration to 5

Algebra
Permutations and CombinationsFind the sine of ZA A 65 B 97 O Simplify your answer and write it as a proper fraction improper fraction or whole nu sin A C F

Algebra
Permutations and CombinationsAccording to her doctor Mrs Brooks s cholesterol level is higher than only 15 of the females aged 50 and over The cholesterol levels among females aged 50 and over are approximately normally distributed with a mean of 240 mg dL and a standard deviation of 20 mg dL What is Mrs Brooks s cholesterol level Carry your intermediate computations to at least four decimal places Round your answer to one decimal place mg dL X E O

Algebra
Permutations and CombinationsThe functions f and g are defined as f x 6x 5 and g x 1 6x a Find the domains of f g fg ff b Find fg x ff x The domain of The domain of H f g x and 1 when x and nd 7 1 Type your answer in interval notation 9 f 17 x is 0 17 4 17 00 7 x is 5 00 6 Type your answer in interval notation b fg x 36x 24x 5 Simplify your answer ff x 36x 60x 25 Simplify your answer H 0 g Simplify your appus
Algebra
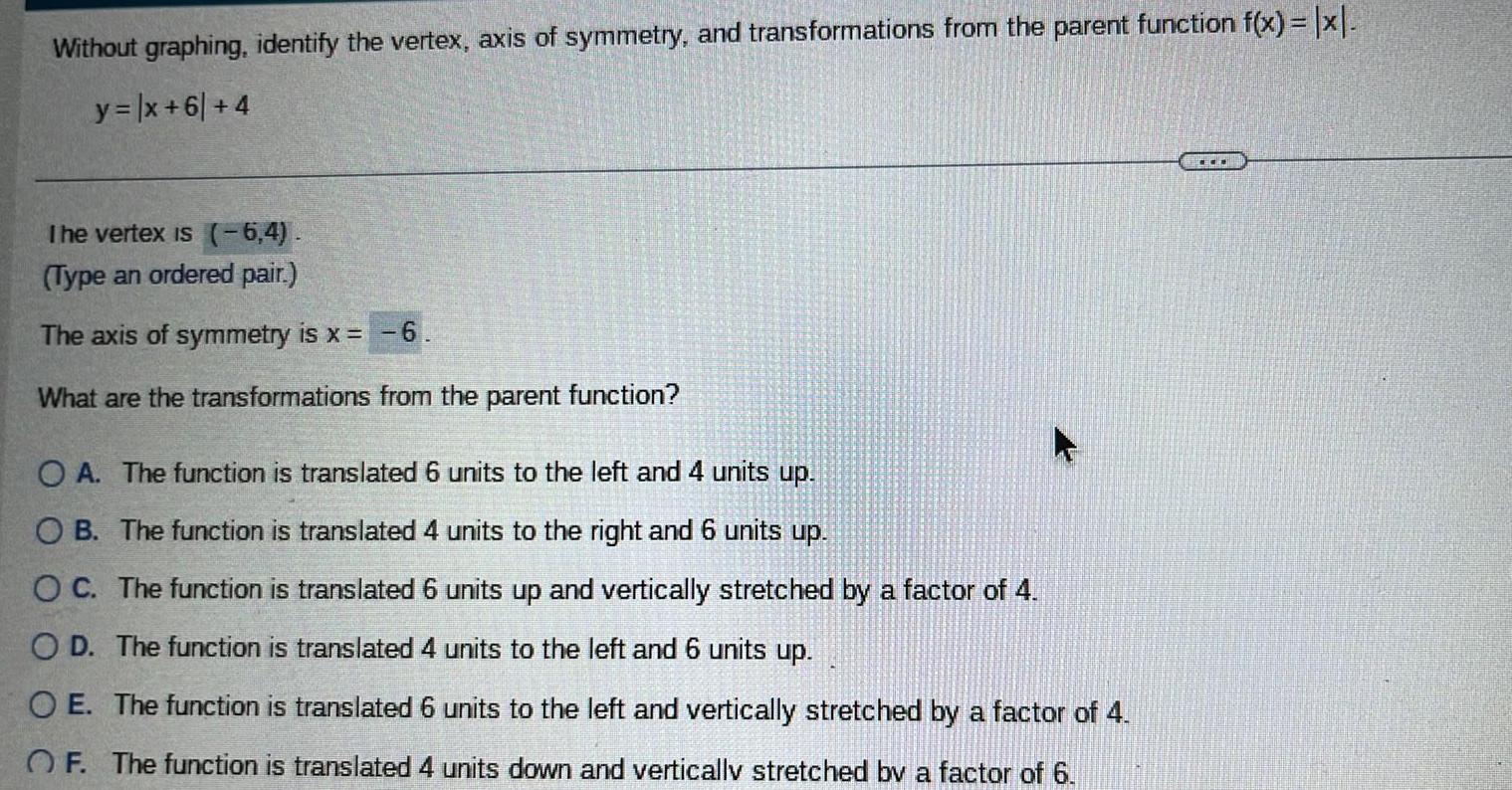
Permutations and CombinationsWithout graphing identify the vertex axis of symmetry and transformations from the parent function f x x y x 61 4 The vertex is 6 4 Type an ordered pair The axis of symmetry is x 6 What are the transformations from the parent function O A The function is translated 6 units to the left and 4 units up OB The function is translated 4 units to the right and 6 units up OC The function is translated 6 units up and vertically stretched by a factor of 4 OD The function is translated 4 units to the left and 6 units up OE The function is translated 6 units to the left and vertically stretched by a factor of 4 OF The function is translated 4 units down and vertically stretched by a factor of 6
Algebra
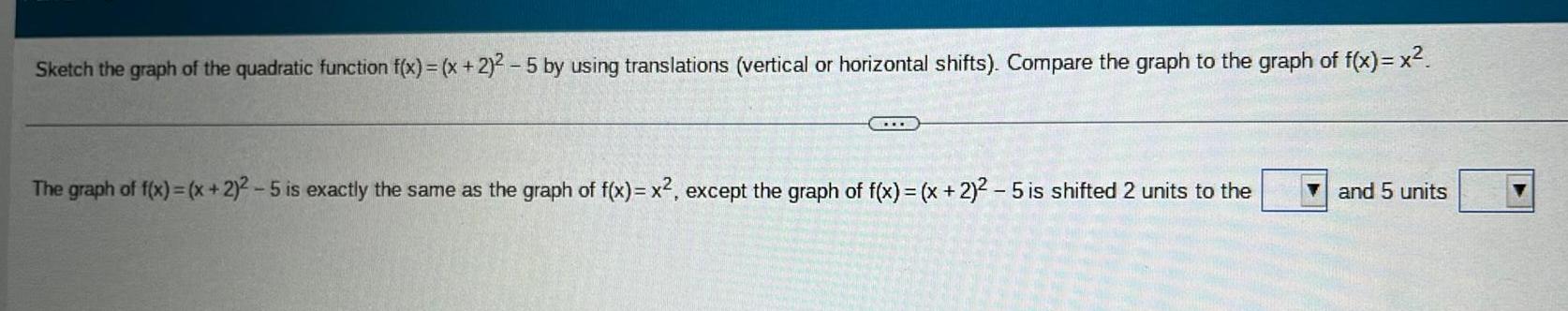
Permutations and CombinationsSketch the graph of the quadratic function f x x 2 2 5 by using translations vertical or horizontal shifts Compare the graph to the graph of f x x E The graph of f x x 2 2 5 is exactly the same as the graph of f x x except the graph of f x x 2 2 5 is shifted 2 units to the and 5 units

Algebra
Permutations and CombinationsBased on the table below find the range of f x 1 3 4 11 12 14 19 X f x 17 8 15


Algebra
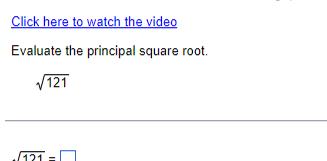
Permutations and CombinationsClick here to watch the video Evaluate the principal square root 121 121

Algebra
Permutations and Combinationslim f x x X X Left hand limit atx x lim f x lim f x h X lim f x x X X Continuity of Functions lim x h lim x h 2x h 20 x 0 0 RSITY OF DELE Since lim f x lim f x x Hence lim f x x Now the value of the function at x x is f x x from equation 1 and 2 we have lim f x f x x f x 0 Thus f x x is continuous at x x Since is an arbitrary element of R Therefore f x is continuous at each point of R Hence f x is continuous on R Example 4 Show that the function f x defined by Exfar if x 0 if x 0 is continuous on R Solution Given function is 1 xsin if x 0 X 0 if x 0 N

Algebra
Permutations and CombinationsI Q 2 I Q 3 Theorem 1 Statement Only A function f X R is said to be continuous at a point EX if and only if for given any E neighborhood V f x of f x there exist a 5 neighborhood V x of x such that if x is any point of XV then f x belongs to V f x is f XV x V f x 5 Discontinuity of a Function at a Point Let X be a non empty subset of R let f X R and let EX Then the function f x is said to be discontinuous at if and only if it is not continuous at x 5 1 Types of Discontinuity Let f be a function defined as f X R and let x EX and let left hand limit right hand limit limit of the function and the value of the function at xare denoted by lim f x lim f x lim f x and f x X A respectively Then 9

Algebra
Permutations and CombinationsProve the following using a proof by contrapositive For x yZ if xy2Z then x2Z or y2Z

Algebra
Permutations and Combinationsnot monotone Can you find a monotone one to one function that is not onto Or a monotone onto function that is not one to one Notes and Remarks

Algebra
Permutations and Combinations3 Raul has 56 bouncy balls He puts three times many balls into red gift bags as he puts into gr gift bags If he puts the same number of balls i each bag how many balls does he put into ea green bag

Algebra
Permutations and CombinationsIt r 0 then ri b therefore from 0 0 9 2 9 5 a 1 22 1 51 0 hot s a s b 5 a 69 from GCD a b 0 GCDa b exists It r to we repeat the process in finite steps n This process terminates In this any ex will arrive at vero remainde after th step we have sequence of integers of such that ostat recrub re n2 n23 p Shume To ser Foster rn b and on Wri n a

Algebra
Permutations and CombinationsPrime numbers An integer pyl which has no divisor except one and the number itself is called a prime number An integer p71 il called 7 prime number if it e not divisible by any other member except II and IP Ex 2 3 5 7 11 13 composte number Composite number an integer which i not a prime number 96 An integer axt called o called a composite number it it has a divisor other than II or a Ex 4 6 8 9 10
Algebra
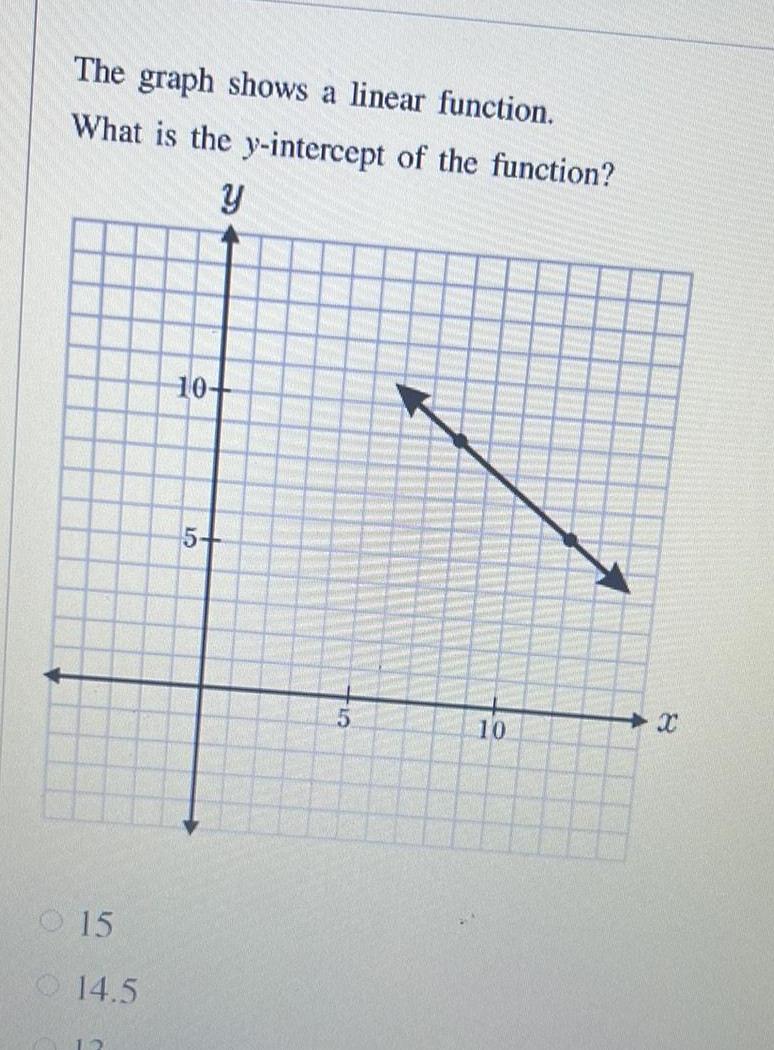
Permutations and CombinationsThe graph shows a linear function What is the y intercept of the function y 15 14 5 10 5 5 10 X

Algebra
Permutations and CombinationsFrom 6 we get 1 A k my By which gives 1 Ak and 1 N or A and B Hence 1 y k my ky Thus the given differential equation can be rewritten as 1 Bk m or ky k my dx for y0 and k my 0 In the integration of Eqn 7 the signs of y and k my play an important role We now discuss the following possible cases Case 1 y 0 and k my 0 0 y m For the case under consideration Eqn 7 can be expressed as d ny In k my m k k my my 1 7 which on integrating yields Iny In k my x c where c is a constant of integration The above equation can be further express as In y In k my x c 1 k exp x c k my Case II y 0 When y 0 then k my 0 because m 0 k 0 In this case Eqn 7 on integration can be written as In y In k my x c