Sequences & Series Questions and Answers
![Question (3): Let g(x) = 3-*, x = [1/3,1].
(a) Show that g(x) satisfies the Fixed Point Theorem.
(b) Find n such that |P-P| < 10-3 where P is the fixed point of g(x).](https://media.kunduz.com/media/sug-question/raw/84693866-1658947653.8468387.jpeg?w=256)
Algebra
Sequences & SeriesQuestion (3): Let g(x) = 3-*, x = [1/3,1].
(a) Show that g(x) satisfies the Fixed Point Theorem.
(b) Find n such that |P-P| < 10-3 where P is the fixed point of g(x).

Algebra
Sequences & SeriesThe Co. XYZ manufactures a product and sells it for $8 a unit. Its fixed costs are $5.000 and its Variable cost per unit is given by the equation. Calculate the amount q of total balance cost 2, 444 (X) = 2200
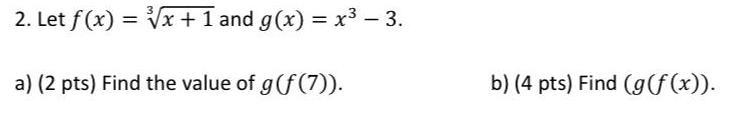
Algebra
Sequences & SeriesLet f(x) = 3√x + 1 and g(x) = x³ − 3.
a) Find the value of g (f (7)).
b) Find (g(f(x)).
![Multiple Choice: The optimal soil pH for growing corn is approximately 6.4. Find the optimal hydrogen ion concentration of the soil. Recall: pH =-log[H+]
(a) -0.8062 moles per liter
(c) 0.8062 moles per liter
(b) 10-6.4 moles per liter
(d) 106.4 moles per liter](https://media.kunduz.com/media/sug-question/raw/84695740-1658947528.3720496.jpeg?w=256)
Algebra
Sequences & SeriesMultiple Choice: The optimal soil pH for growing corn is approximately 6.4. Find the optimal hydrogen ion concentration of the soil. Recall: pH =-log[H+]
(a) -0.8062 moles per liter
(c) 0.8062 moles per liter
(b) 10-6.4 moles per liter
(d) 106.4 moles per liter

Algebra
Sequences & SeriesJack estimates he will need $25,000 to startup a coffee stand. If he has $10,000 to invest in a savings plan that earns 3% interest compounded monthly, how long will it take to reach the $25,000 he needs to start his business? Setup an equation and solve. Label your answer and round to one decimal place.
Algebra
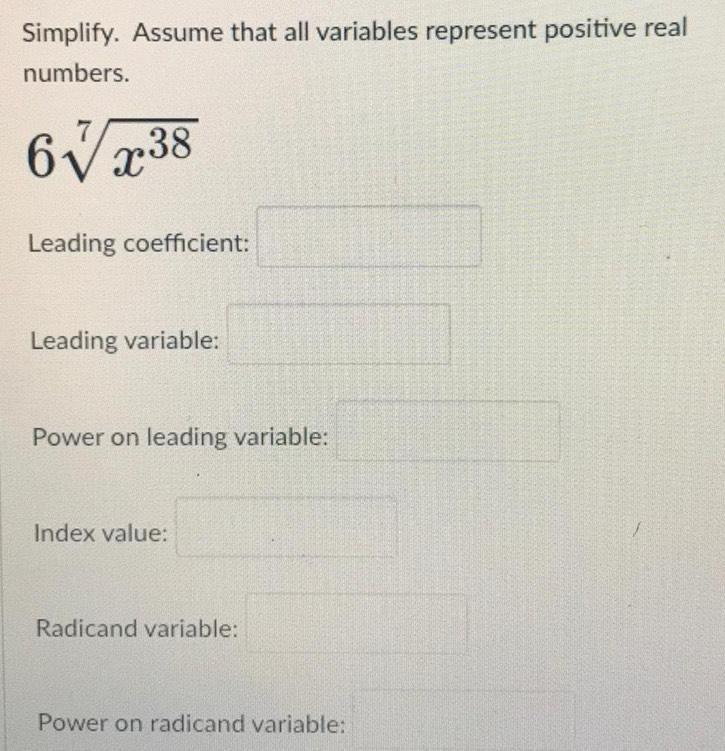
Sequences & SeriesAssume that all variables represent positive real numbers.
6 7 √x38
Leading coefficient:
Leading variable:
Power on leading variable:
Index value:
Radicand variable:
Power on radicand variable:

Algebra
Sequences & SeriesIn ΔFGH, f= 93 inches, h = 89 inches and ∠H=72°. Find all possible values of ∠F, to the nearest degree.

Algebra
Sequences & SeriesCompare and contrast the following in details:
a. An arithmetic sequence and a geometric sequence.
b. A sequence and a series.
c. Discrete data and continuous data.
d. An explicit and a recursive representation.



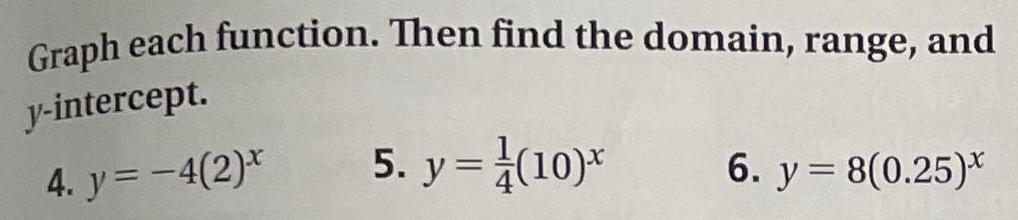
Algebra
Sequences & SeriesGraph each function. Then find the domain, range, and
y-intercept.
4. y = -4(2)x
5. y = 1/4(10) x
6. y = 8(0.25)x
Algebra
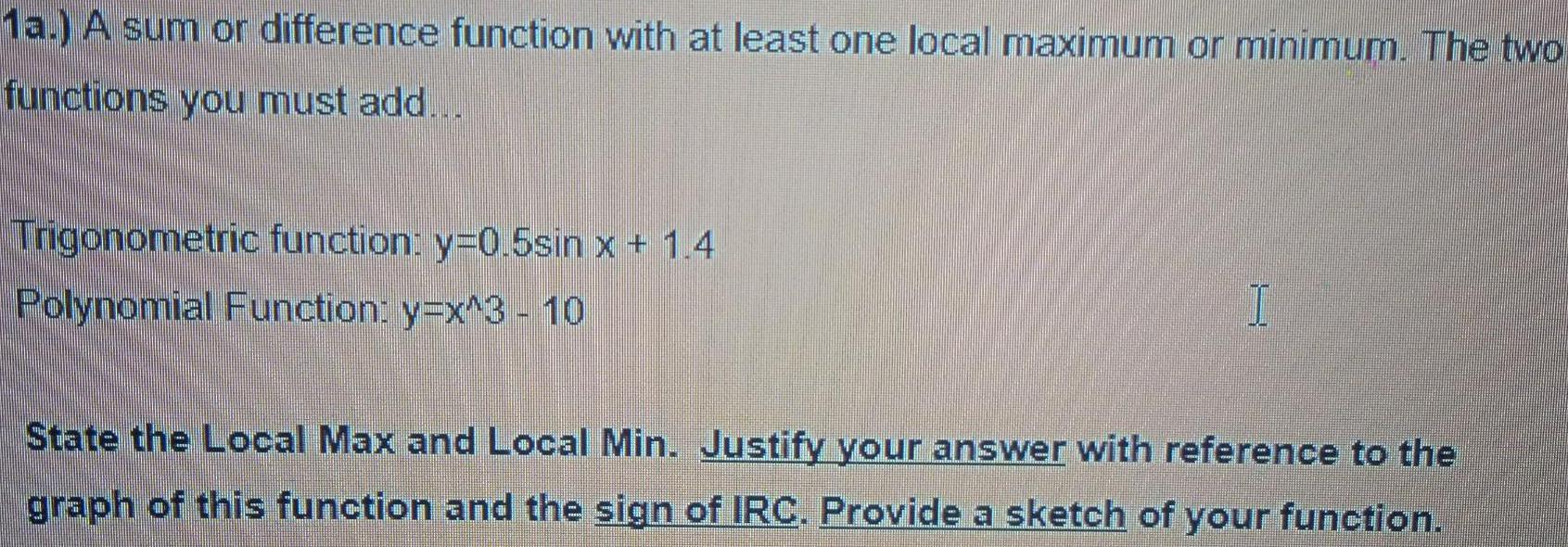
Sequences & SeriesA sum or difference function with at least one local maximum or minimum. The two functions you must add
Trigonometric function: y=0.5sin x + 1.4
Polynomial Function: y=x^3 - 10
State the Local Max and Local Min. Justify your answer with reference to the graph of this function and the sign of IRC. Provide a sketch of your function.

Algebra
Sequences & SeriesEvaluate the following expression, using a calculator if necessary. If the answer does not exist, indicate "DNE."
cos-¹(-0.5)


Algebra
Sequences & SeriesEvaluate the expression, using a calculator if necessary. Round your answer to four decimal places.
sin 102
Algebra
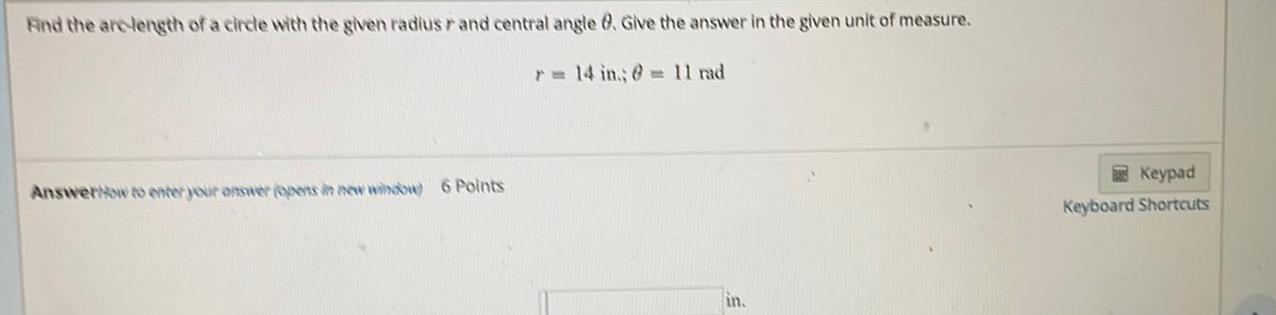
Sequences & SeriesFind the are-length of a circle with the given radius r and central angle θ. Give the answer in the given unit of measure.
r = 14 in; θ = 11 rad

Algebra
Sequences & SeriesGiven cot²θ-3=0, -π≤0≤π
a. Algebraically solve for θ. State the solution(s) as exact radian values.

Algebra
Sequences & SeriesA bicycle when with a radius of 13 inches turns with an angular velocity of 3 radians per second. Find the distance traveled by a point on the bicycle tire in 1 minute.
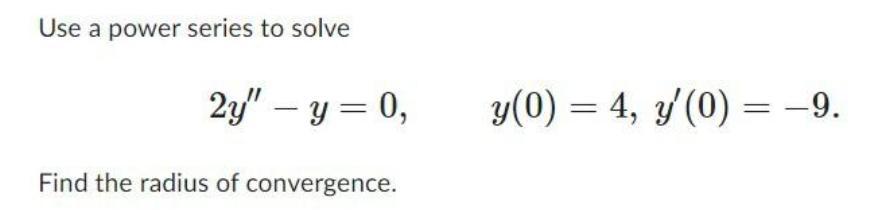
Algebra
Sequences & SeriesUse a power series to solve
2y"-y=0, y(0)=4, y'(0)=-9.
Find the radius of convergence.
Algebra
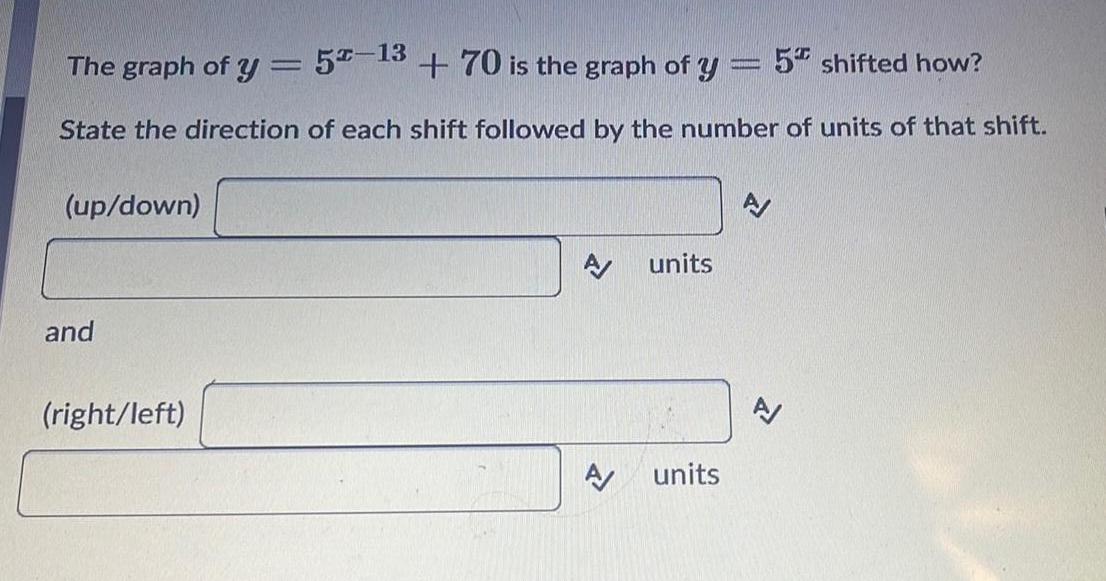
Sequences & SeriesThe graph of y=5^(x-13)+70 is the graph of y=5^x shifted how?
State the direction of each shift followed by the number of units of that shift.
(up/down)___
___ units
and
(right/left)___
___ units

Algebra
Sequences & SeriesThe radioactive isotope Ra-226 has a half-life of about 1600 years. If 10 grams is
present now, how much will be left 500 years from now?
Round to the nearest thousandth of a gram.
a) 8.912 grams
b) 8.052 grams
c) 7.839 grams
d) 7.496 grams



Algebra
Sequences & SeriesConvert the expression from degrees, minutes, seconds (DMS) notation to decimal notation. Round your answer to four decimal places.
67 11 17

Algebra
Sequences & SeriesSolve the equation. Approximate the solution(s) to the nearest hundredth.
e²x-ex- 12 = 0
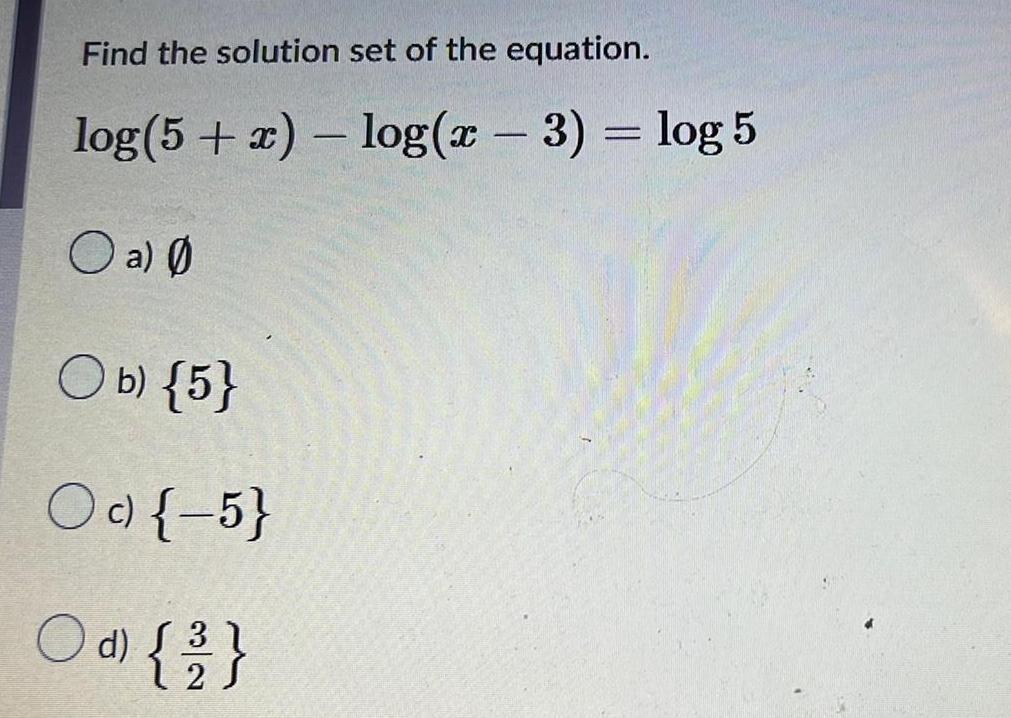
Algebra
Sequences & SeriesFind the solution set of the equation.
log(5+x) -log(x - 3) = log 5
a) Ø
b) {5}
c) {-5}
d) { 1³/12 }

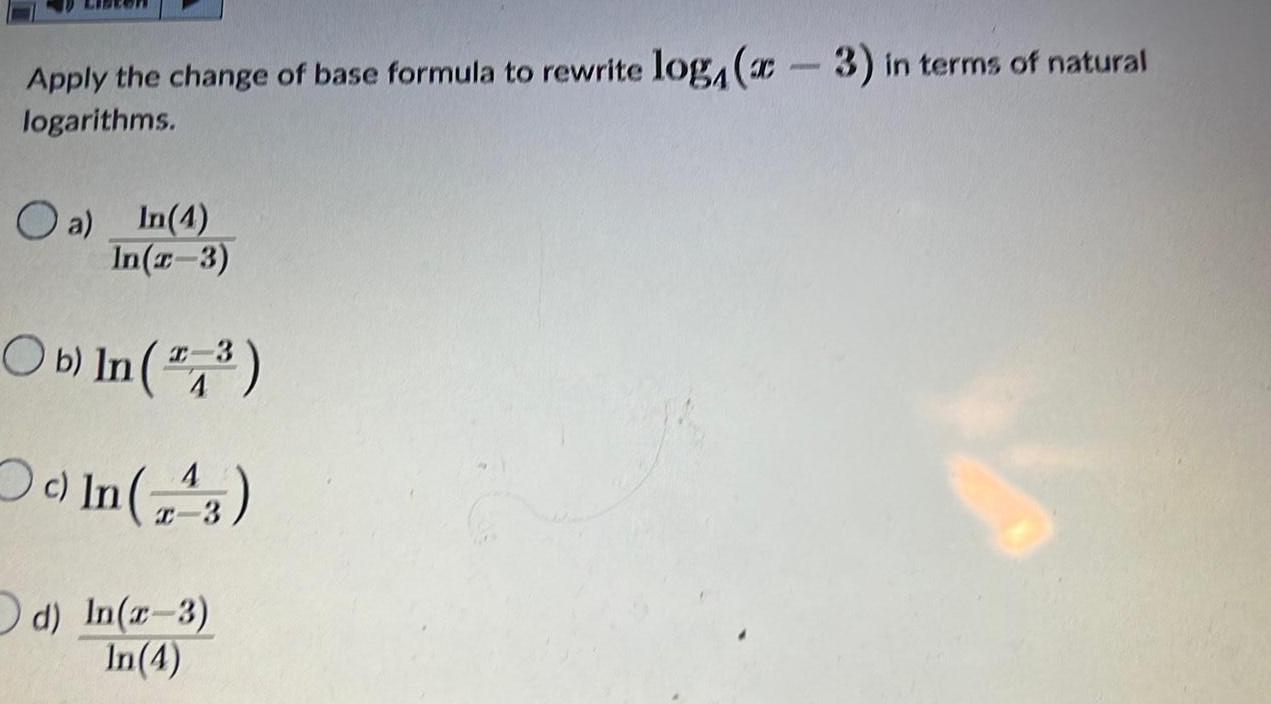
Algebra
Sequences & SeriesApply the change of base formula to rewrite log 4 (x - 3) in terms of natural logarithms.

Algebra
Sequences & SeriesFind r for the infinite geometric sequence. Determine whether the sum will converge.
1, 6, 36, 216, ...

Algebra
Sequences & SeriesFind the sum of the first 8 terms for the following arithmetic sequence.
a4 = 12, a6 = 18

Algebra
Sequences & SeriesFind the arc-length of a circle with the given radius r and central angle θ. Give the answer in the given unit of measure.
r = 52 cm; θ= 12 rad
Algebra
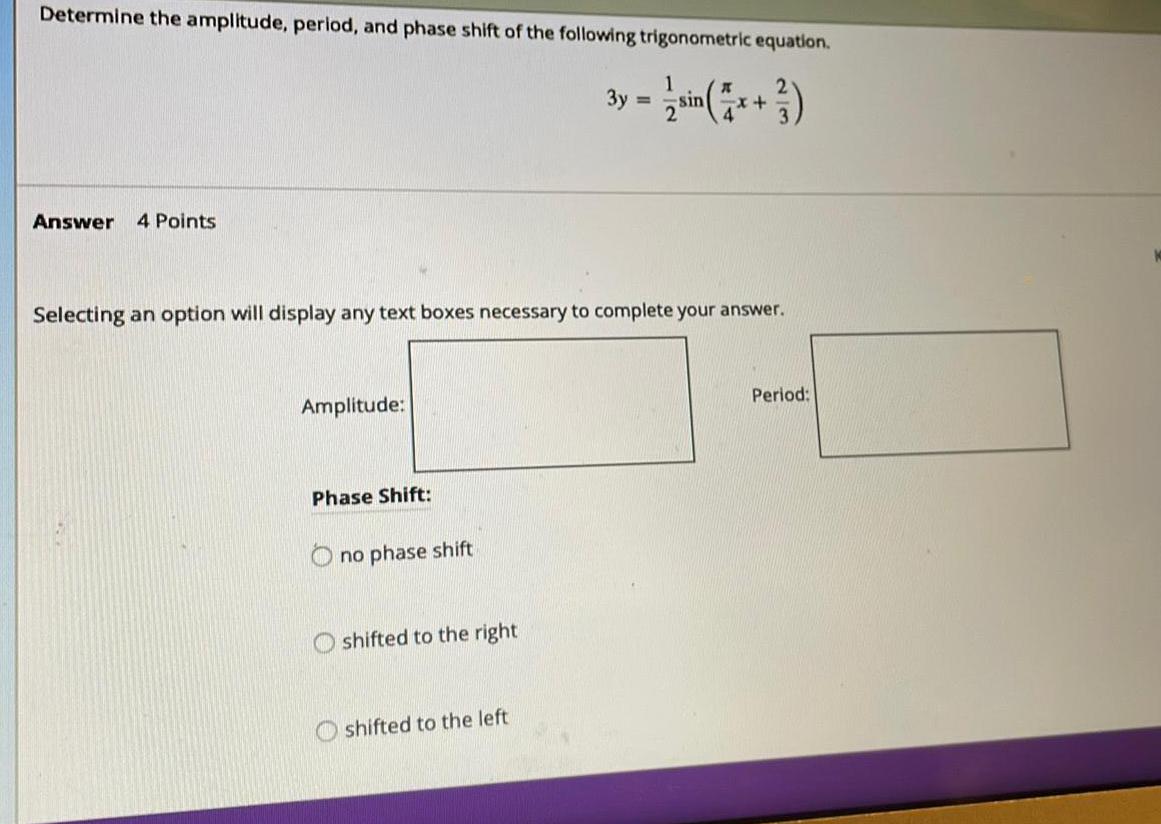
Sequences & SeriesDetermine the amplitude, period, and phase shift of the following trigonometric equation.
3y = 1/2 sin (πx/4+ 2/3)

Algebra
Sequences & SeriesFind the coefficient a of the term in the expansion of the binomial.
Binomial Terms
(x-6y)10 ax8y²
a. 1,619
b. 1,623
c. 1,620
d. 1,621
e. 1,618

Algebra
Sequences & SeriesUse trigonometric identities, algebraic methods, and inverse trigonometric functions, as necessary, to solve the following trigonometric equation on the interval [0, 2π). Round your answer to four decimal places, if necessary. If there is no solution, indicate "No Solution."
2√2cos(x) - 2 = 0
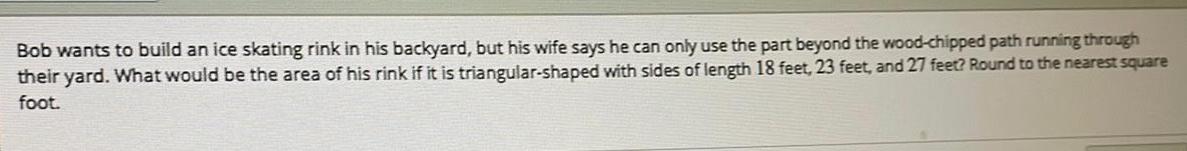
Algebra
Sequences & SeriesBob wants to build an ice skating rink in his backyard, but his wife says he can only use the part beyond the wood-chipped path running through their yard. What would be the area of his rink if it is triangular-shaped with sides of length 18 feet, 23 feet, and 27 feet? Round to the nearest square foot.

Algebra
Sequences & SeriesConsider the following parametric equations:
X = √t-1 and y = t - 4
Determine the domain and range of the equation obtained by eliminating the parameter. Please write your answer in interval notation.

Algebra
Sequences & SeriesA Freshman is planning for a trip to Europe when she graduates in 4 years. She estimates she will need $2000 to pay for the trip. If she has a savings account that earns 7.5% interest compounded monthly, how much does she need to deposit now to pay for her trip? Label your answer and round it to the nearest cent.

Algebra
Sequences & SeriesIf a and n are integers, n > 0, then there exist integers q and r such that a = qn+r, where |r|<n/2.

Algebra
Sequences & SeriesThe weights for 10 adults are 72, 78, 76, 86, 77, 77, 80, 77, 82, 80 kilograms.
Determine the standard deviation.
A. 4.28
B. 3.88
C. 3.78
D. 3.96

Algebra
Sequences & SeriesT-Mobile Park is home to the Seattle Mariners baseball team.
For each game, the amount of money the team brings in as revenue is a function of the number of people in attendance.
If the stadium seats 47929 people and the average ticket price is $30, what are the domain and range of this function?
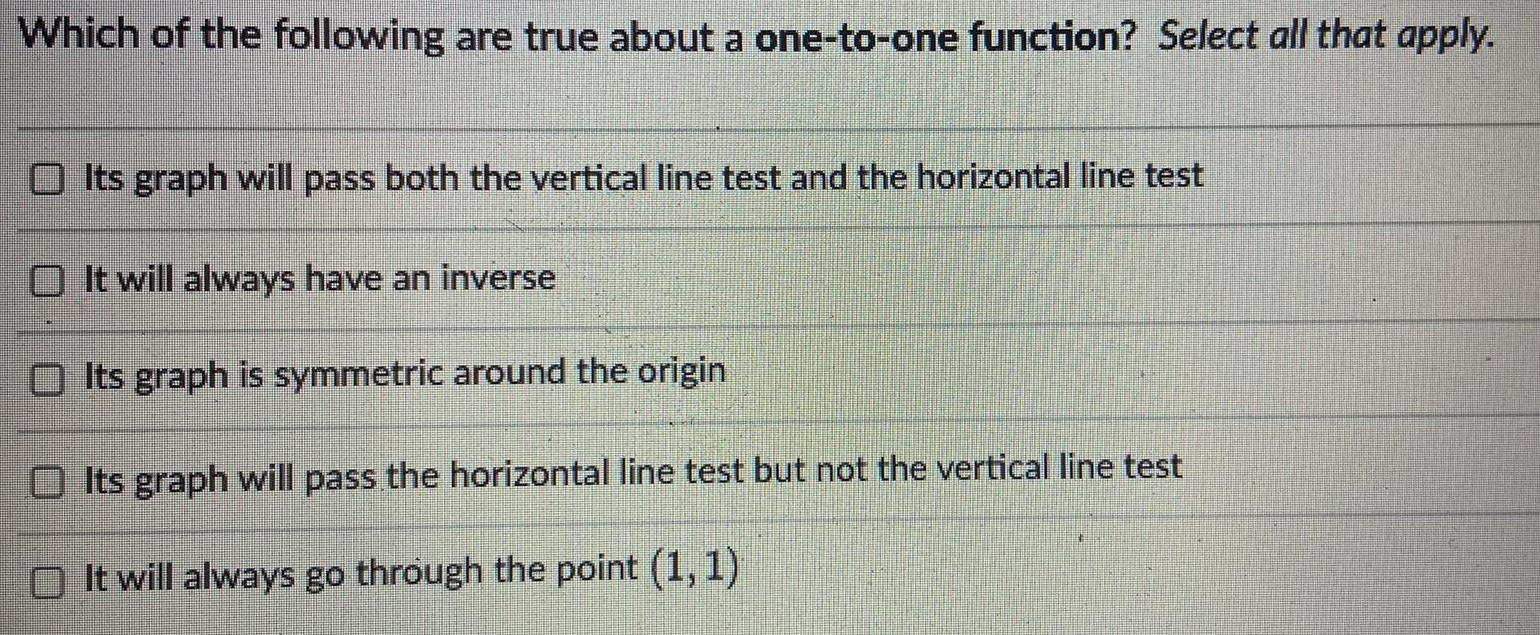
Algebra
Sequences & SeriesWhich of the following are true about a one-to-one function? Select all that apply.
Its graph will pass both the vertical line test and the horizontal line test
It will always have an inverse
Its graph is symmetric around the origin
Its graph will pass the horizontal line test but not the vertical line test
It will always go through the point (1,1)
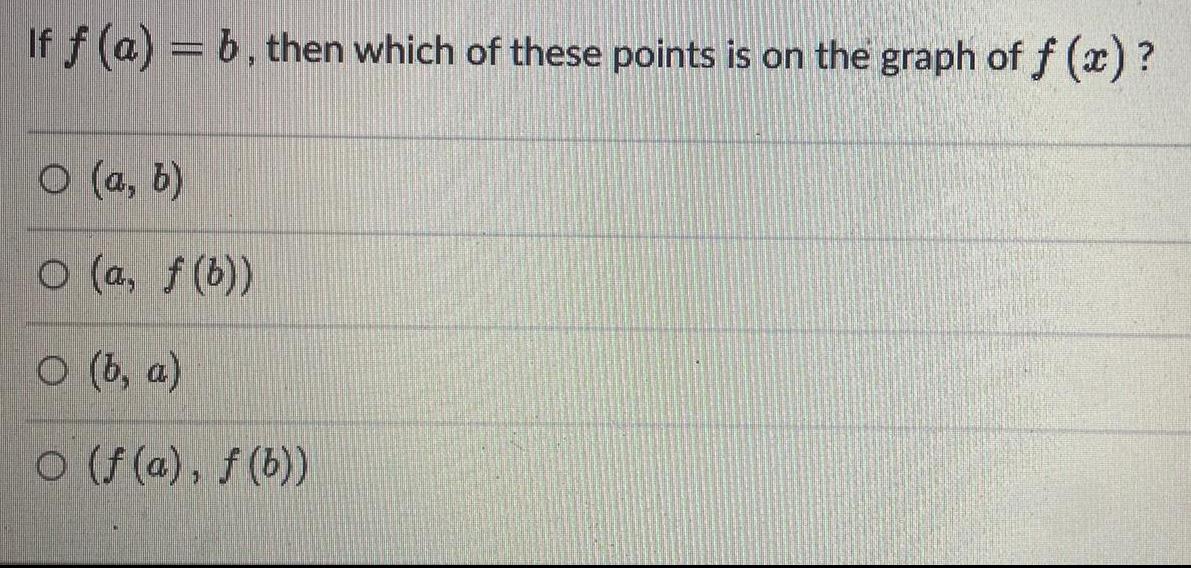
Algebra
Sequences & SeriesIf f (a) = b, then which of these points is on the graph of f(x)?
(a, b)
(a, f(b))
(b, a)
(f (a), f(b))

Algebra
Sequences & SeriesSuppose the function f(t) = 55000e0.061t gives the number of subscribers to a particular YouTube channel t years since 2015.
Which of the following is true?
The number of subscribers decreases as t increases.
The number of subscribers increases as t increases.
The number of subscribers remains constant as t changes.

Algebra
Sequences & SeriesSolve for the remaining angles and side of the triangle described below. Round to the nearest thousandth:
B = 70, c = 6, a = 3

Algebra
Sequences & SeriesWhich of the following situations would be best modeled by an exponential function? Select ALL that apply.
The future value of an investment earning a constant interest rate.
The decay of a radioactive element.
The height of a baseball thrown in the air.
The back and forth motion of a swing.
The area of a circle as the radius increases.
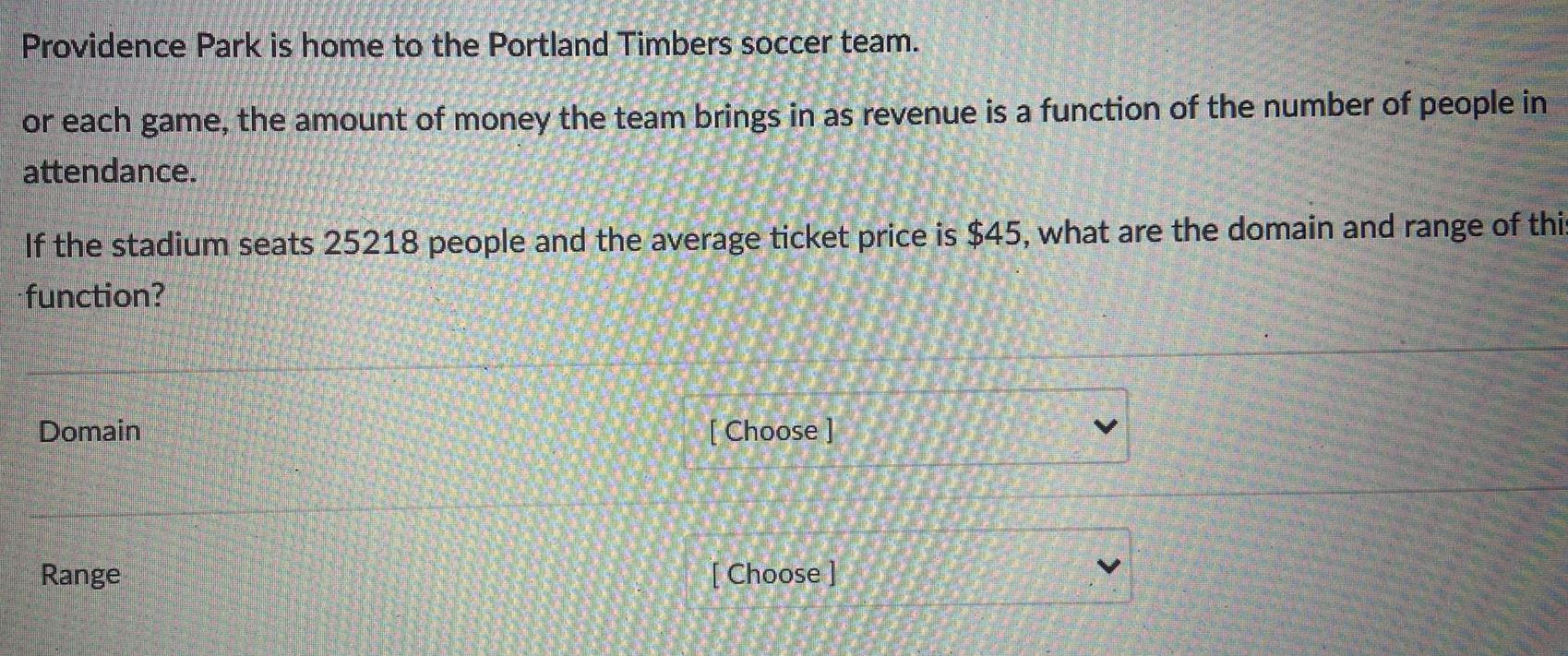
Algebra
Sequences & SeriesProvidence Park is home to the Portland Timbers soccer team. or each game, the amount of money the team brings in as revenue is a function of the number of people in
attendance. If the stadium seats 25218 people and the average ticket price is $45, what are the domain and range of this
function?
Domain
Range

Algebra
Sequences & SeriesIf caffeine leaves the body at a continuous rate of about 16% per hour. How much caffeine is left in the body 8 hours after drinking a cup of coffee containing 100 mg of caffeine?