Application of derivatives Questions and Answers

Calculus
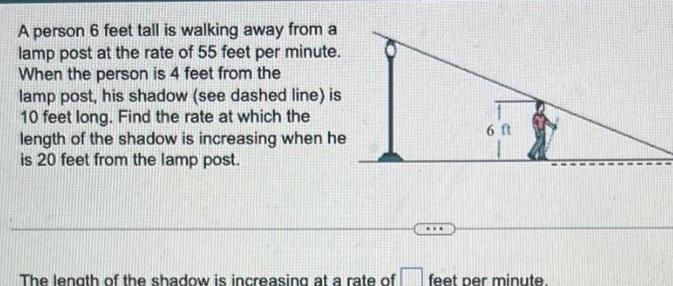
Application of derivativesA person 6 feet tall is walking away from a lamp post at the rate of 55 feet per minute When the person is 4 feet from the lamp post his shadow see dashed line is 10 feet long Find the rate at which the length of the shadow is increasing when he is 20 feet from the lamp post The length of the shadow is increasing at a rate of 6 ft feet per minute
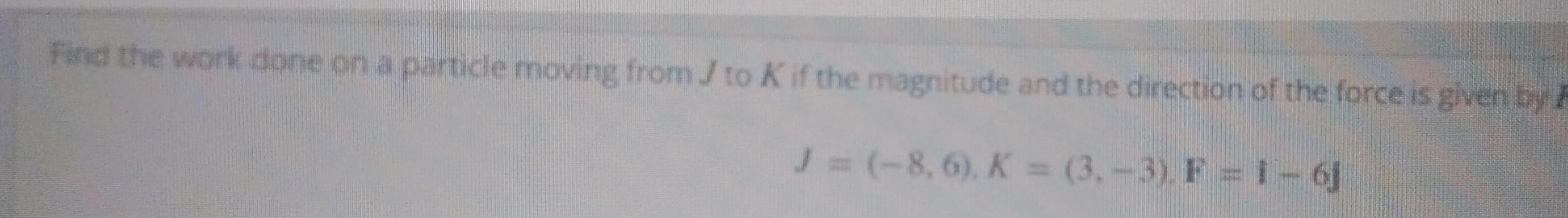
Calculus
Application of derivativesFind the work done on a particle moving from J to K if the magnitude and the direction of the force is given by F J 8 6 K 3 3 F 1 6j

Calculus
Application of derivativesA function f satisfies the following conditions lim f x 6 x limf x 2 x 0 limf x x 6 What can be said about this function O This function has both a horizontal and a vertical asymptote O This function may have a horizontal or vertical asymptote but with the given information we cannot fi O This function has a vertical asymptote but we can t tell whether there is any horizontal asymptote or

Calculus
Application of derivativesYou spin this spinner 3 times Find the probability of these 3 events P red odd 3

Calculus
Application of derivativesFind all points if any of horizontal and vertical tangency to the curve z 5 3 cos 0 y 4 sin 0

Calculus
Application of derivativesA pilot flies in a straight path for 2 h 30 min She then makes a course correction heading 7 to the right of her original course and flies 3 h in the new direction If she maintains a constant speed of 695 mi h how far is she from her starting position Please round the answer to the nearest integer

Calculus
Application of derivativesA ball of radius 10 with a cylindrical hole of radius 9 drilled through its center has volume volume

Calculus
Application of derivativess the eighth term of the recursively defined sequence 3 and an 3 1 3 1 8 for n22 hth term is

Calculus
Application of derivativesFind the 4th term in the sequence defined recursively by 9 3 an 2a 1 5 Select one O a 4 O b 11 c 7 O d 1

Calculus
Application of derivatives9 Find the area between the curves y sketch of the curves and shade the area to be found sin z y cos over the interval 7 7 Include a detailed

Calculus
Application of derivativesThe boundaries of the shaded region are the y axis the line y 1 and the curve y Find the area of this region by writing a as a function of y and integrating with respect to y YA 1 y 1 y 4 x
Calculus
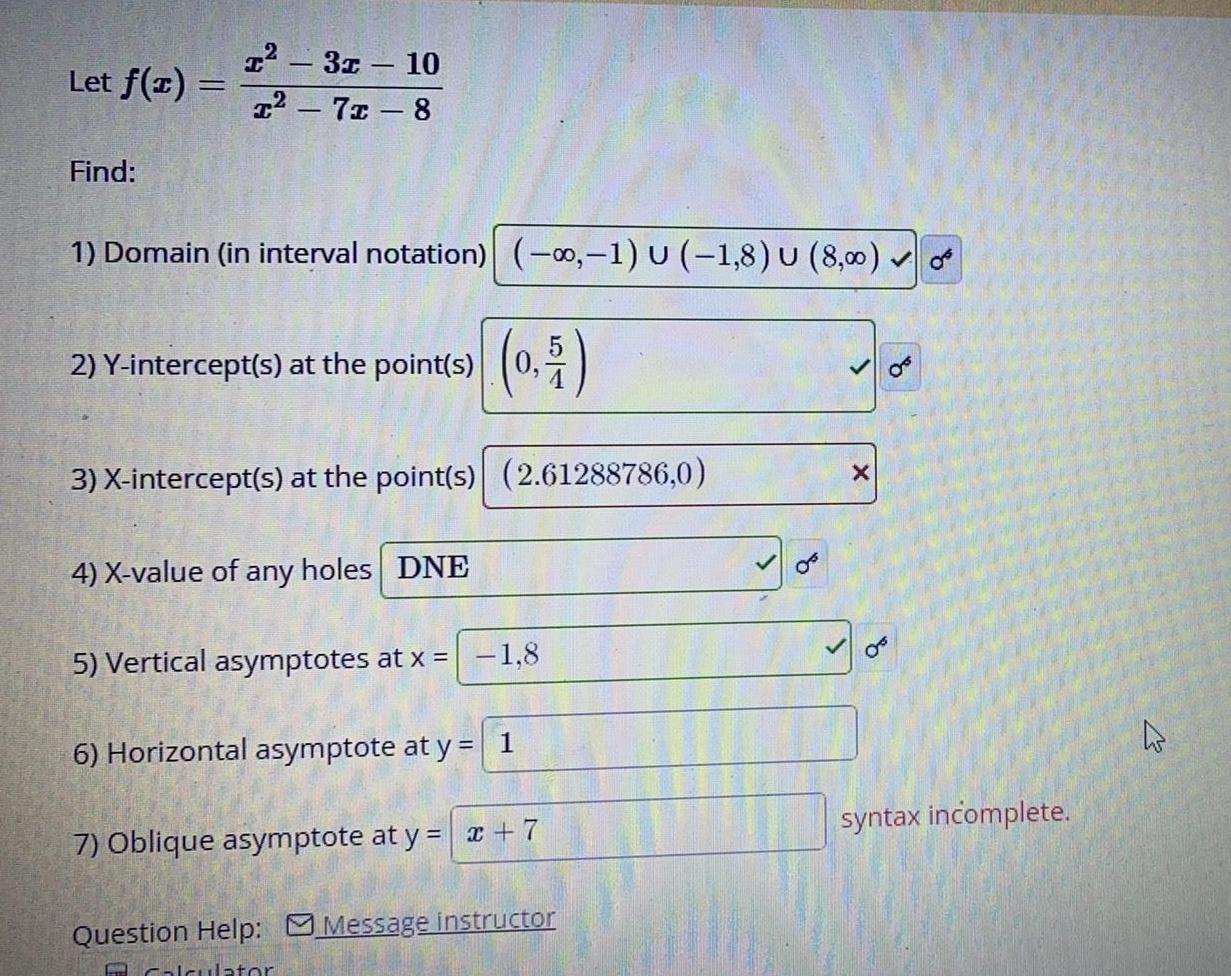
Application of derivativesLet f x Find T 31 10 I 71 8 1 Domain in interval notation 1 U 1 8 U 8 00 2 Y intercept s at the point s 0 5 3 X intercept s at the point s 2 61288786 0 4 X value of any holes DNE 5 Vertical asymptotes at x 1 8 6 Horizontal asymptote at y 1 7 Oblique asymptote at y x 7 Question Help Message instructor E Calculator OF X q OF syntax incomplete 4
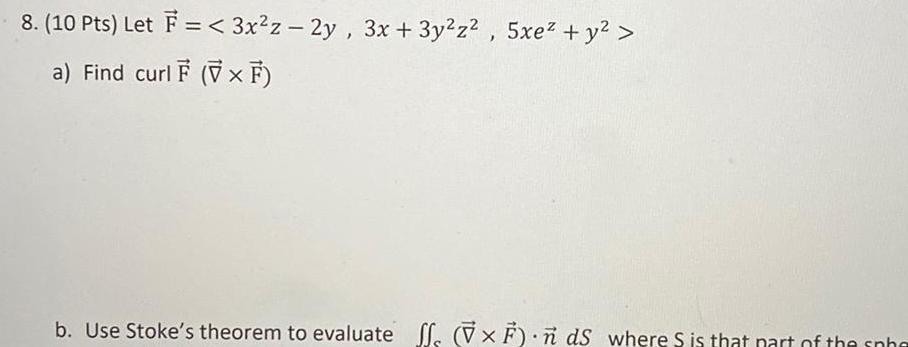
Calculus
Application of derivatives8 10 Pts Let F 3x z 2y 3x 3y z 5xe y a Find curl F VxF b Use Stoke s theorem to evaluate f xF ds where S is that part of the sphe
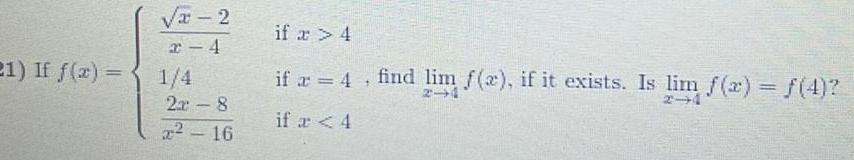
Calculus
Application of derivativesx 2 2 4 21 If f x 1 4 22 8 22 16 if a 4 if x 4 find lim f x if it exists Is lim f x 4 2 4 if a 4

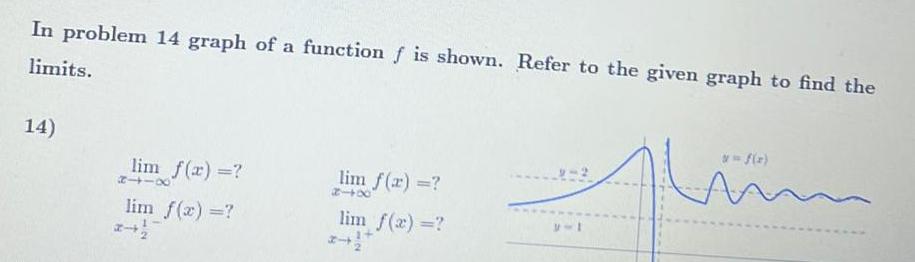
Calculus
Application of derivativesIn problem 14 graph of a function f is shown Refer to the given graph to find the limits 14 lim f x 2118 lim f x lim f x lim f x f m


Calculus
Application of derivativesThe velocity of a car is f t 8t meters per second Use a graph of f t to find the exact distance travelled by the car in meters from t 0 to t 8 seconds Distance

Calculus
Application of derivativesWhat relevant conclusion can be drawn from the following promesise If I play hockey then I am sore the next day I use the whirlpool if I am sore I did not use the whirlpoo OI played hockey O I m sore OI use the whirlpool OI didn t play hockey
Calculus
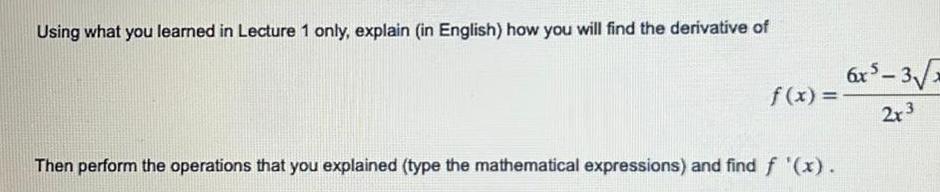
Application of derivativesUsing what you learned in Lecture 1 only explain in English how you will find the derivative of f x Then perform the operations that you explained type the mathematical expressions and find f x 6x5 3 2x 3

Calculus
Application of derivativesA boat is traveling at 34 knots in a direction of 68 It encounters wind moving at 11 knots in a direction of 15 What is the true velocity and direction of the boat Round to the nearest whole number O 23 knots 34 O 41 knots 55 41 knots 34
Calculus
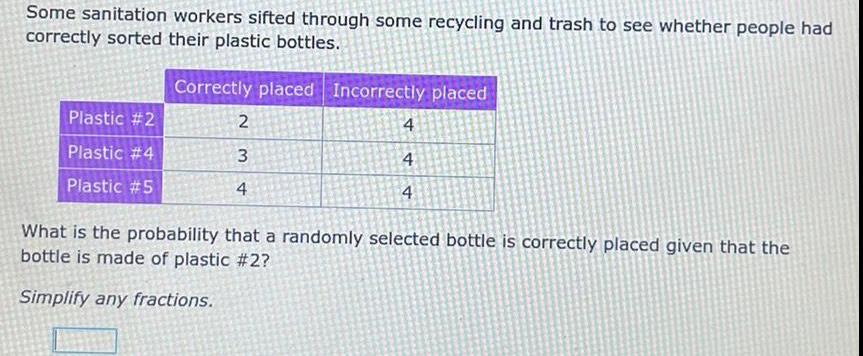
Application of derivativesSome sanitation workers sifted through some recycling and trash to see whether people had correctly sorted their plastic bottles Plastic 2 Plastic 4 Plastic 5 Correctly placed Incorrectly placed 2 3 4 4 4 4 What is the probability that a randomly selected bottle is correctly placed given that the bottle is made of plastic 2 Simplify any fractions

Calculus
Application of derivativesSolve y 4y 5y 4xe sin x 7cos x by the method of undetermined coefficients

Calculus
Application of derivativesYou create a container by rotating the curve y 5 ln x 2 for y 0 to y 3 around the y axis You then fill the container to a height of 1 meters with a liquid that has density 900 kg m The work required to pump the liquid out of this container over its top edge is Joules Recall that acceleration due to gravity is 9 81 m sc0 1
Calculus
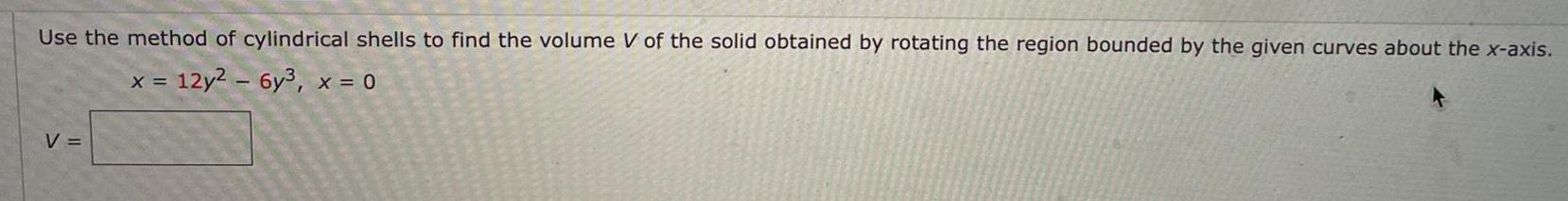
Application of derivativesUse the method of cylindrical shells to find the volume V of the solid obtained by rotating the region bounded by the given curves about the x axis X 12y 6y x 0 V

Calculus
Application of derivativesGiven the graph of function f below which is greater N 3 off ar da is greater of f x de is greater Othey are the same d Explain in detail why you chose this answer S 2 dx or 2 dz f x dx
Calculus
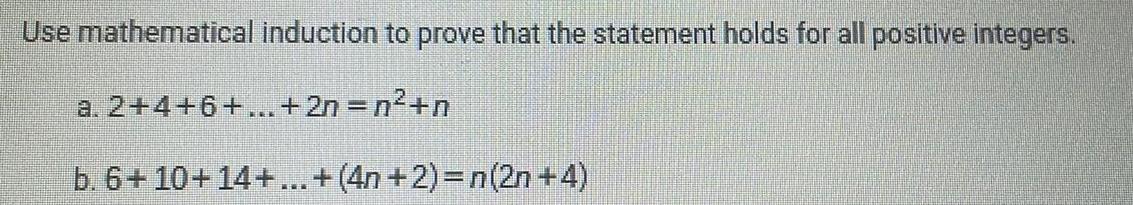
Application of derivativesUse mathematical induction to prove that the statement holds for all positive integers a 2 4 6 2n n n b 6 10 14 4n 2 n 2n 4

Calculus
Application of derivativesSavings Account The table below shows the December balance in a simple interest savings account each year from 1996 to 2000 Year 1996 1997 1998 2000 1999 Balance 18 000 20 016 22 032 24 048 26 064 a The balances form an arithmetic sequence What is d b Write a formula for the balance in the account years after December 1996 e Find the sum of the December balances from 1996 to 2006 inclusive


Calculus
Application of derivativesUse the method of cylindrical y 4 x y 0 shells to find the volume V generated by rotating the region bounded by the given curves about the specified axis x 1 about x 3

Calculus
Application of derivativesP QUESTION 2 Explain why the graph shown is not the graph of y 6 x 3 7
Calculus
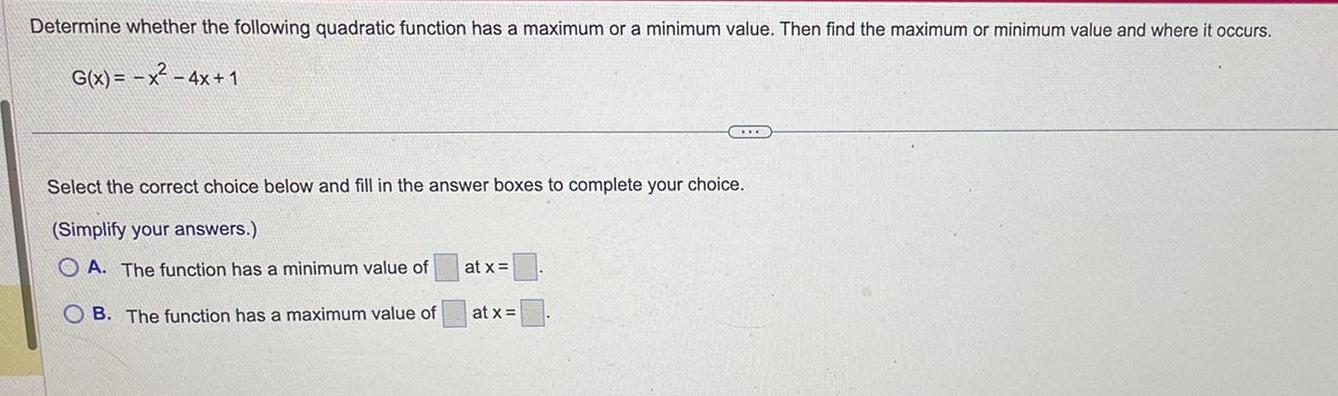
Application of derivativesDetermine whether the following quadratic function has a maximum or a minimum value Then find the maximum or minimum value and where it occurs G x x 4x 1 Select the correct choice below and fill in the answer boxes to complete your choice Simplify your answers OA The function has a minimum value of OB The function has a maximum value of at x CELLO at x
Calculus
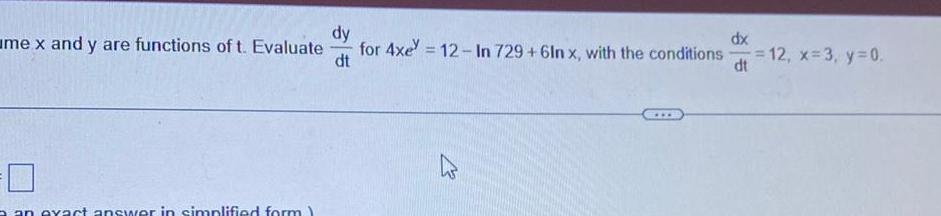
Application of derivativesme x and y are functions of t Evaluate an exact answer in simplified form dy dt dx for 4xe 12 In 729 6In x with the conditions 12 x 3 y 0 dt

Calculus
Application of derivatives5 4 3 b 16 1 C 5 d 4 XX 2 4 The plot above is created with the parametric equations x t a cos bt ly t c sin dt To acheive this graph a 6

Calculus
Application of derivativesUse the given information Focus parabola 83 7 Ox 2 y 7 8 Ox 3 y 9 2 Ox 2 y 5 3 Ox 3 v 5 1 Directrix X 65 8 to write the equation of the

Calculus
Application of derivativesUse the given information Center 0 8 Radius 5 to write the equation of the circle O x y 8 2 5 O x y 8 2 25 O x y 8 25 O x 8 y 25
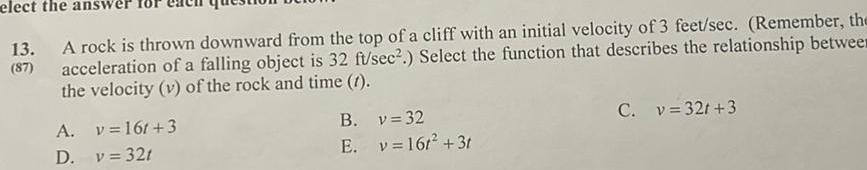
Calculus
Application of derivativeselect the an 13 A rock is thrown downward from the top of a cliff with an initial velocity of 3 feet sec Remember the acceleration of a falling object is 32 ft sec Select the function that describes the relationship betwee the velocity v of the rock and time 1 A D v 16t 3 v 321 B E v 32 v 16t 3t C v 32r 3

Calculus
Application of derivativesA tennis ball is thrown downward with an initial velocity of 10 feet sec from the top of a cliff Remember the acceleration of a falling object is 32 ft sec Select the function that describes the relationship betweer the distance the ball travels d and time 1 A d 161 10r D d 32 B E d 161 10 d 16t C d 321 10

Calculus
Application of derivatives11 Given g x 2x 96x and g x 6x 96 select the local maximum and minimum of g x 86 A Local Max 4 0 Local Min 4 0 C Local Max 4 256 Local Min 0 0 E Local Max 0 0 Local Min 4 256 B Local Max 4 256 Local Min 4 256 D Local Max 4 236 Local Min 4 236
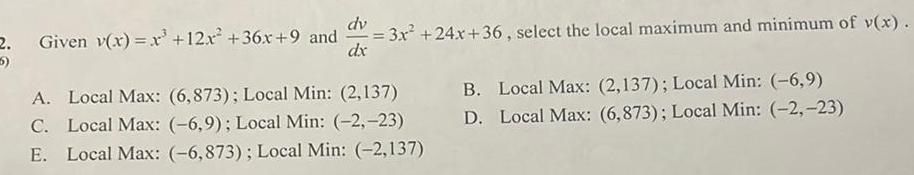
Calculus
Application of derivativesdv 2 Given v x x 12x 36x 9 and d 3x 24x 36 select the local maximum and minimum of v x dx A Local Max 6 873 Local Min 2 137 C Local Max 6 9 Local Min 2 23 E Local Max 6 873 Local Min 2 137 B Local Max 2 137 Local Min 6 9 D Local Max 6 873 Local Min 2 23
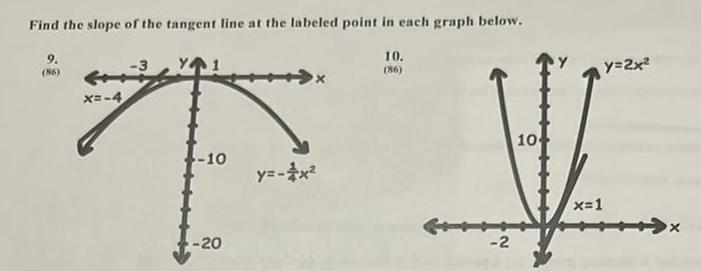
Calculus
Application of derivativesFind the slope of the tangent line at the labeled point in each graph below 9 86 x 4 10 20 y x 10 86 2 10 y 2x x 1
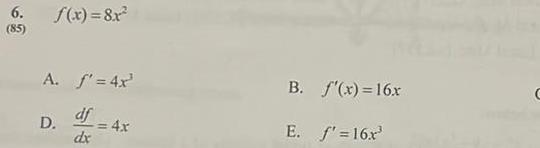
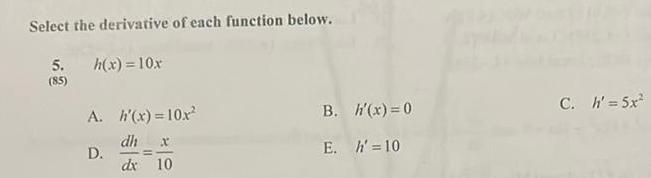
Calculus
Application of derivativesSelect the derivative of each function below 5 85 h x 10x A h x 10x dh x D dx 10 B E h x 0 h 10 C h 5x

Calculus
Application of derivativesAt a restaurant the density function for the time a customer has to wait before being seated is given by f t 2e if t 0 if t 0 Find the probability that a customer will have to wait at least 5 minutes for a table

Calculus
Application of derivativesJump to Problem 1 2 3 4 5 6 7 8 Problem 1 1 point Find the value of k so that the function is a density function Give your answer as a decimal rounded to 6 decimal places k f x kx if 0 x 10 otherwise
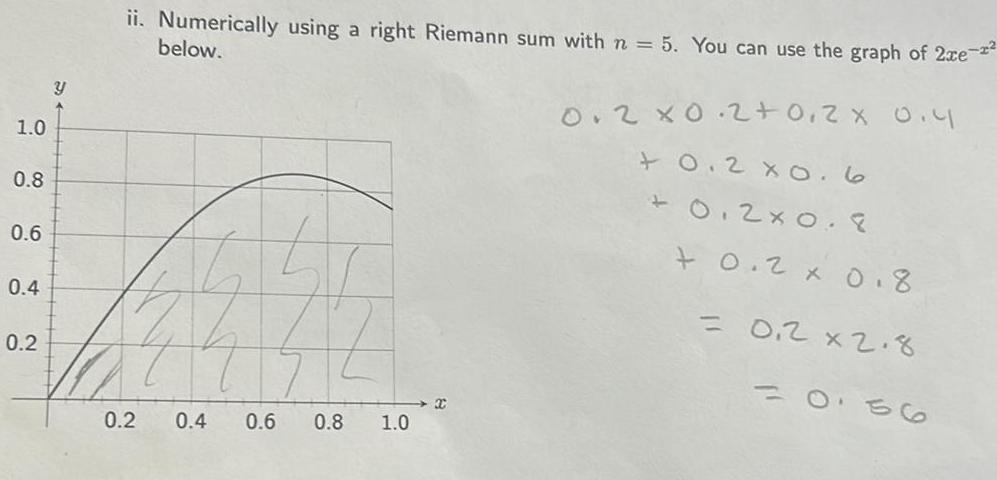
Calculus
Application of derivatives1 0 0 8 0 6 0 4 0 2 Y ii Numerically using a right Riemann sum with n 5 You can use the graph of 2xe below 0 2 0 4 0 6 0 8 1 0 x 0 2X0 2 0 2x 0 4 0 2 x 0 6 0 2x0 8 0 2 x 0 8 0 2 x 2 8 0 56

Calculus
Application of derivativesthe area of the region that is trapped between the curves y 4x and y x HINT Draw a picture