Application of derivatives Questions and Answers

Calculus
Application of derivativesA model used for the yield Y of an agricultural crop as a function of the nitrogen level W in the soil (measured in appropriate units) is
Y=KN /25 + N²
where k is a positive constant. What nitrogen level gives the best yield?

Calculus
Application of derivativesDivide.
(-10x³+7x-5)÷(2x²-3)
Write your answer in the following form: Quotient +

Calculus
Application of derivatives(-7x4+5x³+20x²+10)÷(-x²+x+3)
Write your answer in the following form: Quotient +
-7x4+5x³+20x² +10
-x²+x+3
=
+
2
-x + x + 3
Remainder
2
-x+x+3
X
S
?

Calculus
Application of derivativesDetermine if the following function is concave up or concave down in the first quadrant.
y=5x^2/3
Is the function y=5x^2/3 concave up or concave down in the first quadrant?
Concave up
Concave down

Calculus
Application of derivativesDetermine whether the function y = -2x³ is increasing or decreasing for the following conditions.
(a) x < 0
(b) x > 0
(a) Is the function increasing or decreasing for x < 0?
decreasing
increasing

Calculus
Application of derivativesThe annual total revenue for a product is given by R(x) = 30,000x – 5x² dollars, where x is the number of units sold. To maximize revenue, how many units must be sold? What is the maximum possible annual revenue?
To maximize revenue,_________units must be sold.
(Simplify your answer.
![Let h(x) = -9x - 13 - 6x²- x³
Determine the absolute extrema of h on [-4, 0]. If multiple such values exist, enter the solutions using
a comma-separated list.
The absolute minimum of h is_______and it occurs at x =_____
The absolute maximum of h is_______and it occurs at x =_____](https://media.kunduz.com/media/sug-question/raw/84226586-1657014750.066876.jpeg?w=256)
Calculus
Application of derivativesLet h(x) = -9x - 13 - 6x²- x³
Determine the absolute extrema of h on [-4, 0]. If multiple such values exist, enter the solutions using
a comma-separated list.
The absolute minimum of h is_______and it occurs at x =_____
The absolute maximum of h is_______and it occurs at x =_____
![Consider h(v) = 7v log5( – 6v) on [ – 125/6 ,-1/6]
Determine the interval over which h is continuous and the interval over which h is differentiable.
h is continuous on _______
h is differentiable on _______
-
Use the above information to determine if the Mean Value Theorem may be applied to h over
[ – 125/6 ,-1/6]](https://media.kunduz.com/media/sug-question/raw/84230069-1657014458.3058245.jpeg?w=256)
Calculus
Application of derivativesConsider h(v) = 7v log5( – 6v) on [ – 125/6 ,-1/6]
Determine the interval over which h is continuous and the interval over which h is differentiable.
h is continuous on _______
h is differentiable on _______
-
Use the above information to determine if the Mean Value Theorem may be applied to h over
[ – 125/6 ,-1/6]

Calculus
Application of derivativesFind the points on the curve y = x³ + 3x^2 - 9x + 8 where the tangent is horizontal.
smaller x-value (x, y) =
larger x-value (x, y) =
![4 ln(x + 6)
x + 6
Let f(x)
Determine the absolute extrema of f on [-5, -1]. If multiple such values exist, enter the solutions using
a comma-separated list.
The absolute minimum of fis
+2
The absolute maximum of f is
and it occurs at x =
and it occurs at x =](https://media.kunduz.com/media/sug-question/raw/84224818-1656960671.4069893.jpeg?w=256)
Calculus
Application of derivatives4 ln(x + 6)
x + 6
Let f(x)
Determine the absolute extrema of f on [-5, -1]. If multiple such values exist, enter the solutions using
a comma-separated list.
The absolute minimum of fis
+2
The absolute maximum of f is
and it occurs at x =
and it occurs at x =
![Let h(x) - 52 +9
Determine the absolute extrema of h on [-3, 3].](https://media.kunduz.com/media/sug-question/raw/84224788-1656960557.125407.jpeg?w=256)
![Let g(x) = 17 + 24x + x³ + 9x²
Determine the absolute extrema of g on [-5, -1]. If multiple such values exist, enter the solutions using
a comma-separated list.](https://media.kunduz.com/media/sug-question/raw/84224801-1656960534.6443048.jpeg?w=256)
Calculus
Application of derivativesLet g(x) = 17 + 24x + x³ + 9x²
Determine the absolute extrema of g on [-5, -1]. If multiple such values exist, enter the solutions using
a comma-separated list.
![Let h(x) = x² + 21 + 10x
Determine the absolute extrema of h on [- 7,2].](https://media.kunduz.com/media/sug-question/raw/84224797-1656960514.576982.jpeg?w=256)
Calculus
Application of derivativesLet h(x) = x² + 21 + 10x
Determine the absolute extrema of h on [- 7,2].

Calculus
Application of derivativesFor the polynomial below, -3 is a zero.
g(x)=x²³ - 2x² - 9x + 18
Express g (x) as a product of linear factors.

Calculus
Application of derivatives7. (Factoring) Factor the difference of squares.
a) x² - 121
b) 9m² - 4n²

Calculus
Application of derivatives(1 point)
3sin(x)tan(x)+3¯¯√sin(x)=0
Find all angles in radians that satisfy the
equation. For each solution enter first the angle
solution in [0,π) оr [0,2π) (depending on the
trigonometric function) then the period. When 2
or more solutions are available enter them in
increasing order of the angles. (e.g. x=π/2+2kt
or x=3π/2+kπ etc.)
Note: You are not allowed to use decimals in
your answer. Use pi for π.

Calculus
Application of derivativesFor the polynomial below, 3 is a zero.
g(x)=x²- 4x² + x + 6
Express g (x) as a product of linear factors.

Calculus
Application of derivativesFor the polynomial below, -3 is a zero.
f(x)=x³ - 3x²
Express f(x) as a product of linear factors.

Calculus
Application of derivativesA spherical balloon is inflated at the rate of 67 cm³/sec. At what rate is the
radius increasing when r = 4 cm?

Calculus
Application of derivativesSand falls from an overhead bin and accumulates in a conical pile with a radius
that is always two times its height. Suppose the height of the pile increases at a
rate of 3 cm/s when the pile is 17 cm high. At what rate is the sand leaving the bin
at that instant?
![Find the extrema of y = x³-6x² +9x+2 on [0,2]. (Notice this is the same equation as #4a.)
Label max/min.](https://media.kunduz.com/media/sug-question/raw/84213002-1656959325.7371533.jpeg?w=256)
Calculus
Application of derivativesFind the extrema of y = x³-6x² +9x+2 on [0,2]. (Notice this is the same equation as #4a.)
Label max/min.

Calculus
Application of derivativesFind the unit tangent vector T(t) at the point with the given value of the parameter t.
r(t) =(t²- 3t, 1 + 4t,1/3 t^3+1/2 t^2) ,t = 3
T(3)=

Calculus
Application of derivativesFind the profit function if cost and revenue are given by C(x) = 178 +4.9x and R(x) = 7x -0.05x².
The profit function is P(x) =

Calculus
Application of derivativesFind an equation for the surface consisting of all points that are equidistant from the point (-3, 0, 0) and the plane x = 3.
Identify the surface.
O parabolic cylinder
O hyperbolic paraboloid
O hyperboloid of one sheet
O circular paraboloid
O hyperboloid of two sheets
O ellipsoid
O elliptic cylinder
O cone

Calculus
Application of derivativesFind all local extremes of the function f(x, y) = (x² + y²) e^x²-y²


Calculus
Application of derivativesThe total cost (in dollars) of producing x food processors is C(x) = 1900 + 30x -0.1x².
(A) Find the exact cost of producing the 91st food processor.
(B) Use the marginal cost to approximate the cost of producing the 91st food processor.
(A) The exact cost of producing the 91st food processor is $_______
(B) Using the marginal cost, the approximate cost of producing the 91st food processor is $______
*
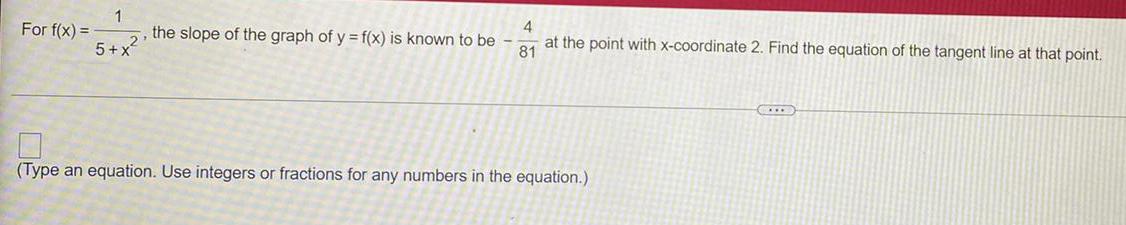
Calculus
Application of derivativesFor f(x)=1/5+x²4the slope of the graph of y = f(x) is known to be -4/81 at the point with x-coordinate 2. Find the equation of the tangent line at that point.
_____(Type an equation. Use integers or fractions for any numbers in the equation.).