Application of derivatives Questions and Answers

Calculus
Application of derivativesThere is given the autonomous system of order 2 a Write the sufficient conditions of the existence and uniqueness of the maximal solution b Write all domains in which the conditions are satisfied c Write all equilibrium points of the given system x xlny j yln x x x 1 4 y y x 4

Calculus
Application of derivativesConsider the function f x y 4x x 14y y Find and classify all critical points of the function If there are more blanks than critical points leave the remaining entries blank fx fy fxx fyy There are several critical points to be listed List them lexicograhically that is in ascending order by x coordinates and for equal x coordinates in ascending order by y coordinates e g 1 1 2 1 2 3 is a correct order The critical point with the smallest x coordinate is Classification minimum local maximum saddle point cannot be determined The critical point with the next smallest x coordinate is Classification minimum local maximum saddle point cannot be determined The critical point with the next smallest x coordinate is Classification minimum local maximum saddle point cannot be determined The critical point with the next smallest x coordinate is Classification minimum local maximum saddle point cannot be determined The critical point with the next smallest x coordinate is Classification minimum local maximum saddle point cannot be determined local local local local local

Calculus
Application of derivativesFind all solutions to 2 sin 0 2 on the interval 0 0 2 0 Give your answers as exact values as a list separated by commas

Calculus
Application of derivativesGiven that the graph of f x passes through the point 4 8 and that the slope of its tangent line at x f x is 4x 3 what is f 2
Calculus
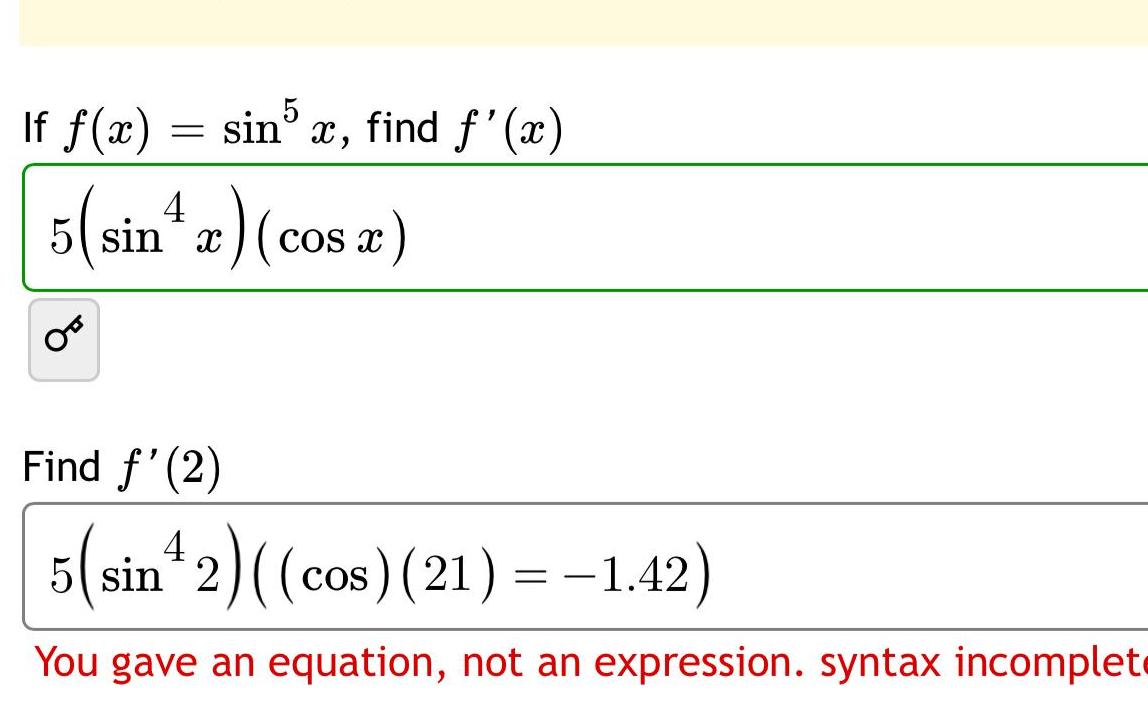
Application of derivativesIf f x sin5 x find f x 5 sin x cos x OB Find f 2 5 sin 2 cos 21 1 42 You gave an equation not an expression syntax incomplet

Calculus
Application of derivativesFind all local extreme values of 2 f x 3 x 3x 8x 70 The FIRST local extreme value moving from left to right on the graph is a Select an answer at The SECOND extreme value moving from left to right on the graph is a Select an answer at
Calculus
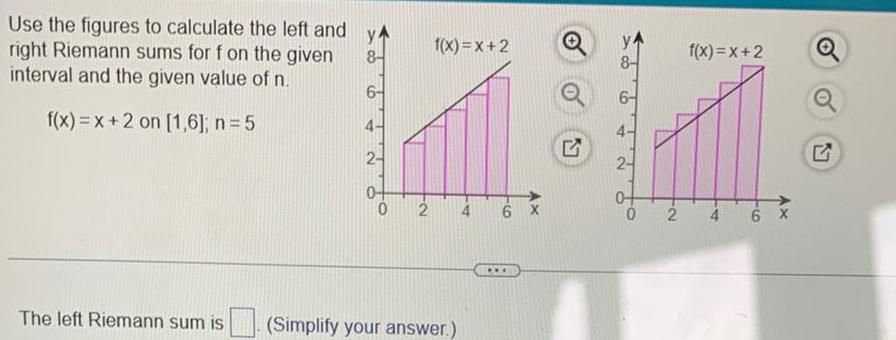
Application of derivativesUse the figures to calculate the left and right Riemann sums for f on the given interval and the given value of n f x x 2 on 1 6 n 5 The left Riemann sum is 8 0 2 f x x 2 Q Simplify your answer 4 6 X f x x 2 2 4 6 X
Calculus
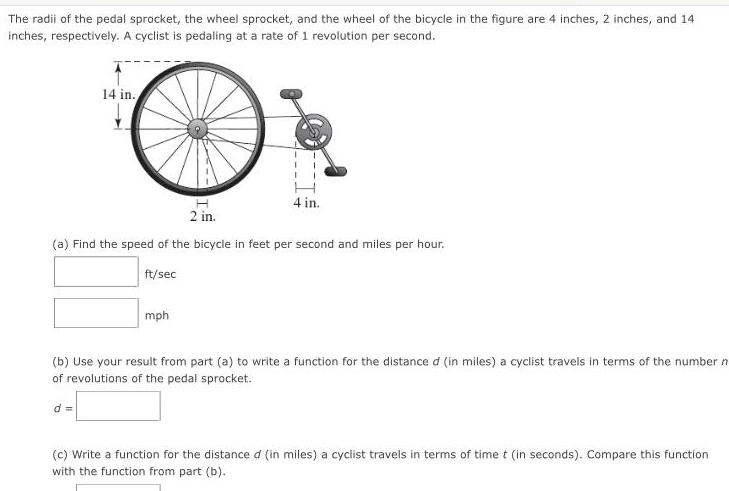
Application of derivativesThe radii of the pedal sprocket the wheel sprocket and the wheel of the bicycle in the figure are 4 inches 2 inches and 14 inches respectively A cyclist is pedaling at a rate of 1 revolution per second 14 in 4 in 2 in a Find the speed of the bicycle in feet per second and miles per hour ft sec mph b Use your result from part a to write a function for the distance d in miles a cyclist travels in terms of the number n of revolutions of the pedal sprocket d c Write a function for the distance d in miles a cyclist travels in terms of time t in seconds Compare this function with the function from part b
Calculus
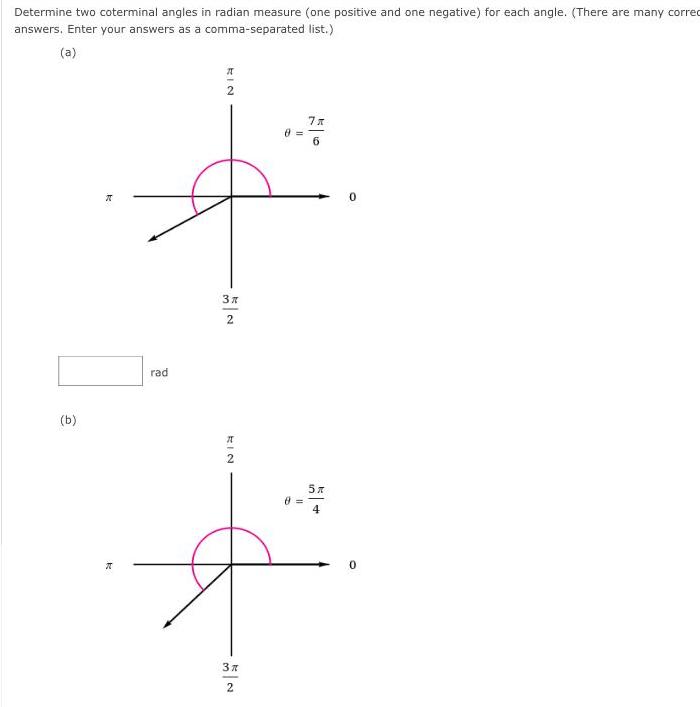
Application of derivativesDetermine two coterminal angles in radian measure one positive and one negative for each angle There are many corred answers Enter your answers as a comma separated list a b N k rad KIN 2 3 2 712 3x 2 8 7x 6 5x

Calculus
Application of derivatives10 Find the directional derivative of f x y z xy z at the point 2 1 1 3 in the direction of 23 k BO

Calculus
Application of derivativesGiven the following initial value problem of a 3rd order ordinary Differential Equation y 5y 6y 0 y 0 1 y 0 y 0 0 a Justify that the differential equation is homogeneous linear with constant coefficients 1P b Determine the general solution of the differential equation using the Euler approach y e x and justify why this approach is applicable at all 4P
Calculus
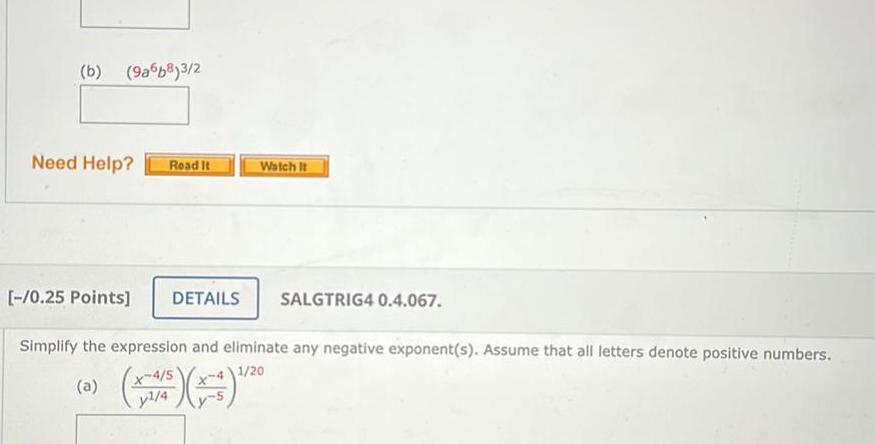
Application of derivativesb 9a6b8 3 2 Need Help Read It Watch It 0 25 Points DETAILS Simplify the expression and eliminate any negative exponent s Assume that all letters denote positive numbers 1 20 a X 4 5 y 4 SALGTRIG4 0 4 067


Calculus
Application of derivatives9 The graph of f consists of the three line segments shown If g x ff t dt find g 4 and g 4 YA paana com 0 f P

Calculus
Application of derivatives5 Let f x 2x tan I COST Write an equation of the tangent line to the curve at the point P 0 1
Calculus
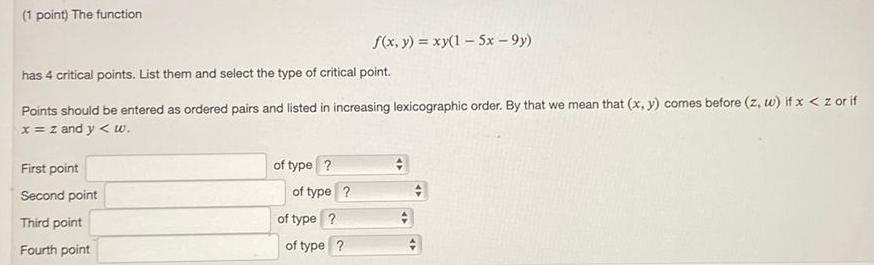
Application of derivatives1 point The function has 4 critical points List them and select the type of critical point Points should be entered as ordered pairs and listed in increasing lexicographic order By that we mean that x y comes before z w if x z or if x z and y w First point Second point Third point Fourth point of type of type f x y xy 1 5x 9y of type of type
Calculus
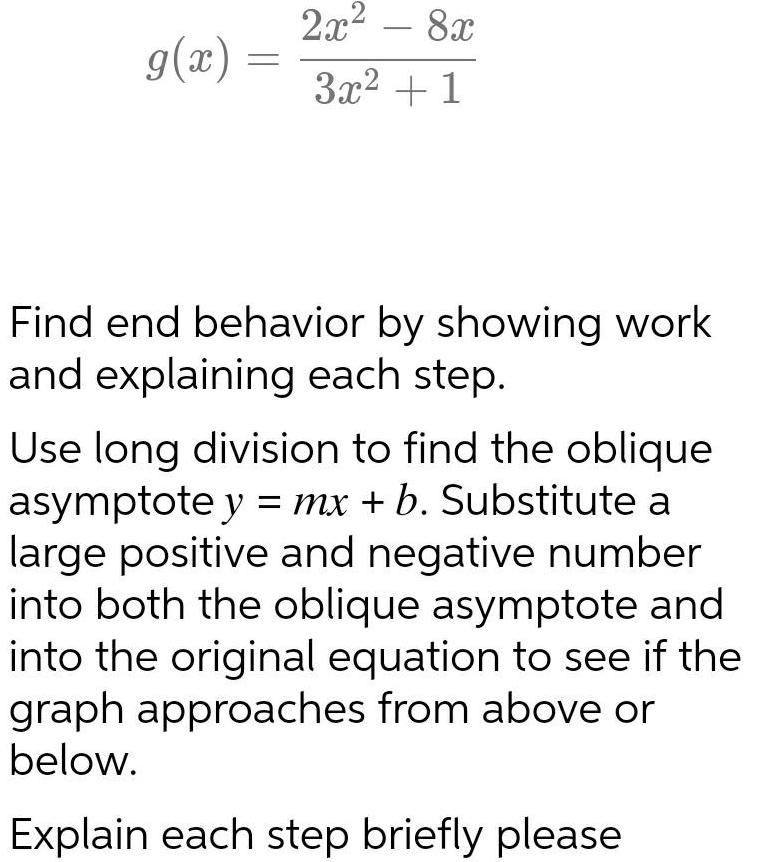
Application of derivativesg x 2x 8x 3x 1 Find end behavior by showing work and explaining each step Use long division to find the oblique asymptote y mx b Substitute a large positive and negative number into both the oblique asymptote and into the original equation to see if the graph approaches from above or below Explain each step briefly please
Calculus
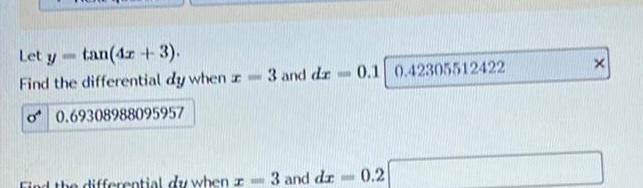
Application of derivativesLet y tan 4z 3 Find the differential dy when z www 3 and dr 0 1 0 42305512422 o 0 69308988095957 Find the differential du when z 3 and dr 0 2 X

Calculus
Application of derivativesX 2000 A television camera at ground level is 2000 feet away from the launching pad of a space rocket that is se to take off vertically as seen in the following figure The angle of elevation of the camera can be found 1 0 tan 200 where is the height of the rocket 0 0 Find the rate of change of the angle of elevation after launch when the camera and the rocket are 5699 feet apart Question Help Video radians per foot Round to five decimal places
Calculus
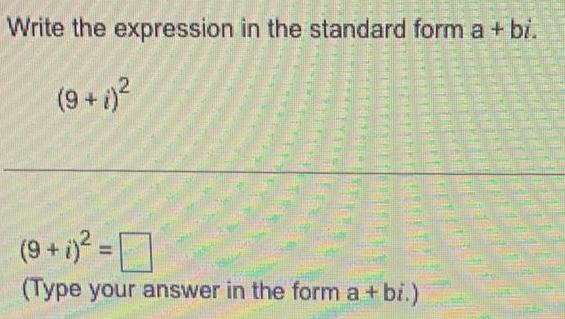
Application of derivativesWrite the expression in the standard form a bi 9 9 i Type your answer in the form a bi
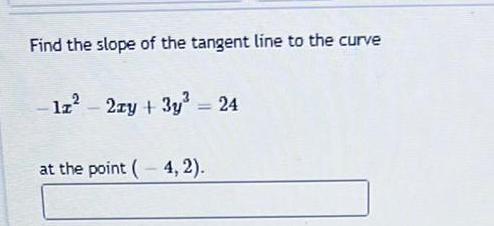
Calculus
Application of derivativesFind the slope of the tangent line to the curve lz 2xy 3y 24 at the point 4 2
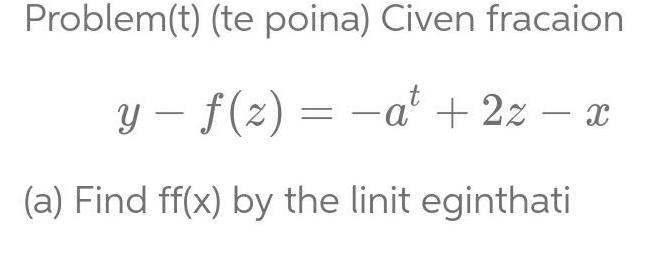
Calculus
Application of derivativesProblem t te poina Civen fracaion t y f 2 a 2z x a Find ff x by the linit eginthati
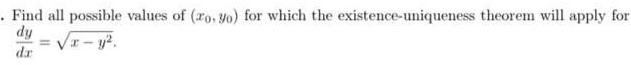
Calculus
Application of derivativesFind all possible values of ro yo for which the existence uniqueness theorem will apply for dy da x y
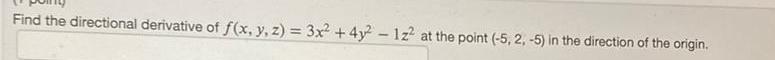
Calculus
Application of derivativesFind the directional derivative of f x y z 3x 4y 12 at the point 5 2 5 in the direction of the origin

Calculus
Application of derivativesFind equations of the tangent plane and normal line to the surface x 12 22 89 at the point 10 7 5 Tangent Plane make the coefficient of x equal to 1 Normal line 10 1 1 0
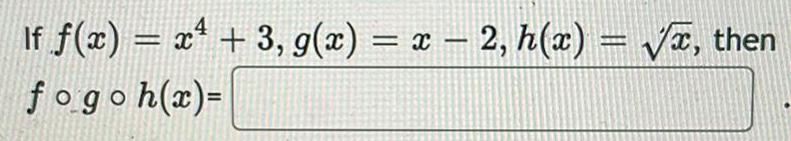

Calculus
Application of derivatives6 A flying distance of 800 km is 10 cm on a map What is the scale factor 1 km 100 000 cm So 800 km scale diagram measurement actual measurement cm Leave scale factor as a fraction in lowest terms

Calculus
Application of derivativesGiven that f x x 2x and g x x 1 find a f g b f g c fg f d and its domain is and its domain is 4 0 9 Note If needed enter co as infinity and co as infinity and its domain is and its domain is x
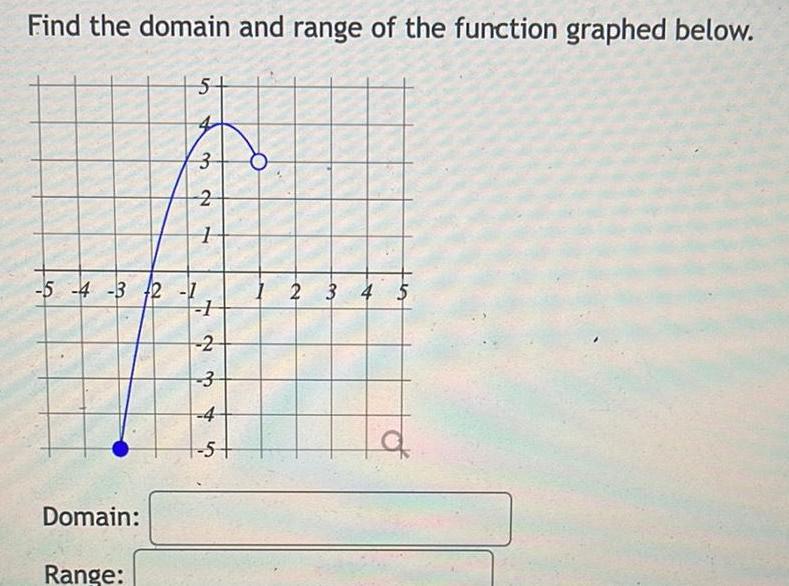
Calculus
Application of derivativesFind the domain and range of the function graphed below Domain 5 5 4 3 2 1 Range 3 2 1 1 234 5 A 1 2 3 4 5 a
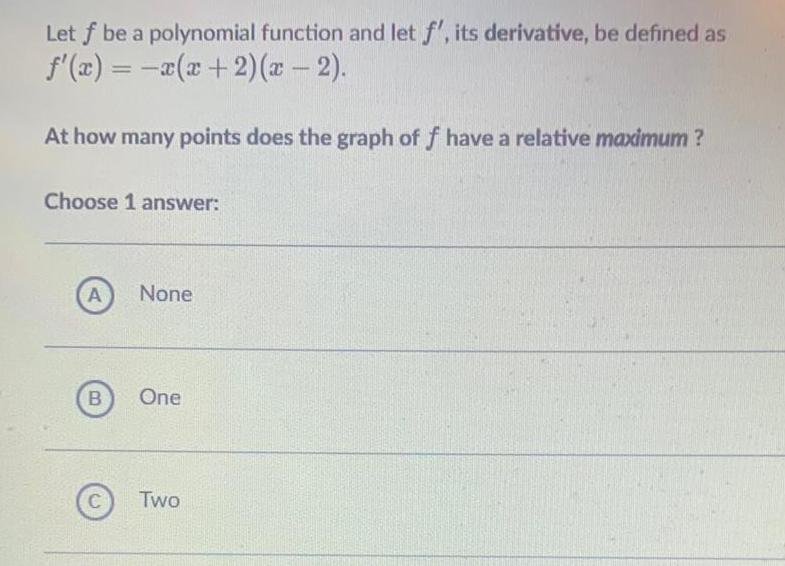
Calculus
Application of derivativesLet f be a polynomial function and let f its derivative be defined as f x x x 2 x 2 At how many points does the graph of f have a relative maximum Choose 1 answer A B None One C Two
Calculus
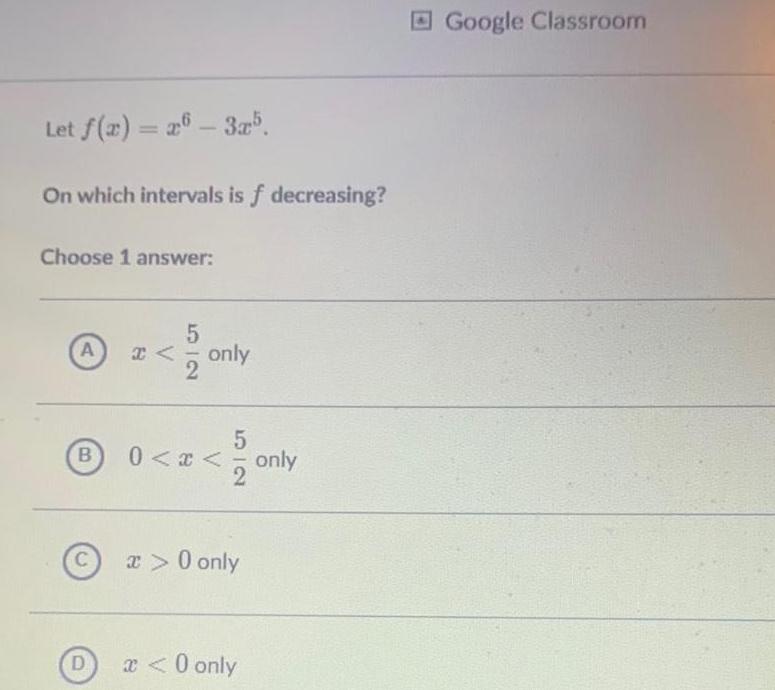
Application of derivativesLet f x 26 3x5 On which intervals is f decreasing Choose 1 answer A B C D 5 only 52 0 x only x 0 only x 0 only Google Classroom

Calculus
Application of derivativesFind the directional derivative of f x y sin x 2y at the point 2 2 in the direction 0 6 The gradient of f is Vf V 2 2 The directional derivative is

Calculus
Application of derivatives1 point Find the domain of the vector functions r t listed below using interval notation a r t In 17t vt 20 0 11 b r t t 1 sin 11 t 1 inf 0 c r t 1 inf e lt VIT 1 12 1 3
Calculus
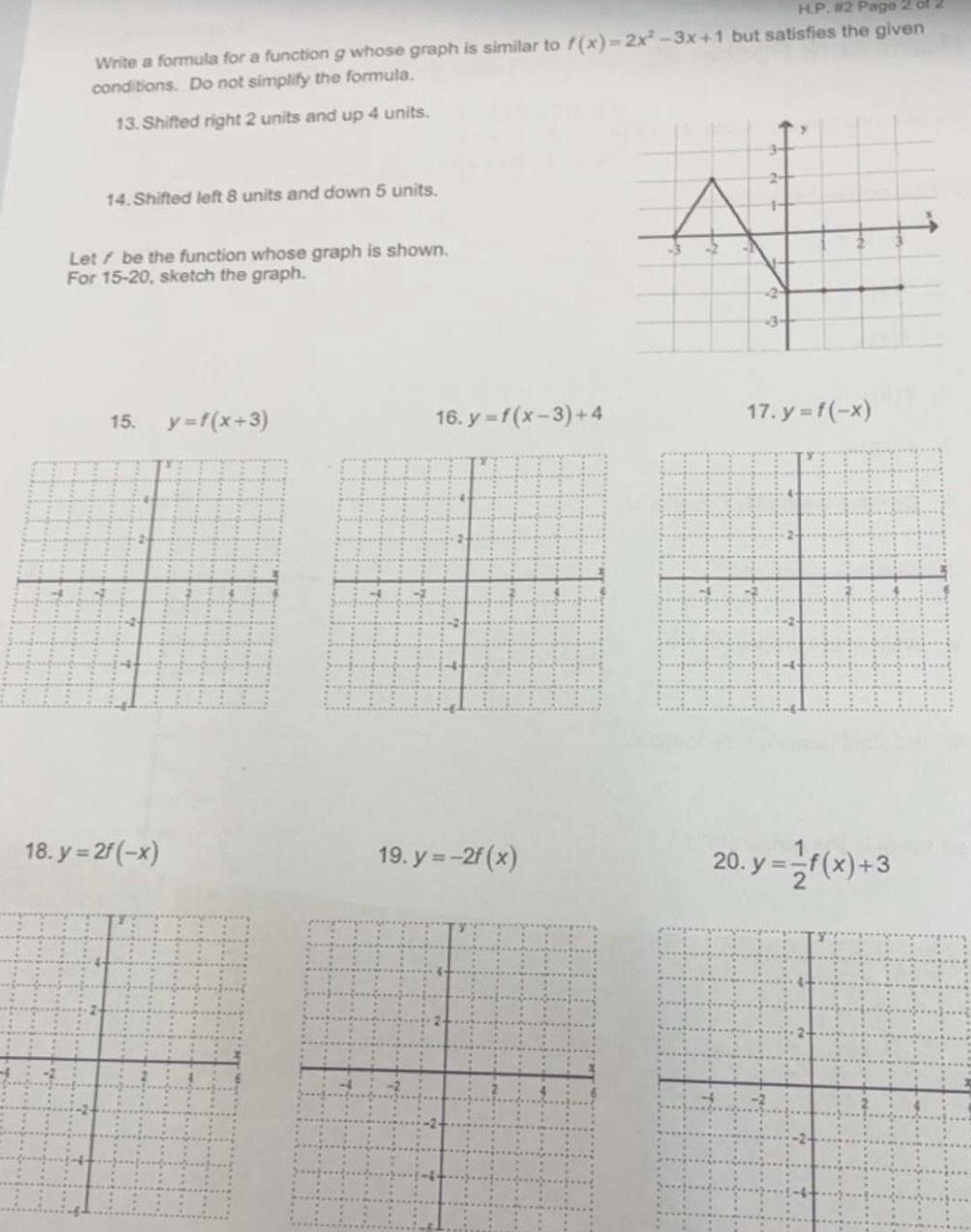
Application of derivativesH P 2 Page 2 of 2 Write a formula for a function g whose graph is similar to f x 2x 3x 1 but satisfies the given conditions Do not simplify the formula 13 Shifted right 2 units and up 4 units 14 Shifted left 8 units and down 5 units Let be the function whose graph is shown For 15 20 sketch the graph 15 18 y 2f x y f x 3 16 y f x 3 4 19 y 2f x 17 y f x y f x 3 20 y


Calculus
Application of derivativesUse the Intermediate Value Theorem to show that the given function has a zero in the interval 0 3 f z z 31 5 f z is continuous f 0 f 3 Number Number on the interval 0 3

Calculus
Application of derivativesAn ant climbs up the trunk of a tree following the path modelled by the circular helix of mathbf r t langle 3 cos t 3 sin t t rangle i Find the length of the trunk helix that has the reference point r 0 3i in terms of t ii If the ant starts at the point 3 0 0 and climbs up the trunk helix for a distance of 10 units what is the ant s final position ii Find the curvature of the path along the trunk helix at the point 3 0 0
Calculus
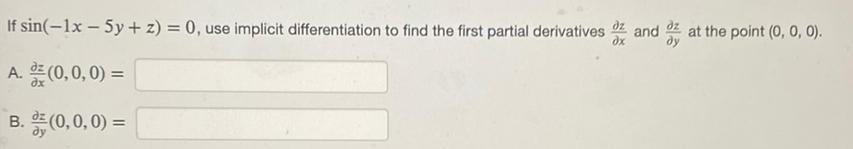
Application of derivativesIf sin 1x 5y z 0 use implicit differentiation to find the first partial derivatives A 0 0 0 B 0 0 0 and at the point 0 0 0

Calculus
Application of derivativesFind the equation of the tangent plane to the surface z 7x5 3y 2xy at the point 3 1 1698 Z
Calculus
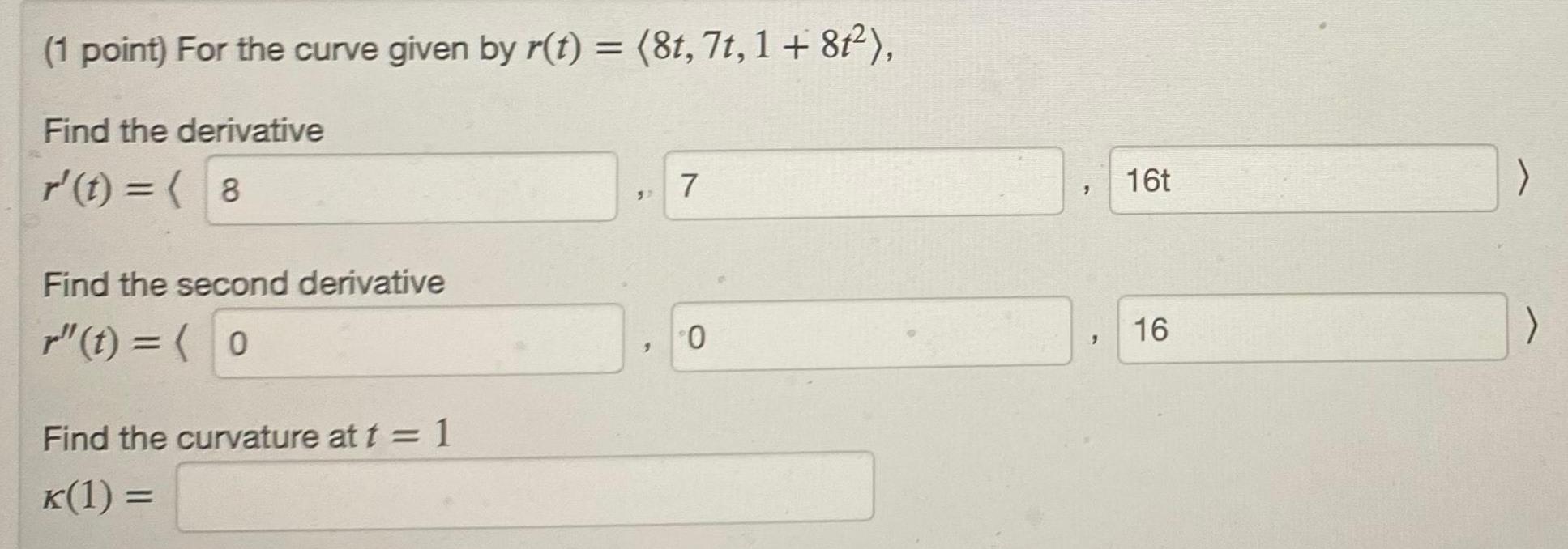
Application of derivatives1 point For the curve given by r t 8t 7t 1 8f Find the derivative r t 8 Find the second derivative r t 0 Find the curvature at t 1 K 1 7 0 16t 16

Calculus
Application of derivativesSketch the region enclosed by y 4x and y 9x Decide whether to integrate with respect to x or y Then find the area of the region

Calculus
Application of derivativesThe table below gives the height and shoe sizes of six randomly selected men 73 5 78 11 Height Shoe size 67 8 5 70 9 5 75 12 13 If a man has a shoc size of 10 5 what would be his predicted height Height expected Equation r 66 8 studying for a science exam x and

Calculus
Application of derivativesDetermine the number of terms n in each arithematic series 0 a 11 a 44 S 330
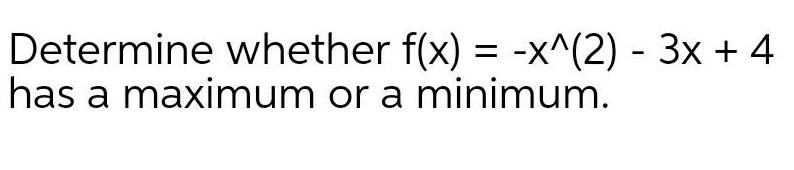
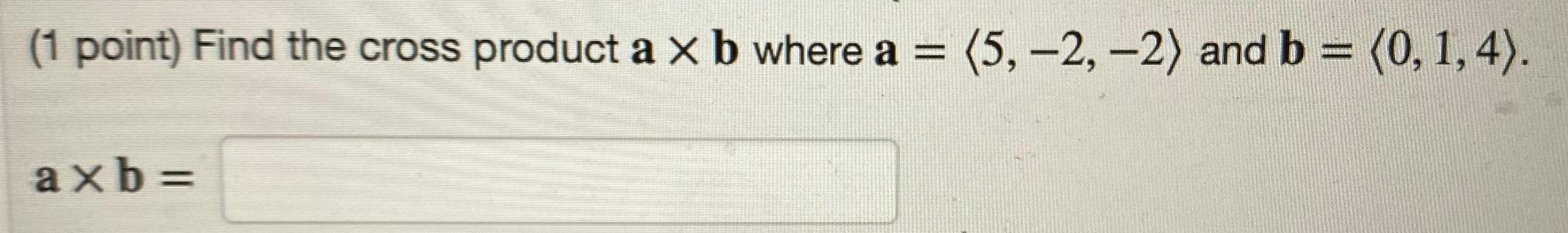

Calculus
Application of derivativesThe velocity of an object in km per minute t minutes after it begins moving along the positive t axis is given by v t Use geometry to evaluate the following quantities Since you are using geometry you must include a graph of the v t function in your work 2 a S v t dt 0 C 1t 2 2t 10 b Upload your handwritten work for part a and then write a sentence that interprets the meaning of the quantity you found in part a Include units in your answer Edit Insert Y U if 0 t 2 if 2 t 4 if 4 t 7 v t dt Y Formats E T BI Ux x II C A AH

Calculus
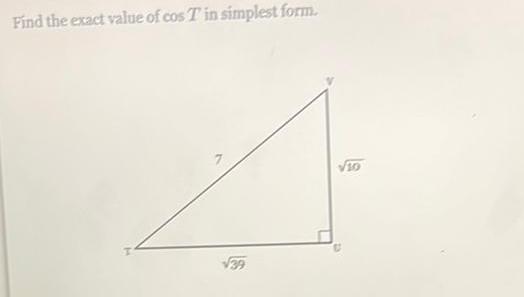
Application of derivativesWatch help video Find the exact value of cos T in simplest form 7 V10

Calculus
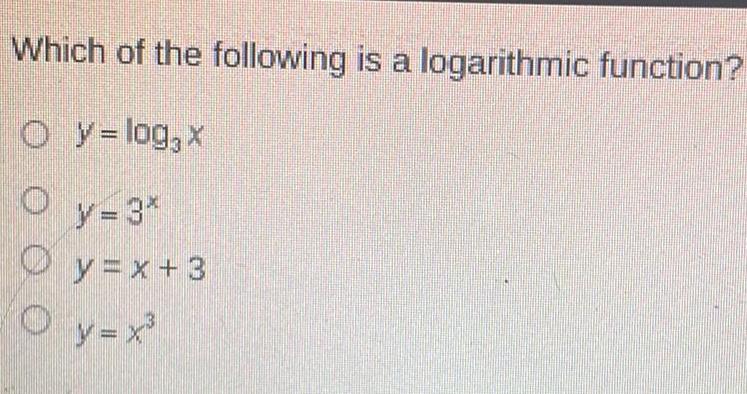
Application of derivativesWhich of the following is a logarithmic function O y log x O y 3 y x 3 O y x