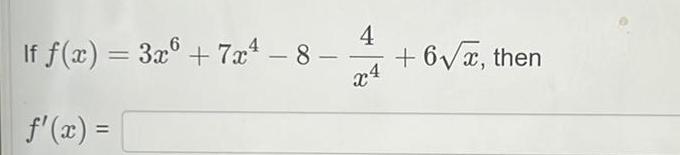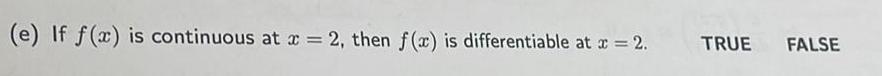Differentiation Questions and Answers
Calculus
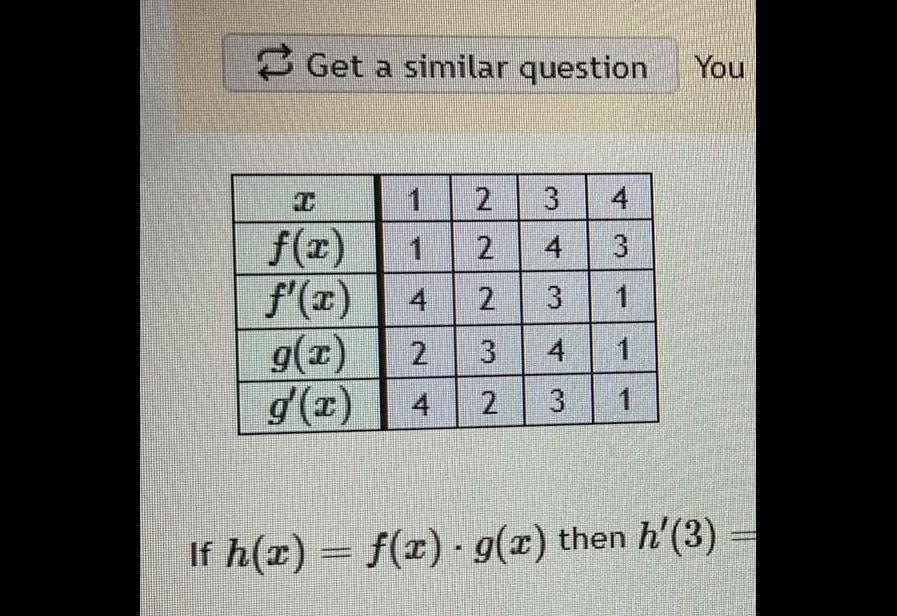
DifferentiationGet a similar question You H 1 22 4 f x 1 f x 4 2 3 4 3 1 4 1 g x 2 3 g x 4 2 3 1 If h x f x g x then h 3
Calculus
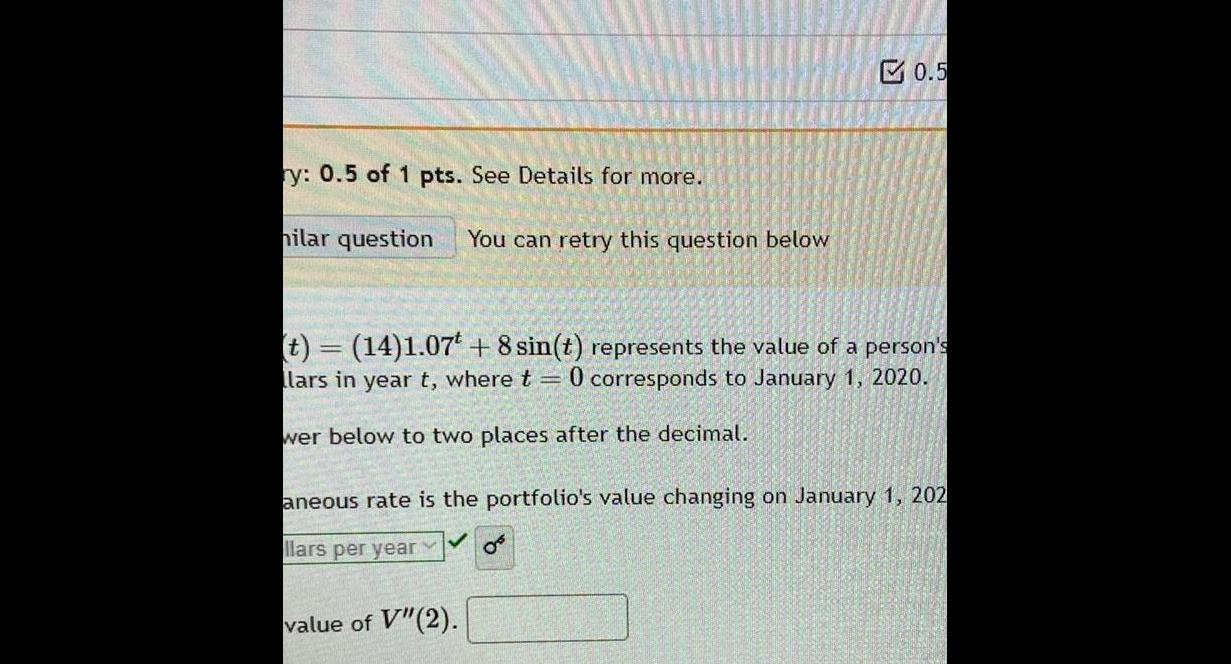
Differentiationry 0 5 of 1 pts See Details for more hilar question You can retry this question below 0 5 t 14 1 07 8 sin t represents the value of a person s llars in year t where t 0 corresponds to January 1 2020 wer below to two places after the decimal aneous rate is the portfolio s value changing on January 1 202 llars per year value of V 2 0
Calculus
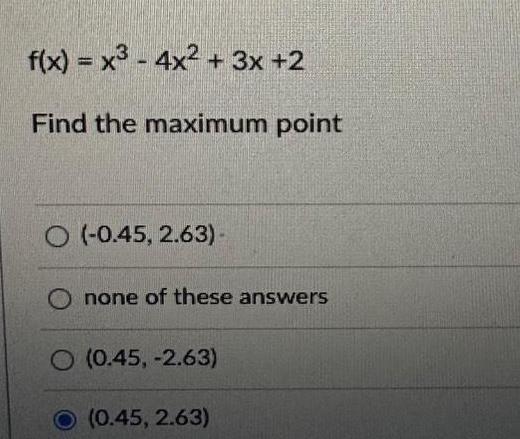
Differentiationf x x 4x 3x 2 Find the maximum point O 0 45 2 63 O none of these answers O 0 45 2 63 0 45 2 63

Calculus
Differentiation20 To answer questions 18 20 use the formulas for functions of the difference and sum of two angles to find without tables or calculator the cosine sine and tangent of 255 tan 255 A 3 3 3 3 OB 3 3 3 3 OC 3 3 3 3 OD 3 3 3 3
Calculus
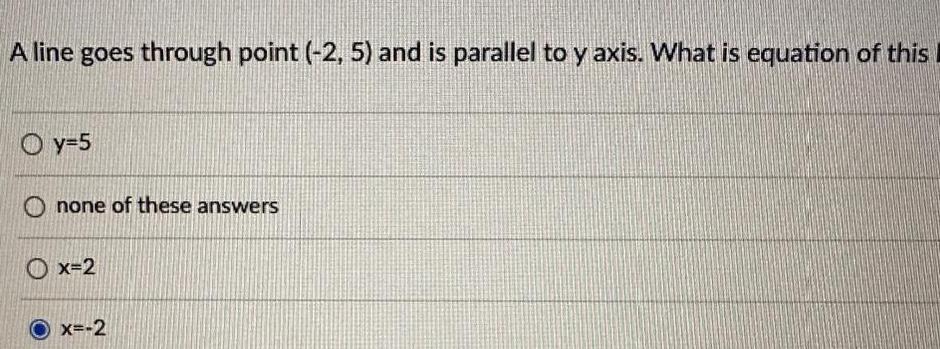
DifferentiationA line goes through point 2 5 and is parallel to y axis What is equation of this Oy 5 none of these answers x 2 x 2
Calculus
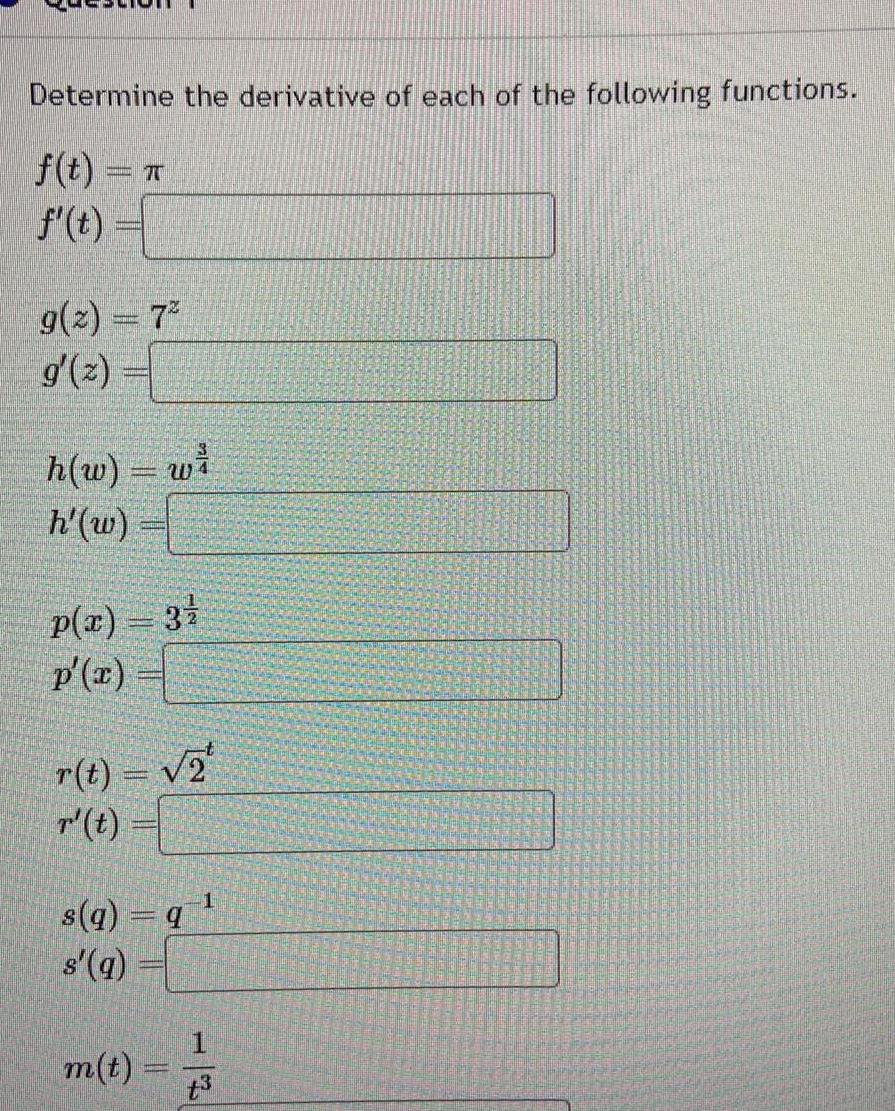
DifferentiationDetermine the derivative of each of the following functions f t T g 2 7 g z h w w wa h w p x 31 p x r t 2 r t 1 s q q s q 1 m t 7 7 3 3
Calculus
DifferentiationIviarshmallow t n last try 0 of 1 pts See Details for et a similar question You can retry is a function with f 17 15 and he following values of f

Calculus
Differentiation1 point The solution of a certain differential equation is of the form y t a exp 51 b exp 61 where a and b are constants The solution has initial conditions y 0 1 and y 0 1 Find the solution by using the initial conditions to get linear equations for a and b y t


Calculus
DifferentiationIf f x 3arcsec 8x find f x f x Find 3 3 NOTE Different choices can be made for the output range of the inverse secant function which lead to different formulas for derivative of the inverse secant For this problem you need to use the formula given in the textbook That formula does NOT i an absolute value
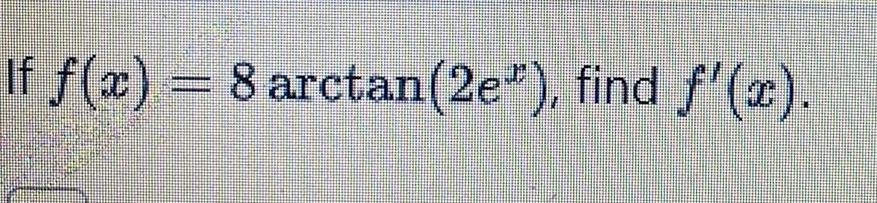

Calculus
Differentiationestion 3 ose that f is a function given as f x plify the expression f a h h e an expression for f x h f x h f x I writing as a single fraction using a common denominator si x h f x h x h f x h e derivative of the function at is the limit of the difference

Calculus
DifferentiationThe average time spent on food growth on collection averaged across all humans ie in the global human day is 0 8 h person day 1 1 a Estimate the total daily rate of somatic energy in J per day that is currently invested globally by all persons in this activity Assume an average total MET for this activity of 4 an energy consumption of 1 1 W kg per MET and average human mass of 50 kg b Assume each person in the global population consumes 10MJ of food energy per day which represents the total energy provided by all food growth and collection What is the ratio between this total food energy provided to all humans and the food energy consumed in order to do the agricultural activity in part 2 2 In other words how many joules of food energy are provided for every
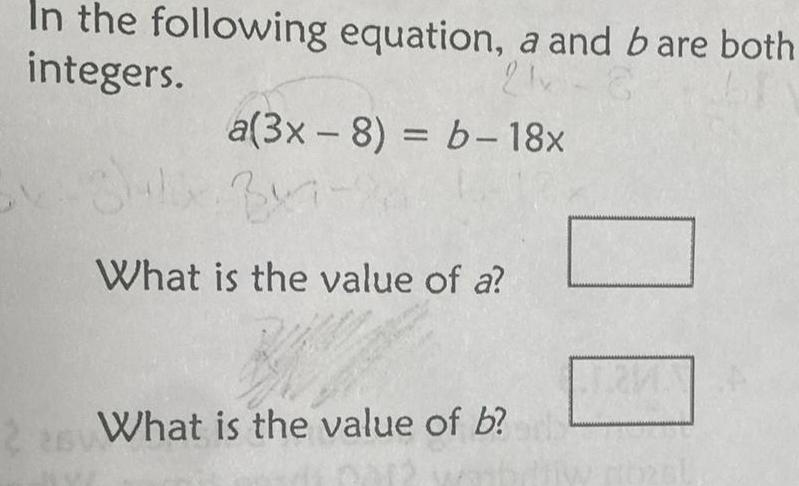
Calculus
DifferentiationIn the following equation a and bare both integers 21x 26 a 3x 8 b 18x 347 2 3 What is the value of a What is the value of b 0 129
Calculus
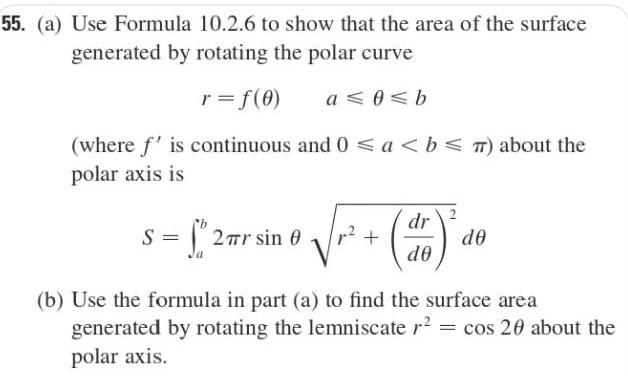
Differentiation55 a Use Formula 10 2 6 to show that the area of the surface generated by rotating the polar curve r f 0 a 0 b where f is continuous and 0 a b 7 about the polar axis is S 2 r sin 0 b Use the formula in part a to find the surface area generated by rotating the lemniscate r cos 20 about the polar axis dr 10 do


Calculus
Differentiationd Consider the linear control system begin aligned frac d x 1 dt x 2 u frac d x 2 d t b x 1 x 2 y x 1 x 2 end aligned where x x t ER and x2 x t R represent the state U u x x E R is the input YER is the output and bER is the control parameter i Is this system observable Explain your answer ii Is

Calculus
Differentiationy 7x 3 3x 2 yo a Apply the Product Rule Let u Rule and b multiplying the factors to produce a sum of simpler terms to differentiate 7x 3 and v 3x y Simplify your answer Find y y 0 3x 2 d UV 7x 3 3x 2 E dx b Multiply the factors of the original expression u and v to produce a sum of simpler terms
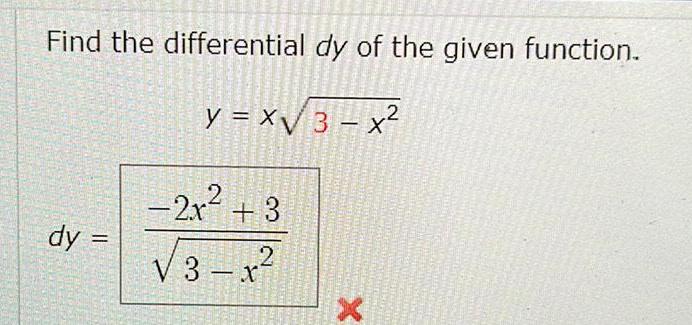

Calculus
DifferentiationFind the differential dy of the given function y 5x sin x dy 5 2 sin x cos x X

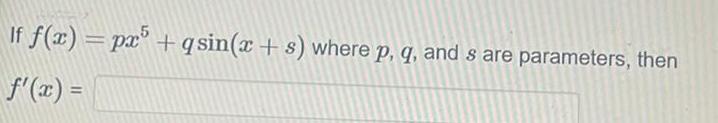
Calculus
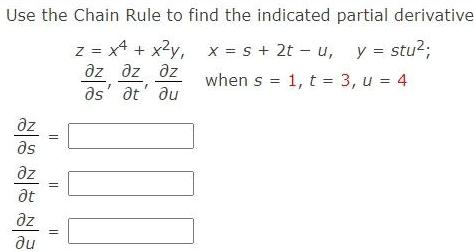
DifferentiationUse the Chain Rule to find the indicated partial derivative Z z x x y x y x s 2t u y stu z z z when s 1 t 3 u 4 as at du z s z at az du

Calculus
DifferentiationIn this question we will investigate the number of intervals necessary to achieve a given level of accuracy using Simpson s method To make the project concrete we focus on the integra dz I a Let f x Find the fourth derivative of f x b Which of the following best describes the behaviour of f 4 z on the interval 1 1 7 O Increasing Decreasing Sometimes increasing sometimes decreasing c What is the maximum value of f 4 z on the interval 1 1 7 max f x 15 51 7 13 in an equivalent form where n need not be an integer d Let L denote the value found above Taking a 1 and b 1 7 and assuming n 0 isolate n to express the inequality L b a 1 S 180 n 107 n H e Use the results above to find the smallest even integer n for which 1 1 7 1


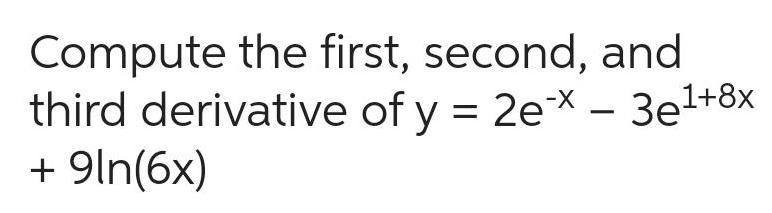

Calculus
Differentiationxy 2 calculate the values of W Aw and the total differential to dw when x y z goes from 3 2 1 2 9 2 1 1 05 Round your answer for Aw to three decimal places 10 4 Find both points on the surface xzx y 8 where the tangent plane is horizontal
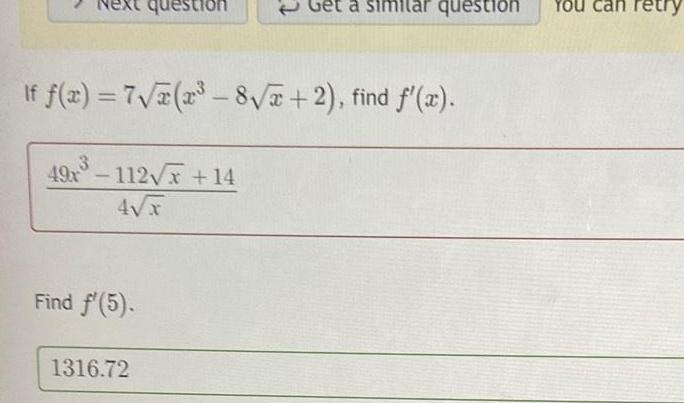
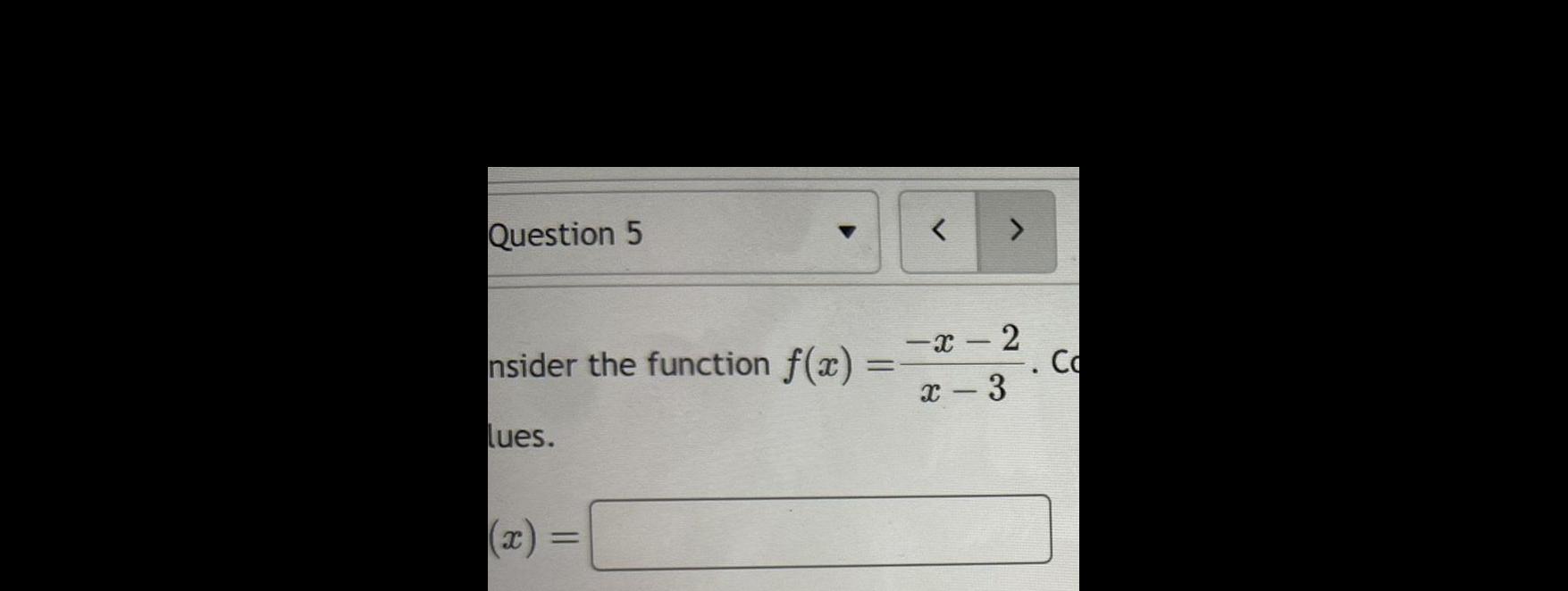
Calculus
DifferentiationIf f x 7 x 2 8 x 2 find f x 3 49x 112 x 14 4 x Find f 5 bet a similar question 1316 72 You can
Calculus
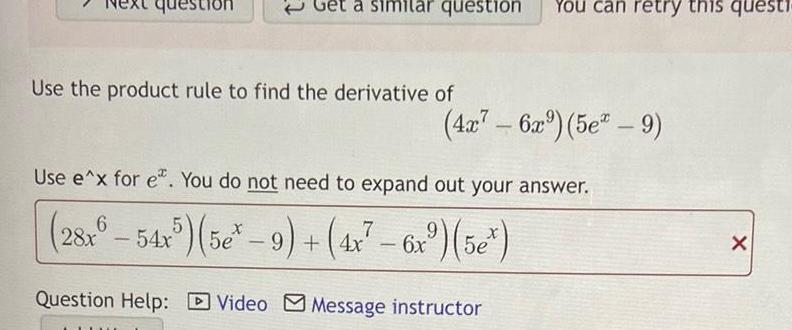
Differentiationquestion Get a simil question You can retry this questi Use the product rule to find the derivative of 4x 6x 5e 9 Use e x for e You do not need to expand out your answer 28x6 54x5 5e 9 4x 6x 5e 6x Question Help Video Message instructor X

Calculus
DifferentiationFind the second derivative for the following functions 1 f x xsin x 2 f x cot x
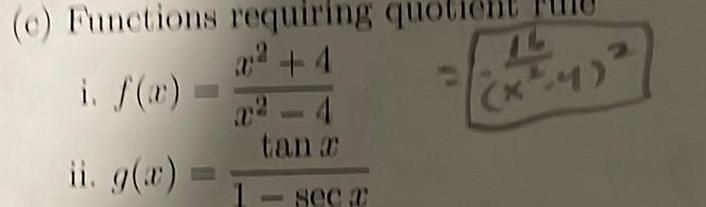

Calculus
DifferentiationAn object is dropped from a tower 174 ft above the ground The object s height above ground t sec into the fall is s 174 161 a What is the object s velocity speed and acceleration at time t b About how long does it take the object to hit the ground c What is the object s velocity at the moment of impact The object s velocity at time t is The object s speed at time t is ft sec The object s acceleration at time t is ft sec Simplify your answer It takes sec for the object to hit the ground Round to the nearest tenth The object s velocity at the moment of impact is ft sec Round to the nearest tenth

Calculus
DifferentiationA 10 ft ladder is leaning against a house when its base starts to slide away By the time the base is 8 ft from the house the base is moving away at the rate of 18 fusec a What is the rate of change of the height of the top of the ladder b At what rate is the area of the triangle formed by the ladder wall and ground changing then c At what rate is the angle between the ladder and the ground changing then a The rate of change of the height of the top of the ladder is fusec Simplify your answer b The area is changing att sec Simplify your answer This question 1 point s possible c The angle is changing atrad sec Simplify your answer y 1 0

Calculus
DifferentiationThe diameter of a tree was 20 in During the following year the circumference increased 3 in About how much did the tree s diameter increase About how much did the tree s cross sectional area change The tree s diameter increased by Type an exact answer using z as needed The estimated change in area is Type an integer or a decimal Do not round CLIS

Calculus
DifferentiationAssume that all variables are implicit functions of time t Find the indicated rate dy dt dx x 4 6y 14 1 when x 4 and y 1 find Simplify your answer
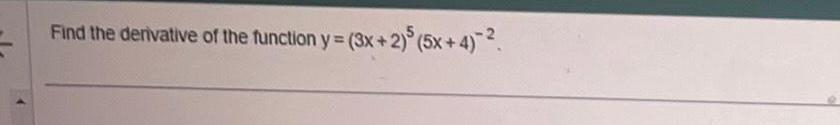

Calculus
DifferentiationWrite the function in the form y f u and u g x Then find dy y e 7x Choose the correct form of y in terms of u OA y u 7x where u e OB y e where u 3 7 OC y e3 7u where u x OD y e where u 3 7x 11 as a function of x
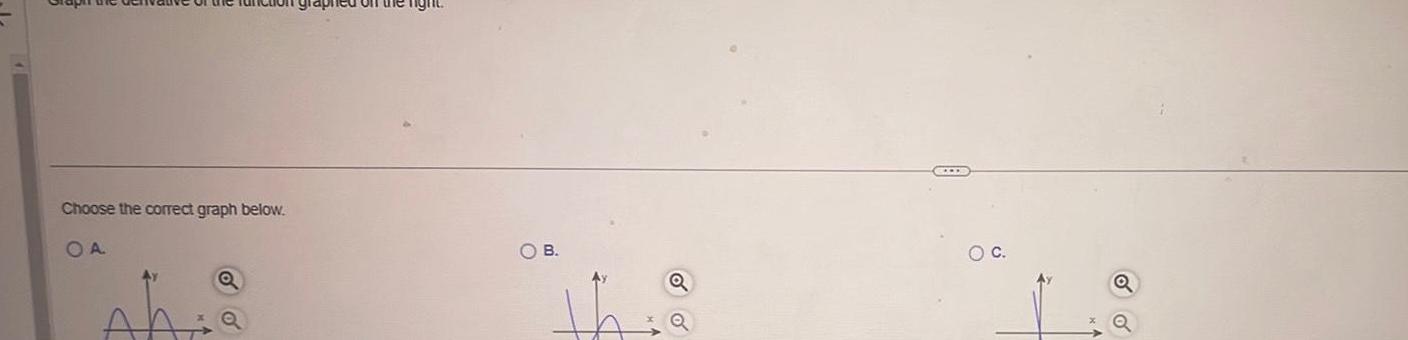

Calculus
DifferentiationAranscript Find the slope of the function s graph at the given point Then find an equation for the line tangent to the graph there f x 4x 5x 2 6 The slope of the function s graph at 2 6 is Simplify your answer The equation for the tangent line through 2 6 is y

Calculus
DifferentiationA function value and a quadrant are given Find the other five function values Give exact answers cot 0 2 Quadrant II sin 0 cos 0 tan 0 Simplify your answer Type an exact answer using radicals as needed Rationalize all dond

Calculus
DifferentiationUsing the functions defined below determine the indicated values f x 3 10x g x x x 5 a g 2 b f 3 g 2 c g 2 f 3

Calculus
DifferentiationConsider the following 6x 7x a Factor the given expression b Set the expression equal to zero and solve for x Enter your answers as a comma separate X

Calculus
Differentiation17 Relative change in blood flow Another law of Poiseuille says that when blood flows along a blood vessel the flux F the volume of blood per unit time that flows past a given point is proportional to the fourth power of the radius R of the blood vessel KR We will show why this is true in Section 6 3 A partially clogged artery can be expanded by an operation called angioplasty in which a balloon tipped catheter is inflated inside the artery in order to widen it and restore the normal blood flow Show that the relative change in F is about four times the relative change in R How will a 5 increase in the radius affect the flow of blood
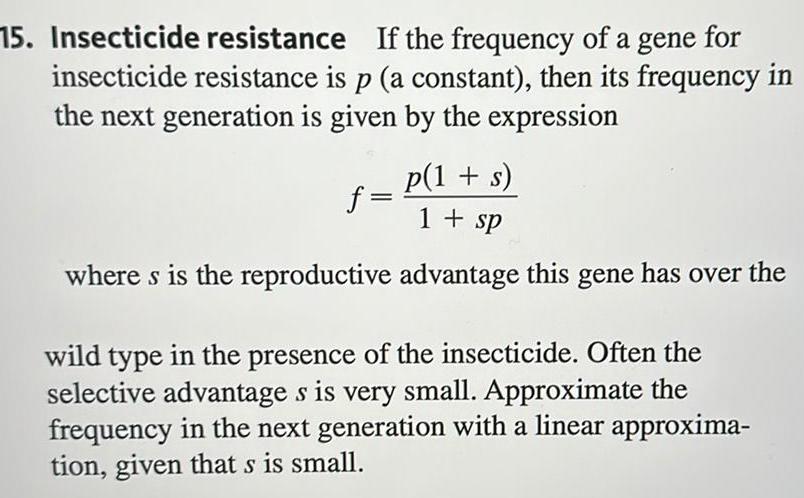
Calculus
Differentiation15 Insecticide resistance If the frequency of a gene for insecticide resistance is p a constant then its frequency in the next generation is given by the expression p 1 s 1 sp where s is the reproductive advantage this gene has over the f wild type in the presence of the insecticide Often the selective advantage s is very small Approximate the frequency in the next generation with a linear approxima tion given that s is small