Indefinite Integration Questions and Answers

Calculus
Indefinite Integration3 Determine the equation of the tangent line to the curve f x x 6 at x 10D A3
Calculus
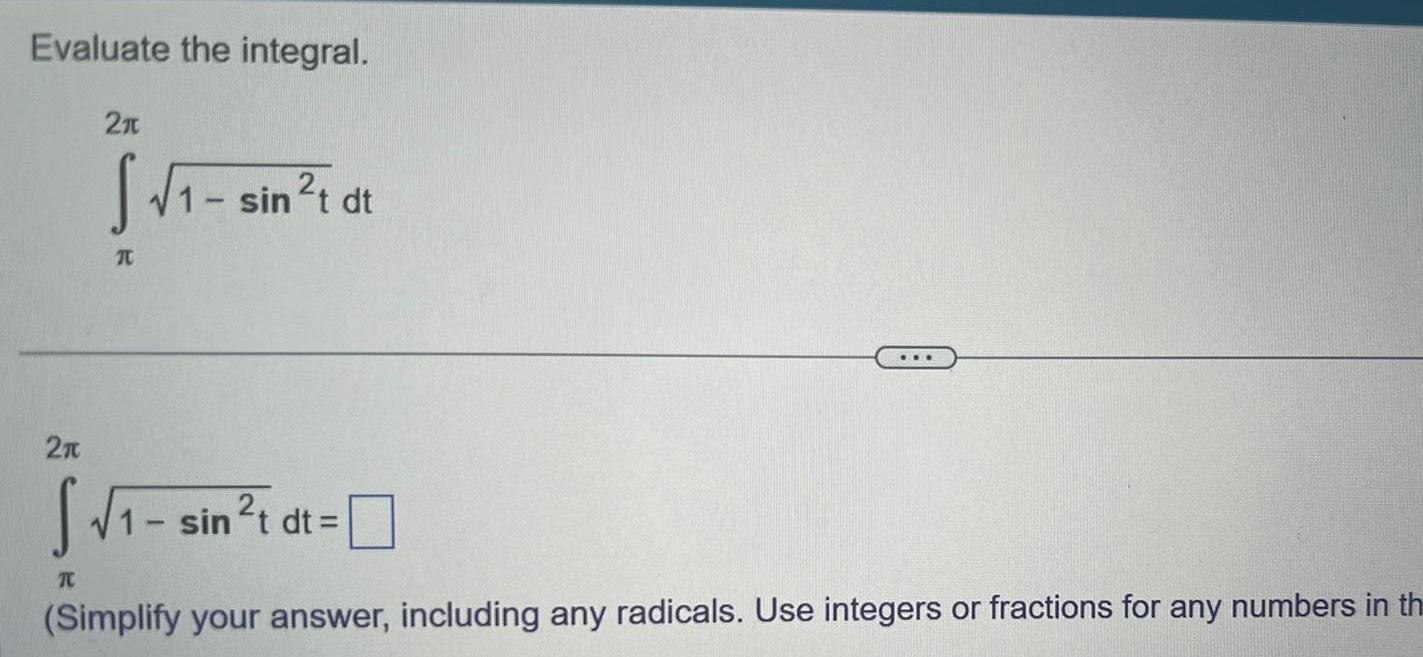
Indefinite IntegrationEvaluate the integral 2n 21 TC S sin t dt I si sin t dt Simplify your answer including any radicals Use integers or fractions for any numbers in th

Calculus
Indefinite IntegrationSS S p sin dp do do 0 0 0 Evaluate the spherical coordinate integral The value is Type an exact answer using as needed

Calculus
Indefinite IntegrationDetermine if the improper integral converges and if so evaluate it dr 8 2 Write F if the integral doesn t converge
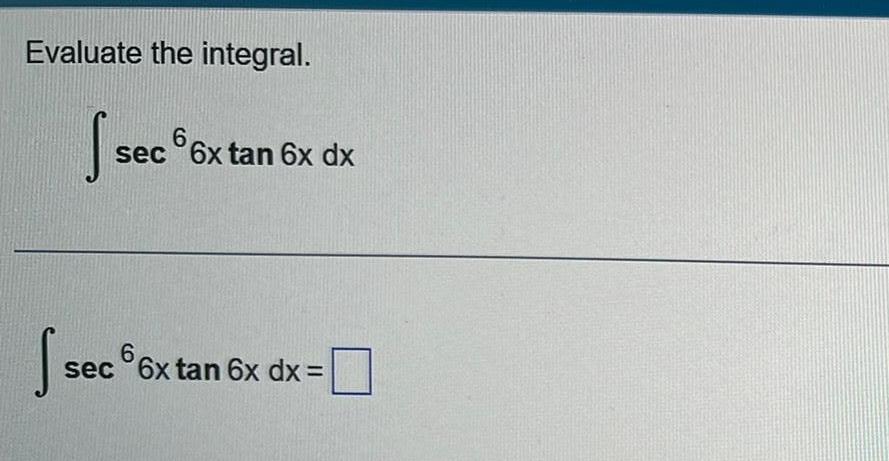
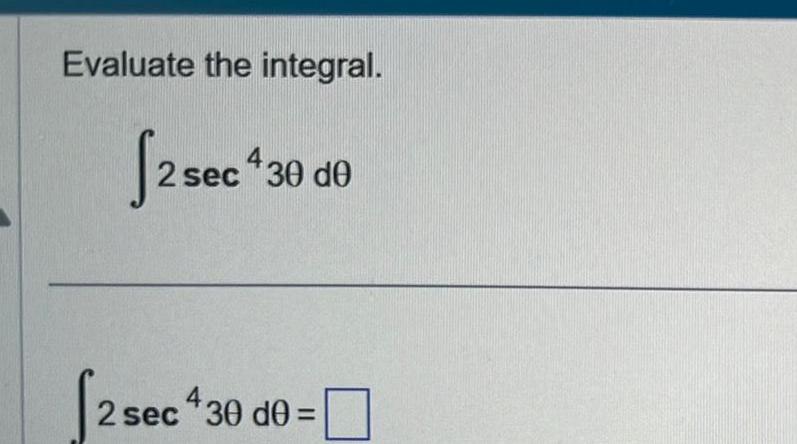

Calculus
Indefinite IntegrationBox Office Receipts The total worldwide box office receipts for a long running movie are approximated by the following function where 7 x is measured in millions of dollars and x is the number of years since the movie s release T x 120x x 4 How fast are the total receipts changing 1 yr 4 yr and 6 yr after its release Round your answers to two decimal places after 1 yr 38 40 million year after 4 yr after 6 yr S million year million year

Calculus
Indefinite IntegrationThe graphs of f and g are given Find a formula for the function g g x 5 an g y 5 5 f x x 50 X

Calculus
Indefinite IntegrationThe gross domestic product G of Switzerland was 685 4 billion dollars in 2013 Give a formula for G in billions of dollars t years after 2013 if G increases by a 4 per year G t b 6 billion dollars per year G t

Calculus
Indefinite Integration2 A particle moves with position function s t4 4t 20t 20t t 0 a At what time does the particle have a velocity of 20 m b At what time is the acceleration 0 What is the significance of this value of t
Calculus
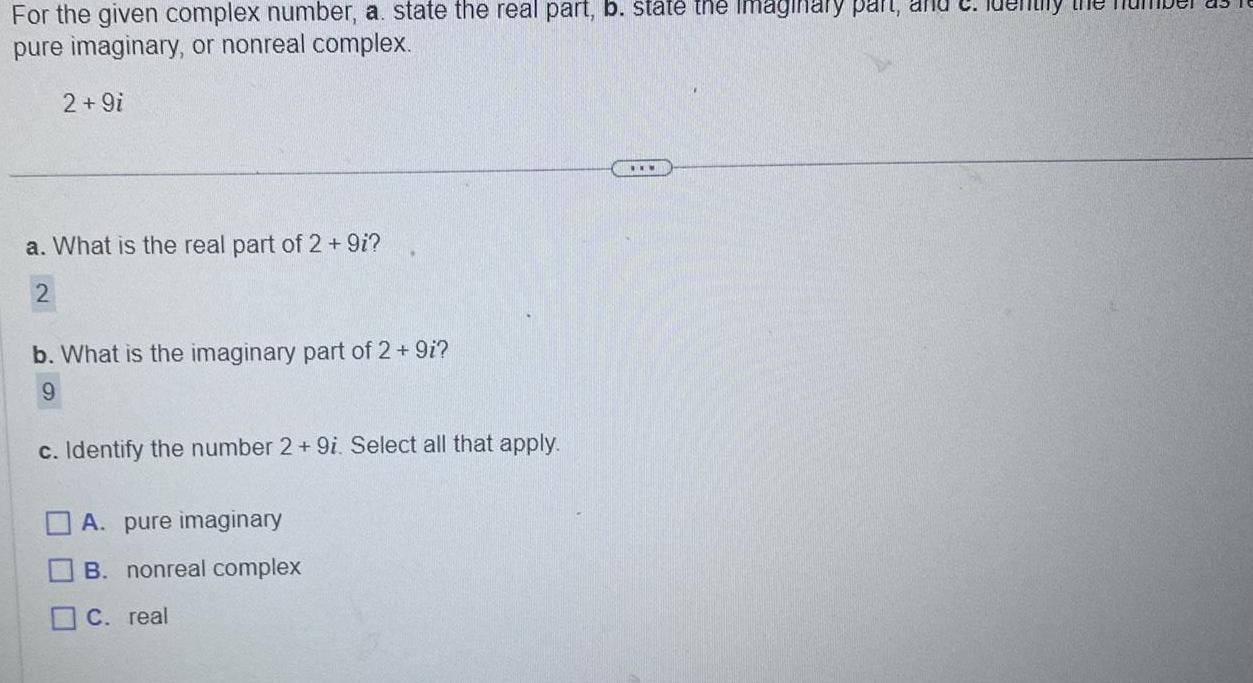
Indefinite IntegrationFor the given complex number a state the real part b state the Imaginary part and pure imaginary or nonreal complex 2 9i a What is the real part of 2 9i 2 b What is the imaginary part of 2 9i 9 c Identify the number 2 9i Select all that apply A pure imaginary B nonreal complex C real y the nu

Calculus
Indefinite IntegrationSuppose f 5 9 and f 5 2 1 The equation of the tangent line of f at 5 is t x 2 Approximate f 5 46 to the nearest 2 decimal places f 5 46 A
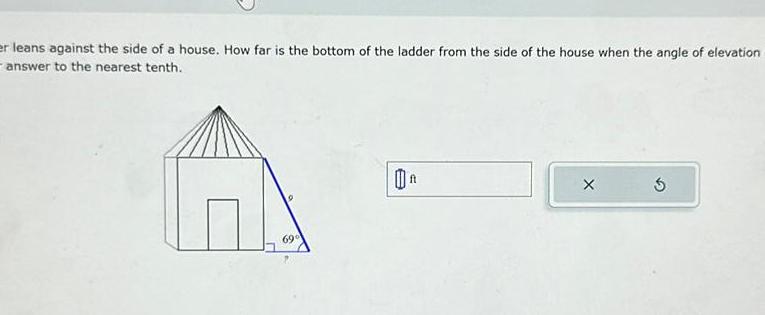
Calculus
Indefinite Integrationer leans against the side of a house How far is the bottom of the ladder from the side of the house when the angle of elevation answer to the nearest tenth 69 0 X

Calculus
Indefinite IntegrationConsider a triangle ABC like the one below Suppose that B 58 C 67 and a 30 The figure is not drawn to scale Solve the triangle Round your answers to the nearest tenth If there is more than one solution use the button labeled or A 0 b 0 c 0 or O X No solution

Calculus
Indefinite IntegrationSolve the triangle using the Law of Sines Assume b and c 19 and ZC 63 Round the length to two decimal places a LA 28 A B
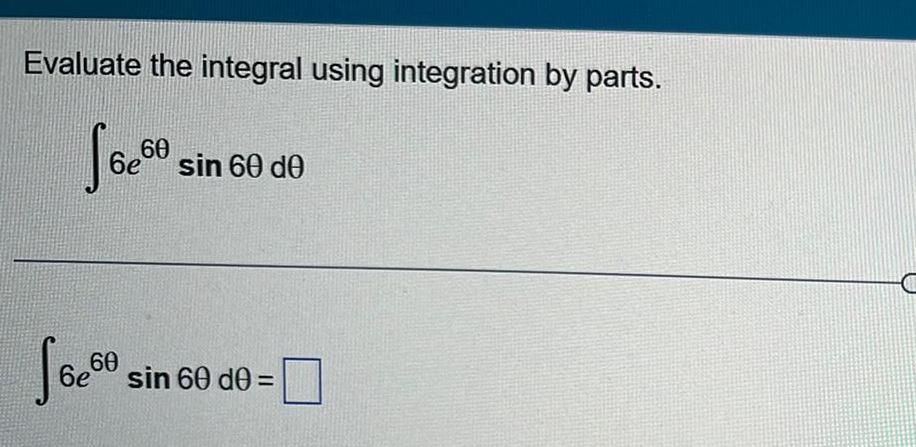
Calculus
Indefinite IntegrationEvaluate the integral using integration by parts Jo 60 6e 60 6e sin 60 de sin 60 d0
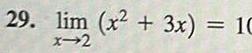
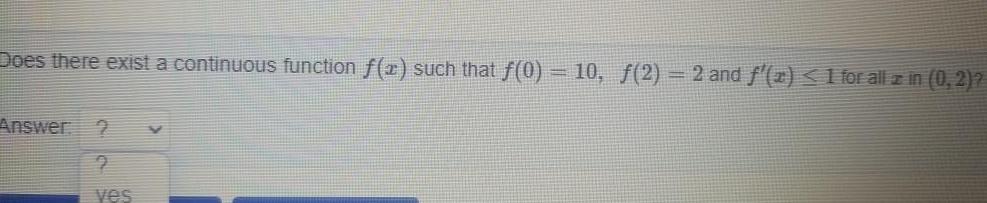
Calculus
Indefinite IntegrationDoes there exist a continuous function f x such that f 0 10 2 2 and f x 1 for all z in 0 2 Answer 2 9 ves

Calculus
Indefinite IntegrationFind all angles 8 between 0 and 180 satisfying the given equation Round your answer to one decimal place Enter your answers as a comma separated list Enter your answer in degrees sin 8 H 6
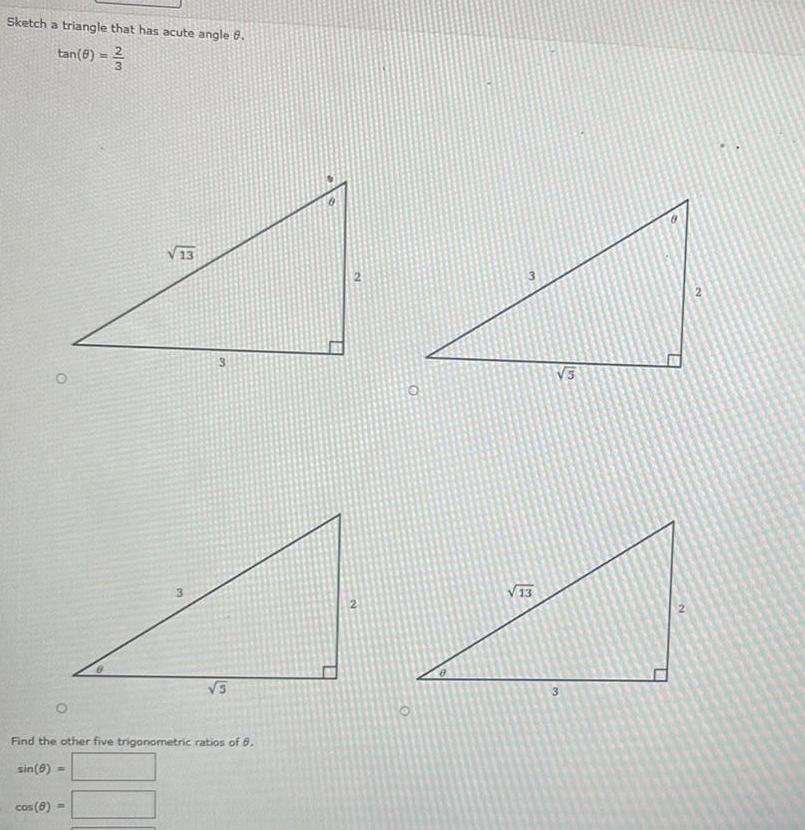
Calculus
Indefinite IntegrationSketch a triangle that has acute angle 8 tan 8 O cos 8 3 12 3 3 Find the other five trigonometric ratios of 8 sin 6 3 O 3 13 3 Cal N

Calculus
Indefinite Integrationa Evaluate the integral 2 32 x 4 0 Your answer should be in the form kr where k is an integer What is the value of k daretan x Hint do ao a1 32 b Now lets evaluate the same integral using power series First find the power series for the function f x 34 Then integrate it from 0 to 2 4 and call it S S should be an infinite series What are the first few terms of S P da a2 a3 1 as c The answers to part a and b are equal why Hence if you divide your infinite series from b by k the answer to a you have found an estimate for the value of 7 in terms of an infinite series Approximate the value of by the first 5 terms d What is the upper bound for your error of your estimate for if you use the first 7 terms Use the alternating series estimation 01 K

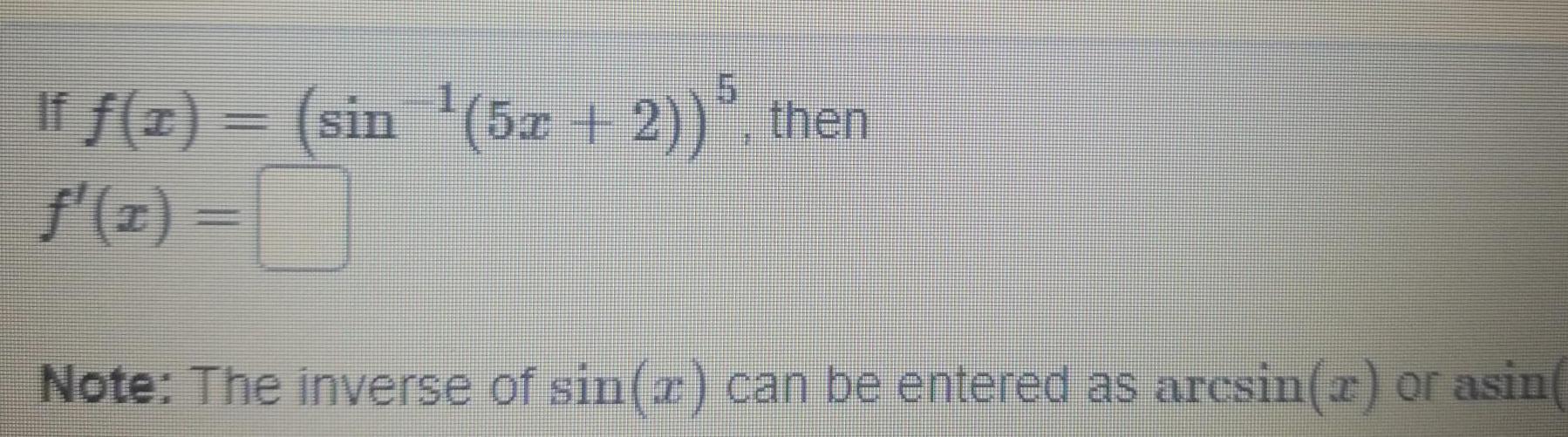
Calculus
Indefinite IntegrationIf f x sin 5x 2 then f x Note The inverse of sin z can be entered as arcsin r or asin
Calculus
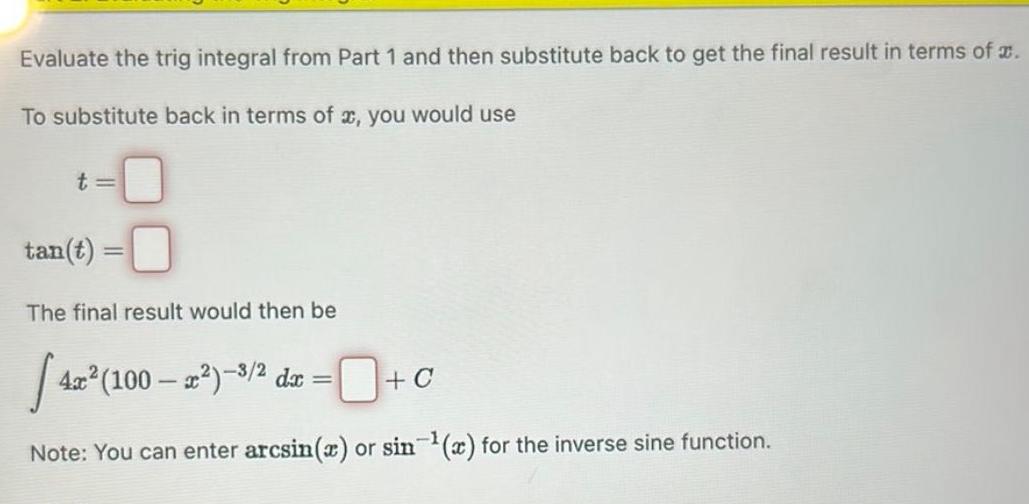
Indefinite IntegrationEvaluate the trig integral from Part 1 and then substitute back to get the final result in terms of To substitute back in terms of x you would use t tan t The final result would then be 42 4x 100 x 3 2 dx C Note You can enter arcsin x or sin x for the inverse sine function
Calculus
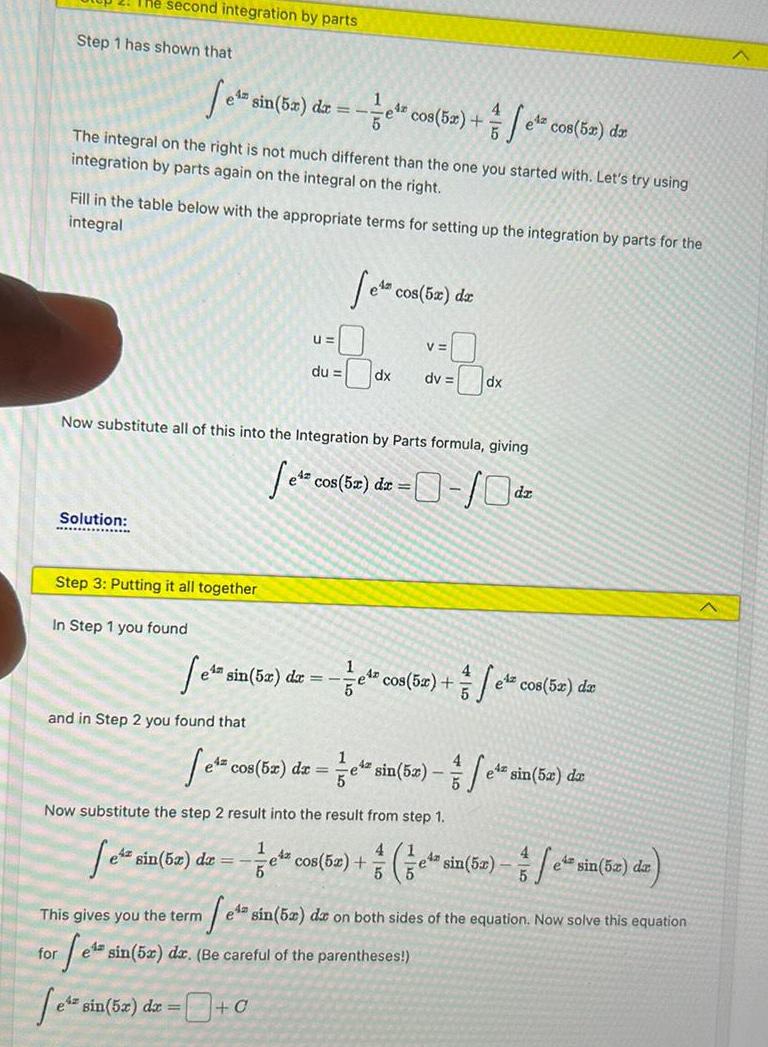
Indefinite Integration2 The second integration by parts Step 1 has shown that 1 4 e s sin 5x dx e cos 5x The integral on the right is not much different than the one you started with Let s try using integration by parts again on the integral on the right Solution Fill in the table below with the appropriate terms for setting up the integration by parts for the integral Step 3 Putting it all together In Step 1 you found and in Step 2 you found that U du ez sin 5x dx 1 5 e s cos 5x da 0 Now substitute all of this into the Integration by Parts formula giving e cos 5x dx 0 10 dx V 1 fe co dv dx e sin 5x dx e cos 5x fe el cos 5x dx cos 5x dx e cos bx dx e sin 52 e sin 52 da 5 Now substitute the step 2 result into the result from step 1 4 Setz 42 cos 52 e sin 5x fe 5 5 e sin 5x da This gives you the term e sin 52 dar on both sides of the equation Now solve this equation fets sin 52 dz Be careful of the parentheses for e sin 5x dx 0
Calculus
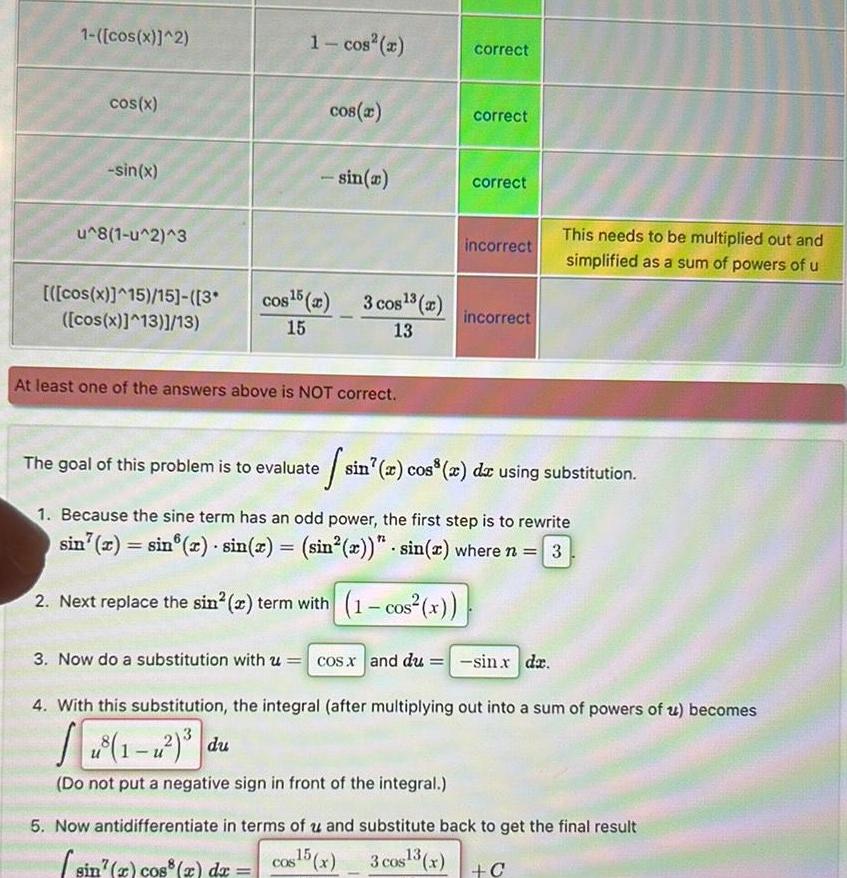
Indefinite Integration1 cos x 2 cos x sin x u 8 1 u 2 3 cos x 15 15 3 cos x 13 13 1 cos cos x sin x cos 5 2 3 cos 2 15 13 At least one of the answers above is NOT correct correct correct correct incorrect incorrect The goal of this problem is to evaluate sin x cos x dx using substitution 1 Because the sine term has an odd power the first step is to rewrite sin x sin x sin x sin x sin x where n 3 2 Next replace the sin 2 term with 1 cos x This needs to be multiplied out and simplified as a sum of powers of u 3 Now do a substitution with u cos x and du sin x dx 4 With this substitution the integral after multiplying out into a sum of powers of u becomes Do not put a negative sign in front of the integral 5 Now antidifferentiate in terms of u and substitute back to get the final result cos15 x 3 cos 3 x sin x cos x dx C
Calculus
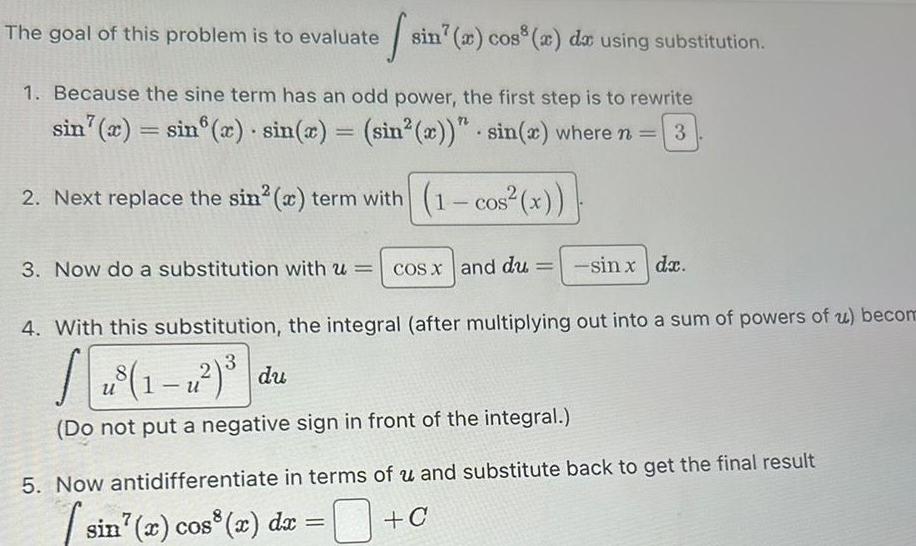
Indefinite IntegrationThe goal of this problem is to evaluate 1 Because the sine term has an odd power the first step is to rewrite sin x sin x sin x sin x sin x where n 3 2 Next replace the sin x term with 1 cos x 3 Now do a substitution with u sin a cos a da using substitution u cos x and du sin x dx 4 With this substitution the integral after multiplying out into a sum of powers of u becom SE u Do not put a negative sign in front of the integral 5 Now antidifferentiate in terms of u and substitute back to get the final result sin x cos x dx C

Calculus
Indefinite IntegrationThe goal of this problem is to evaluate Part 1 The Partial Fraction Setup 82 16 x 8x Perform the partial fraction decomposition of 8x 16 x28x dx using partial fractions 8x16 x 8x and find the values of the coefficients
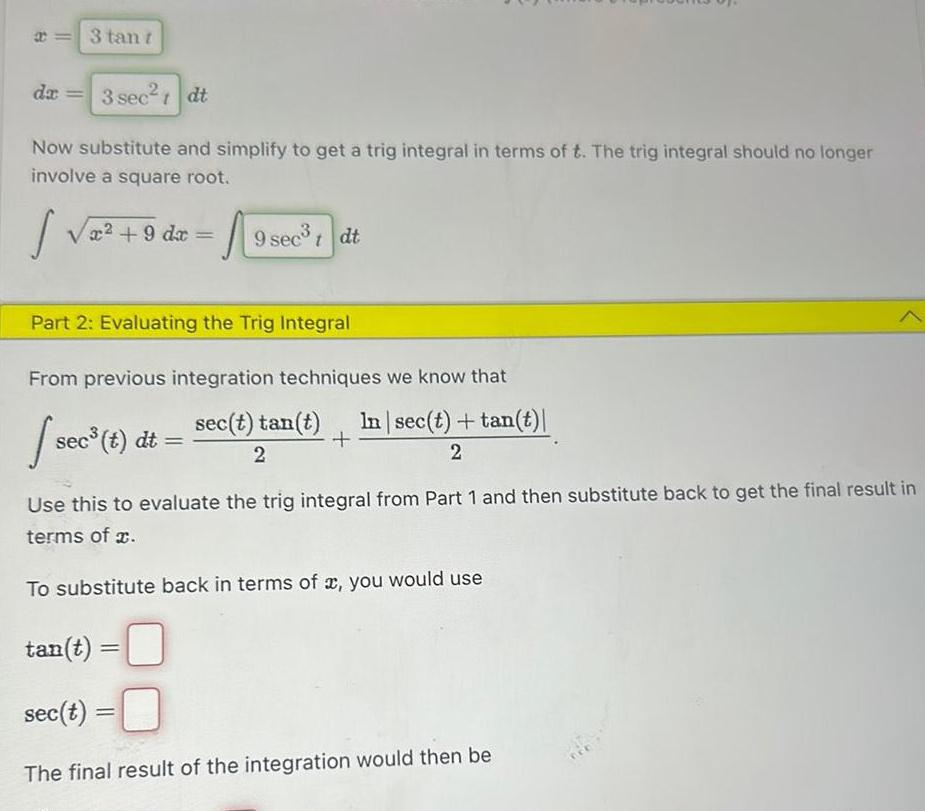
Calculus
Indefinite IntegrationB 3 tan t da 3 sec tdt Now substitute and simplify to get a trig integral in terms of t The trig integral should no longer involve a square root Sv x 9 dx sec Part 2 Evaluating the Trig Integral From previous integration techniques we know that sec t tan t In sec t tan t 2 2 sec t dt tan t 19 sec Use this to evaluate the trig integral from Part 1 and then substitute back to get the final result in terms of x To substitute back in terms of x you would use 9 sec t dt sec t 0 The final result of the integration would then be
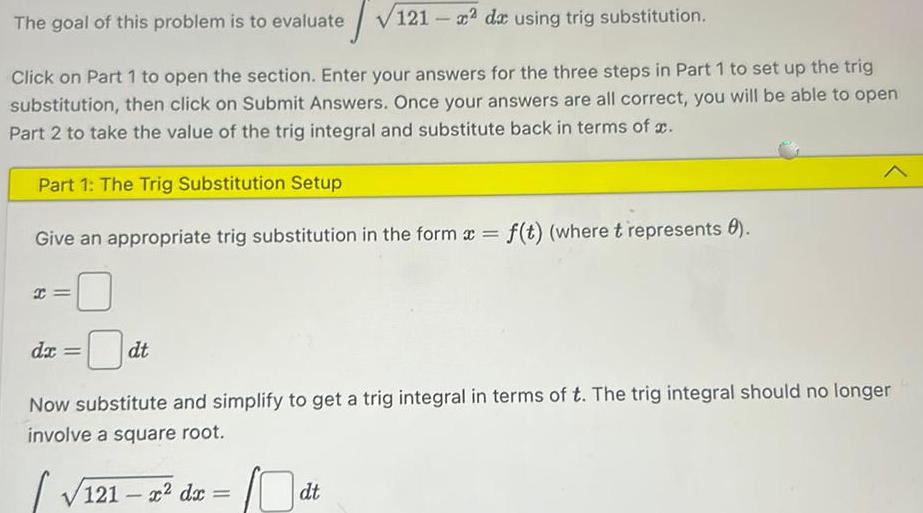
Calculus
Indefinite IntegrationThe goal of this problem is to evaluate 121 2 de using trig substitution Click on Part 1 to open the section Enter your answers for the three steps in Part 1 to set up the trig substitution then click on Submit Answers Once your answers are all correct you will be able to open Part 2 to take the value of the trig integral and substitute back in terms of Part 1 The Trig Substitution Setup Give an appropriate trig substitution in the form x f t where t represents 8 I dx dt Now substitute and simplify to get a trig integral in terms of t The trig integral should no longer involve a square root 121 x dx dt
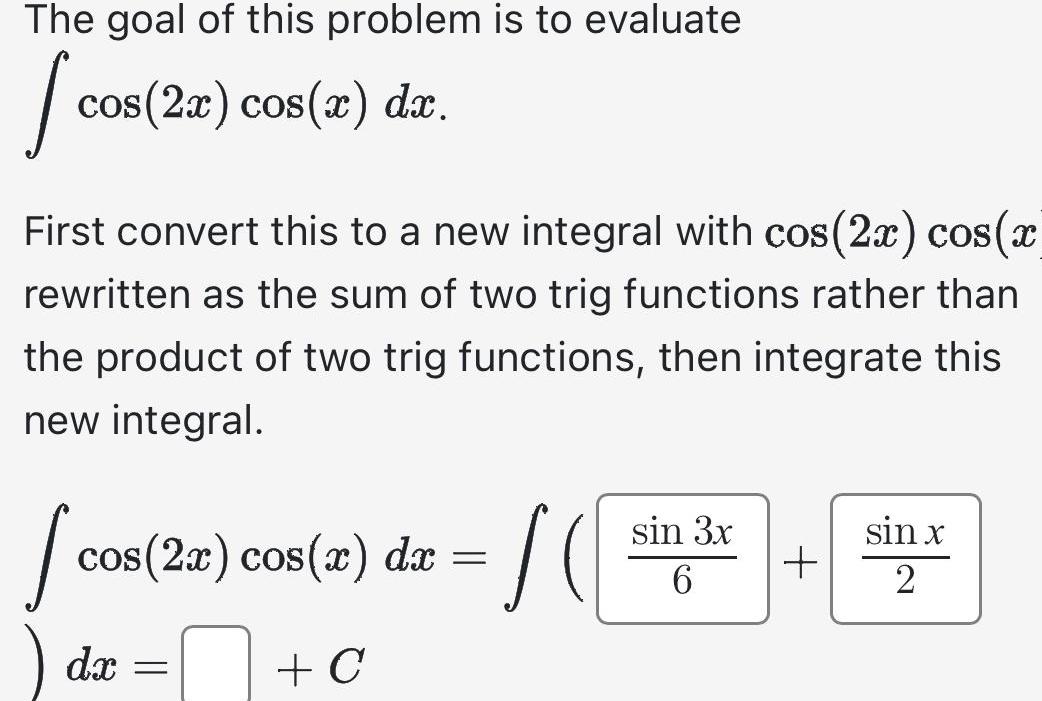
Calculus
Indefinite IntegrationThe goal of this problem is to evaluate J cos 2x cos x dx First convert this to a new integral with cos 2x cos x rewritten as the sum of two trig functions rather than the product of two trig functions then integrate this new integral J cos 2x cos x dx C dx SO sin 3x 6 sin x 2

Calculus
Indefinite IntegrationThe goal of this problem is to evaluate cos 2x cos x dx First convert this to a new integral with cos 2x cos x rewritten as the sum of two trig functions rather than the product of two trig functions then integrate this new integral cos cos 2x cos x dx da C

Calculus
Indefinite IntegrationI Q 1 3 1 Continuity from the Right at a Point A function f X R is said to be right continuous or continuous form the right at a point x EX if the right hand limit of f exists at x and equal to f x i e lim f x f x X 3 2 Continuity from the Left at a Point A function f X R is said to be Left continuous or continuous form the Left at a point x X if the Left hand limit of f exists at x and equal to f x i e lim f x f x X 4 Continuous Function A function f X R is said to be continuous on X if and only if f is continuous at each point of X Example 1 Show that the function f x is not continuous at x 0 Solution Given function is f x since f x is not defined for x 0 therefore it cannot be continuous at x 0 Moreover we know that lim f x lim does not exist in R Hence f x is not continuous at x 0 Value Addition Note If the limit of a function f X R does not exist at a point x X in R then the function f X R cannot be continuous at x

Calculus
Indefinite IntegrationRemark 2 4 2 a Fig 1 4 2 20 d The definition of a graph does not allow more than one line joining two points Also it does not allow any line joining a point to itself Line joining points to itself is called a loop Fig 1 5 is a loop Fig 1 5 V 2 MULTI GRAPH Definition If more than one line joining two vertices are allowed then the resulting object is called a multi graph Lines joining the same points are called multiple lines Fig 1 6 is an example of a multi graph Fig 1 6

Calculus
Indefinite IntegrationThe battery charge of a cell phone is tracked with a function over the course of one day Graph a possible function below that correctly represents the domain and range of the situation Co Battery charge 150 1 25 100 75 50 25 0 25 4 8 12 16 20 24 28 Time hours

Calculus
Indefinite IntegrationThus o satisfies condition of GCD a b Further het diviles c divides b and 8 also b 8 c divides 8 thie continuing C divides ni Since a b 8 process we finally have that This shows that I satisfiel condition of GCD Hexe GCD a b

Calculus
Indefinite IntegrationHonda Motor Company has discovered a problem in the exhaust system of one of its automobile lines and has voluntarily agreed to make the necessary modifications to conform to government safety requirements Standard procedure is for the firm to pay a flat fee to dealers for each modification completed Honda is trying to establish a fair amount of compensation to pay dealers and has decided to choose a number of randomly selected mechanics and observe their performance and learning rate Based on the results of this test Honda decided to pay a 60 fee for each repair 3 hours x 20 per flat rate hour Southwest Honda Inc is considering whether to participate in the program If they can average three hours or less on each repair what Honda is willing to pay them for it will make sense for them to agree They performed their own test repairs to help in their decision process Six mechanics working independently have completed two modifications each On average the mechanics took 10 hours to do the first unit and 8 00 hours to do the second The dealership expects to perform 360 such modifications which works out to 60 modifications per mechanic including the first two units already built Use Exhibit 6 5 On average how many hours will it take Southwest Honda to perform each modification using all six mechanics as planned Round your answer to 1 decimal place Answer is complete but not entirely correct

Calculus
Indefinite IntegrationA crate with mass 32 5 kg initially at rest on a warehouse floor is acted on by a net horizontal force of 140 N How far does the crate travel in 10 0 s

Calculus
Indefinite IntegrationThe graphs of two functions f and g are given below 2 7 3 1 2 f Evaluate each limit if the limit does not exist say why a lim f x g x b lim f x g x c lim f x g x d lim f x g x 3 2 g x x 1 f x h lim e lim f x g x f lim g x g lim f x cos x 4 0 2 14 2 2

Calculus
Indefinite IntegrationQuestion 8 Given f x Domain 1 x 4 V and g x 16 x 3 find the domain of f g x

Calculus
Indefinite IntegrationSubmit Answer DETAILS SCALCET9 2 7 052 8 0 11 Points The quantity in pounds of a gourmet ground coffee that is sold by a coffee company at a price of p dollars per pound is Q f p a What is the meaning of the derivative f 5 What are its units O The rate of change of the quantity of coffee sold with respect to the price per pound when the price is 5 per pound The units are pounds dollars pound O The price of the coffee as a function of the supply The units are pounds O The rate of change of the price per pound with respect to the quantity of coffee sold when the price is 5 per pound The units are dollars pound O The rate of change of the price per pound with respect to the quantity of coffee sold when the price is 5 per pound The units are pounds dollars pound O The rate of change of the quantity of coffee sold with respect to the price per pound when the price is 5 per pound The units are dollars pound b Is f 5 positive or negative Explain O positive O negative

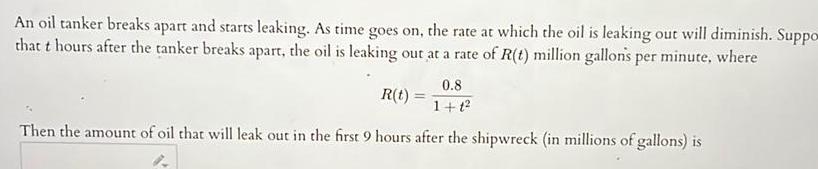
Calculus
Indefinite IntegrationAn oil tanker breaks apart and starts leaking As time goes on the rate at which the oil is leaking out will diminish Suppo that t hours after the tanker breaks apart the oil is leaking out at a rate of R t million gallons per minute where R t 0 8 1 1 Then the amount of oil that will leak out in the first 9 hours after the shipwreck in millions of gallons is

Calculus
Indefinite IntegrationCalculate a Riemann sum S3 3 on the square R 0 3 x 0 3 for the function g x y f x y 6 The contour plot of f x y is shown in Figure 4 Choose sample points and use the plot to find the values of f x y at these points Use the values of f x y to evaluate g x y accordingly S3 3 PP P P21 P31 0 1 3 FIGURE 4 Contour plot of f x y P32 2

Calculus
Indefinite IntegrationCompute the Riemann sum S4 3 to estimate the double integral of f x y 6ry over R 1 3 x 1 2 5 Use the regular partition and upper right vertices of the subrectangles as sample points
Calculus
Indefinite IntegrationFind the minimum and maximum values of the function f x y x y subject to the given constraint a y 32 fmin

Calculus
Indefinite IntegrationThis problem asks you to evaluate U X 2 using integration by parts Fill in the table below with the appropriate terms for setting up the integration by parts du 1 dx sin 10x dx a sin 10 da Crain 1 V Now substitute all of this into the Integration by Parts formula giving fas x cos 10x 10 S Complete the last integration to get 10 6 6 dv cos 10x dx cos 10x 10 2 da

Calculus
Indefinite Integrationx 3 e 8 da using integration by parts Fill in the table below with the appropriate terms for setting up the integration by parts Complete the last integration to get u u 3 du 1 dx dv e 8x dx Now substitute all of this into the Integration by Parts formula giving x 3 e 8 dx x 3 6 V 0 10 dr 3 e 8x da C