Limits & Continuity Questions and Answers

Use the power series 1/1+x = Σ∞ n=0 (-1)ⁿ xⁿ | x | < 1 to find a power series for the function, centered at 0. h(x) = x/x² - 1 = 1/1 + x + 1/1-x h(x) = Σ∞ n=0 no Determine the interval of convergence. (Enter your answer using interval notation.)

Find a) the average rate of change of the function over the interval [3,3.01] and b) the instantaneous rate of change at x = 3. f(x)= x^2 + 2x-3

An object moves along a horizontal path with a velocity v(t) = 2t², where v(t) is measured in meters per second. If the object has an initial position of x(0) = 1 meter, what is the position of the object when its acceleration is 8? 1) 6.333 2) 5.333 3) 4.333 4) -2.333

Use the power series 1/1+x = Σ∞ n= Σ∞ n=10 (-1)ⁿxⁿ , | x | < 1 to find a power series for the function, centered at 0. f(x) = In(x + 1) = ∫1/1+x dx f(x) = Σ∞ n=0 Determine the interval of convergence. (Enter your answer using interval notation.)

Simplify [(2i)²]³/8i Enter your answer in the box.

Students in a classroom earned an ice cream reward for reaching their CCSS goal. 15 students ordered the single scoop cone, 5 ordered the kid scoop, and 3 ordered the single scoop waffle cone. Their teacher ordered the Holiday Hot Fudge Hurricane. What was the total for their order? Show all your work, label

The equation of the ellipse that has a center at (6, 1), a focus at (10,1), and a vertex at(1, 1) is [ (x-c)^2/A^2] + [(y-D)^2/B^2]=1 where a)A= b)B= c)C= D)D=

A circle has a radius of 3 inches. A) What is the diameter? B) What is the area of the circle? C) What is the circumference of the circle? A circle has a diameter of 8 meters. A) What is the radius? B) What is the area of the circle? C) What is the circumference of the circle? What is the perimeter of the figure to the right? What is the area of the figure to the right?

Suppose s(t) = 8t - 2t² on the interval (0,4). What is the distance traveled on [0, 4]? 1) 0 2) 4 3) 8 4)16

What is the median? What is the lower quartile ? What is the Upper quartile ? What is the minimum value? What is the maximum value? The above five values are called the

Find the compositions. f(x) = 6x +9, g(x) = 9 - X (a) f₀ g (b) g₀ g (c) g₀ g

The equation of the ellipse that has a center at (1,3), a focus at (4,3), and a vertex at (6,3), is (x - C)^2/A^2 + (y - D)^2/B^2 =1 where, a)A= b)B= C)C= D)D=

Kelsey takes out a loan for $6000 to start a business after high school. The bank charges her 8% interest for the loan. After 5 years how much interest will be added on to the loan?

Compute the average rate of change of f(x) = x² – 2x + 2 on the interval [1, 5).

Rewrite using sines and cosines: tan(y)csc(y). tan(y)csc(y) =1/cos(y)

Find the following (if possible): 5x/101 + 5x + 2 mod 991 = 5

Find the complex zeros of f(x)=x³ +13x² +57x+85.

Suppose sinθ = 3/5, cosθ= 4/5 sin(2θ)=24/25 cos(2θ) =7/25

The velocity of a particle on the time interval 0 ≤ t ≤ 3 is v(t) = sin(3πt), where v(t) is in cm/s. What is the average velocity for the interval? (1) Vavg = 1/9π (2) Vavg = 2/9π (3) Vavg = 0 (4) Vavg = 1

If f(x) = x³ + 2x, what's the average value of f(x) over the interval[(1, 3]?

If f(x) = 4ˣ , what formula will describe f(x)'s average value over the interval (-1, 3]? Don't evaluate the formula. If f(x) = x³ + 2x, what is the average value of f(x) over the interval (1, 3)?

Form a polynomial whose real zeros are -2, 2, 3 and that has a degree of 3. Use a leading coefficient of 1.

If c = -0.477, simplify the expression cos(cos^(-1)(c)). If undefined, enter Ø. Provide your answer below:

Use the power series 1/1+x = Σ∞ n=0 (-1)ⁿ xⁿ , Ixl < 1 to determine a power series for the function, centered at 0, f(x) = 18/(x+1)³ = d²/dx² (9/x+1] f(x) = Σ∞ n=0 (-1)ⁿ xⁿ . (n+2)!/n! Determine the interval of convergence. (Enter your answer using interval notation.) (-1,1)

Find an exponential regression curve for the data set An exponential regression curve for the data set is y= ___ . e (Type Integers or decimals rounded to three decimal places as needed.)

Find a power series for the function, centered at c, and determine the interval of convergence. f(x) = 3/(3x + 2), c = 6

Consider the ellipse with equation (x + 9)²/3² + (y - 3)²/2² = 1 The semimajor axis has length The semiminor axis has length The vertices are located at (-6,3),(-12,3)

What is the average value of f(x), between x = 0 and x = 3 for f(x) = 2x/(x² + 3) ?

The fourth graders at Long Elementary will visit the Zoo on Friday .There are 215 students and 12 adults attending . If a school bus will seat 72 passengers , how many busses will be needed for the trip ?

Convert the rectangular coordinates (5, -5√3) to polar form. Let r >0 and 0<θ < 2π. Enter your answer by filling in the boxes. Enter coordinates as simplified fractions or radicals in simplest form.

The initial point of ã is (-5,1) and its terminal point is (-1, -3). What is the value of ll a ll? Enter your answer, as a simplified radical, in the box.

An oblique cone has a radius of 7 units and a volume of (784π / 3) cubic units. What is the height of the cone?

tan α =- 8/15, α lies in quadrant II , and cos β = 5/6, β lies in quadrant I. (a) sin(α + β) (b) cos(α + β) (c) tan(α + β)

7900 dollars is placed in an account with an annual interest rate of 5.5%. How much will be in the account after 11 years, to the nearest cent?

Convert r =5/(3 sinθ - cosθ) to rectangular form. Enter your answer in slope-intercept form by filling in the boxes. Enter values so that fractions are simplified. y =

Determine and classify the discontinuities, if any, of f. f(x) = -6 if x≠ 2 0 if x= 2 State the removable discontinuities, if any, of f. If multiple removable discontinuities exist, enter the solutions using a comma-separated list. (a) f has a removable discontinuity at x = (b) f has no removable discontinuities. State the non-removable discontinuities, if any, of f. If multiple nonremovable discontinuities exist, enter the solutions using a comma-separated list. (a) f has a non-removable discontinuity at x = (b) f has no nonremovable discontinuities.

Determine and classify the discontinuities, if any, of 8. s(x) = 5x² - 9² +1 State the removable discontinuities, if any, of s. If multiple removable discontinuities exist, enter the solutions using a comma-separated list. (a) s has a removable discontinuity at x = __ s has no removable discontinuities. State the non-removable discontinuities, if any, of s. If multiple non removable discontinuities exist, enter the solutions using a comma-separated list. (a) s has a non-removable discontinuity at x = __ s has no non removable discontinuities.

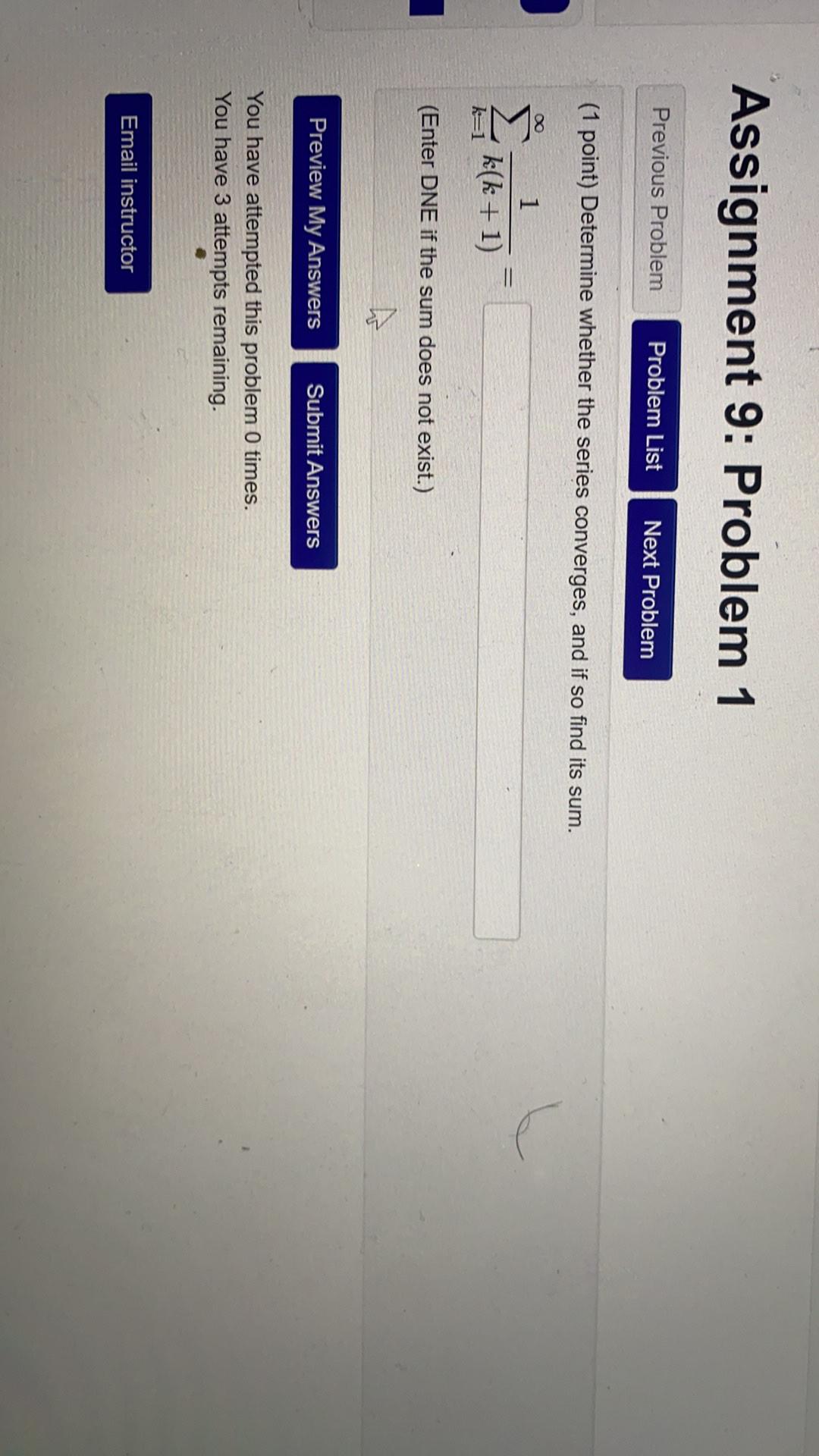
Determine whether the series converges, and if so find its sum. Σ∞ k=1 1/k(k+1) = ________

Use the table to find lim 3x². x-> -3 X - 3.01 -3.001 -3.0001 ⟶ ⟵ -2.9999 -2.999 -2.99 f(x) = 3x^2 27.1803 27.0180 27.0018 ⟶ ⟵ 26.9982 26.9820 26.8203 lim 3x² = x-> -3

Determine whether the series is convergent or divergent. If convergent, find the sum; if divergent, enter div. Σ∞ n=0 n/√n²+18

Question 9 Solve for θ if -8 sinθ + 6 = 4√2 + 6 and 0 ≤θ< 2π. θ= π only θ= π/4 and θ=3π/4 θ= 5π/4 and θ=7π/4 θ= 5π/9 only θ= 5π/3 and θ=5π/6

In{(x + 1)/(x^3 + 1)} Find the Domain and Range. f(x) = cos^-1(In(tanx)). Find the Domain

Divide: (3 - 4i)/(7 - i) Write your answer in a + bi form

A tower that is 109 feet tall casts a shadow 110 feet long. Find the angle of elevation of the sun to the nearest degree. The angle of elevation is ______degrees. (Round to the nearest degree)

R is the region bounded by the functions f(x) = (x/2) - 3 and g(x) = -(x/2) - 9 over the interval [a, b] where a = -5 and b = -1. Represent the area of R by writing an integral with respect to x. You do not need to simplify. Provide your answer below:

Determine and classify the discontinuities, if any, of p. p(x) = 9x / (10x - x²) State the removable discontinuities, if any, of p. If multiple removable discontinuities exist, enter the solutions using a comma-separated list. (a) p has a removable discontinuity at x = __ p has no removable discontinuities. State the non-removable discontinuities, if any, of p. If multiple non removable discontinuities exist, enter the solutions using a comma-separated list. (a) p has a non-removable discontinuity at x = __ p has no non removable discontinuities.

In a circle of diameter 10, find the area of a sector whose central angle is 45° and round off to the nearest hundredths. A. 562.50 B. 9.82 C. 39.27 D. NO correct choices

Determine and classify the discontinuities, if any, of k. k(x) = 7x / (x² + 256) State the removable discontinuities, if any, of k. If multiple removable discontinuities exist, enter the solutions using a comma-separated list. (a) k has a removable discontinuity at x = __ k has no removable discontinuities. State the non-removable discontinuities, if any, of k. If multiple non removable discontinuities exist, Enter the solutions using a comma-separated list. (a) k has a non-removable discontinuity at x = __ k has no non removable discontinuities.

Determine the intervals on which f is continuous. Enter the solution using interval notation. f(x) = 1 - 8 ln( - 5 - 6x) f is continuous on _______

Consider the function f(x) = 2 - 8x^2on the interval [- 5,8]. Find the average or mean slope of the function on this interval, i.e. (f(8) - f(-5))/(8 - (-5))=___________ By the Mean Value Theorem, we know there exists a c in the open interval (-5,8) such that f'(c) equal to this mean slope. For this problem, there is only one c that works. Find it. ____________