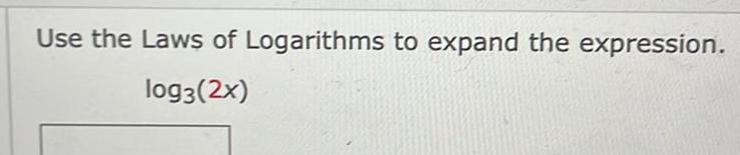
Application of derivatives Questions and Answers

Calculus
Application of derivativesTwo cars are moving towards an intersection One car is 170 meters north of the intersection and moving towards the intersection at 11 m s while the other is 200 meters west of the intersection and moving towards the intersection at 10 m s How rapidly are the cars approaching each other 5 seconds later The cars are getting Select an answer 3 decimal places at m s Enter your answer rounded to

Calculus
Application of derivativesIn 2005 the world population was 6 4 billion The exponential growth rate was 1 2 per year Fin the exponential growth function
Calculus
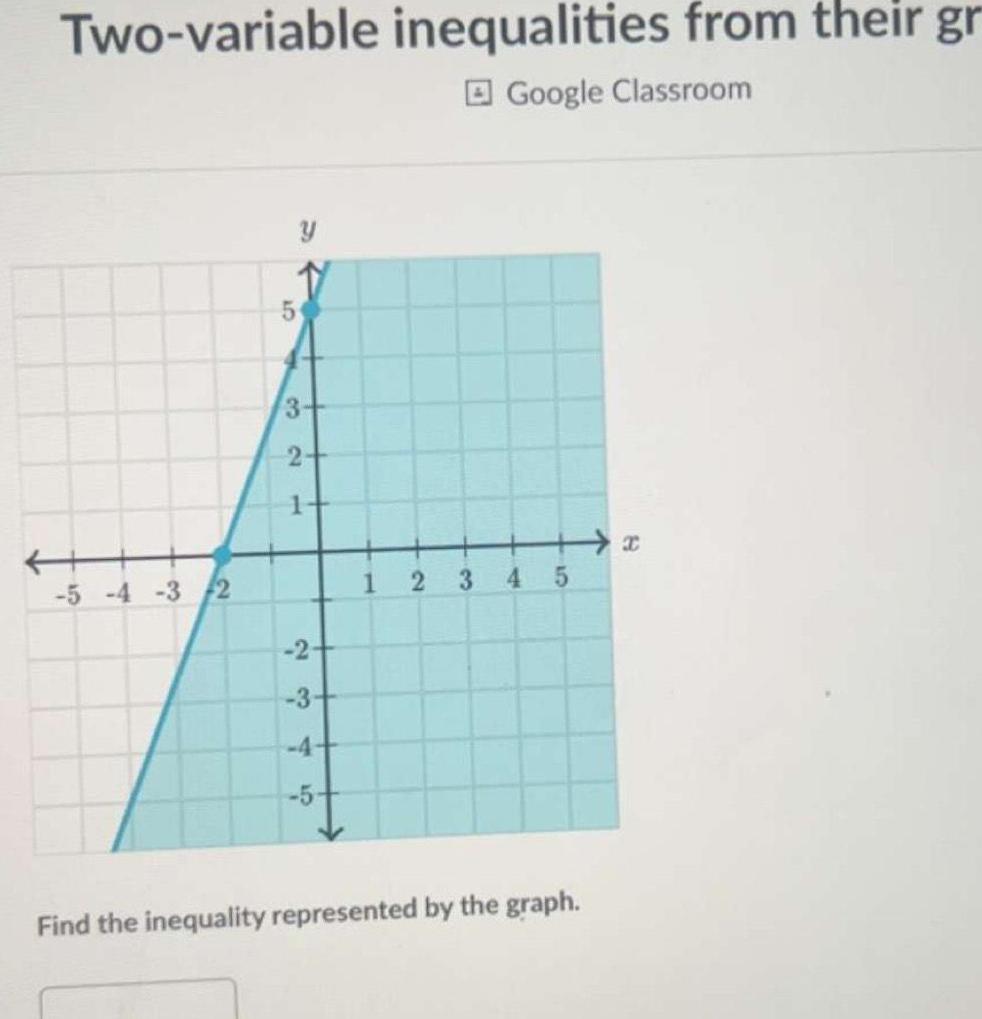
Application of derivativesTwo variable inequalities from their gr Google Classroom 5 4 3 2 5 Y 3 2 L 2 3 4 cr 1 2 3 4 5 Find the inequality represented by the graph

Calculus
Application of derivativesThe population of a small town in central Florida has shown a linear decline in the years 1994 2004 In 1994 the population was 45200 people In 2004 it was 36300 people A Write a linear equation expressing the population of the town P as a function of t the number of years since 1994 Answer B If the town is still experiencing a linear decline what will the population be in 2009
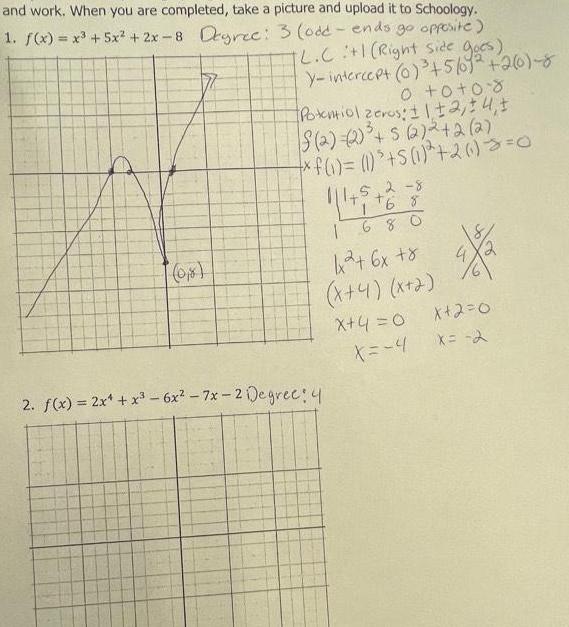
Calculus
Application of derivativesand work When you are completed take a picture and upload it to Schoology 1 f x x 5x 2x 8 Degree 3 odd ends go opposite L C 1 Right Side Goes y intercept 0 5 092 260 8 0 0 08 Potentiol zeros 1 2 4 2 2 5 2 2 2 2 f 1 1 5 1 26 0 111 5 2 8 0 8 8 1 680 1x 6x 8 x 4 x 2 X 4 0 X 4 2 f x 2x x 6x 7x 2 Degree 4 X 2 0 x 2

Calculus
Application of derivativesQuilt squares are cut on the diagonal to form triangular quilt pieces The hypotenuse of the resulting triangles is 36 cm long What is the side length of each piece Round your answer to he nearest hundredth

Calculus
Application of derivativesFind the equation in factored form and sketch the graph of a polynomial function with an inflection point at 0 1 and is tangent to the x axis at 2 The point 0 12 is on the function
Calculus
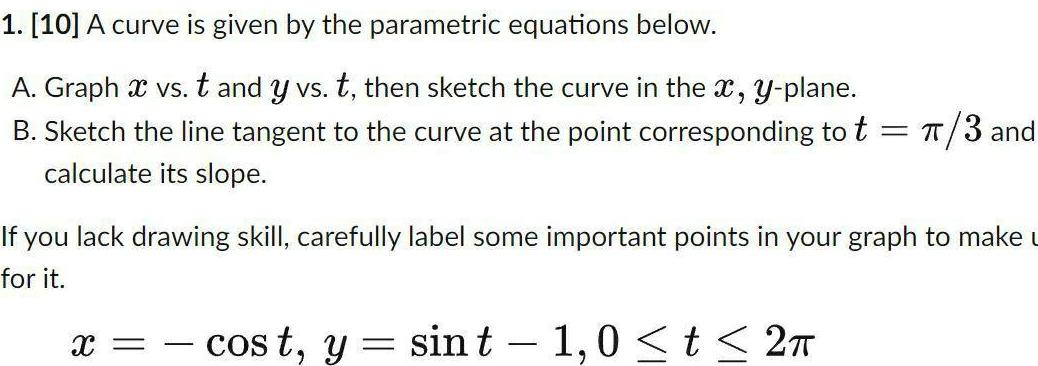
Application of derivatives1 10 A curve is given by the parametric equations below A Graph x vs t and y vs t then sketch the curve in the x y plane B Sketch the line tangent to the curve at the point corresponding to t 3 and calculate its slope If you lack drawing skill carefully label some important points in your graph to make u for it X cost y sin t 1 0 t 2
Calculus
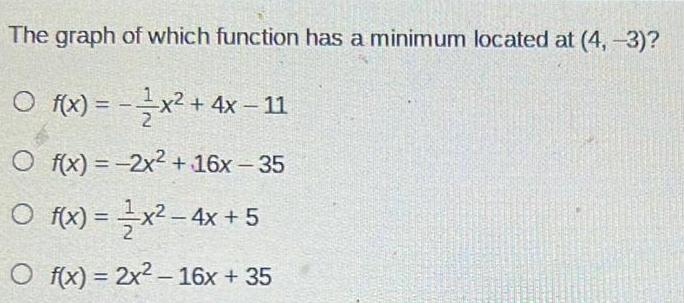
Application of derivativesThe graph of which function has a minimum located at 4 3 f x x 4x 11 Of x 2x 16x 35 Of x x 4x 5 O f x 2x 16x 35
Calculus
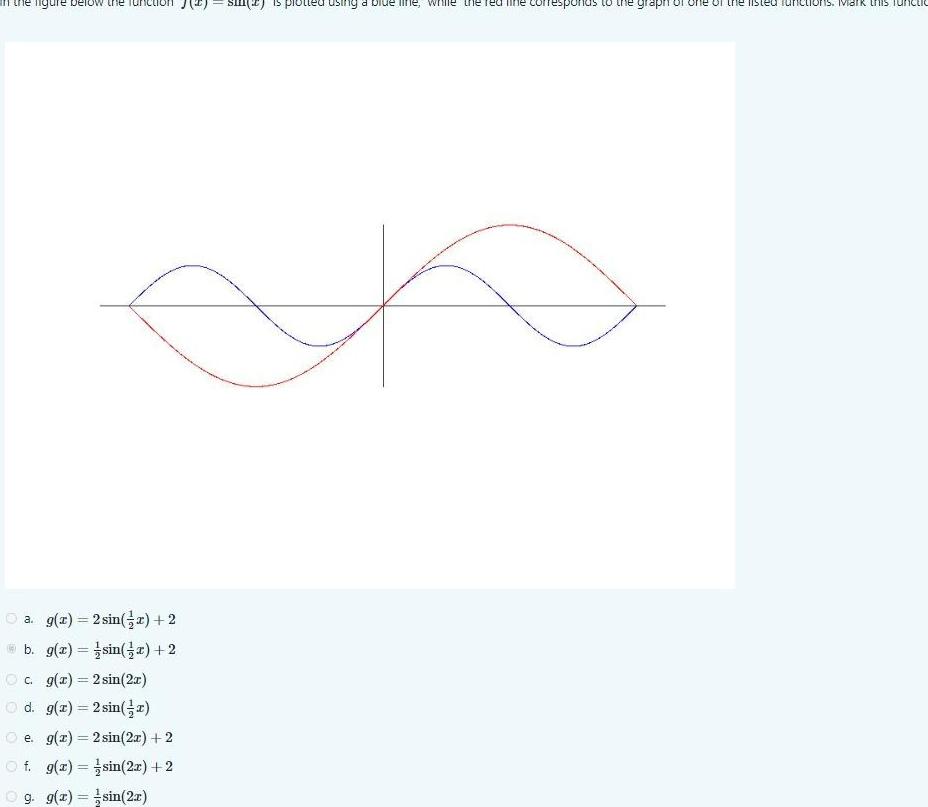
Application of derivatives160 B gure below the a g x 2 sin x 2 b g x sin x 2 c g x 2 sin 2x d g x 2 sin x e g x 2 sin 2x 2 f g x sin 2x 2 g g x sin 2x plotted using a blue line while the red corresponds to graph the listed Mark this Tu

Calculus
Application of derivatives13 Use an Addition or Subtraction Formula to write the expression as a COMBINED trigonometric function of one number and then find its exact value Round to 2 decimal places if needed LOS 3T 27 21 7 3 sin sin 7 COS 27 21 tan tan 18 1 tan kla kla TT tan 18
Calculus
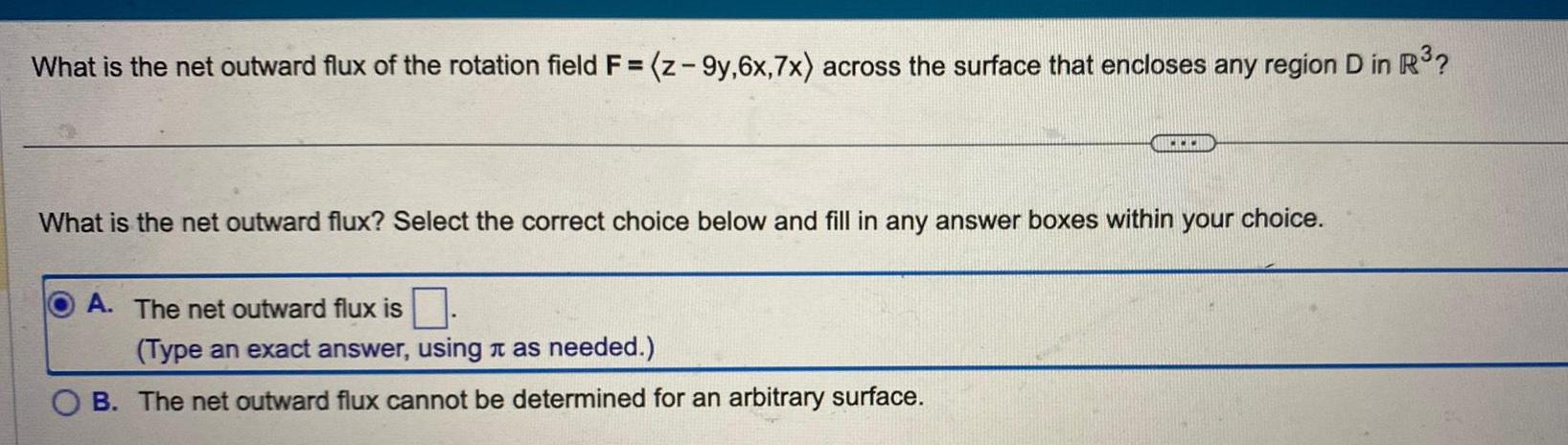
Application of derivativesWhat is the net outward flux of the rotation field F z 9y 6x 7x across the surface that encloses any region D in R www What is the net outward flux Select the correct choice below and fill in any answer boxes within your choice A The net outward flux is Type an exact answer using as needed OB The net outward flux cannot be determined for an arbitrary surface

Calculus
Application of derivativesUse Stokes Theorem to find the circulation of the following vector field around any simple closed smooth curve C F y z 2xyz 3xy z The circulation is
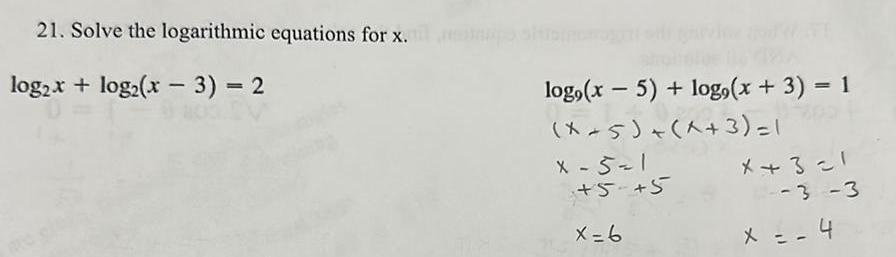
Calculus
Application of derivatives21 Solve the logarithmic equations for x apo shtronomo log x log x 3 2 log x 5 logo x 3 1 X 5 x 3 1 X 5 1 5 5 x 6 x 3 1 3 3 X 4

Calculus
Application of derivativesSimplify 82 1 212 A x 82 1 OC 2x x 18 O E 22 8 24 13 OB 8x x4 D 2x z 1
Calculus
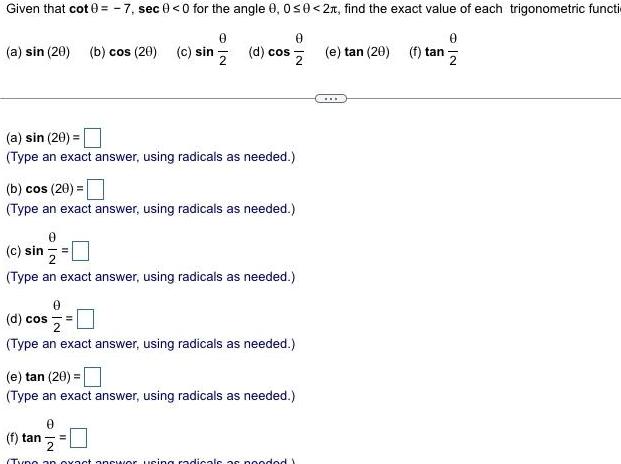
Application of derivativesGiven that cot 0 7 sec 0 0 for the angle 0 0 0 2 find the exact value of each trigonometric functi 0 a sin 20 b cos 20 c sin d cos 2 a sin 20 Type an exact answer using radicals as needed 0 b cos 20 Type an exact answer using radicals as needed c sin Type an exact answer using radicals as needed 0 d cos Type an exact answer using radicals as needed e tan 20 Type an exact answer using radicals as needed f tan 0 2 Tyno an exact answer using radicals as pooded Y 0 e tan 20 f tan 2
Calculus
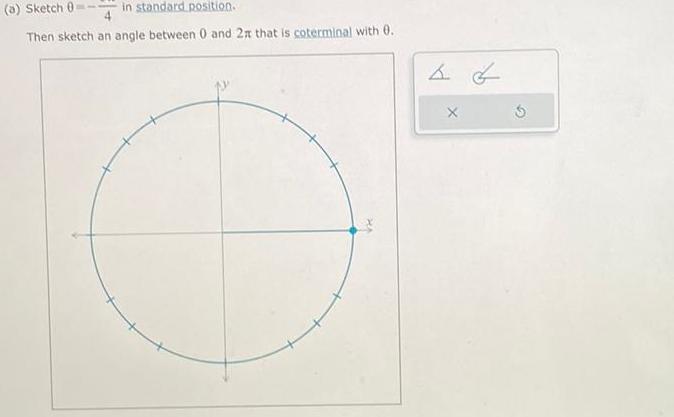
Application of derivativesa Sketch 0 in standard position Then sketch an angle between 0 and 2x that is coterminal with 0 L
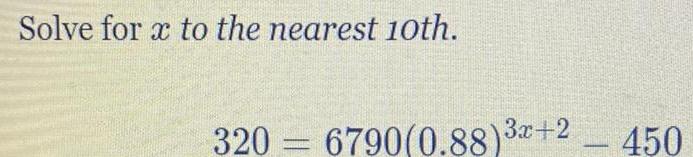
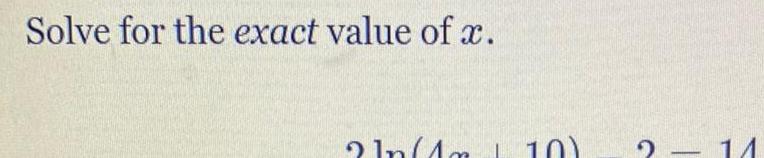
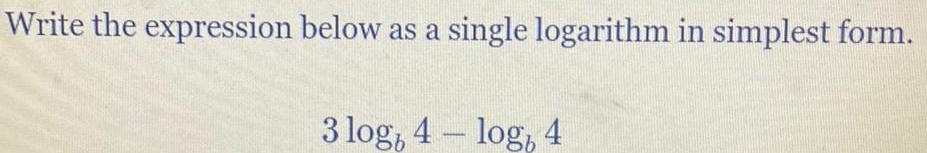
Calculus
Application of derivativesWrite the expression below as a single logarithm in simplest form 3 log 4 log 4
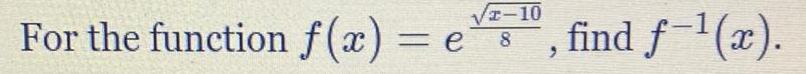

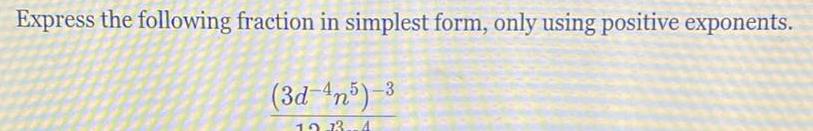
Calculus
Application of derivativesExpress the following fraction in simplest form only using positive exponents 3d 4n5 3 19 33 4

Calculus
Application of derivativesExpand the binomial by using Pascal s Triangle to determine the coefficients 2v 3 6

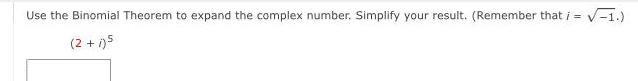
Calculus
Application of derivativesUse the Binomial Theorem to expand the complex number Simplify your result Remember that i 1 2 1 5
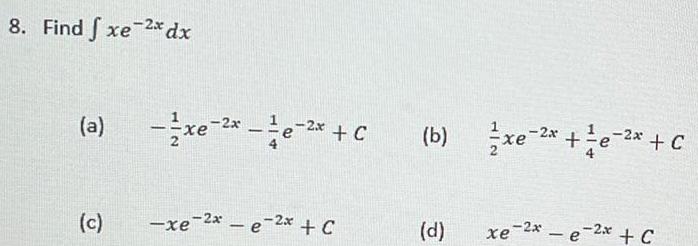
Calculus
Application of derivatives8 Find fxe 2 dx a c x x e x C xe 2x e 2x C b d xe 2x e 2x C xe 2 2x C
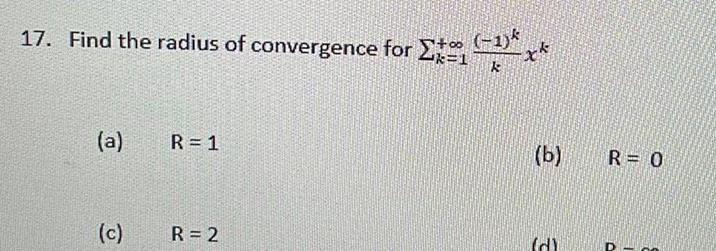
Calculus
Application of derivatives17 Find the radius of convergence for 1 k 1 xk k a c R 1 R 2 b 3 R 0

Calculus
Application of derivatives12 Find the slope of the tangent line to the curve x t 1 y 2t t 1 at t 1 a c 5 1 b d 4 3
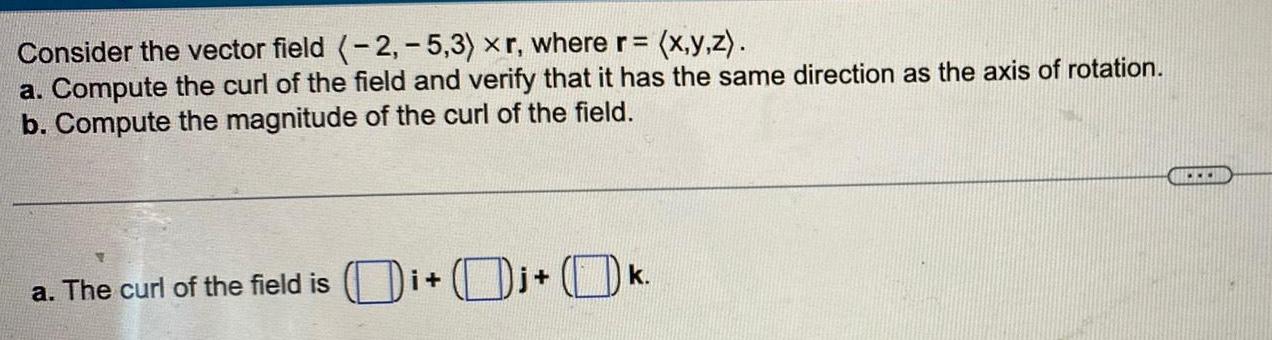
Calculus
Application of derivativesConsider the vector field 2 5 3 xr where r x y z a Compute the curl of the field and verify that it has the same direction as the axis of rotation b Compute the magnitude of the curl of the field a The curl of the field is i k

Calculus
Application of derivatives3 3 points Find an equation of the tangent line to the graph of the function at the given point y ln 2 1 227 1 2 2 1
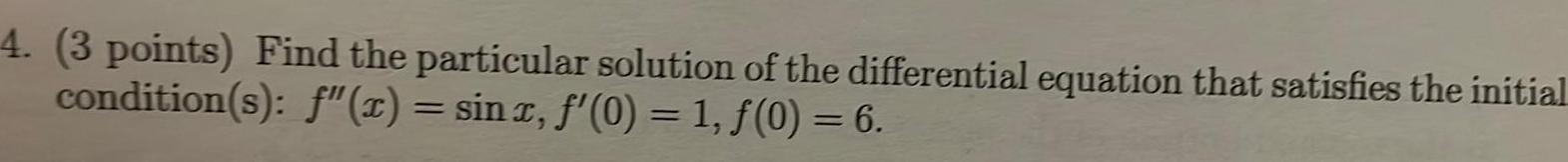
Calculus
Application of derivatives4 3 points Find the particular solution of the differential equation that satisfies the initial condition s f x sinx f 0 1 f 0 6
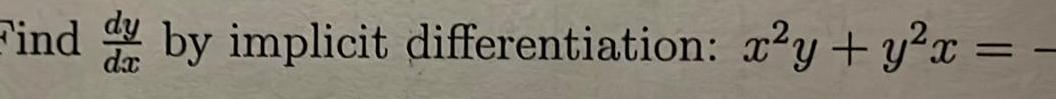


Calculus
Application of derivatives49 If 3 identical prizes are available for a contest with 50 entrants in how many ways can the prizes be distributed What is the answer A 3 260 OB 117 600 C 9 800 D 19 600


Calculus
Application of derivatives32 For the geometric sequence with a 5 and ag 405 find the common ratio OA B 119 113 C 9 D 3

Calculus
Application of derivatives12 Find the roots of this function f x x 2x 2 A 1 2 and 1 2 OB 1 i and 1 i OC 1 2 2 and 1 2 2 OD 1 2i and 1 2i

Calculus
Application of derivativesFind the lengths of the circular arc Assume r 9 and 0 132 6 6T X S S 0
Calculus
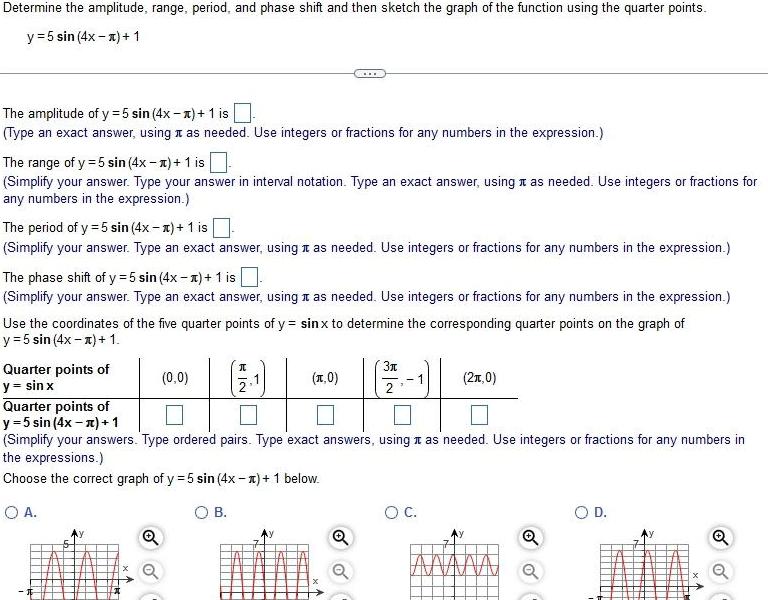
Application of derivativesDetermine the amplitude range period and phase shift and then sketch the graph of the function using the quarter points y 5 sin 4x x 1 The amplitude of y 5 sin 4x x 1 is Type an exact answer using as needed Use integers or fractions for any numbers in the expression The range of y 5 sin 4x 1 1 is Simplify your answer Type your answer in interval notation Type an exact answer using it as needed Use integers or fractions for any numbers in the expression The period of y 5 sin 4x x 1 is Simplify your answer Type an exact answer using as needed Use integers or fractions for any numbers in the expression The phase shift of y 5 sin 4x 1 is Simplify your answer Type an exact answer using as needed Use integers or fractions for any numbers in the expression Use the coordinates of the five quarter points of y sinx to determine the corresponding quarter points on the graph of y 5 sin 4x x 1 Quarter points of y sin x 0 0 I 2 B x 0 3x Quarter points of y 5 sin 4x x 1 Simplify your answers Type ordered pairs Type exact answers using as needed Use integers or fractions for any numbers in the expressions Choose the correct graph of y 5 sin 4x 1 below O A 2 0 O C O D

Calculus
Application of derivativesConsider the following region R and the vector field F a Compute the two dimensional divergence of the vector field b Evaluate both integrals in Green s Theorem and check for consistency F 2y 5x R is the triangle with vertices 0 0 4 0 and 0 4 a The two dimensional divergence is 0 b Set up the integral over the region SS 0 0 Type exact answers dy dx
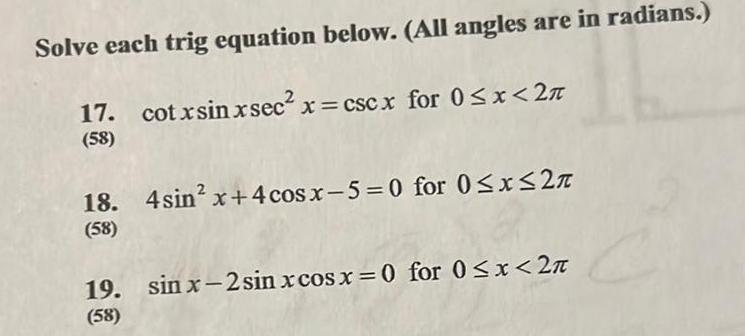
Calculus
Application of derivativesSolve each trig equation below All angles are in radians 17 cotxsinxsec x cscx for 0 x 2 58 18 4sin2x 4cosx 5 0 for 0 x 27 58 19 sin x 2sinxcosx 0 for 0 x 2 58
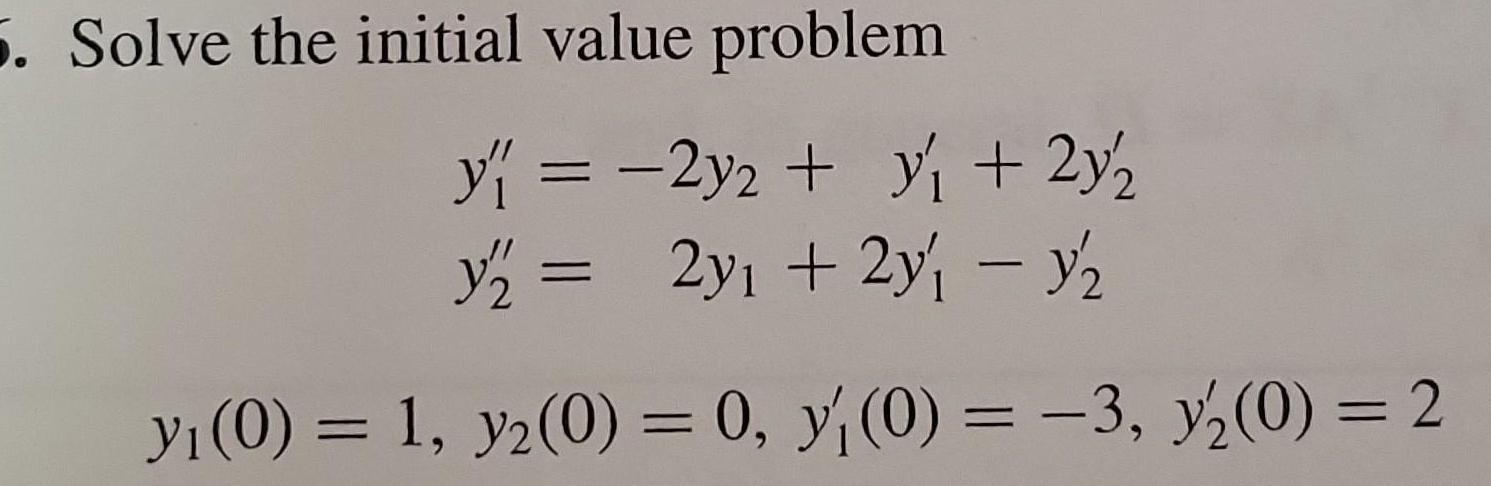
Calculus
Application of derivatives5 Solve the initial value problem y 2y y 2y y 2y 2y y y 0 1 y 0 0 y 0 3 y 0 2
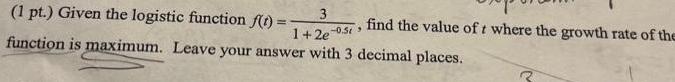
Calculus
Application of derivatives1 pt Given the logistic function f t 3 function is maximum Leave your answer with 3 decimal places 1 2e 05 find the value of t where the growth rate of the
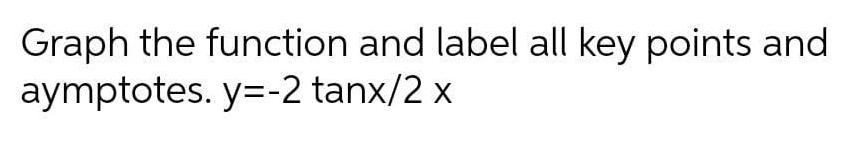
Calculus
Application of derivativesGraph the function and label all key points and aymptotes y 2 tanx 2 x
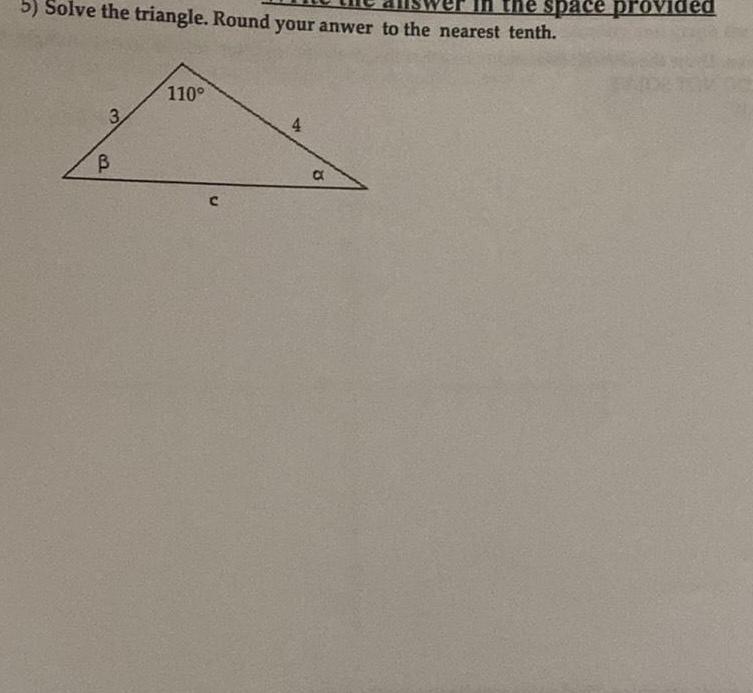
Calculus
Application of derivatives5 Solve the triangle Round your anwer to the nearest tenth wer in the space provided 3 B 110 C 4 a

Calculus
Application of derivativesLet s solve the exponential equation 4e 80 a First we isolate e to get the equivalent equation b Next we take in of each side to get the equivalent equation c Now we use a calculator to find x Round your answer to three decimal pla

Calculus
Application of derivativesa Most calculators can find logarithms with base following Round your answers to three decimal places logs 16 log log and base e To find logarithms with different bases we use the Change of Base Formula b Do we get the same answer if we perform the calculation in part a using In in place of log O Yes the result is the same No the result is not the same 8 To find logs 16 we wn

Calculus
Application of derivativesThe logarithm of a product of two numbers is the same as the sum of the logarithms of these numbers So log2 4 8 log2 4