Application of derivatives Questions and Answers
Calculus
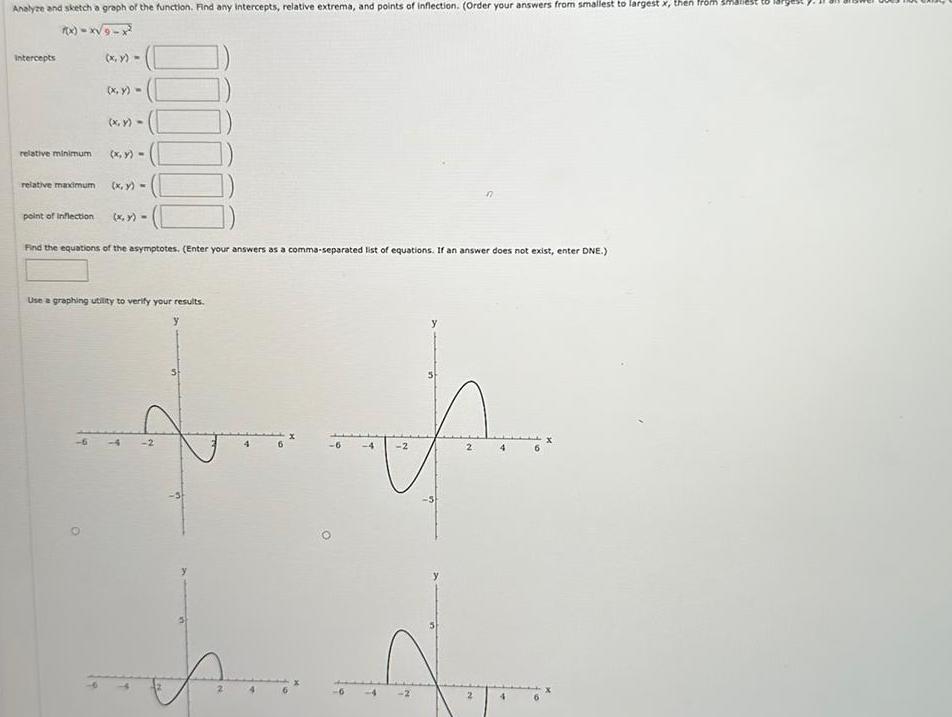
Application of derivativesAnalyze and sketch a graph of the function Find any intercepts relative extrema and points of inflection Order your answers from smallest to largest x then from smallest to 7x x 9 x Intercepts relative minimum relative maximum point of Inflection x y x y x y x y Find the equations of the asymptotes Enter your answers as a comma separated list of equations If an answer does not exist enter DNE Use a graphing utility to verify your results sults 5 t 3 N 2 4
Calculus
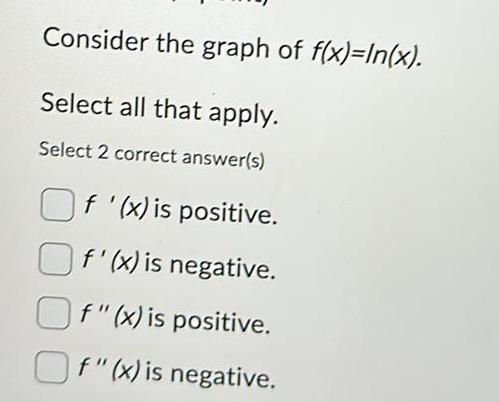
Application of derivativesConsider the graph of f x In x Select all that apply Select 2 correct answer s Of x is positive f x is negative Of x is positive Of x is negative

Calculus
Application of derivativesFind the nth Maclaurin polynomial for the function 4x 3 f x x 1 n 4 P4 x 3 7x 7x 7x 7x

Calculus
Application of derivativesFind the nth Taylor polynomial for the function centered at c f x In x n 4 c 3 1 P4 x ln 3 x 3 8 x 3 x 3 324 x 3 4 18

Calculus
Application of derivativesA parent function is given by y 2 The following transformations took place Vertical shift 3 units upwards Horizontal shift 4 units to the right Reflection about the y axis Vertical compression by a factor of 1 3 Give the equation of the transformed function Indicate where each transformation occurs in your equation Sketch a graph of the transformed function and include a table of values State the domain and range of the transformed function C 8
Calculus
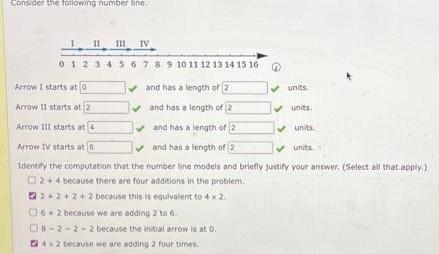
Application of derivativesConsider the following number line III 0 1 2 3 4 5 Arrow I starts at 0 Arrow II starts at 2 Arrow III starts at IV 6 7 8 9 10 11 12 13 14 15 16 and has a length of 2 and has a length of 2 and has a length of 2 units Arrow IV starts at and has a length of 2 units Identify the computation that the number line models and briefly justify your answer Select all that apply 02 4 because there are four additions in the problem 2 2 2 2 because this is equivalent to 4 x 2 6 2 because we are adding 2 to 6 D8 2 2 2 because the initial arrow is at 0 142 because we are adding 2 four times units units
Calculus
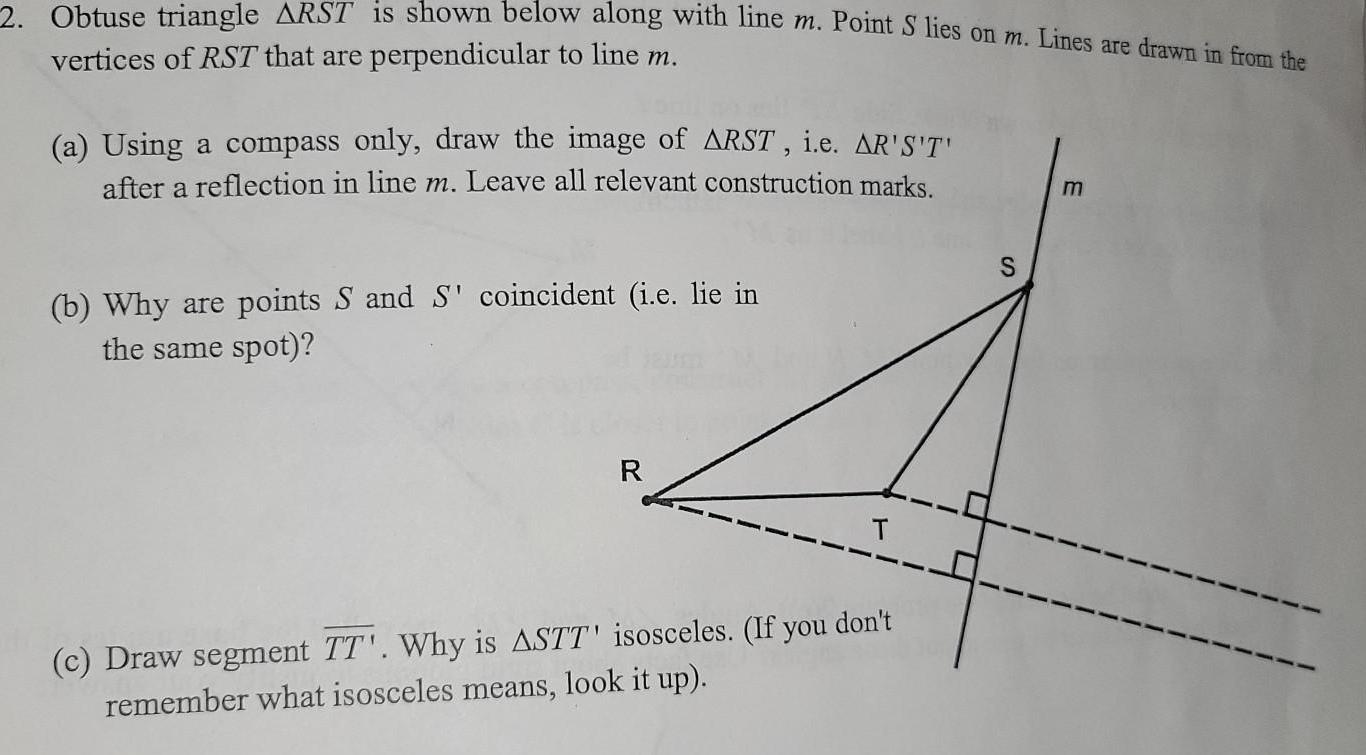
Application of derivatives2 Obtuse triangle ARST is shown below along with line m Point S lies on m Lines are drawn in from the vertices of RST that are perpendicular to line m a Using a compass only draw the image of ARST i e AR S T after a reflection in line m Leave all relevant construction marks b Why are points S and S coincident i e lie in the same spot R T c Draw segment TT Why is ASTT isosceles If you don t remember what isosceles means look it up S m

Calculus
Application of derivatives1 How many significant figures are there in following number 3 006 Answer 2 How many significant figures are there in following number 0 009 Answer 3 Round the following number to three significant figures 0 08707 Answer 4 Round the following number to three significant figures 12 18 Answer 5 Round the following number to three significant figures 252 874
Calculus
Application of derivativesConsider the equation y 1 2 cos 4x Give all answer in exact form NO DECIMALS Represent phase shift and vertical shift with a signed number to show direction and distance n teft n right 0 What is the amplitude 1 What is the period length 2 How far apart are the critical points 3 What is the phase shift 4 What is the vertical shift 5 What is the equation for for midline

Calculus
Application of derivativesa x y a u v a Solve the system u 2x 3y v x 5y for x and y in terms of u and v Then find the value of the Jacobian b Find the image under the transformation u 2x 3y v x 5y of the triangular region in the xy plane bounded by the x axis the y axis and the line x y 2 Sketch the transformed image in the uv pla a x y

Calculus
Application of derivativesA 23 ft ladder leans against a building so that the angle between the ground and the ladder is 75 How high does the ladder reach on the building ft Report the exact answer or use the col

Calculus
Application of derivativesSolve the following trigonometric equations without using a calculator Find all solutions where 0 x 2 If no solution exists type DNE 2 cos x 1 0 Solution Select an answer 18 Answer in RADIANS

Calculus
Application of derivativesA 9 foot ladder is placed against a building If the base of the ladder is 4 feet away from the building how far up the building will the ladder reach Round the answer to the nearest tenth X

Calculus
Application of derivativesFind the length of an arc if the radius of the arc is 1 7 cm and the measure of the arc is 1 6 radians cm round answer to three decimal places
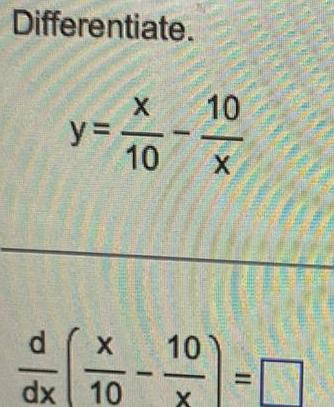

Calculus
Application of derivativesFind the linearization L x y of the function f x y e cos y at the points 0 0 and 0 0 7 The linearization at 0 0 is L x y Type an exact answer using as needed COO

Calculus
Application of derivativesFind the directions in which the function increases and decreases most rapidly at Po Then find the derivatives of the function in these directions f x y z x y yz Po 1 1 4 5 27 j The direction in which the given function f x y z x y yz increases most rapidly at Po 1 1 4 is u 27 Type exact answers using radicals as needed The direction in which the given function f x y z x y yz decreases most rapidly at Po 1 1 4 is v k Type exact answers using radicals as needed 27

Calculus
Application of derivativesTwo coins are tossed Assume that each event is equally likely to occur a Use the counting principle to determine the number of sample points in the sample space b Construct a tree diagram and list the sample space c Determine the probability that no tails are tossed d Determine the probability that exactly one tail is tossed e Determine the probability that two tails are tossed 1 Determine the probability that at least one tail is tossed a There is are sample point s in the sample space b Construct a tree diagram Choose the correct answer below OA OB What is the sample space Choose the correct answer below OA H T HH HT TH TT OB H T H T OC H T D HH HT TH TT c The probability that no tails are tossed is Simplify your answer d The probability that exactly one tail is tossed is Simplify your answer Simplify your answer e The probability that two tails are tossed is 1 The probability that at least one tail is tossed is Simplify your answer GECER OC OD IK

Calculus
Application of derivativesSelect all expressions that are equivalent to 2 10 A 2 9 2 B 2 11 2 C 10 2 D 10 2 E 2 5 2
Calculus
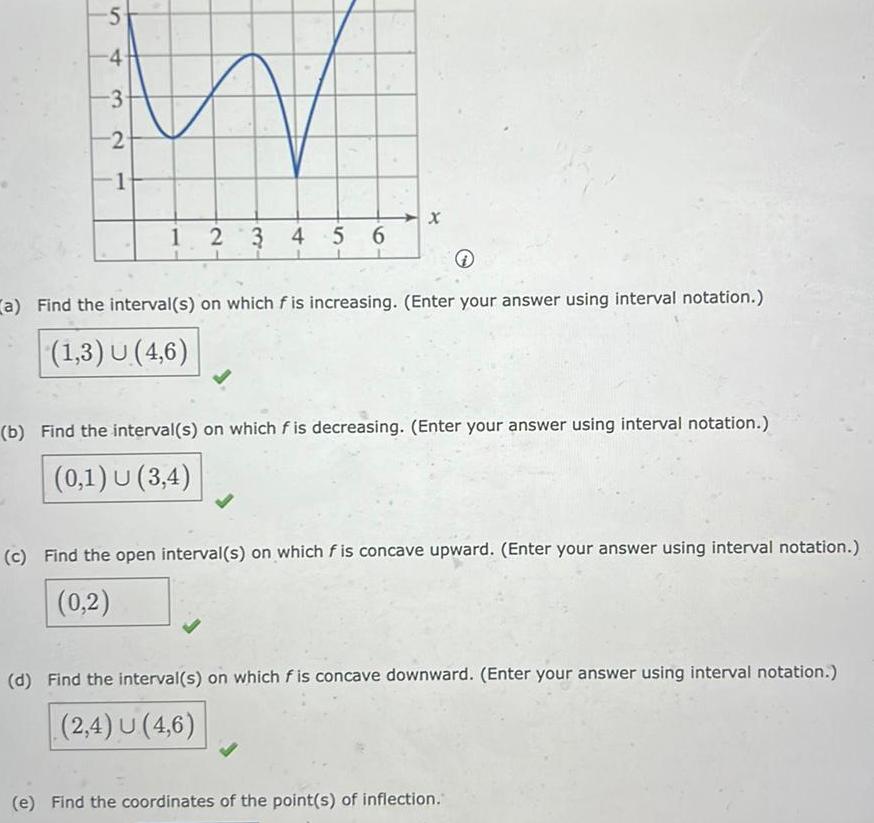
Application of derivatives5 4 3 2 1 1 2 3 4 5 6 a Find the interval s on which f is increasing Enter your answer using interval notation 1 3 U 4 6 b Find the interval s on which f is decreasing Enter your answer using interval notation 0 1 U 3 4 c Find the open interval s on which f is concave upward Enter your answer using interval notation 0 2 d Find the interval s on which fis concave downward Enter your answer using interval notation 2 4 U 4 6 e Find the coordinates of the point s of inflection

Calculus
Application of derivativesAfter applying L Hospital s Rule once we have lim 8 sec x 1 x0 x sec x tan x needed and we will do so here applying the rule for a second time To do so we need to find additional derivatives The derivative of 8 sec x 1 with respect to x is The derivative of x sec x tan x with respect to x is So we still have an indeterminate limit of type o lo We recall that L Hospital s Rule can be applied repeatedly as
Calculus
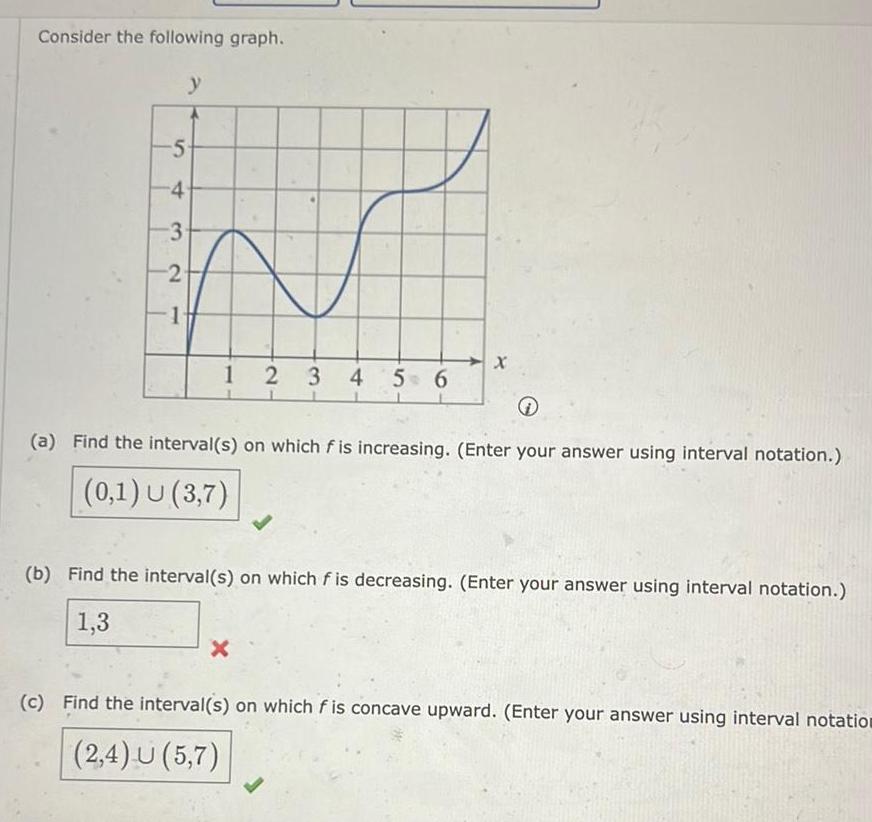
Application of derivativesConsider the following graph 5 4 3 2 y 1 1 2 3 4 5 6 X a Find the interval s on which f is increasing Enter your answer using interval notation 0 1 U 3 7 b Find the interval s on which f is decreasing Enter your answer using interval notation 1 3 X c Find the interval s on which f is concave upward Enter your answer using interval notation 2 4 U 5 7

Calculus
Application of derivativesK Find the area under the standard normal curve to the right of z 1 25 OA 0 5843 OB 0 7193 OC 0 6978 OD 0 8944

Calculus
Application of derivativesRecall that in general if we have a limit of the following form where both f x 0 and g x 0 then the limit may or may not exist and is called an indeterminate form of type 0 0 We note that this type of limit can be evaluated for certain functions by dividing numerator and denominator by the highest power of x that occurs in the denominator However we are give the following limit where this method does not work f x lim x a g x f x lim x10 For the evaluation of indeterminate forms of type ew where other methods do not work we use L Hospital s Rule This rule states that if f and g are differentiable and g x 0 on an open interval I that contains a except possibly at a where lim f x 0 and lim g x 0 then the following holds as long as the limit on the right side exists or is or x a x a g x cos x 1 x 4x4 We can apply L Hospital s Rule for the given function using a 0 f x cos x 1 1x f x lim lim x a g x x a g x 16x x and g x 4x4 We need to first find both f x and g x


Calculus
Application of derivativesregulation size by the International Football Association FIFA field is variable if it is between 100 and 110 m long and between 64 and 73 m wide it is considered DA 50 Part a A particular field is 108 5 m long and 68 5 m wide What is the area of this field in square meters A sin cotan m cos asin tan 7 8 9 9 HOME acos E TAL 4 S 6 Grade Summary Deductions Potential 0 100 Submissions Attempts remaining 10
Calculus
Application of derivativesGiven that cos 0 3 10 10 and is in Quadrant IV what is sin 0 Provide your answer below
Calculus
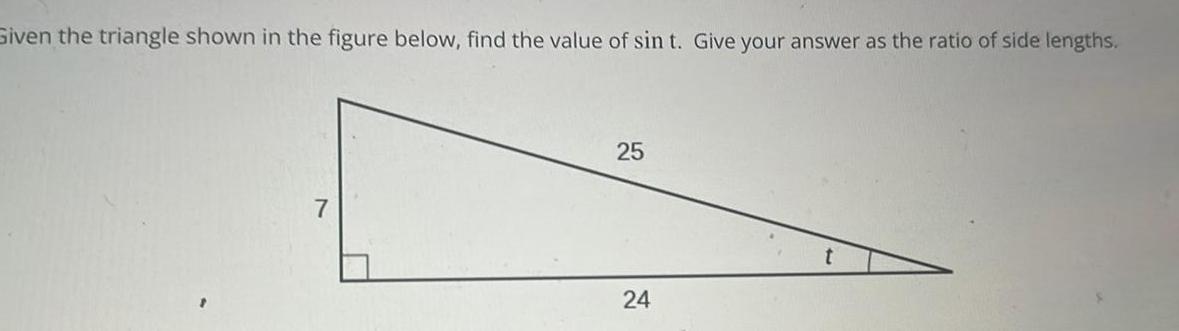
Application of derivativesGiven the triangle shown in the figure below find the value of sin t Give your answer as the ratio of side lengths 25 24
Calculus
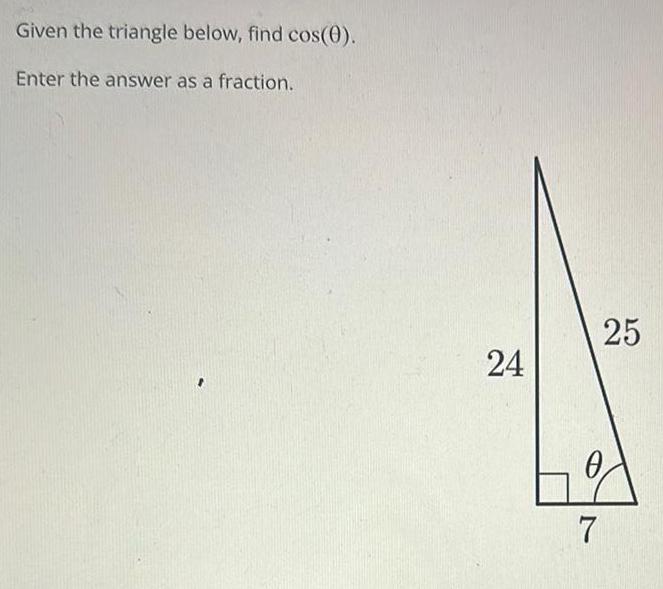
Application of derivativesGiven the triangle below find cos 0 Enter the answer as a fraction 24 7 25

Calculus
Application of derivatives0 Provide your answer below 65 ft A hiker is standing on the ground looking up to the top of a tree with an angle of elevation of 0 54 The figure shown above represents the situation where the base of the triangle is the eye level of the hiker and the vertical side is the tree Based on the dimensions shown in the figure what is the distance a in feet from the hiker to the base of the tree Rounc one decimal place

Calculus
Application of derivativesIn terms of the cotangent of a positive acute angle what is the expression for cot 55
Calculus
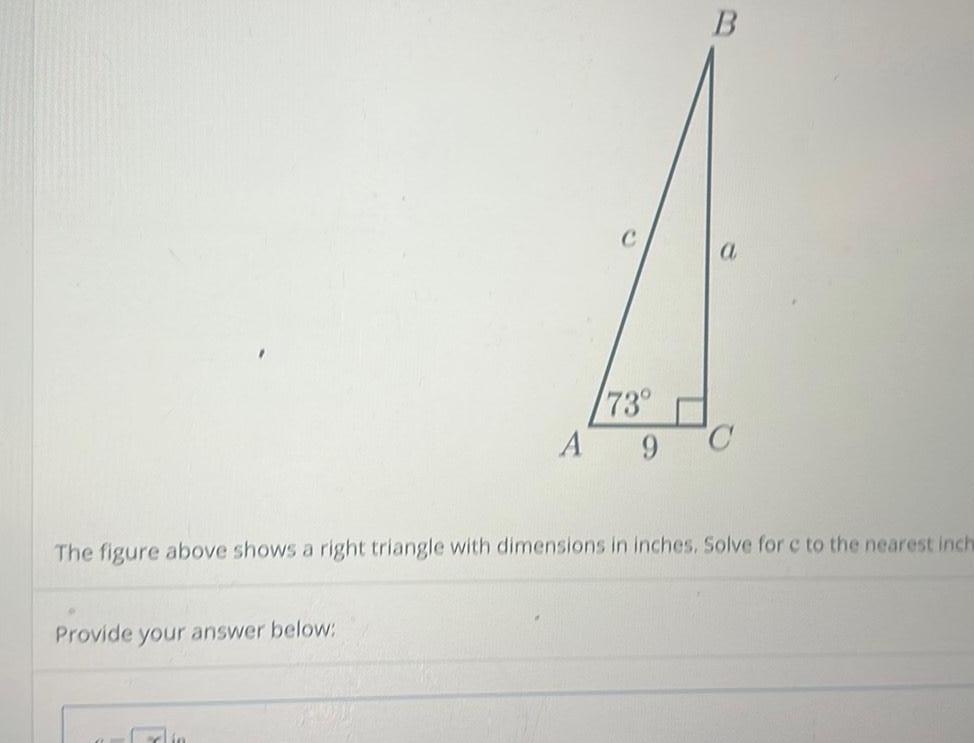
Application of derivativesProvide your answer below B The figure above shows a right triangle with dimensions in inches Solve for c to the nearest inch Erlin 73 A 9 C

Calculus
Application of derivatives1 x x 6x 9x 4 of the function What is are the local minimum minima Select the correct choice below and if necessary fill in the answer box to complete your choice A The local minimum minima is are atx Use a comma to separate answers as needed Type integers or simplified fractions B The function has no local minimum What is are the local maximum maxima Select the correct choice below and if necessary fill in the answer box to complete your choice A The local maximum maxima is areat x Use a comma to separate answers as needed Type integers or simplified fractions OB The function has no local maximum
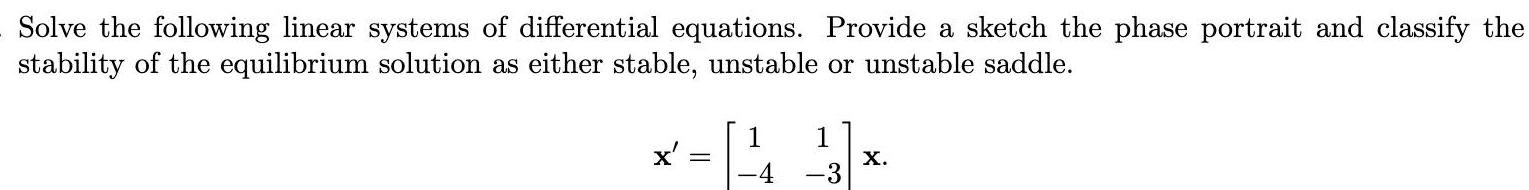
Calculus
Application of derivativesSolve the following linear systems of differential equations Provide a sketch the phase portrait and classify the stability of the equilibrium solution as either stable unstable or unstable saddle x 1 3 X

Calculus
Application of derivatives2 Evaluate cos 8 B where sine with in the second quadrant and tan with B in the fourth quadrant

Calculus
Application of derivativesWhat is the value of cot 0 if the terminal side of angle 0 intersects the unit circle in the first quadrant at x 45
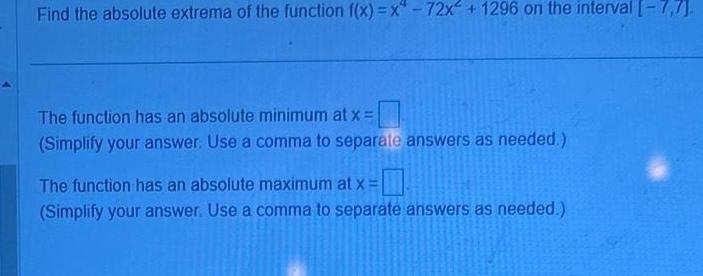
Calculus
Application of derivativesFind the absolute extrema of the function f x x 72x 1296 on the interval 7 7 The function has an absolute minimum at x Simplify your answer Use a comma to separate answers as needed The function has an absolute maximum at x Simplify your answer Use a comma to separate answers as needed

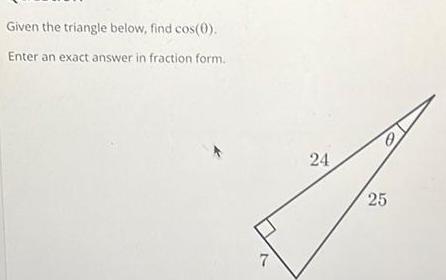
Calculus
Application of derivativesGiven the triangle below find cos 0 Enter an exact answer in fraction form 7 24 25
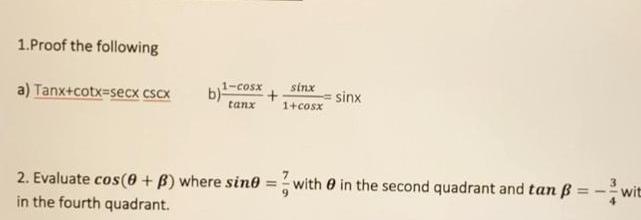
Calculus
Application of derivatives1 Proof the following a Tanx cotx secx cScx 1 cosx tanx b sinx 1 cosx sinx 2 Evaluate cos 0 B where sine with in the second quadrant and tan B wit in the fourth quadrant
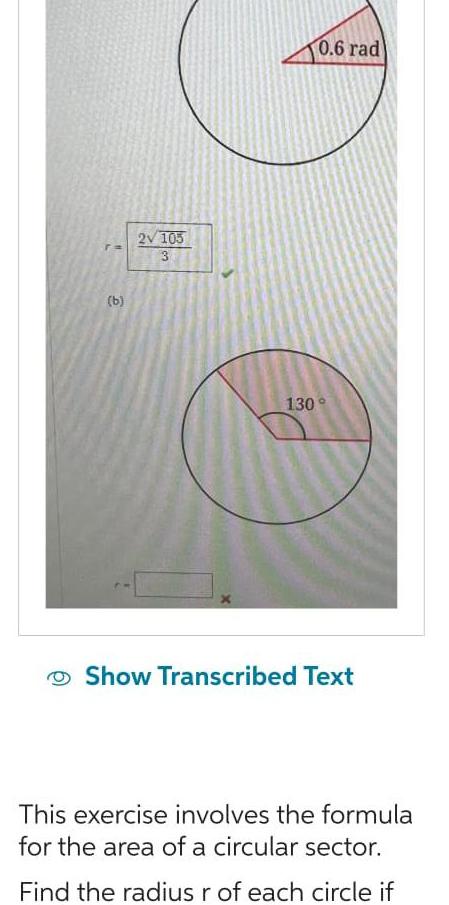
Calculus
Application of derivativesb 2V 105 3 0 6 rad 130 Show Transcribed Text This exercise involves the formula for the area of a circular sector Find the radius r of each circle if

Calculus
Application of derivativesFind the coordinates of the point on the unit circle at an angle of 3 4 Give your answer in the form x y and leave any fractions in fraction form

Calculus
Application of derivatives9 Hint Let c x au y bv and z cw Then integrate over an appropriate region in uvw space Evaluate the given integral over the solid ellipsoid a b SSS3 xyz dx dydz x y z D HI
Calculus
Application of derivativesSolve the system u 2x y v 2x 3y for x and y in terms of u and v Then find the x y value of the Jacobian Find the image under the transformation of the d u v triangular region with vertices 0 0 3 6 and 3 2 in the xy plane Sketch the transformed region in the uv plane The function for x in terms of u and vis x

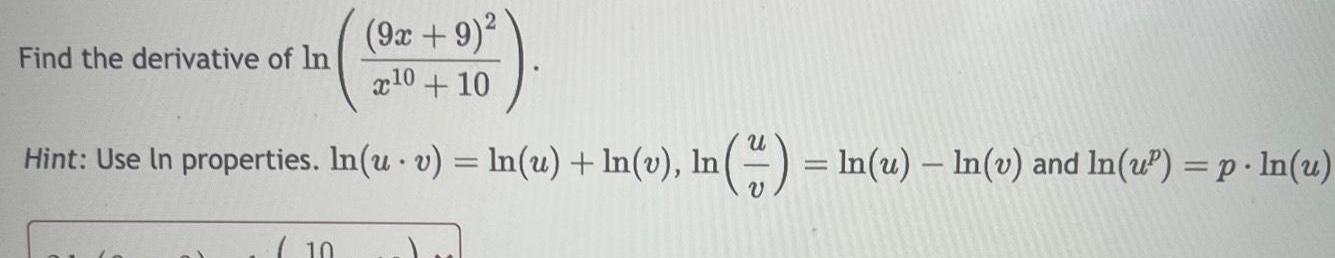
Calculus
Application of derivatives9x 9 x10 10 Hint Use In properties In u v ln u ln v ln ln u ln v and ln u p ln u Find the derivative of In 10



Calculus
Application of derivativesWrite cos F 00 11 in terms of the cosine of a positive acute angle Provide your answer below

Calculus
Application of derivativesQuestion The terminal side of angle intersects the unit circle in the first quadrant at y What are the exact values of sin cos 0 Provide your answer below