Application of derivatives Questions and Answers

Calculus
Application of derivativesFind the particular antiderivative of the following derivative that satisfies the given condition dR dt 64 1 R 4 50
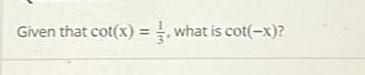
Calculus
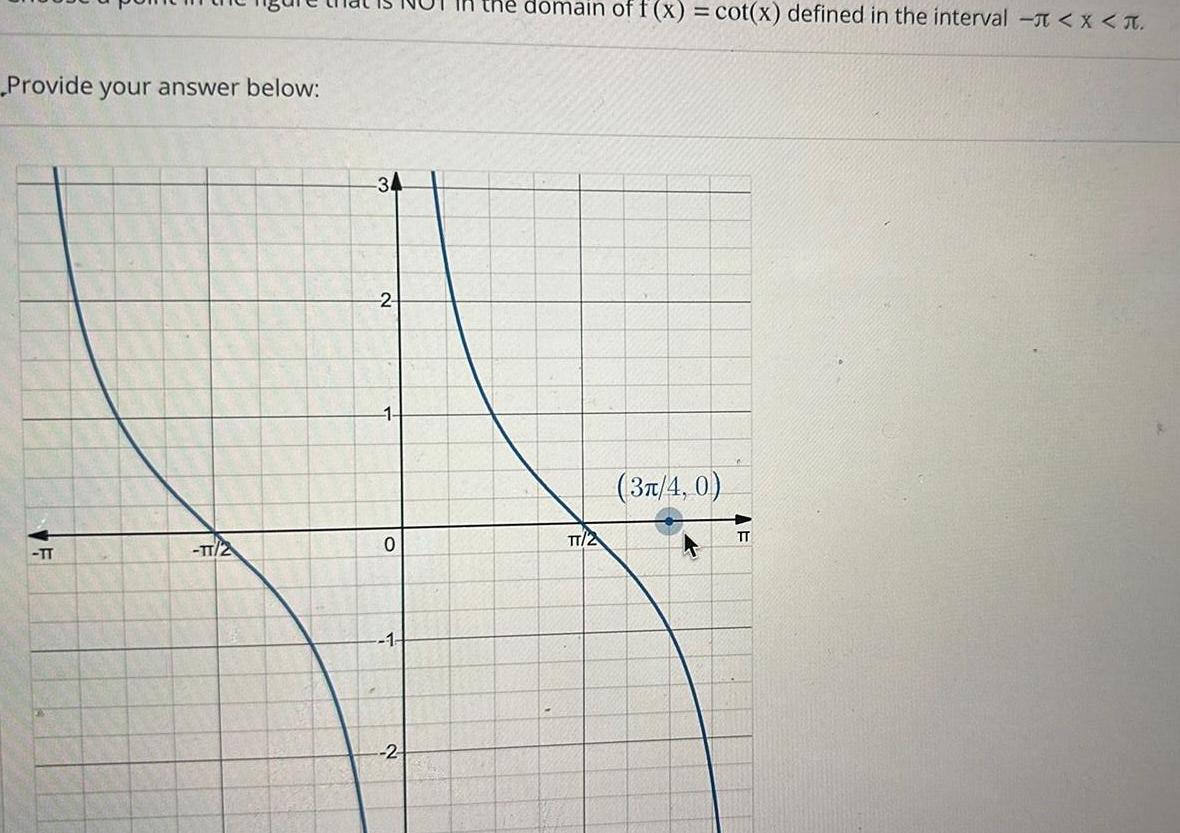
Application of derivativesChoose one point in the figure that is NOT in the domain of cot x defined in the Provide your answer below TT 12 TTA TT 2 4 0 0 TT 2 12 TT
Calculus
Application of derivatives2 Simplify the following a sinx cos x secx cscx sin cos x b cos x sinx 4 tanx secx 2 secx c 6 tanx secx 2 secx sin x cos x d sin x cos x 1 e sin x cos x secx sinx sinx cos x 1 cos x g tanx 2 cosx sinx sinx 1 cos x sin u 3 5 and and 3 Assuming that evaluate each of the following exactly a cos u v c tan u v b sin u v d cos u v sin v 4 5 that u and v are between 0 am

Calculus
Application of derivativessin u 5 and and 3 Assuming that evaluate each of the following exactly a cos u v c tan u v b sin u v d cos u v sin v tan x b d cs x 27 5 that u and v are between 0 and 2 4 Find an equivalent expression for each of the following a sed x c cof x 27
Calculus
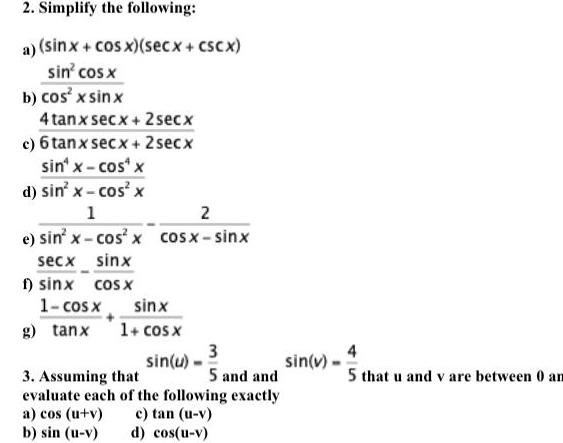
Application of derivativesProvide your answer below TT TT 2 2 1 0 1 2 In the domain of f x cot x defined in the interval X T TT 2 3 4 0 TT

Calculus
Application of derivativesThe Taylor series for f x x at 2 is cn x 2 3 Find the first few coefficients CO C1 C2 C3 C4 n 0
Calculus
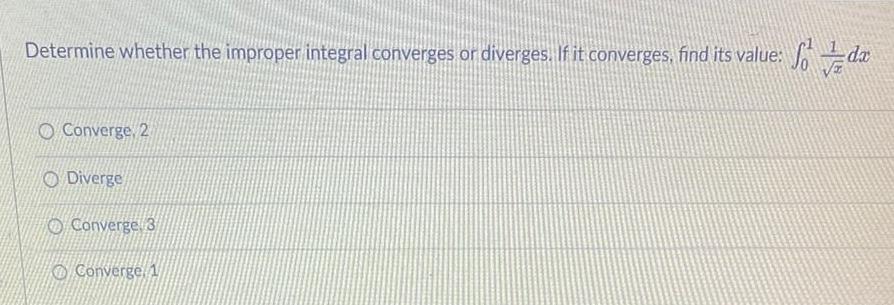
Application of derivativesSo Determine whether the improper integral converges or diverges If it converges find its value da Converge 2 Diverge Converge 3 Converge 1

Calculus
Application of derivativesAssume a is opposite side a is opposite side b and y is opposite side c Solve each triangle for the unknown sides and angles if possible If there is more than one possible solution give both 21 y 41 2 a 2 49 b 3 13 23 a 120 b 6 c 7 22 B 58 7 a 10 6 c 15 7 24 y 115 a 18 b 23

Calculus
Application of derivatives5 15 pts If tanx 1 6 and x is in the fourth quadrant 6 18 pts Prove the following tan x sin x tan xsin x

Calculus
Application of derivatives3 The asymptotic expression f n O g n means there exists a constant c 0 and an integer No such that for all nz No f n sc g n Consider f n n and g n gn It is a fact that n O lg Determine the smallest positive constant No such that for all n No f n sc g n given that c 32 Be careful The floor over the log makes this problem interesting 5 points

Calculus
Application of derivativesind the interval of convergence of the power series Be sure to include a check for convergence at the endpoints of the interval If the answer is an interval enter your answer using interval notation If the answer is a finite set of values enter nswers as a comma separated list of values 1 x 2 n8n n 1 6 10

Calculus
Application of derivativesThe function f x 16x 4 is one to one a Find the inverse of f and check the answer b Find the domain and the range of f and f c Graph f f1 and y x on the same coordinate axes
Calculus
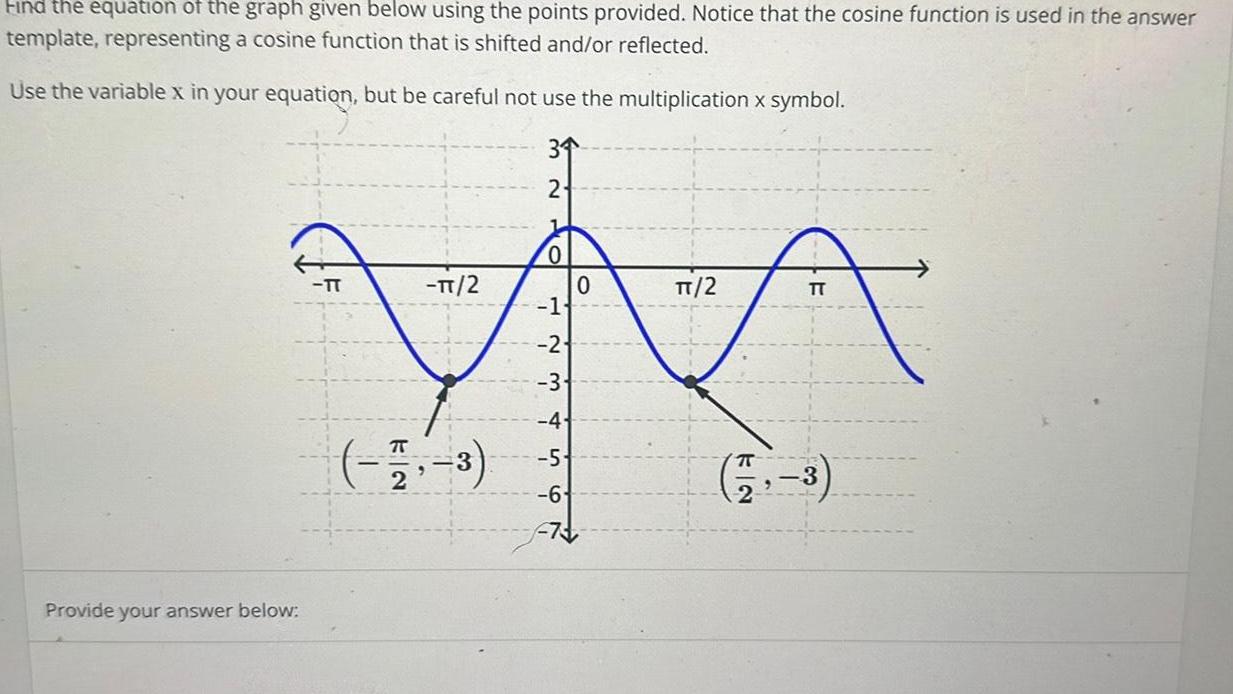
Application of derivativesFind the equation of the graph given below using the points provided Notice that the cosine function is used in the answer template representing a cosine function that is shifted and or reflected Use the variable x in your equation but be careful not use the multiplication x symbol 34 2 Provide your answer below N TT TT 2 2 3 0 1 2 3 6 0 TT 2 E 3 t

Calculus
Application of derivativesdz Draw a dependency diagram and write a chain rule formula for where z h m n m g q n f q dq Choose the correct dependency diagram below OA dn n z h N OB dz dq Choose the correct chain rule formula A dz dq OC dz dq OD dz 11 dz dh dm m az dm az dn am dq on dq dz dm dz dn am dq an dq dz dm dz dn am dq an dq az dn az dm I B m z h m n dz dm dm dq 9 Uz on dn B n C m q dz dm dm dq z h m n m m n dq dz n q
Calculus
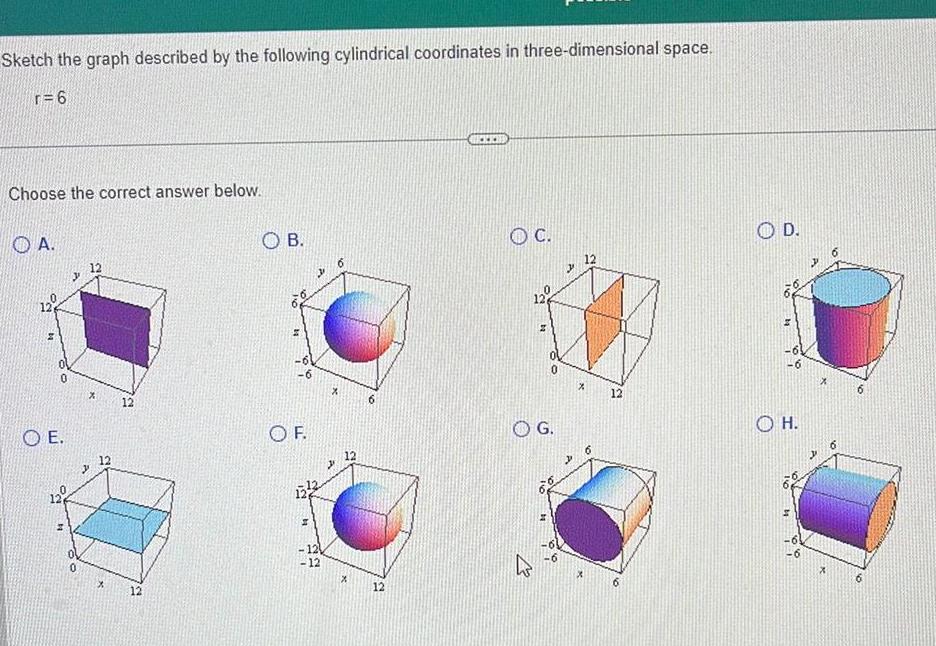
Application of derivativesSketch the graph described by the following cylindrical coordinates in three dimensional space r 6 Choose the correct answer below A 12 OE 12 12 12 OB 11 6 OF 126 H 12 12 X 12 X 12 O C 12 0 O G X 12 OD OH X
Calculus
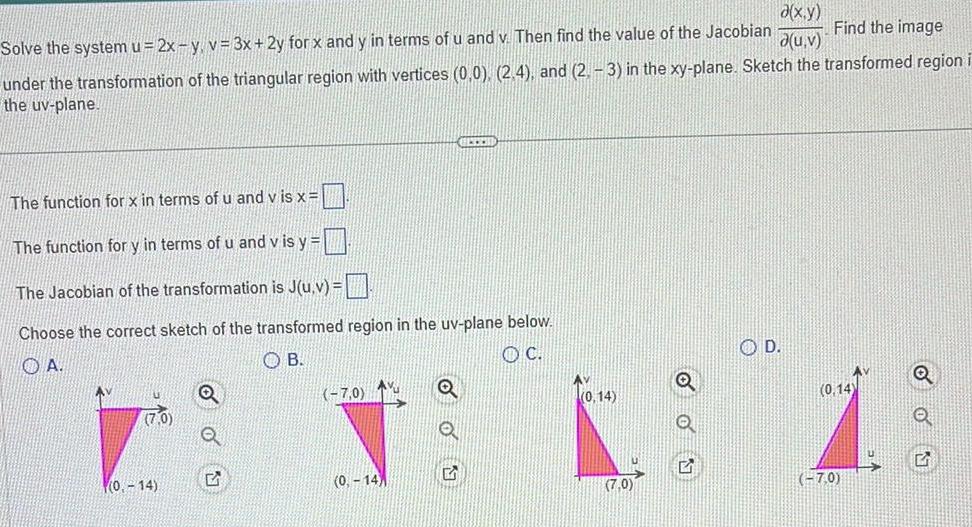
Application of derivativesa x y Find the image d u v Solve the system u 2x y v 3x 2y for x and y in terms of u and v Then find the value of the Jacobian under the transformation of the triangular region with vertices 0 0 2 4 and 2 3 in the xy plane Sketch the transformed region the uv plane The function for x in terms of u and vis x The function for y in terms of u and v is y The Jacobian of the transformation is J u v Choose the correct sketch of the transformed region in the uv plane below OA OB OC 0 14 OU 7 0 0 14 ELTER Q Q 0 14 7 0 D 0 14 7 0 ON
Calculus
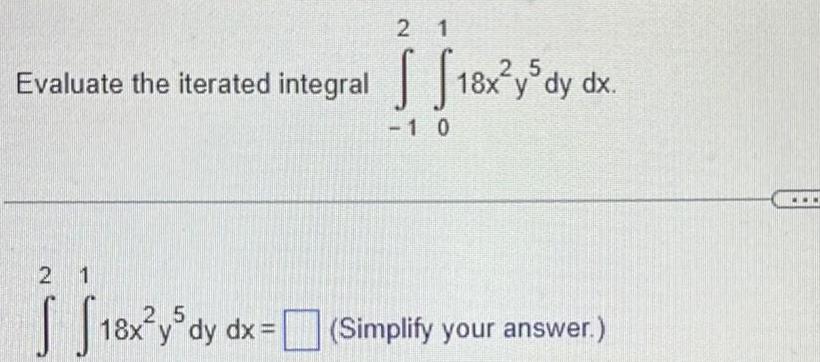
Application of derivativesEvaluate the iterated integral 2 1 25 S S 18x y dy dx 21 5 18x y dy 1 0 dx x Simpaty Simplify your answer



Calculus
Application of derivativesOn the graph of f x sec x and the interval 2 0 for what value of x does f x 1 Choose all answers that apply Select all that apply 2 0 37 IT 32

Calculus
Application of derivatives6 A patient is injected with a drug and t hours later the concentration of the drug remaining in the patient s mg cm What is the average concentration of drug during the 3t bloodstream is given by C t 1 36 first 8 hours after the injection 10pts Use substitution

Calculus
Application of derivativesOn the graph of f x csc x and the interval 2 0 set the moving point to a value of x such that f x 1 Provide your answer below 2 T 3TT 2 I 1 1 1 I 13 10 0 1 TT 2 1 I 10 5 0 5

Calculus
Application of derivativesThe equation T 0 6sin 2 t 8 101 4 describes the body temperature of a cow in Fahrenheit where t is time in 2 Do not include the units in your hours What is the temperature of the cow to the nearest tenth of a degree when t answer NOTE The angle is in radians
Calculus
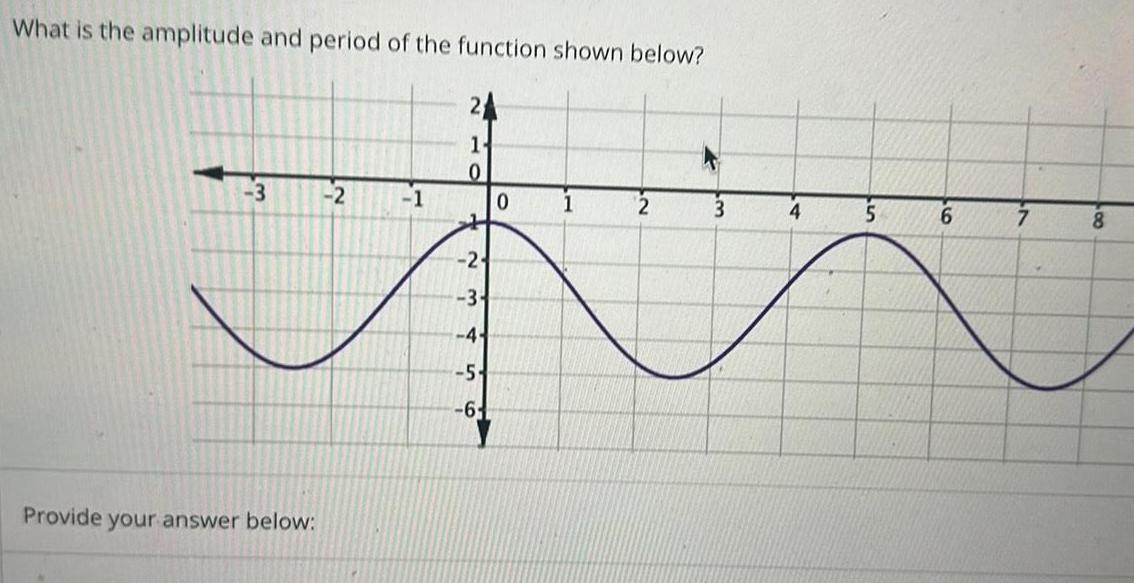
Application of derivativesWhat is the amplitude and period of the function shown below 3 Provide your answer below 2 1 1 0 1 24 3 4 5 56 6 0 2 3 4 5 6 8

Calculus
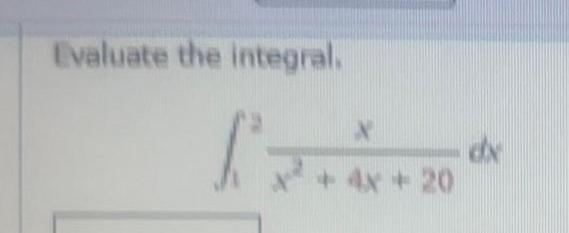
Application of derivativesExplain why each of the following integrals is improper s dx x 7 O Since the function y O Since the function y O Since the integral 1 O Since the function y O Since the integral dx x 9 O Since the function y O Since the function y O Since the function y 500 1 x 7 has an infinite discontinuity at x7 the integral is a Type 1 improper integral O Since the integral O Since the integral I 1 x 7 1 X dx dx x 7 has an infinite discontinuity at x 1 the integral is a Type 2 improper integral has an infinite discontinuity at x 7 the integral is a Type 2 improper integral has an infinite interval of integration it is a Type 1 improper integral has an infinite interval of integration it is a Type 2 improper integral 1 has an infinite discontinuity at x 9 the integral is a Type 1 improper integral x 9 1 x 9 1 9 has an infinite discontinuity at x 9 the integral is a Type 2 improper integral dx x 9 dx 9 has an infinite discontinuity at x 8 the integral is a Type 1 improper integral has an infinite interval of integration it is a Type 1 improper integral has an infinite interval of integration it is a Type 2 improper integral

Calculus
Application of derivativesa Sketch the garden use INSERT SHAPES and INSERT DRAW b How many meters of stone do you need to create the border FO 12 9 Danielle wants to create a patio in her backyard She would like it to be 6 ft by 12 ft What is the area of the patio Sketch the patio INSERT SHAPES and INSERT DRAW C 13 10 A basketball with a diameter 24 cm is packaged so that it just fits into a cube shaped box Calculate the amount of empty space in the box round to 1 decimal place T 17
Calculus
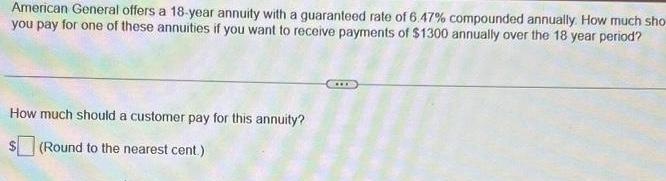
Application of derivativesAmerican General offers a 18 year annuity with a guaranteed rate of 6 47 compounded annually How much sho you pay for one of these annuities if you want to receive payments of 1300 annually over the 18 year period How much should a customer pay for this annuity Round to the nearest cent
Calculus
Application of derivativesz and z where z 9xy 3x y x 3s 3t and y 3s 3t 4 0 Type an expression usings and t as the variables 4 0 Type an expression using s and t as the variables
Calculus
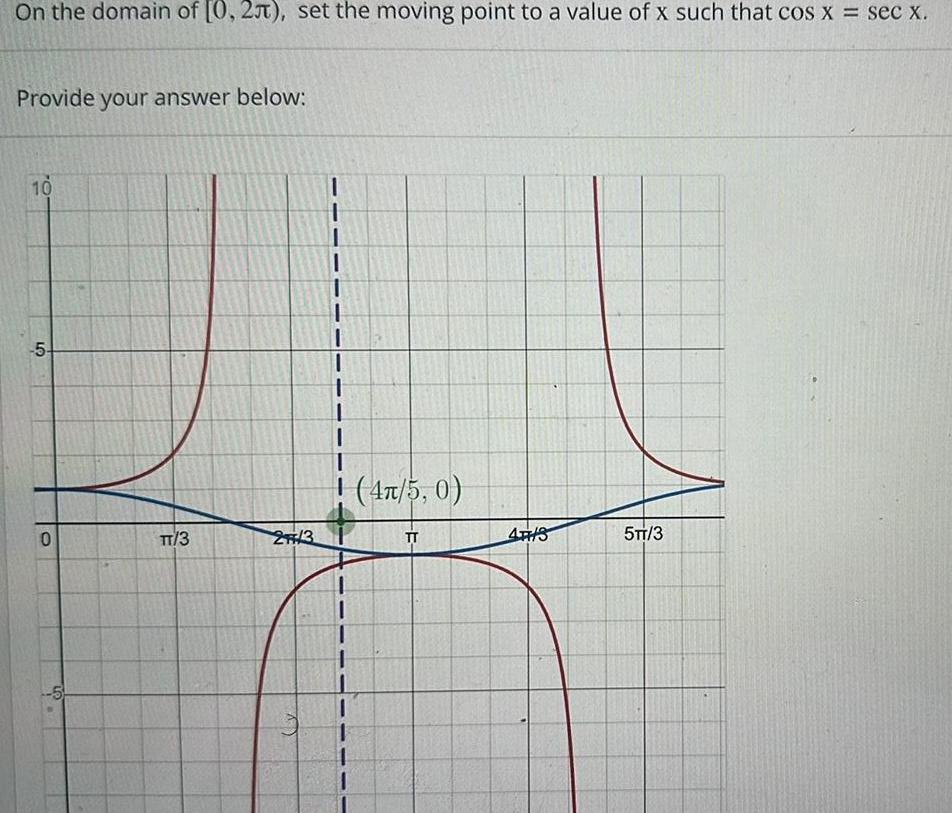
Application of derivativesOn the domain of 0 2 set the moving point to a value of x such that cos x sec x Provide your answer below 10 5 0 5 TT 3 27 3 y 13 I 1 1 T 4 5 0 TT 4TH 3 5TT 3

Calculus
Application of derivativesQuestion The equation d 5sin 24t measures the displacement of a swinging pendulum in simple harmonic motion t is measured in seconds and d is measured in centimeters What is the displacement to the nearest centimeter when t 4 NOTE The angle is in radians Provide your answer below
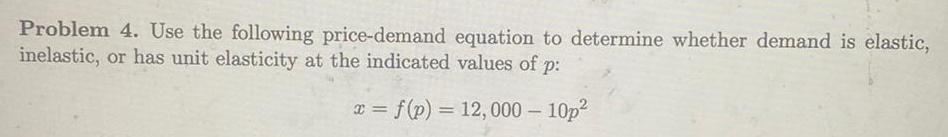
Calculus
Application of derivativesProblem 4 Use the following price demand equation to determine whether demand is elastic inelastic or has unit elasticity at the indicated values of p x f p 12 000 10p

Calculus
Application of derivativesProblem 3 A 7 ft tall person is walking away from a 20 ft tall lamppost at a rate of 5 ft sec Assume the scenario can be modeled with right triangles At what rate is the length of the person s shadow changing when the person is 16 ft from the lamppost
Calculus
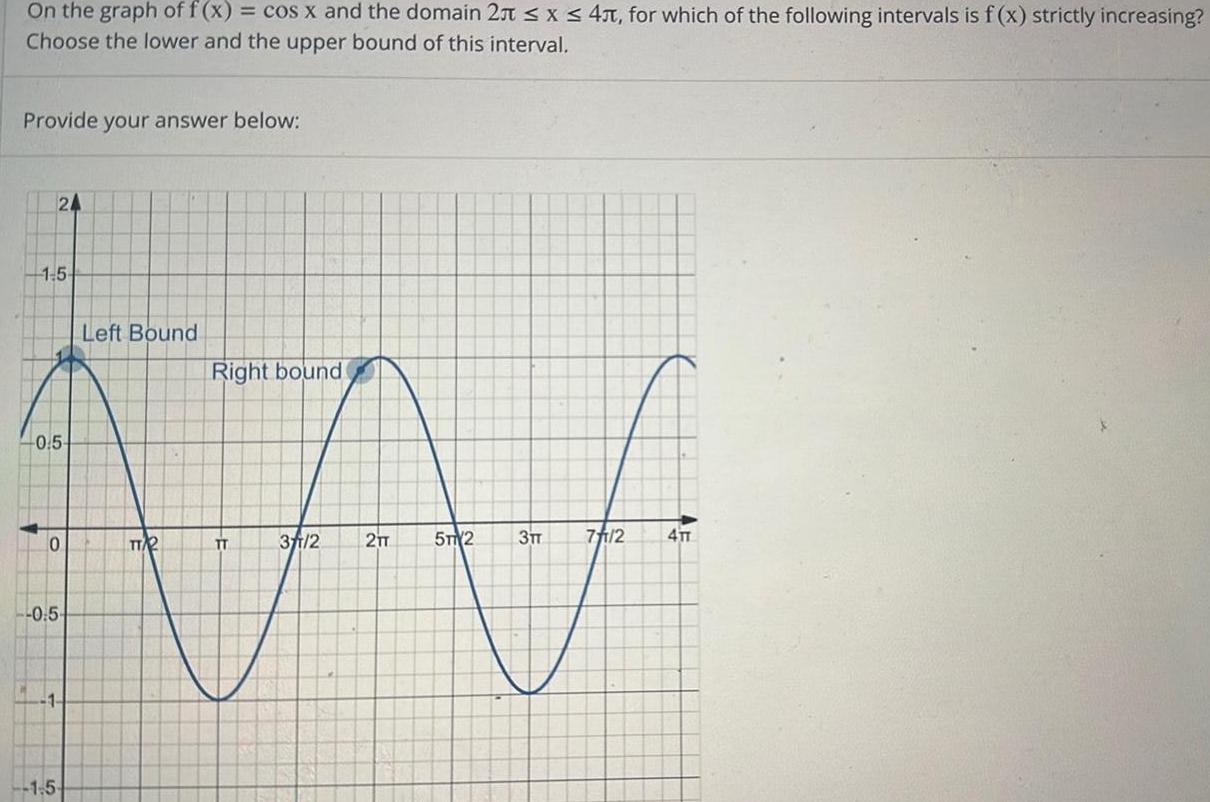
Application of derivativesOn the graph of f x cos x and the domain 2 x 4 for which of the following intervals is f x strictly increasing Choose the lower and the upper bound of this interval Provide your answer below 24 1 5 0 5 0 0 5 1 1 5 Left Bound TT2 Right bound TT 31 2 2TT 5T2 3TT 7 11 2 4TT
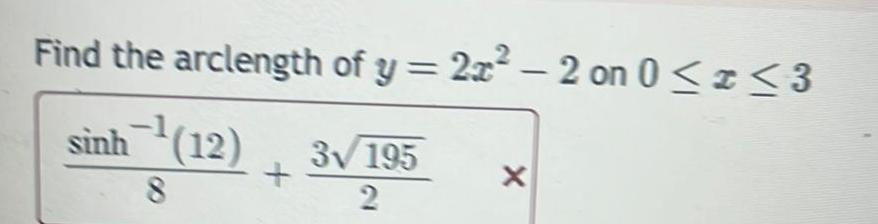

Calculus
Application of derivativesOn the graph of f x sin x and the domain 2 x 4 for which of the following intervals is f x strictly increasin Make sure to use the blue points to represent the first interval and the red points to represent the second period Provide your answer below 3 2 1 2T 2n 0 1 5TT 2 3 0 7TT 2 4 0 1 4TT 77 2 1
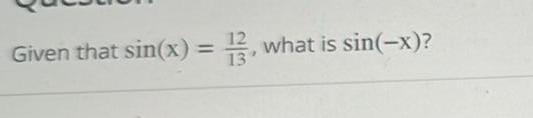

Calculus
Application of derivativesFind the surface area of revolution about the x axis of y 4x 7 over the interval 0 x
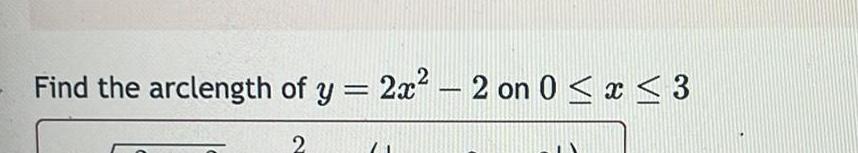
Calculus
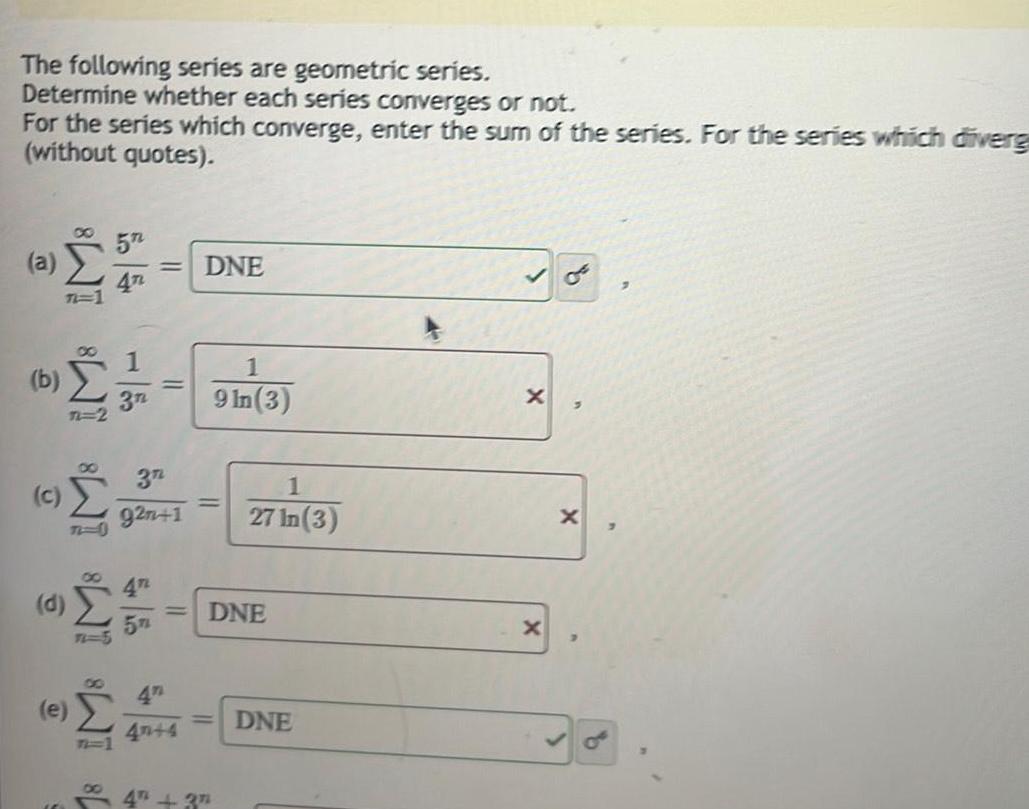
Application of derivativesThe following series are geometric series Determine whether each series converges or not For the series which converge enter the sum of the series For the series which diverg without quotes a b c O 18 IM8 IM8 IM8 M8 IM8 n 2 e 5 4T 1 3n 3n 92n 1 40 4 DNE 1 9 In 3 4 37 1 27 In 3 DNE DNE X X 3 X 7
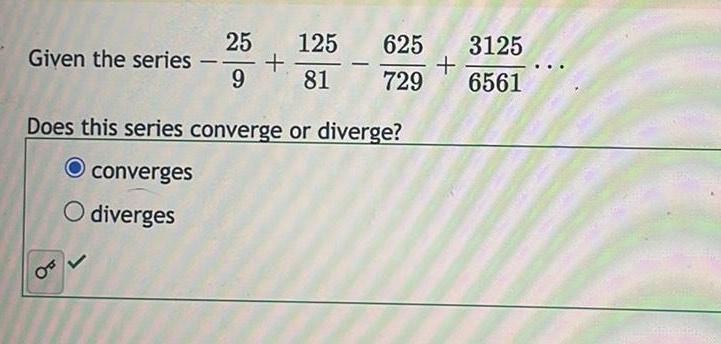
Calculus
Application of derivativesGiven the series OF 25 125 9 81 625 3125 729 6561 Does this series converge or diverge O converges O diverges

Calculus
Application of derivativesFind the limit of the sequence 1n 8n 5 2n 8n 6 an 2 n 8n 5 2 1 X syntax incomplete
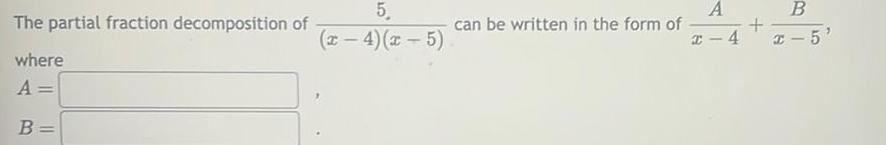
Calculus
Application of derivativesThe partial fraction decomposition of where A B 5 x 4 x 5 can be written in the form of I A 4 B x 5
Calculus
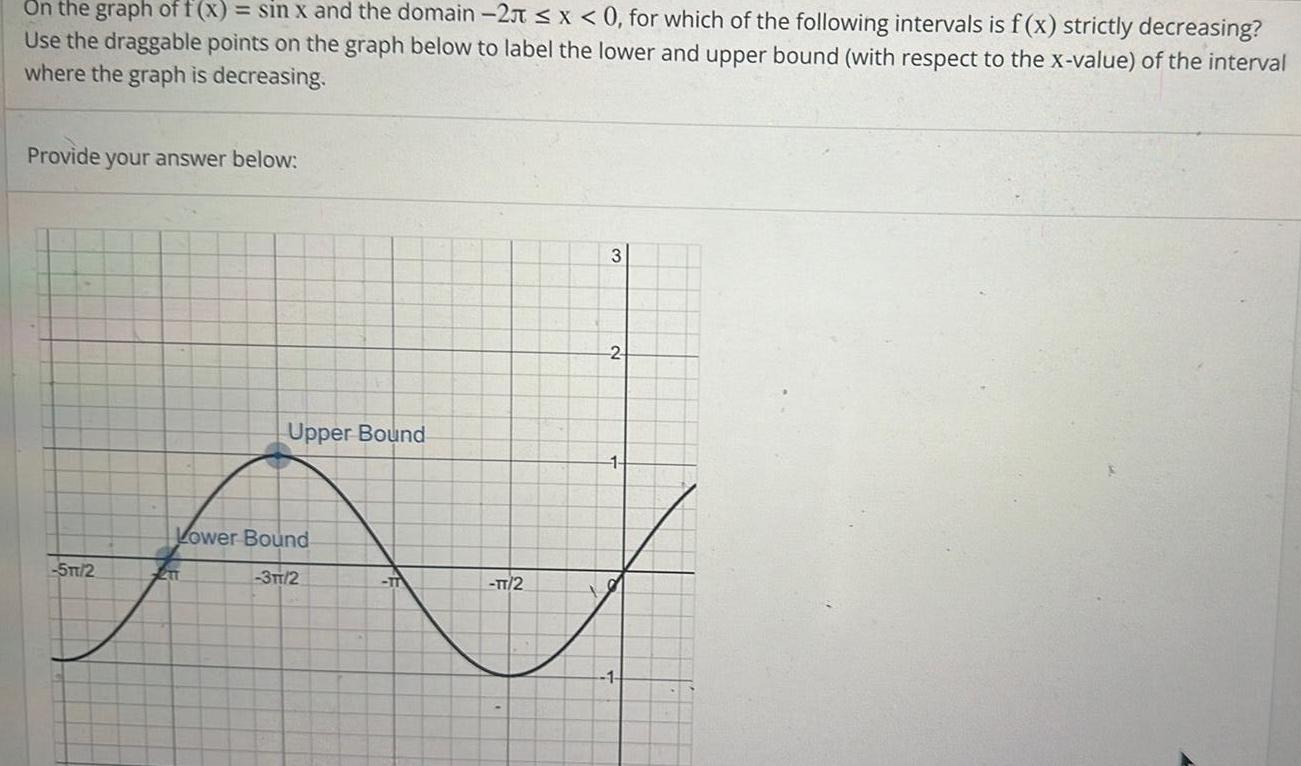
Application of derivativesOn the graph of f x sin x and the domain 2 x 0 for which of the following intervals is f x strictly decreasing Use the draggable points on the graph below to label the lower and upper bound with respect to the x value of the interval where the graph is decreasing Provide your answer below 5m 2 Upper Bound Lower Bound 3TT 2 4TT TT 2 3 2
Calculus
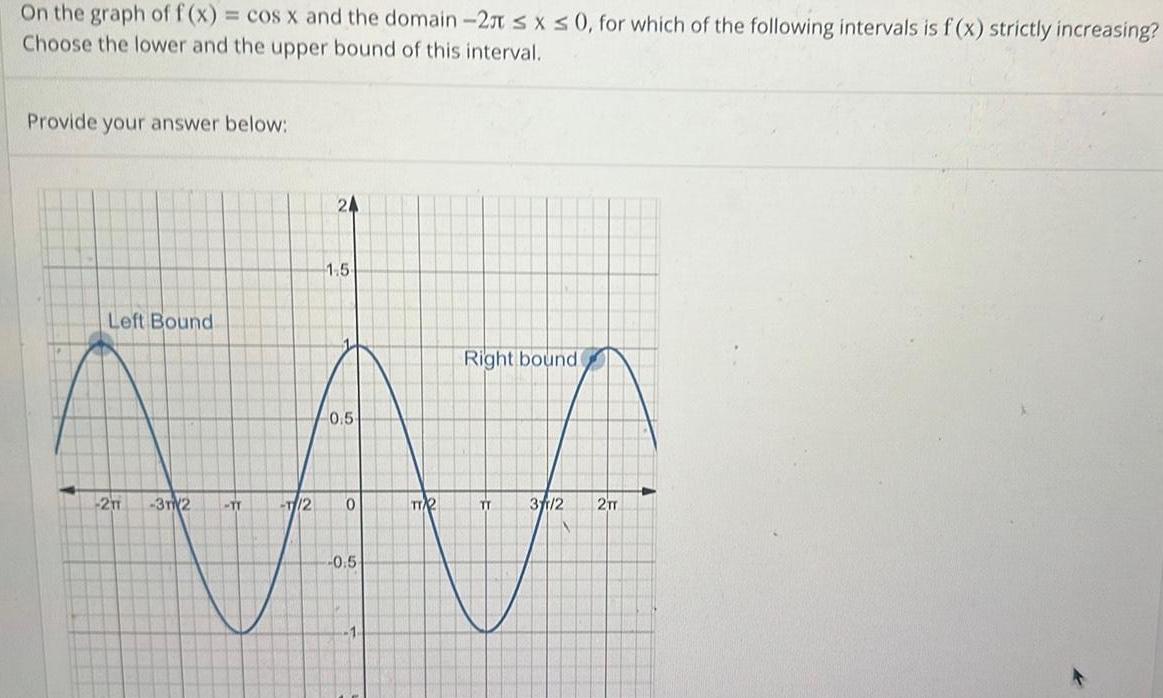
Application of derivativesOn the graph of f x cos x and the domain 2 x 0 for which of the following intervals is f x strictly increasing Choose the lower and the upper bound of this interval Provide your answer below Left Bound 2TT 32 TT T 2 24 1 5 0 5 0 0 5 TT 2 Right bound TT 3 1 2 2TT
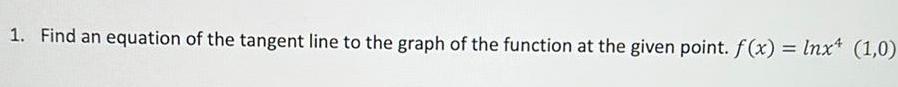
Calculus
Application of derivatives1 Find an equation of the tangent line to the graph of the function at the given point f x Inx4 1 0
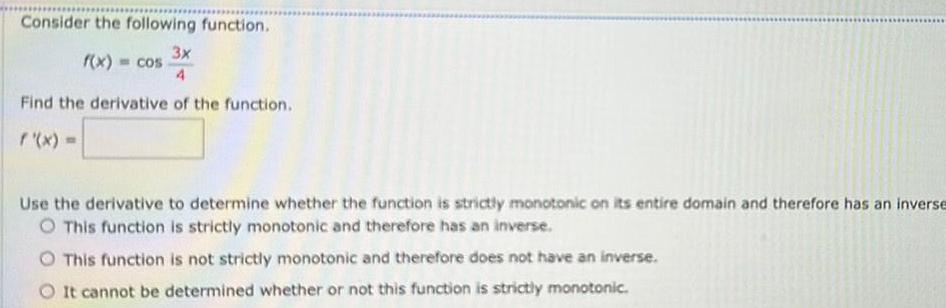
Calculus
Application of derivativesConsider the following function 3x 4 Find the derivative of the function f x f x cos Use the derivative to determine whether the function is strictly monotonic on its entire domain and therefore has an inverse O This function is strictly monotonic and therefore has an inverse This function is not strictly monotonic and therefore does not have an inverse It cannot be determined whether or not this function is strictly monotonic

Calculus
Application of derivativesdentify the real numbers X0 X X2 X3 and x4 in the figure such that each of the following is true Select all that appl a f x 0 xo 0x1 0x2 b 3 XA none of these F x 0 O xo 0x1 0x2 3 X4 Onone of these d c f x does not exist O xo 0x 0x2 X3 X4 none of these f has a relative maximum 0 0 Xo 0x1 X2 X3 X4 none of these e f has a point of inflection xo 0 x1 n X3 X

Calculus
Application of derivativesThe velocity of an automobile starting from rest is given by the equation below where v is measured in feet per second and t is the time in seconds Round your answers to three decimal pla v t 95t 6t 18 a Find the acceleration at 5 seconds ft sec b Find the acceleration at 10 seconds ft sec2 c Find the acceleration at 20 seconds ft sec