Differential equations Questions and Answers

Calculus
Differential equations42Y BIOMEDICAL CHOLESTEROL An experimental drug lowers a patient s blood serum cholesterol at the rate of t 25 t2 units per day where t is the number of days since the drug was administered 0 t 5 Find the total change during the first 3 days

Calculus
Differential equationsIn this question we will use a spreadsheet to approximate the root of f x x5 3x 9 using Newton s method with 1 The picture below shows a spreadsheet being used to perform the calculation A downwards arrow indicates the contents from the cell above will be copied down a Which entry should go in cell A1 O 5 B1 4 9 B1 2 A1 5 3 A1 3 9 00 5 A1 4 9 A1 2 O B1 5 3 B1 3 9 01 b Which entry should go in cell B1 00 O A1 5 3 A1 3 9 01 O 5 B1 4 9 B1 2 O B1 5 3 B1 3 9 O 5 A1 4 9 A1 2 c Which entry should go in cell C1 O A1 5 3 A1 3 9 O B1 5 3 B1 3 9 O 5 B1 4 9 B1 2 A 2 A1 B1 C1 1234 B C

Calculus
Differential equationsA rectangle is inscribed with its base on the x axis and its upper corners on the parabola y 5x What are the dimensions of such a rectangle with the greatest possible area Width 2 5 Height 5

Calculus
Differential equations1 3 2 A baseball diamond is a square with sides of 90 feet To the nearest foot how long is a throw to first base from third base the child to reach the water 3 A soccer ball is kicked from the ground and its height in meters above ground is modeled by the function 4 9t 19 6t where t represents the time in seconds after the ball is kicked How long is the ball in 0 4 9 19 6t e air 04 9t

Calculus
Differential equationsUse Newton s Method with the function f z z 2 and initial value o 1 to calculate 21 22 23
Calculus
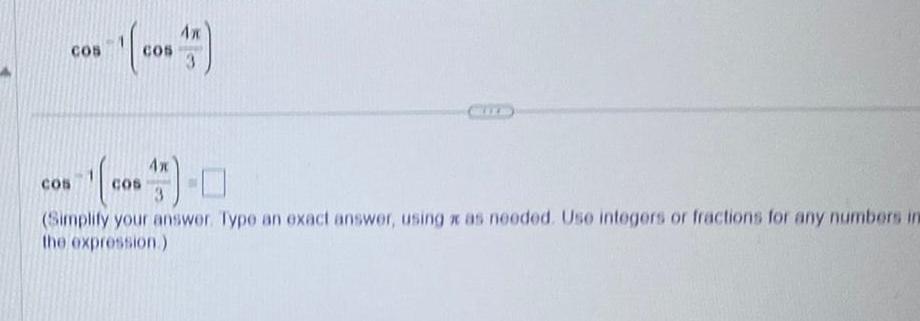
Differential equationsCOS COS COS 3 4x COS 3 Simplify your answer Type an exact answer using x as needed Use integers or fractions for any numbers in the expression

Calculus
Differential equationswatch the video that describes finding inverse sine values Click here to watch the video Find the exact value of the expression sin 9 sin 12 0 Type an exact answer using x as m

Calculus
Differential equationsFind an equation of the ellipse with the given characteristics Vertices 0 18 eccentricity
Calculus
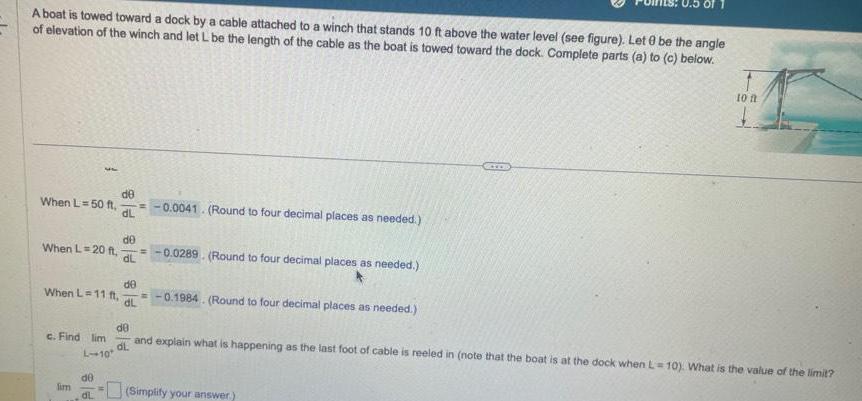
Differential equationsA boat is towed toward a dock by a cable attached to a winch that stands 10 ft above the water level see figure Let 0 be the angle of elevation of the winch and let L be the length of the cable as the boat is towed toward the dock Complete parts a to c below When L 50 ft When L 20 ft 37 When lim de dL de dl de nL 11 ft 0 1984 Round to four decimal places as needed dL 0 0041 Round to four decimal places as needed de 0 0289 Round to four decimal places as needed dL de c Find lim and explain what is happening as the last foot of cable is reeled in note that the boat is at the dock when L 10 What is the value of the limit dl L 10 10 ft Simplify your answer

Calculus
Differential equationsK The figure to the right shows the shape and dimension of a small dam Assuming the water level is at the top of the dam and that the shape of the dam is an isosceles triangle find the total force on the face of the dam OB 0 1000 9 8 27 y 3 dy The total force is 21 45x10 N CIT 18 m 27m Save nended Round to the nearest hundredth Corre
Calculus
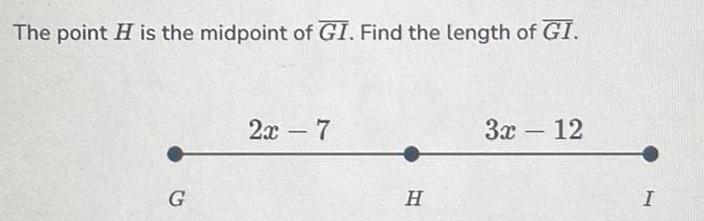
Differential equationsThe point H is the midpoint of GI Find the length of GI G 2x 7 H 3x 12 I


Calculus
Differential equations847m8110m123m 81 6m 18115m781818 16m6 m5 7m Show your work here Hint To add an exponent r type exponent or press A

Calculus
Differential equationsAn outboard motor has an initial price of 1 440 A service contract costs 350 for the first year and increases 100 per year thereafter The total cost of the outboard motor in dollars after n years is given by C n 1 440 200n 40n where ne 1 Complete parts A through C A Ay 4 500 20 OB Ay 4 500 20 C 4 500 20 Q OD 4 500 20 C When is the average cost per year minimum This time is frequently referred to as the replacement time for this piece of equipment The average cost per year is minimum after years 17
Calculus
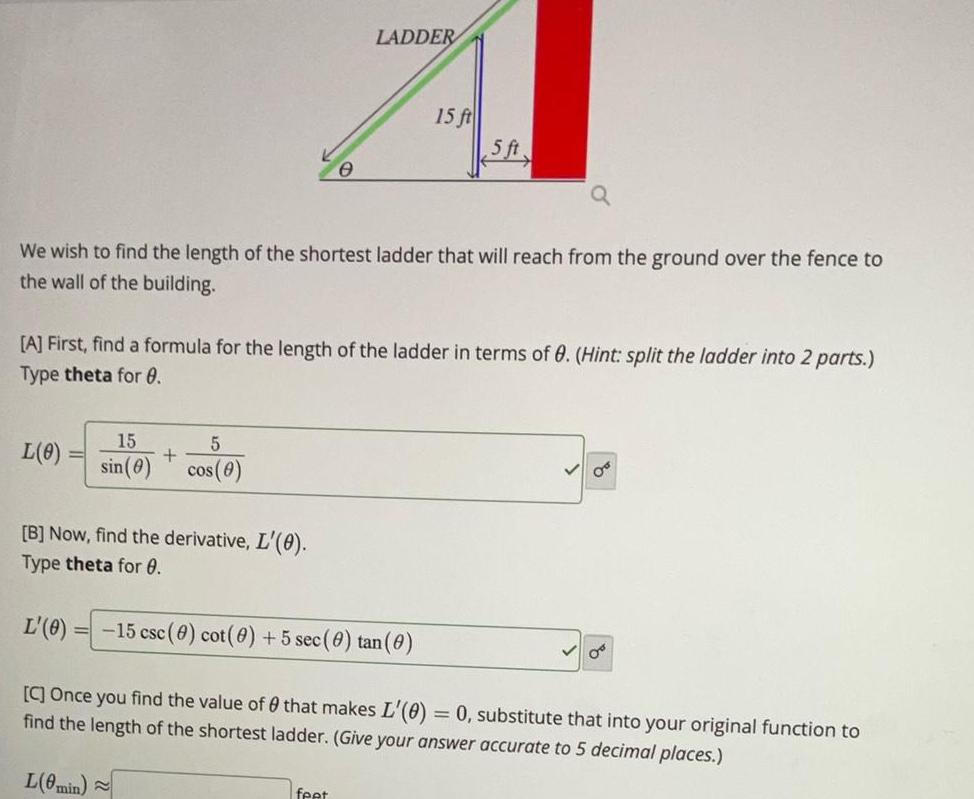
Differential equationsL 0 We wish to find the length of the shortest ladder that will reach from the ground over the fence to the wall of the building 15 5 sin 0 cos 0 B Now find the derivative L 0 Type theta for 0 L 0 0 A First find a formula for the length of the ladder in terms of 0 Hint split the ladder into 2 parts Type theta for 0 LADDER 15 ft 15 csc 0 cot 0 5 sec 0 tan 0 feet 5 ft C Once you find the value of that makes L 0 0 substitute that into your original function to find the length of the shortest ladder Give your answer accurate to 5 decimal places L 0min

Calculus
Differential equations1 In this question we will use a spreadsheet to approximate the value y 1 The picture below shows a spreadsheet being usec to implement a linear approximation scheme ie Euler s method with initial value y0 y 0 5 and a step size of 0 1 to make such an approximation in 10 steps A downwards arrow indicates that the contents from the cell above will be dragged down Column B holds values of t Column A holds approximations of y t and Column C holds approximations of y t 234 56 789 10 dy dt y 6t A 5 B 0 y 0 5 C Now using a spreadsheet or otherwise make the following approximations of y 1 for decreasing step sizes At Give your answers correct to at least 3 decimal places a Using Euler s method with 1 step t 1 starting at y 0 5 we find y 1 ANSWER b Using Euler s method with 2 steps t 1 2 starting at y 0 5 we find y 1 ANSWER c Using Euler s method with 10 steps t 1 10 starting at y 0 5 we find y 1 Answer
Calculus
Differential equationsA manufacturer of hospital supplies has a uniform annual demand for 500 000 boxes of bandages It costs 10 to one box of bandages for one year and 160 to set up the plant for production How many times a year should the company produce boxes of bandages in order to minimize the total storage and setup costs The company should produce boxes of bandages time s a year
Calculus
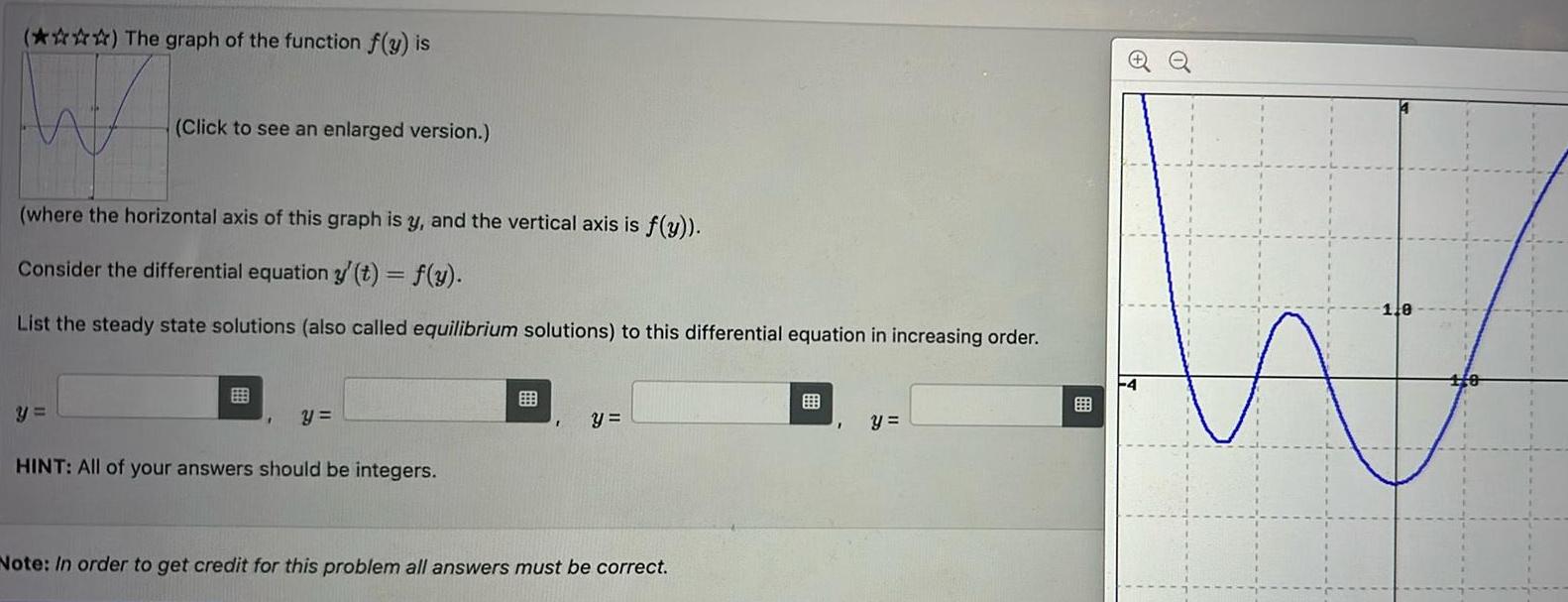
Differential equationsThe graph of the function f y is HAP Click to see an enlarged version where the horizontal axis of this graph is y and the vertical axis is f y Consider the differential equation y t f y List the steady state solutions also called equilibrium solutions to this differential equation in increasing order y HINT All of your answers should be integers y Note In order to get credit for this problem all answers must be correct y O
Calculus
Differential equationsNumerically approximate the solution to the differential equation dB dt with initial value B 900 when t 0 Compute each value using linear approximation this method is also called Euler s method A At 1 and 1 step B 1 B At 0 5 and 2 steps B 1 0 08B c At 0 25 and 4 steps B 1 Remark on an exam you may be asked to do a few simple iterations of Euler s method by hand and you may also be asked about using a spreadsheet so make sure you know how to do it both ways For this question Euler s Method leads to a nice pattern If you simplify every step you may find an easy way to do it by hand

Calculus
Differential equationsTwo lakes have populations of fish but the conditions are quite different in these lakes In the first lake the fish population is growing and satisfies the differential equation dy dt Time where t is time in years At time t 0 there were 600 fish in this lake In the second lake the population is dying due to pollution Its population satisfies the differential equation years dy dt 0 7y 0 8y and initially there were 8000 fish in this lake At what time will the fish populations in the two lakes be identical

Calculus
Differential equationsUse Euler s method with the given step size to estimate y 1 4 where y x is the solution of the initial value problem y x xy y 1 0 1 Estimate y 1 4 with a step size Ax 0 2 Answer y 1 4 2 Estimate y 1 4 with a step size Ax 0 1 Answer 11 B

Calculus
Differential equationsFor each of the following differential equations determine if the proposed function is a solution a Let C and k be constants Given the differential equation f t kf t the function f t Cet is b Given the differential equation 3y the function y 2e 3 is c Given the differential equation f t 1 f t the function f t e t is 2y d Given the differential equation 2 the function y t 3t is a solution

Calculus
Differential equationsIs each of the following functions a solution to the differential equation y 11y 30y 0 Yes Ma 1 y 3ebm 2 y 6e 5w 3 y There are only 4 attempts allowed for this question 6a 7e 0

Calculus
Differential equationsFind all values of r so that the function y x solves the differential equation x y 6xy 14y 0 into the equation and find values of r that satisfy the resulting equation Hint Plug y T If there are more than one answer use commas to separate the answere

Calculus
Differential equationsThe functions are all solutions of equation y x xy 2y 4x x 0 Find the constant c which produces a solution which also satisfies the initial condition y 5 3
Calculus
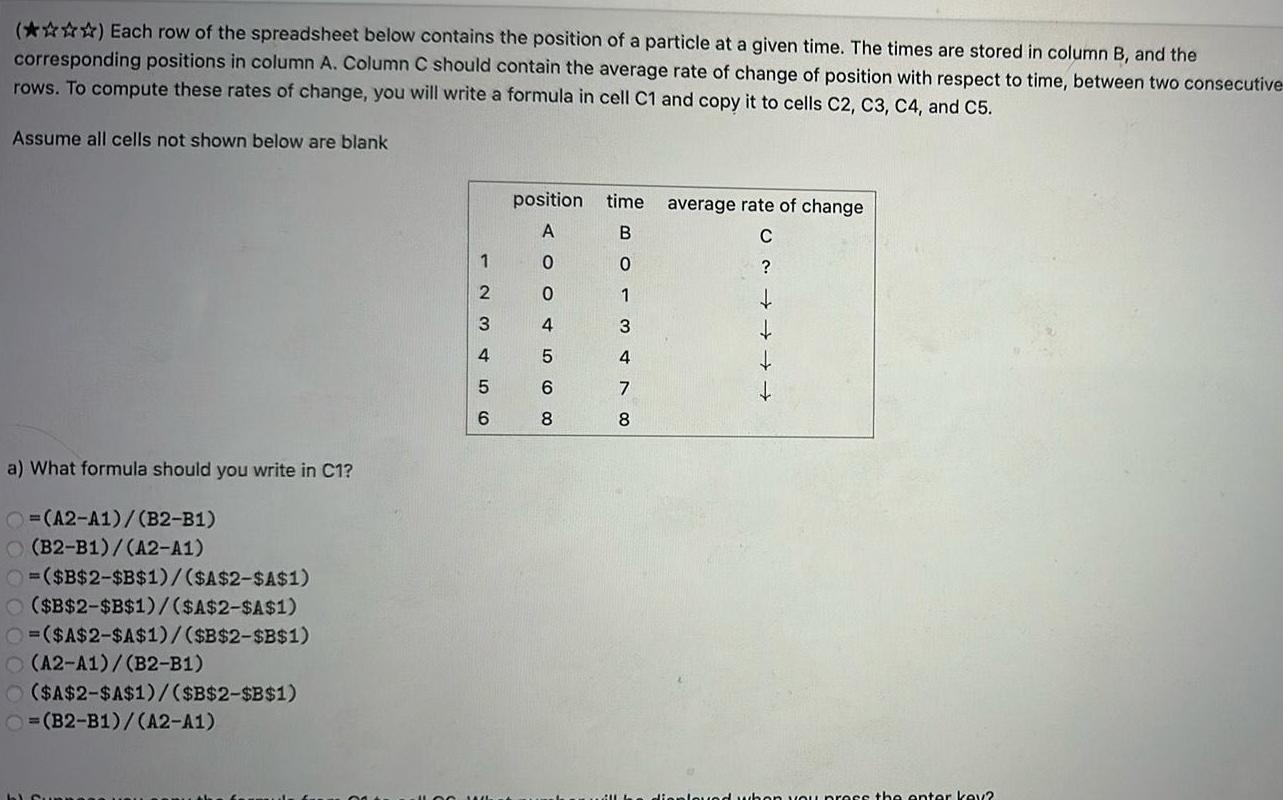
Differential equationsEach row of the spreadsheet below contains the position of a particle at a given time The times are stored in column B and the corresponding positions in column A Column C should contain the average rate of change of position with respect to time between two consecutive rows To compute these rates of change you will write a formula in cell C1 and copy it to cells C2 C3 C4 and C5 Assume all cells not shown below are blank a What formula should you write in C1 O A2 A1 B2 B1 B2 B1 A2 A1 B 2 B 1 A 2 A 1 B 2 B 1 A 2 A 1 O A 2 A 1 B 2 B 1 A2 A1 B2 B1 A 2 A 1 B 2 B 1 O B2 B1 A2 A1 123456 6 position A004568 time B 0 1 3 4 7 8 average rate of change C diceloved when you press the enter key

Calculus
Differential equations2 Give three examples of Ordinary Differential Equations of second order with constant coefficients one example for each case not solved in the lectures and solve these equations 25 points 3 Test for exactness If exact solve If not find an integrating factor and solve Find the general and particular solution 4 y m dx 5 x n dy 0 with initial condition y m 1 n where m and n are last two digits of your student ID number 4 Find the eigenvalues and eigenvectors for the matrix 353 46 0 25 points 25 points
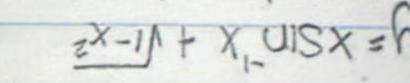
Calculus
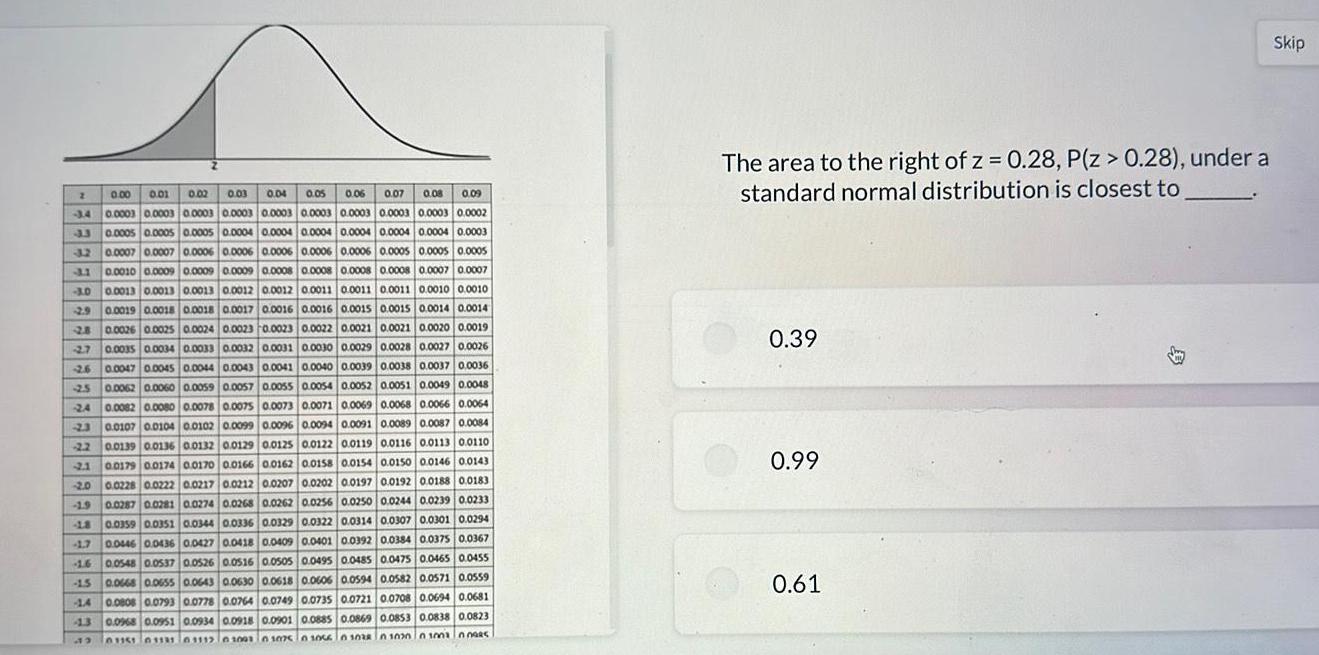
Differential equations2 0 00 0 01 0 02 0 03 0 04 0 05 0 06 0 07 0 08 0 09 3 4 0 0003 0 0003 0 0003 0 0003 0 0003 0 0003 0 0003 0 0003 0 0003 0 0002 33 0 0005 0 0005 0 0005 0 0004 0 0004 0 0004 0 0004 0 0004 0 0004 0 0003 32 0 0007 0 0007 0 0006 0 0006 0 0006 0 0006 0 0006 0 0005 0 0005 0 0005 31 0 0010 0 0009 0 0009 0 0009 0 0008 0 0008 0 0008 0 0008 0 0007 0 0007 3 0 0 0013 0 0013 0 0013 0 0012 0 0012 0 0011 0 0011 0 0011 0 0010 0 0010 2 9 0 0019 0 0018 0 0018 0 0017 0 0016 0 0016 0 0015 0 0015 0 0014 0 0014 2 8 0 0026 0 0025 0 0024 0 0023 0 0023 0 0022 0 0021 0 0021 0 0020 0 0019 27 0 0035 0 0034 0 0033 0 0032 0 0031 0 0030 0 0029 0 0028 0 0027 0 0026 26 0 0047 0 0045 0 0044 0 0043 0 0041 0 0040 0 0039 0 0038 0 0037 0 0036 2 5 0 0062 0 0060 0 0059 0 0057 0 0055 0 0054 0 0052 0 0051 0 0049 0 0048 24 0 0082 0 0080 0 0078 0 0075 0 0073 0 0071 0 0069 0 0068 0 0066 0 0064 2 3 0 0107 0 0104 0 0102 0 0099 0 0096 0 0094 0 0091 0 0089 0 0087 0 0084 2 2 0 0139 0 0136 0 0132 0 0129 0 0125 0 0122 0 0119 0 0116 0 0113 0 0110 2 1 0 0179 0 0174 0 0170 0 0166 0 0162 0 0158 0 0154 0 0150 0 0146 0 0143 2 0 0 0228 0 0222 0 0217 0 0212 0 0207 0 0202 0 0197 0 0192 0 0188 0 0183 0 0287 0 0281 0 0274 0 0268 0 0262 0 0256 0 0250 0 0244 0 0239 0 0233 0 0359 0 0351 0 0344 0 0336 0 0329 0 0322 0 0314 0 0307 0 0301 0 0294 0 0446 0 0436 0 0427 0 0418 0 0409 0 0401 0 0392 0 0384 0 0375 0 0367 1 6 0 0548 0 0537 0 0526 0 0516 0 0505 0 0495 0 0485 0 0475 0 0465 0 0455 1 5 0 0668 0 0655 0 0643 0 0630 0 0618 0 0606 0 0594 0 0582 0 0571 0 0559 1 4 0 0008 0 0793 0 0778 0 0764 0 0749 0 0735 0 0721 0 0708 0 0694 0 0681 19 4 8 1 7 13 0 0968 0 0951 0 0934 0 0918 0 0901 0 0885 0 0869 0 0853 0 0838 0 0823 61151 03331 01112 01001 01075 01066 0 1038 0 1030010010095 The area to the right of z 0 28 P Z 0 28 under a standard normal distribution is closest to 0 39 0 99 0 61 Skip
Calculus
Differential equationsMatch the surfaces with the appropriate description horizontal plane non horizo paraboloid hyperbolic paraboloid cone hyperboloid of one sheet or hyperbolon Note Make sure that you can sketch each of these surfaces paying special attent 1 x 2y 3z VENERE hyperbolic paraboloid horizontal plane non horizontal plane circular cylinder parabolic cylinder ellipsoid elliptic paraboloid hyperbolic paraboloid cone hyperboloid one sheet hyperboloid two sheets V V 2 z6x 2y 3 z y 3x V 4 z 3 5 3x 6y2 22z 4 6 6y z 4 7 z 6x 2y 8 y 62 9 y x 2


Calculus
Differential equationsSolve the exponential equation by taking the logarithm of both sides You will need to use a scientific calculator for this problem 5 127 Your answer must be correct to at least 3 decimal places Recall the change of base formula for logarithms log A can be calculated as In A In h

Calculus
Differential equationsThe radius of a spherical balloon is increasing at the rate of 0 7 cm minute How fast is the volume changing when the radius is 7 4 cm The volume is changing at a rate of n minute cm Type an integer or a decimal Round to one decimal place as needed
Calculus
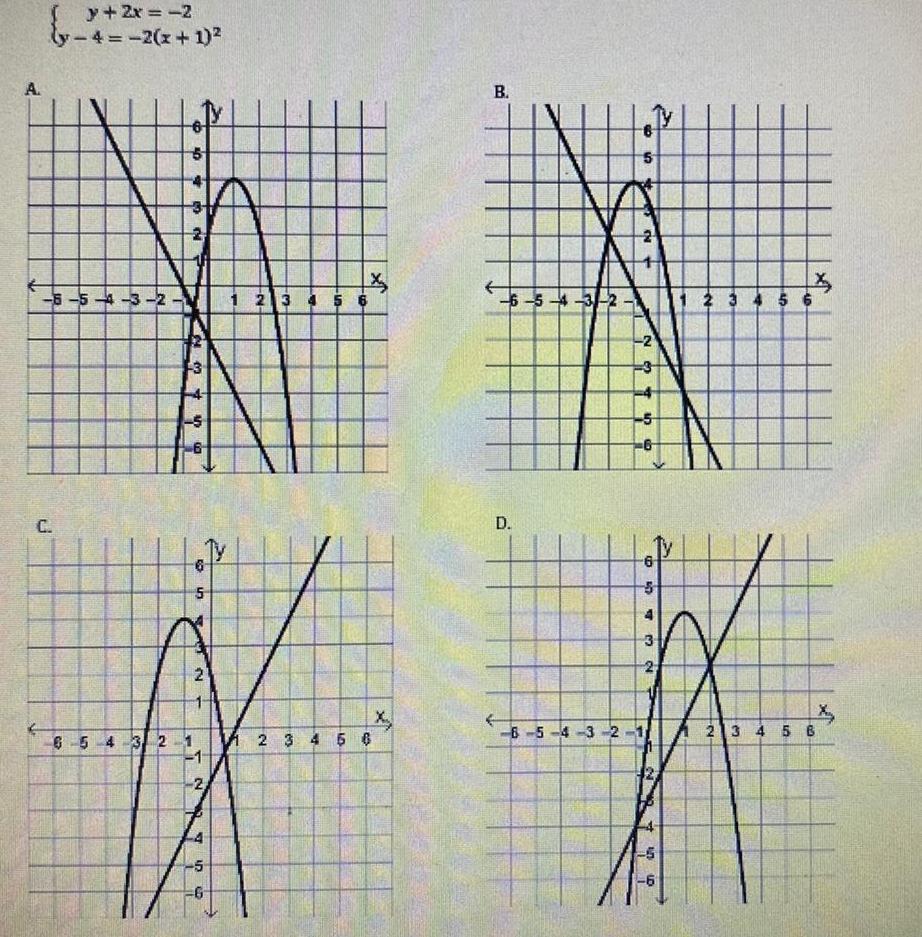
Differential equationsA y 2x 2 ly 4 2 x 1 6 5 4 3 2 C 2 3 4 5 6 B 5 A N 6 5 4 3 2 1 1 2 ST ch 6 x 1 2 3 4 5 6 1 2 3 4 5 6 x X B 6 5 4 3 2 D 5 2 2 19 5 6 6 57 4 6 5 4 3 2 1 12 10 1 2 3 4 5 6 AT LD X 2 3 4 5 6 X
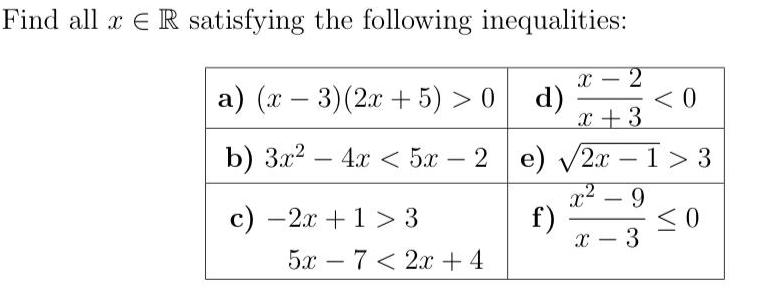
Calculus
Differential equationsFind all x ER satisfying the following inequalities X a x 3 2x 5 0 b 3x 4x 5x2 c 2x 1 3 5x7 2x 4 2 x 3 e 2x 1 3 9 x x 3 0 d f X 0


Calculus
Differential equationsSome biologists model the number of species S in a fixed area A such as an island by the species area relationship log S log c k log A where c and k are positive constants that depend on the type of species and habitat a Solve the equation for S S h Hein
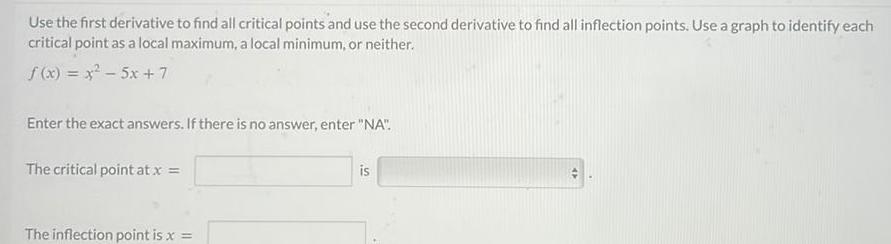
Calculus
Differential equationsUse the first derivative to find all critical points and use the second derivative to find all inflection points Use a graph to identify each critical point as a local maximum a local minimum or neither f x x 5x 7 Enter the exact answers If there is no answer enter NA The critical point at x The inflection point is x is


Calculus
Differential equationsUse the Law of Cosines to solve the triangle Round your answers to two decimal places a 13 b 6 c 18 A B C
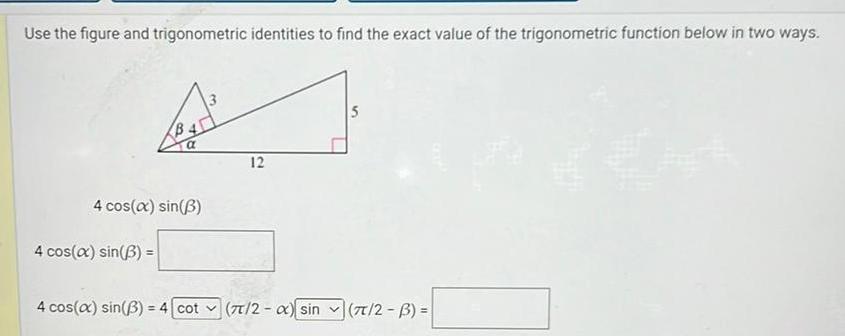
Calculus
Differential equationsUse the figure and trigonometric identities to find the exact value of the trigonometric function below in two ways B4 4 cos x sin 3 4 cos x sin 3 12 4 cos x sin 3 4 cot 7 2 x sin 7 2 B

Calculus
Differential equationsConsider the function f 2 x 42 9 on the interval 0 4 Verify that this function satisfies the three hypotheses of Rolle s Theorem on the inverval f x is on 0 4 answer is a word f x is on 0 4 answer is a word and f 0 f 4 answer is a number Then by Rolle s theorem there exists a e such that f c 0 Find the value c C

Calculus
Differential equationsFind the equation of the line through the point 2 5 that cuts off the least area from the first quadrant
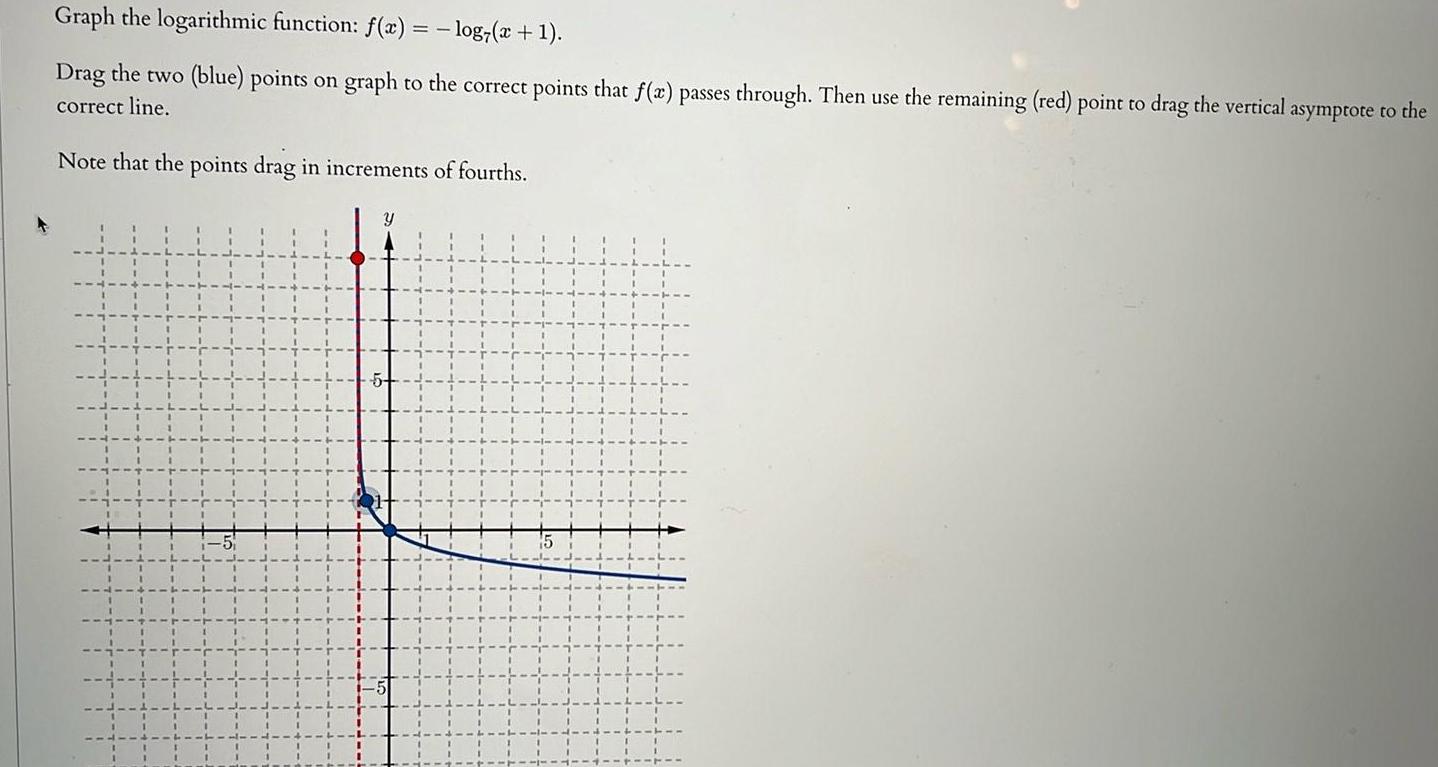
Calculus
Differential equationsGraph the logarithmic function f x log x 1 Drag the two blue points on correct line graph to the correct points that f x passes through Then use the remaining red point to drag the vertical asymptote to the Note that the points drag in increments of fourths Y

Calculus
Differential equationsCondense the left hand side into a single logarithm log 9 log x log y log IED help formulas
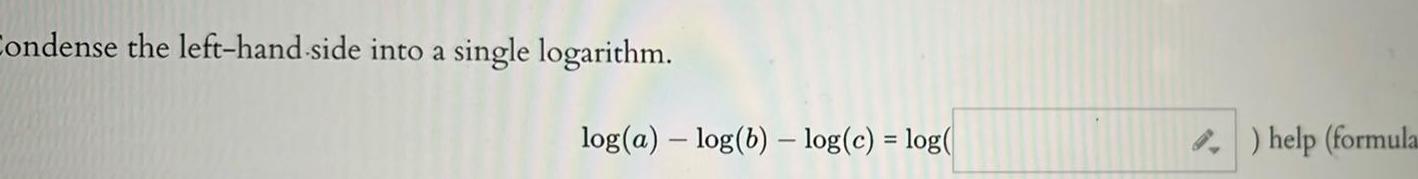
Calculus
Differential equationsCondense the left hand side into a single logarithm log a log b log c log 4 help formula

Calculus
Differential equationsFind the Taylor polynomials of orders 0 1 2 and 3 generated by fat a f x x a 0 P x Simplify your answer P X P X 0 Py x

Calculus
Differential equationsSuppose that x and y are related by the equation 5x 4y 2 and use implicit differentiation to determine dx

Calculus
Differential equationsy t 7 t 1 y 2 In 1 t t 3 y 1 4 Sorry we can t solve this one just yet Determine largest interval astch on which the
Calculus
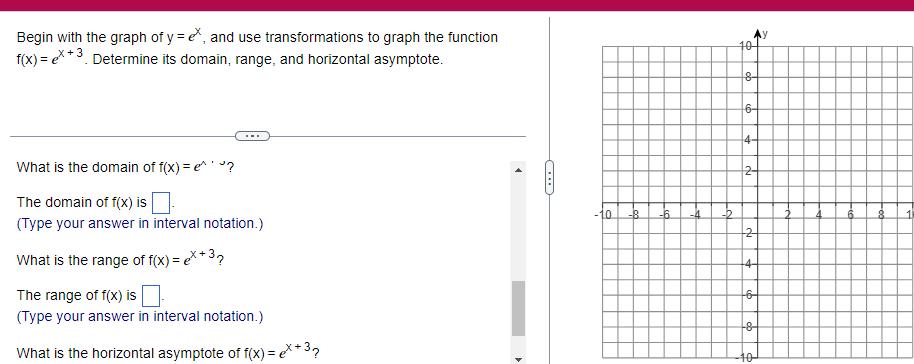
Differential equationsBegin with the graph of y e and use transformations to graph the function f x e Determine its domain range and horizontal asymptote What is the domain of f x e The domain of f x is Type your answer in interval notation What is the range of f x ex 37 The range of f x is Type your answer in interval notation What is the horizontal asymptote of f x x 3 4 10 8 6 4 2 10 8 6 4 2 2 4 2 6 8 40 8 11