Application of derivatives Questions and Answers

Calculus
Application of derivativesNow we must find the radius of convergence for the Maclaurin series First we set up the ratio test as follows lim 816 an 1 an lim 818 n 2 n n 1 4 n 2 x 1 Step 6 Now we can simplify and evaluate the limit Ixl lim x 4 n 1 4 n 1 B n 0 4 n 1 x That is the positive number R such that the series converges if Ix al R and diverges of La

Calculus
Application of derivativesStep 3 We have found the following values of the function and its derivatives f 0 4 f 0 8 f 0 24 f 0 96 4 0 480 Therefore the Maclaurin series begins as f x f 0 f 0 x f 0 2 f 0 x3 4 0 x4 3 4 X x ARK

Calculus
Application of derivatives480 1 x 6 Step 2 We have found the following derivatives of f x f x 4 1 x f x 8 1 x f x 24 1 x 4 96 1 x 5 4 x 480 1 x 6 The Maclaurin series uses the function and its derivatives evaluated at 0 which we find as follow f 0 f 0 F 0 f 0 4 0 480 1 x 6 f x Submit Skip you cannot come back

Calculus
Application of derivativesIf f x b x 4 for all x write a formula for b n 0 O by O b O b Ob by O by f 7 7 4 f 4 4 f 4 7 f 7 4 7 f 4 4 7

Calculus
Application of derivatives6 sin x 5 sin x 1 0 0 2 X Select 4 correct answer s 1 30 3 31 0 T 4 5T 4 2 3 5 94 5


Calculus
Application of derivatives2 cos x 3 cos x 1 0 0 2 X Select 3 correct answer s 0 5 3 3 2 5 6 11

Calculus
Application of derivativestan x 3 X Select 2 correct answer s HO 130 TU 57 6 11 6 3 0 2 No solution 5 3


Calculus
Application of derivativesProblems 4 7 Simplify each rational expression If the rational expression cannot be simplified so state 4 5 7 48y5 15y3 4a 9b 2a 3b 3x 1 3x 29x 10 3 x 5r 15

Calculus
Application of derivativesIgnoring the constant of integration integrating the right side gives Part 4 of 5 We now have u t tan t C Since u 0 9 we can substitute into the equation and solve for C Submit Skip you cannot come back sec t

Calculus
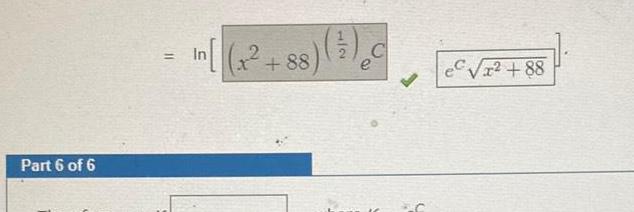
Application of derivativesWe now integrate each side of the differential equation Ignoring the constant of integration we can integrate the left hand side of the equation to obtain integral 10 dy
Calculus
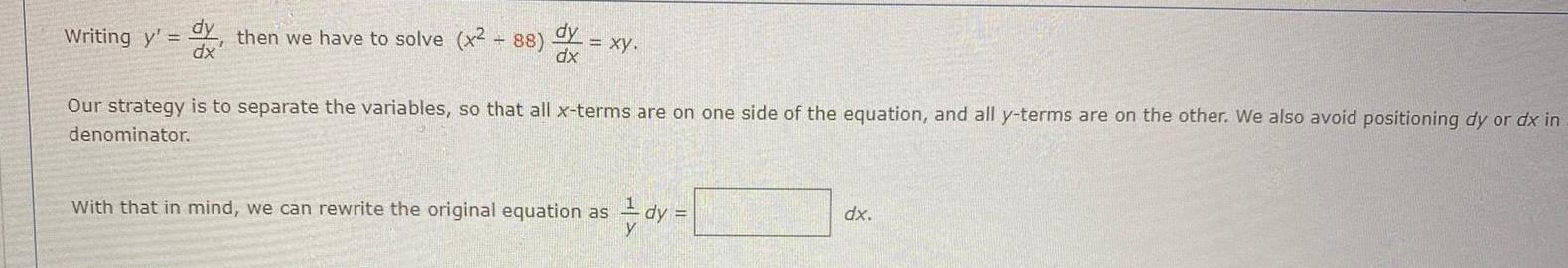
Application of derivativesWriting y dy then we have to solve x 88 dx xy dx Our strategy is to separate the variables so that all x terms are on one side of the equation and all y terms are on the other We also avoid positioning dy or dx in denominator With that in mind we can rewrite the original equation as dy dx
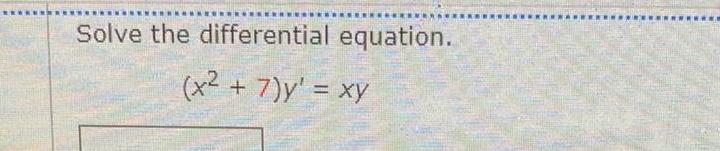

Calculus
Application of derivatives8 pts 5 For the functions below sketch the graph of the function labeling the axes Use the graph to determine whether f x has an inverse that is also a function Give a specific reason for your answer If the function has an inverse that s also a function find a formula for the inverse function f x showing all work on how your reached your answer a f x 6 2x x 4 5
Calculus
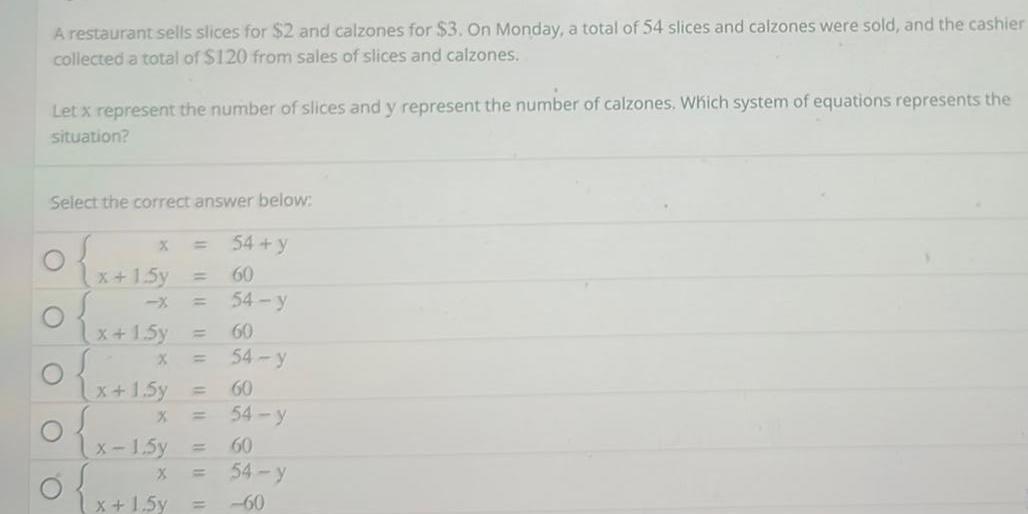
Application of derivativesA restaurant sells slices for 2 and calzones for 3 On Monday a total of 54 slices and calzones were sold and the cashier collected a total of 120 from sales of slices and calzones Let x represent the number of slices and y represent the number of calzones Which system of equations represents the situation Select the correct answer below 54 y 60 54 y 60 54 y 60 54 y 60 54 y 60 O O O O x 1 5y x 1 5y X x 1 5y 1111 x 1 5y x 1 5y X
Calculus
Application of derivativesThe costs of a company are represented by the function C x 0 22x 1 750 Its revenues are represented by function R x 4 1x Find the function that represents the company s profits Provide your answer below

Calculus
Application of derivativessolution to the following system of equations by graphing 2x y x y Drag the movable points to graph each of the linear equations The plot automatically calculates and shows the coordinates of the intersection of the two lines Provide your answer below 10 5 10 5 0 2 0 t 4 2 5 10 4

Calculus
Application of derivativesThe graph of a function is given Graph the inverse function 7 6 5 44 3 2 6 5 4 3 2 1 2 3 S 4 6
Calculus
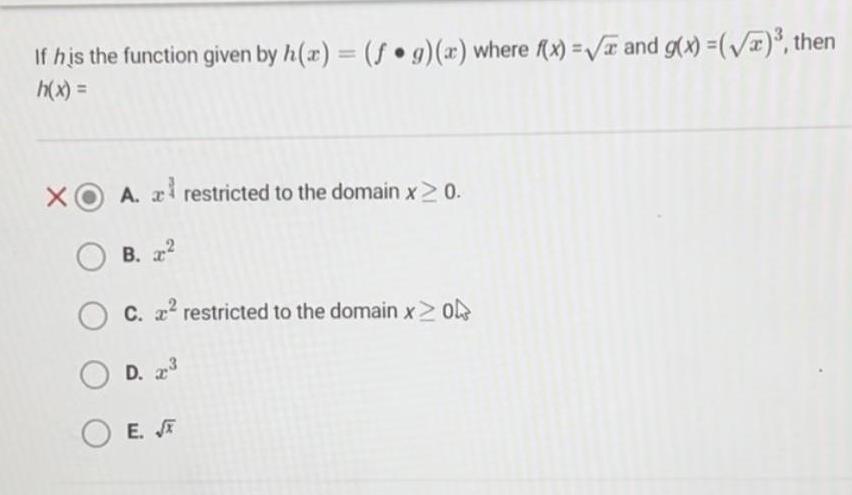
Application of derivativesIf his the function given by h x f g x where f x and g x then h x O A a restricted to the domain x 0 OB OC 2 restricted to the domain x 0 OD 23 OE J

Calculus
Application of derivativesSuppose the following about a certain object 1 The object s altitude is a function of time represented by the function A x where here x is time II The temperature of the object is a function of its velocity represented by the function T x where here x is the object s velocity III The weight of the object is a function of its altitude represented by the function x where here x is the object s altitude IV The velocity of the object is a function of its weight represented by the function V x where here x is the object s weight The function F 1 which gives us the object s temperature at time t can be represented as A F t A o WoVoT t XO B F t TO Vo AoW t OC F t To Wo VoA t F t To VoWo A t OE F t To Wo AoV t

Calculus
Application of derivatives1 sin x on the domain the domain of g is If g x OA O B 4 4 C all real numbers OD 1 1 O E 0 1
Calculus
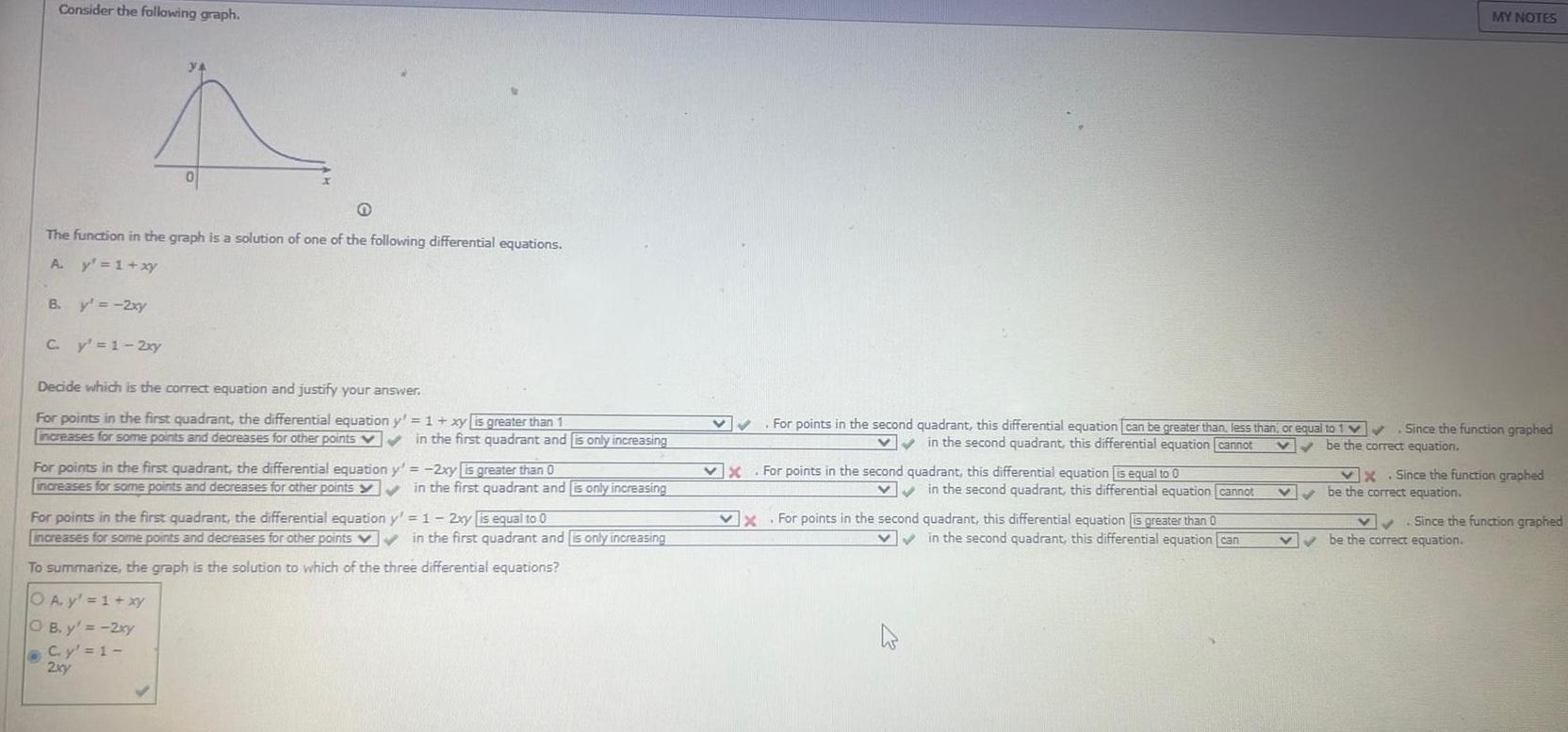
Application of derivativesConsider the following graph Q The function in the graph is a solution of one of the following differential equations A y 1 xy B y 2xy O 0 C y 1 2xy Decide which is the correct equation and justify your answer For points in the first quadrant the differential equation y 1 xy is greater than 1 increases for some points and decreases for other points v in the first quadrant and is only increasing For points in the first quadrant the differential equation increases for some points and decreases for other points y 2xy is greater than 0 in the first quadrant and is only increasing For points in the first quadrant the differential equation y 1 2xy is equal to 0 increases for some points and decreases for other points in the first quadrant and is only increasing To summarize the graph is the solution to which of the three differential equations OA y 1 xy OB y 2xy C y 1 2xy For points in the second quadrant this differential equation can be greater than less than or equal to 1 Since the function graphed in the second quadrant this differential equation cannot V be the correct equation X For points in the second quadrant this differential equation is equal to 0 in the second quadrant this differential equation cannot X For points in the second quadrant this differential equation is greater than 0 x in the second quadrant this differential equation can MY NOTES v X Since the function graphed be the correct equation Since the function graphed be the correct equation

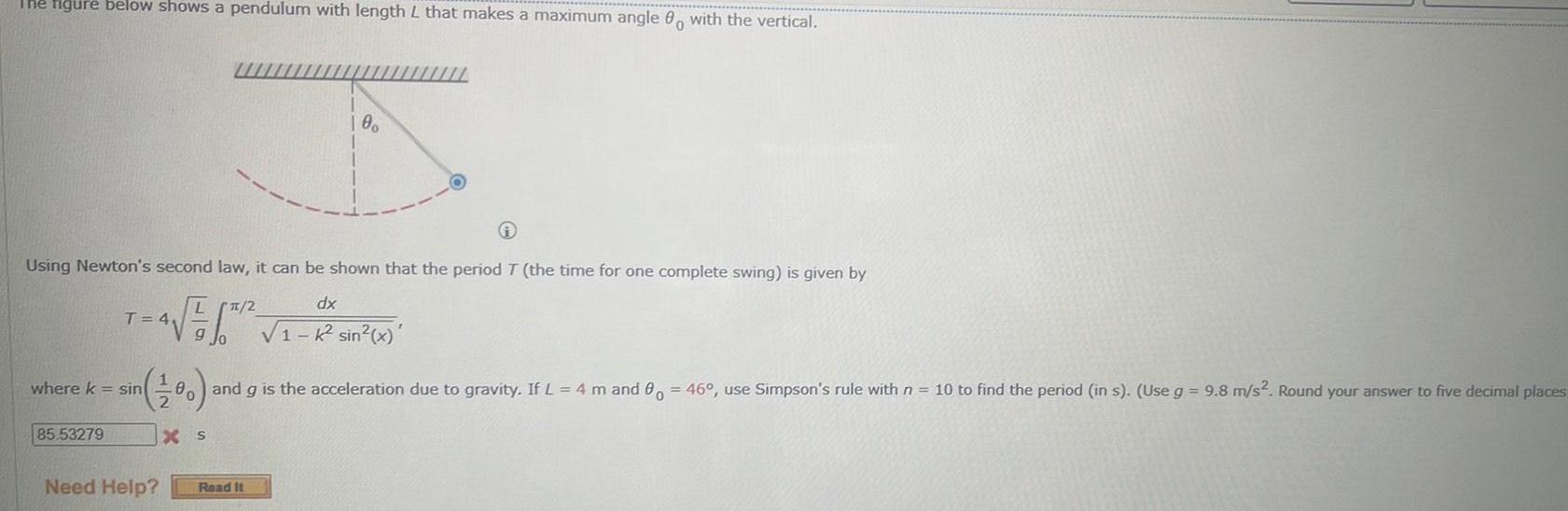
Calculus
Application of derivativesThe figure below shows a pendulum with length L that makes a maximum angle 00 with the vertical Using Newton s second law it can be shown that the period T the time for one complete swing is given by LT 2 dx T 4 4 K sin x where k sin 100 and g is the acceleration due to gravity If L 4 m and 0 46 use Simpson s rule with n 10 to find the period in s Use g 9 8 m s Round your answer to five decimal places XS 85 53279 100 Need Help Read It


Calculus
Application of derivativesFind the exact length of the curve v 2x 2 0 x 1 3 2 2 1 y X Enhanced Feedback Please try again Remember that the arc length formula for the curve v Fly r ve his

Calculus
Application of derivativesHalo blight is a highly contagious fungus that can spread in some farm crops Five hours after the blight was found 0 46 acres of the field is infected 12 hours later so a total of 17 hours after the blight was found the number of acres infected has grown to 30 7 acres Find the rate at which the blight spreads as a decimal rounded to two decimal places A r Find the number of acres infected when the blight is first identified A Acres The field Is a total of 160 acres Estimate the time for the field to be entirely infected round to the nearest hour

Calculus
Application of derivativesWhat area of the brain is responsible for precisely starting stopping or coordinating movements O brain stem motor areas O precommand areas O motor cortex of the cerebrum spinal level Sen

Calculus
Application of derivativesProblem Design the optimal conical container having a cover which hold 0 2m of oil a Find the dimensions r and h of the can that will minimize the cost of the metal to manufacture the container Answers r 0 4070m Height 1 15266m Volume 0 1999m Total area 2 08m b Repeat but without a cover Answers r m h m c Using calculus verify if it is the same as per Excel solver Your excel sheet should have the following format A 1 2 3 4 5 6 7 Radius Height Volume Desired Volume Top area Side area Total area B 2

Calculus
Application of derivativesUsing the minimum payment balance formula You have a credit card with an APR of 24 The card requires a minimum monthly payment of 5 of the balance You have a balance of 5500 You stop charging and make only the minimum monthl payment What is the balance on the card after three years Enter your answer rounded to the nearest cent

Calculus
Application of derivativesWrite the equation of a sinusoidal graph with the following properties 2 A 3 period 3 flipped over y axis right phase shift TC 4

Calculus
Application of derivativesThe current 1 in amperes flowing through a AC alternatice current circuit at time t is 1 220sin 30rt I 6 120 a What is the period b What is the amplitude c What is the phase shift inluding direction

Calculus
Application of derivativestion 17 Outside temperature over a day can be modeled as a sinusoidal function Suppose you know the temperature is 50 degrees at midnight and the high and low temperature during the day are 57 and 43 degrees respectively Assuming is the number of hours since midnight find an equation for the temperature T in terms of t Work does not need to be shown but provide an explanation in the answer box for full credit

Calculus
Application of derivativesUse the following figure to answer the questions 13 B 54 a 15 C a Solve the triangle b State the area of the triangle

Calculus
Application of derivativesProve the following identity is true 1 cos x cos x sin x 1 tan x full points show your work on yo

Calculus
Application of derivativesThe height in feet of the tide t hours after midnight is modeled in the function in the graph h M 12 20 10 10 20 18 a Is the tide rising or falling at 1 00 AM Be sure to explain b At what time does low tide occur c What is the amplitude of the function and give a practical interpretation of your answer funct and give a practical interpretation of your answer

Calculus
Application of derivatives1 Solve the following equations round to 2 decimal places where appropriate a 3 12 b 10 2 4x 2

Calculus
Application of derivativesFind c if a 2 51 mi b 3 51 mi and ZC 40 2 degrees CROBRED mi Assume ZA is opposite side a LB is opposite side b and ZC is opposite side c


Calculus
Application of derivativesFor the following vector convert from magnitude and direction to x and y coordinates r 3 0 20 X y



Calculus
Application of derivativesThe table below gives the number of calories for each type of candy in a certain box These are the only types of candy in the box Type of candy Number of calories Caramel 300 Peppermint 150 Cinnamon 250 Chocolate 250 200 Butterscotch A conditional statement is given below Give the converse contrapositive and inverse of the statement Also use the table above to decide if each statement is true or false Assume that any candy mentioned comes from the box Given statement Converse Contrapositive Inverse If a candy is chocolate then the candy has 250 calories True If Choose one then Choose one If Choose one then Choose one If Choose one then Choose one True O False O True False O True False O False X

Calculus
Application of derivativesDetermine a general formula or formulas for the solution to the following equation Then determine the specific solutions if any on the interval 0 2 sin 0 cos 0 2 Describe in the most concise way possible the general solution to the given equation where k is any integer Select the correct choice below and fill in all the answer boxes within your choice Simplify your answers Type any angle measures in radians Use the smallest non negative angle possible when describing the general form of each angle Use ascending order entering the expression based on the smallest angle first Type exact answers using as needed Use integers or fractions for any numbers in the expressions OA 0 OB 0 O c 0 O D 0 k k or 0 k or 0 k or 0 E There is no solution k k or 0 k k or 0 k or 0 K k

Calculus
Application of derivatives6 Anle Corporation has a current stock price of 16 58 and is expected to pay a dividend of 1 20 in one year Its expected stock price right after paying that dividend is 18 81 a Anle s equity cost of capital is Round to two decimal places b The portion of Anle s equity cost of capital that is expected to be satisfied by the dividend yield is Round to two decimal places The portion of Anle s equity cost of capital that is expected to be satisfied by capital gains is

Calculus
Application of derivatives4 How can the concept of families of polynomials help us analyze and compare different type of functions

Calculus
Application of derivatives1 Perform long division Express the answer in quotient form and write the statement that could be used to check the division x 9x 5x 3 divided by x 2