When it comes to geometry, trapezoids are an important concept to understand. They are commonly encountered in real-life situations, such as when you see a wheelbarrow in a garden or pass over a bridge and observe its trusses. Trapezoids are particularly relevant in fields like architecture and construction.
In this article, we will explore the area of a trapezoid and learn how to calculate it. We will discuss the definition of a trapezoid, the formula to find its area, and provide step-by-step examples to illustrate the concept.
What is a Trapezoid?
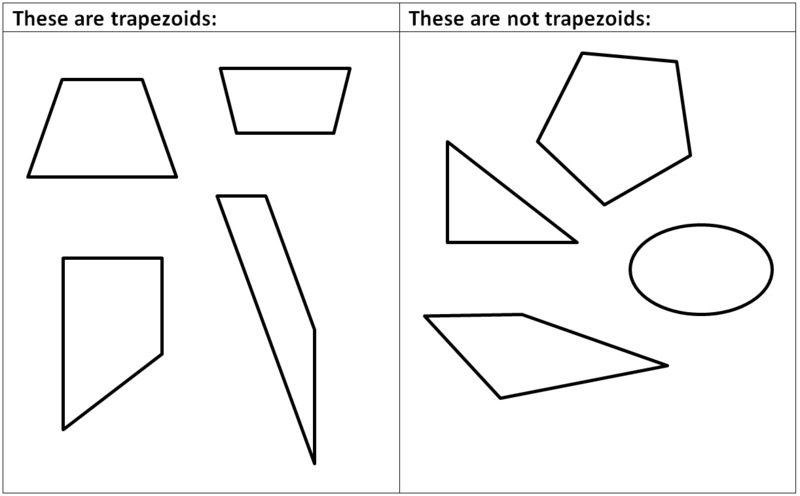
Before we dive into calculating the area of a trapezoid, let’s first understand what a trapezoid is. A trapezoid is a quadrilateral, which means it is a four-sided plane figure. One defining characteristic of a trapezoid is that it has exactly one pair of parallel sides. These parallel sides are referred to as the bases of the trapezoid. The other two sides, which are not parallel, are known as the legs or lateral sides of the trapezoid.
It’s worth noting that the term “trapezoid” is used differently in different regions. In some countries, a trapezoid is defined as a quadrilateral with at least one pair of parallel sides, while in others, it refers to a quadrilateral with exactly one pair of parallel sides. In the latter case, what is called a trapezoid in some regions is referred to as a trapezium in others.
What is the Area of a Trapezoid?
The area of a trapezoid is the measure of the space enclosed within its boundaries in a two-dimensional plane. It is expressed in square units such as square centimeters (cm²), square meters (m²), square inches (in²), or square feet (ft²). To calculate the area of a trapezoid, we use a specific formula that takes into account the lengths of the bases and the height of the trapezoid.

Area of Trapezoid Formulas
To calculate the area of a trapezoid, we use the following formula:
Area = ½ * height * (base1 + base2)
In this formula, the height refers to the perpendicular distance between the two bases of the trapezoid. The bases are the parallel sides of the trapezoid, and base1 and base2 represent their respective lengths.
It’s important to note that the height of the trapezoid must be a perpendicular distance. If the height is not perpendicular to the bases, you will need to find the perpendicular height by drawing a line from one base to the other.
How to Find the Area of a Trapezoid?
To find the area of a trapezoid, follow these steps:
Step 1: Identify the lengths of the bases and the height of the trapezoid.
Step 2: Substitute the values of base1, base2, and height into the area formula: Area = ½ * height * (base1 + base2).
Step 3: Calculate the product of the height and the sum of the bases.
Step 4: Divide the result by 2 to find the area of the trapezoid.
Let’s work through an example to illustrate the process.
Example: Consider a trapezoid with a base1 length of 10 cm, a base2 length of 15 cm, and a height of 8 cm. Find the area of the trapezoid.
Solution: Using the formula for the area of a trapezoid, we substitute the given values:
Area = ½ * 8 * (10 + 15)
= ½ * 8 * 25
= 4 * 25
= 100 cm²
Therefore, the area of the trapezoid is 100 cm².
Area of Trapezoid Without Known Height
In some cases, you may be given a trapezoid with the lengths of all its bases and legs, but the height is not provided. In such situations, you need to calculate the height of the trapezoid first before finding its area.
To find the height of a trapezoid, follow these steps:
Step 1: Divide the trapezoid into two right triangles by drawing a diagonal from one of the upper vertices to the opposite lower base.
Step 2: Use the Pythagorean theorem to calculate the length of the height.
Step 3: Once you have the height, proceed to calculate the area of the trapezoid using the formula: Area = ½ * height * (base1 + base2).
Let’s work through an example to demonstrate the process.
Example: Consider a trapezoid with base lengths of 10 cm and 18 cm, and leg lengths of 6 cm each. Find the area of the trapezoid.
Solution: To find the height of the trapezoid, we divide it into two right triangles.
Triangle 1: Base = 18 - 10 = 8 cm, Leg = 6 cm
Triangle 2: Base = 18 - 10 = 8 cm, Leg = 6 cm
Now, we can use the Pythagorean theorem to find the height.
Height = √(Leg^2 - Base^2)
= √(6^2 - 4^2)
= √(36 - 16)
= √20
≈ 4.47 cm
With the height calculated, we can now find the area of the trapezoid.
Area = ½ * 4.47 * (10 + 18)
= ½ * 4.47 * 28
≈ 62.6 cm²
Therefore, the area of the trapezoid is approximately 62.6 cm².
Area of a Trapezoid When Given Diagonals
In some cases, you may be given the lengths of the diagonals of a trapezoid and the angle between them, and you need to calculate the area of the trapezoid. To do this, you can use the formula for the area of a trapezoid and apply trigonometric principles.
Let’s consider a trapezoid with diagonals of lengths d1 and d2 and an angle α between them. To find the area of this trapezoid, follow these steps:
Step 1: Use the formula for the area of a trapezoid: Area = ½ * height * (base1 + base2).
Step 2: Calculate the height of the trapezoid using the lengths of the diagonals and the angle between them.
Step 3: Substitute the values of base1, base2, and height into the area formula.
Step 4: Calculate the area of the trapezoid.
Let’s work through an example to illustrate this process.
Example: Consider a trapezoid with diagonals of lengths 10 cm and 14 cm, and an angle of 60 degrees between them. Find the area of the trapezoid.
Solution: To find the area of the trapezoid, we first need to calculate the height. We can use the formulas for the diagonals and the angle between them to find the height.
Height = √(d1^2 - (d2 * cos(α))^2)
= √(10^2 - (14 * cos(60))^2)
= √(100 - (14 * 0.5)^2)
= √(100 - 49)
= √51
≈ 7.14 cm
Now that we have the height, we can substitute the values into the area formula.
Area = ½ * 7.14 * (10 + 14)
= ½ * 7.14 * 24
≈ 85.68 cm²
Therefore, the area of the trapezoid is approximately 85.68 cm².
Area of Different Types of Trapezoids
Trapezoids come in various forms, each with its own unique properties. Let’s take a look at some common types of trapezoids and how to calculate their areas.
Isosceles Trapezoid
An isosceles trapezoid is a trapezoid with two congruent legs. In other words, the non-parallel sides of an isosceles trapezoid have the same length. To find the area of an isosceles trapezoid, follow the same steps as mentioned earlier:
- Identify the lengths of the bases and the height of the trapezoid.
- Substitute the values into the area formula: Area = ½ * height * (base1 + base2).
- Calculate the product of the height and the sum of the bases.
- Divide the result by 2 to find the area of the trapezoid.
Right Trapezoid
A right trapezoid is a trapezoid with one right angle. To find the area of a right trapezoid, follow the same steps as mentioned earlier:
- Identify the lengths of the bases and the height of the trapezoid.
- Substitute the values into the area formula: Area = ½ * height * (base1 + base2).
- Calculate the product of the height and the sum of the bases.
- Divide the result by 2 to find the area of the trapezoid.
Scalene Trapezoid
A scalene trapezoid is a trapezoid with no congruent sides. To find the area of a scalene trapezoid, follow the same steps as mentioned earlier:
- Identify the lengths of the bases and the height of the trapezoid.
- Substitute the values into the area formula: Area = ½ * height * (base1 + base2).
- Calculate the product of the height and the sum of the bases.
- Divide the result by 2 to find the area of the trapezoid.
How to Derive Area of Trapezoid Formula?
The formula for the area of a trapezoid can be derived by dividing the trapezoid into two triangles and summing their areas. Let’s go through the derivation step-by-step:
- Start with a trapezoid with bases a and b, and height h.
- Divide the trapezoid into two right triangles by drawing a diagonal from one vertex to the opposite base.
- The two right triangles have bases a and b, and heights h and h.
- The area of each right triangle is ½ * base * height.
- Sum the areas of the two right triangles to get the area of the trapezoid: ½ * a * h + ½ * b * h.
- Combine like terms: ½ * (a + b) * h.
- Simplify the expression to obtain the final formula for the area of a trapezoid: (a + b) * h / 2.
This derivation shows that the area of a trapezoid is equal to the average of the lengths of the bases multiplied by the height.
Properties of Trapezoid
In addition to understanding how to calculate the area of a trapezoid, it’s useful to be familiar with some of its properties. These properties can help you identify trapezoids and understand their characteristics. Here are a few important properties of trapezoids:
- Trapezoids have one pair of parallel sides: This is the defining characteristic of a trapezoid. The parallel sides are referred to as the bases of the trapezoid, while the non-parallel sides are known as the legs.
- The sum of the interior angles of a trapezoid is 360 degrees: Just like any other quadrilateral, the sum of the interior angles of a trapezoid is always 360 degrees.
- The diagonals of a trapezoid intersect at a point: The point of intersection of the diagonals is called the intersection point. The diagonals of a trapezoid divide it into four triangles.
- The mid-segment of a trapezoid is parallel to the bases: The mid-segment is a line segment connecting the midpoints of the legs of a trapezoid. It is always parallel to the bases and half their length.
- The height of a trapezoid is the perpendicular distance between the bases: The height is a line segment perpendicular to the bases of the trapezoid. It connects the bases at their midpoints.
Understanding these properties can help you identify and analyze trapezoids in various geometric scenarios.
Perimeter of Trapezoid
While the focus of this article is on the area of a trapezoid, it’s worth briefly discussing the perimeter of a trapezoid as well. The perimeter of a trapezoid is the sum of the lengths of all its sides.
To calculate the perimeter of a trapezoid, add the lengths of the four sides together. The formula for the perimeter of a trapezoid can be expressed as:
Perimeter = base1 + base2 + leg1 + leg2
It’s important to note that the bases and legs of a trapezoid may have different lengths, so it’s necessary to consider each side individually when calculating the perimeter.
Solved Examples on Area of Trapezoid
Let’s work through a few additional examples to solidify our understanding of calculating the area of a trapezoid.
Example 1: Consider a trapezoid with a base1 length of 8 cm, a base2 length of 12 cm, and a height of 6 cm. Find the area of the trapezoid.
Solution: Using the formula for the area of a trapezoid, we substitute the given values:
Area = ½ * 6 * (8 + 12)
= ½ * 6 * 20
= 3 * 20
= 60 cm²
Therefore, the area of the trapezoid is 60 cm².
Example 2: Consider a trapezoid with a base1 length of 5 inches, a base2 length of 9 inches, and a height of 7 inches. Find the area of the trapezoid.
Solution: Using the formula for the area of a trapezoid, we substitute the given values:
Area = ½ * 7 * (5 + 9)
= ½ * 7 * 14
= 7 * 7
= 49 inches²
Therefore, the area of the trapezoid is 49 inches².
Example 3: Consider a trapezoid with a base1 length of 12 meters, a base2 length of 16 meters, and a height of 9 meters. Find the area of the trapezoid.
Solution: Using the formula for the area of a trapezoid, we substitute the given values:
Area = ½ * 9 * (12 + 16)
= ½ * 9 * 28
= 4.5 * 28
= 126 meters²
Therefore, the area of the trapezoid is 126 meters².
How Kunduz Can Help You Learn How To Find The Area of Trapezoid?
If you need further assistance in understanding how to find the area of a trapezoid, Kunduz is here to help. Kunduz is an online learning platform that offers comprehensive math courses, including geometry. Our experienced instructors provide step-by-step explanations and practice problems to help you master the concepts.
With Kunduz, you can access high-quality educational resources anytime, anywhere. Whether you’re a student looking to improve your math skills or a teacher seeking additional teaching materials, Kunduz has the tools you need for success.
Visit our website and enroll in our geometry course to begin your journey toward mastering the area of a trapezoid and other fundamental mathematical concepts.
