In the field of geometry, the concept of a centroid holds significant importance, particularly in relation to triangles. A triangle is a three-sided figure with three interior angles. Depending on the lengths of its sides and the measures of its angles, a triangle can be classified into various types such as scalene, isosceles, equilateral, acute-angled, obtuse-angled, and right-angled triangles. The centroid is a key property of a triangle, and understanding its definition, formula, properties, and applications is crucial in geometry.
What is a Centroid?
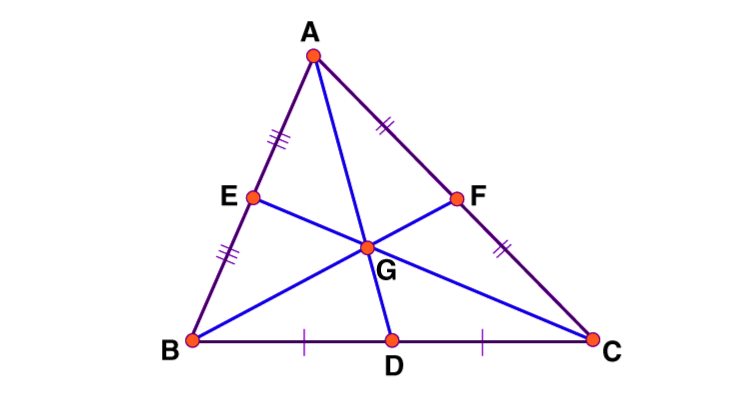
The centroid can be defined as the point where the three medians of a triangle intersect. A median is a line segment that connects the midpoint of a side to the opposite vertex of a triangle. The centroid of a triangle divides each median in a ratio of 2:1. It can be found by taking the average of the x-coordinate and y-coordinate points of all three vertices of the triangle.

Centroid Formula
The formula for finding the centroid of a triangle is as follows: Centroid of a triangle = ((x1 + x2 + x3)/3, (y1 + y2 + y3)/3)
In this formula, (x1, y1), (x2, y2), and (x3, y3) represent the coordinates of the three vertices of the triangle. By substituting these values into the formula, we can determine the centroid of the triangle.
Derivation of Centroid Formula
To derive the centroid formula, we consider a triangle with vertices A, B, and C. Let (x1, y1), (x2, y2), and (x3, y3) be the coordinates of these vertices, respectively. The midpoint of the side BC is D, the midpoint of AB is E, and the midpoint of AC is F. The coordinates of these midpoints can be calculated using the midpoint formula.
Using the coordinates of these midpoints, we can determine the coordinates of the centroid G. The x-coordinate of G is the average of the x-coordinates of D, E, and F, and the y-coordinate of G is the average of the y-coordinates of D, E, and F. Simplifying this expression gives us the centroid formula.
Centroid Theorem and Proof
The centroid theorem states that the centroid of a triangle is located at two-thirds of the distance from each vertex to the midpoint of the opposite side. In other words, if PQR is a triangle with centroid V, and S, T, and U are the midpoints of the sides PQ, QR, and PR, respectively, then according to the centroid theorem:
- QV = (2/3)QU
- PV = (2/3)PT
- RV = (2/3)RS
To prove the centroid theorem, we can use vector notation and the properties of medians. By representing the vertices of the triangle as vectors, we can express the medians in terms of these vectors and show that they intersect at a point that divides each median in a 2:1 ratio.
Properties of Centroid
The centroid of a triangle has several important properties:
- It is the center of the object and serves as the center of gravity.
- The centroid always lies inside the object and is the point of concurrency of the medians.
- The centroid divides each median in a ratio of 2:1.
These properties make the centroid a significant point in geometry, particularly in the study of triangles.
Centroid of a Triangle
The centroid of a triangle is the point where all three medians intersect. As mentioned earlier, a median is a line segment that connects the midpoint of a side to the opposite vertex. The centroid of a triangle divides each median into segments that are in a ratio of 2:1. This means that the distance from the centroid to the vertex is twice the distance from the centroid to the midpoint of the side.
Centroid of a Square
In a square, the centroid is the point where the diagonals intersect. Since all sides of a square are equal, it is easy to locate the centroid. The centroid divides each diagonal into segments that are in a ratio of 2:1.

Centroid of a Trapezoid
The centroid of a trapezoid is the point where the diagonals intersect. A trapezoid is a quadrilateral with one pair of parallel sides. The centroid divides each diagonal into segments that are in a ratio of 2:1.

Centroid of a Circle
In a circle, the centroid is the center of the circle. Since all points on the circumference of a circle are equidistant from the center, the centroid coincides with the center of the circle.

Centroid Formula for Different Shapes
The centroid formula can be used to find the centroid of various geometric shapes. Here is a table that provides the centroid formulas for different shapes:
| Shapes | Figure | x̄ | ȳ | Area |
| Triangular area |  | – | h/3 | bh/2 |
| Quarter-circular area |  | 4r/3π | 4r/3π | πr2/4 |
| Semicircular area |  | 0 | 4r/3π | πr2/2 |
| Quarter-elliptical area |  | 4a/3π | 4b/3π | πab/4 |
| Semi elliptical area |  | 0 | 4b/3π | πab/2 |
| Semiparabolic area |  | 3a/8 | 3h/5 | 2ah/3 |
| Parabolic area |  | 0 | 3h/5 | 4ah/3 |
| Parabolic spandrel |  | 3a/4 | 3h/10 | ah/ |
These formulas can be used to find the centroid of shapes with different areas and dimensions.
Difference between Orthocentre and Centroid
Both orthocenter and centroid are significant points in a triangle, but they hold different properties. Here is a comparison between the two:
| Orthocenter | Centroid |
|---|---|
| It is the point of intersection of the altitudes of a triangle. | It is the point of intersection of all the three medians of a triangle. |
| The orthocenter of a triangle may lie inside or outside of a triangle. | The centroid of a triangle always lies inside the triangle. |
| The orthocenter does not divide the altitudes of a triangle in any particular ratio. | The centroid of a triangle divides the medians of the triangle in the ratio 2 : 1. |
Difference between Centroid and Incenter
The incentre and centroid are two important points in a triangle. Here is a comparison table for better understanding:
| Centroid | Incentre |
|---|---|
| It is the point of intersection of all the three medians of a triangle. | The incentre is the point of intersection of the angle bisectors of a triangle. |
| The centroid of a triangle always lies inside the triangle. | The incentre of a triangle always lies inside the triangle. |
| The centroid of a triangle divides the medians of the triangle in the ratio 2 : 1. | The incentre does not divide the angle bisectors of a triangle in any particular ratio. |
How to Find the Centroid?
To find the centroid of a triangle, you can use the centroid formula mentioned earlier. Simply substitute the coordinates of the triangle’s vertices into the formula to calculate the coordinates of the centroid. This method can be applied to any triangle, regardless of its type or dimensions.
Solved Examples on Centroid
Let’s solve a few examples to further illustrate the concept of the centroid. These examples will provide step-by-step solutions and demonstrate how to apply the centroid formula.
Example 1: Find the centroid of the triangle with vertices A(2, 6), B(4, 9), and C(6, 15).
Solution: We can use the centroid formula to find the centroid of this triangle. By substituting the given coordinates into the formula, we get: Centroid of the triangle = ((2 + 4 + 6)/3, (6 + 9 + 15)/3) = (12/3, 30/3) = (4, 10)
Therefore, the centroid of the triangle with vertices A(2, 6), B(4, 9), and C(6, 15) is (4, 10).
Example 2: Find the centroid of the triangle with vertices A(1, 5), B(2, 6), and C(4, 10).
Solution: Using the centroid formula, we can calculate the centroid of this triangle as follows: Centroid of the triangle = ((1 + 2 + 4)/3, (5 + 6 + 10)/3) = (7/3, 21/3) = (7/3, 7)
Hence, the centroid of the triangle with vertices A(1, 5), B(2, 6), and C(4, 10) is (7/3, 7).
Example 3: If the centroid of a triangle is located at (3, 3), and the vertices of the triangle are (2, 3), (-2, 7), and (k, 4), find the value of k.
Solution: To find the value of k, we need to substitute the given coordinates into the centroid formula and solve for k. By substituting the coordinates (3, 3) and (2, 3) into the formula, we get: 3 = (2 + (-2) + k)/3
Simplifying this equation, we get: 9 = 2 – 2 + k 9 = k
Therefore, the value of k is 9.
How Kunduz Can Help You Learn Centroid?
At Kunduz, we understand the importance of mastering geometry concepts like the centroid. We offer comprehensive resources, including tutorials, step-by-step explanations, and practice problems, to help you understand and apply the centroid formula and related concepts. Our expert instructors are always available to assist you in your learning journey and provide personalized guidance. With Kunduz, you can build a strong foundation in geometry and confidently solve problems involving centroids and other geometric principles.
