In the realm of geometry, angles play a pivotal role in defining the relationships between lines, shapes, and figures. One such relationship is that of complementary angles. Complementary angles are a fundamental concept in geometry, and understanding them is crucial to solving various mathematical problems. In this article, we will explore the definition of complementary angles, learn how to find them, examine their properties, and delve into some interesting facts and examples. So let’s dive in and unravel the fascinating world of complementary angles!
What are Complementary Angles?
Complementary angles are a pair of angles that add up to exactly 90 degrees. When two angles are complementary, they are said to complement each other, as their sum completes a right angle. In other words, if the sum of two angles is 90°, then those angles are considered complementary angles.
Angles can be formed by two rays meeting at a common endpoint, known as a vertex. The space between these rays is what we refer to as an angle. Complementary angles can be part of the same figure, such as a triangle or quadrilateral, or they can be separate angles.
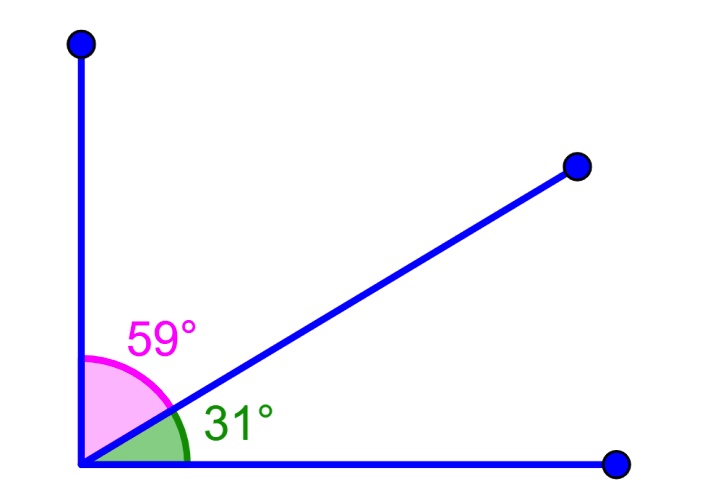
How To Find Complementary Angles?
To find complementary angles, you need to determine the measure of one angle and then subtract it from 90 degrees. The result will be the measure of its complement. Let’s take an example to illustrate this process:
Suppose we have an angle A with a measure of x degrees. The complement of angle A, which we’ll call angle B, can be found by subtracting the measure of angle A from 90 degrees. Therefore, angle B = 90 – x degrees.
It’s important to note that for two angles to be complementary, they must both be acute angles. An acute angle is an angle that measures less than 90 degrees. If one or both of the angles are obtuse (greater than 90 degrees) or right angles (exactly 90 degrees), they cannot be complementary angles.
How To Find the Measure of Complementary Angles?
To find the measure of complementary angles, you need to know the measure of one of the angles. Let’s say we have angle A and we know its measure is 40 degrees. The complement of angle A, which we’ll call angle B, can be found by subtracting the measure of angle A from 90 degrees. Therefore, angle B = 90 – 40 = 50 degrees.
This means that if angle A measures 40 degrees, then angle B, its complement, measures 50 degrees.
Adjacent Complementary Angles
Adjacent complementary angles are a special type of complementary angles. They are two complementary angles that share a common vertex and a common side. In other words, they are side by side and form a linear pair. The sum of measures of adjacent complementary angles is always 180 degrees.
For example, let’s consider two adjacent complementary angles, angle X and angle Y. If angle X measures 30 degrees, then angle Y, its complement, measures 60 degrees. Together, they form a linear pair and their sum is 90 degrees.
Properties of Complementary Angles
Complementary angles exhibit several interesting properties that are worth exploring. Let’s take a look at some of these properties:
- Sum of Measures: The sum of the measures of two complementary angles is always equal to 90 degrees. This property defines the essence of complementary angles.
- Acute Angles: Both angles in a pair of complementary angles are acute angles. An acute angle is an angle that measures less than 90 degrees.
- Non-Adjacent Complementary Angles: Complementary angles can be non-adjacent as well. They do not have to share a common vertex or side.
- Unique Complement: Each angle has a unique complement. If angle A is the complement of angle B, then angle B is the complement of angle A.
Types of Complementary Angles
Complementary angles can be classified into two types: adjacent complementary angles and non-adjacent complementary angles.
Adjacent Complementary Angles
Adjacent complementary angles are two angles that share a common vertex and a common side. They are side by side and form a linear pair. The sum of their measures is always 180 degrees.
For example, let’s consider angle P and angle Q. If angle P measures 40 degrees, then angle Q, its complement, measures 50 degrees. Together, they form a linear pair and their sum is 90 degrees.
Non-Adjacent Complementary Angles
Non-adjacent complementary angles are two angles that do not share a common vertex or side. They can be located in different figures or even in different parts of the same figure. The sum of their measures is always 90 degrees.
For example, let’s consider angle X and angle Y. If angle X measures 30 degrees, then angle Y, its complement, measures 60 degrees. Even though they are not adjacent, their sum is 90 degrees, making them complementary angles.
Complementary Angles Theorem and Proof
The complementary angles theorem states that if two angles are complementary to the same angle, then they are congruent to each other. In other words, if angle A and angle B are both complementary to angle C, then angle A is congruent to angle B.
To prove this theorem, let’s consider angle P and angle Q, which are both complementary to angle R. We need to show that angle P is congruent to angle Q.
By the definition of complementary angles, we know that angle P + angle R = 90 degrees and angle Q + angle R = 90 degrees.
Now, let’s subtract angle R from both equations:
angle P + angle R – angle R = 90 degrees – angle R angle P = 90 degrees – angle R
angle Q + angle R – angle R = 90 degrees – angle R angle Q = 90 degrees – angle R
From the two equations, we can see that angle P = angle Q, which means that angle P is congruent to angle Q. Thus, the complementary angles theorem is proven.
Facts About Complementary Angles
Here are some interesting facts about complementary angles:
- Complementary angles are always acute angles. They can never be obtuse or right angles.
- The sum of the measures of complementary angles is always equal to 90 degrees.
- Two right angles can never be complementary angles, as their sum is 180 degrees, not 90 degrees.
- Complementary angles are often used in trigonometry to calculate different ratios such as sine, cosine, and tangent.
- Complementary angles can be found in various geometric shapes and figures, such as triangles, quadrilaterals, and polygons.
- Complementary angles are not limited to geometric shapes; they can also be found in real-life situations. For example, when the sun is at an angle of 30 degrees above the horizon, the complement of that angle would be 60 degrees, representing the angle between the horizon and the ground.
Complementary Angles vs. Supplementary Angles
Complementary angles and supplementary angles are two important concepts in geometry. While complementary angles add up to 90 degrees, supplementary angles have a sum of 180 degrees.
The main difference between complementary angles and supplementary angles is the measure of their sum. Complementary angles add up to a right angle, which is 90 degrees, while supplementary angles add up to a straight angle, which is 180 degrees.
For example, let’s consider angle A and angle B. If angle A measures 40 degrees, then its complement, angle B, measures 50 degrees. Together, they form a pair of complementary angles. On the other hand, if angle C measures 120 degrees, then its supplement, angle D, measures 60 degrees. Together, they form a pair of supplementary angles.
It’s important to note that complementary angles cannot be supplementary angles at the same time, as their sum would exceed 180 degrees. Similarly, supplementary angles cannot be complementary angles, as their sum would be less than 180 degrees.
To summarize, complementary angles add up to 90 degrees, while supplementary angles add up to 180 degrees.
(272).jpg)
Frequently Asked Questions About Complementary Angles
Let’s address some common questions about complementary angles:
What do Complementary Angles Add up to?
Complementary angles always add up to 90 degrees. It is a defining property of complementary angles that their sum is equal to a right angle.
What is a Pair of Complementary Angles?
A pair of complementary angles refers to two angles whose sum is 90 degrees. When two angles are complementary, they are said to complement each other.
What is the Sum of Two Complementary Angles?
The sum of two complementary angles is always equal to 90 degrees. Complementary angles are defined by their sum being a right angle.
Can Two Right Angles be Complementary Angles?
No, two right angles cannot be complementary angles. Complementary angles have a sum of 90 degrees, while two right angles have a sum of 180 degrees.
Are Complementary Angles Congruent?
Complementary angles are not necessarily congruent. Congruent angles have the same measure, while complementary angles have a sum of 90 degrees. However, if two angles are complementary to the same angle, then they are congruent to each other.
Solved Examples on Complementary Angles
Let’s solve some example problems involving complementary angles:
Example 1: Find the measures of two complementary angles if one angle is 40 degrees.
Solution: If one angle is 40 degrees, the other angle, its complement, can be found by subtracting 40 from 90. Therefore, the measure of the other angle is 90 – 40 = 50 degrees. So, the two complementary angles are 40 degrees and 50 degrees.
Example 2: If the measure of one angle is 60 degrees, what is the measure of its complement?
Solution: The complement of an angle can be found by subtracting its measure from 90 degrees. In this case, the complement of an angle measuring 60 degrees is 90 – 60 = 30 degrees.
Example 3: If two angles are complementary and one angle measures 75 degrees, what is the measure of the other angle?
Solution: The sum of two complementary angles is 90 degrees. If one angle measures 75 degrees, then the measure of the other angle can be found by subtracting 75 from 90. Therefore, the measure of the other angle is 90 – 75 = 15 degrees.
How Kunduz Can Help You Learn Complementary Angles?
Learning about complementary angles can be challenging, but Kunduz is here to assist you every step of the way. Kunduz is an online learning platform that provides comprehensive resources, including lessons, practice problems, and interactive quizzes, to help you master the concept of complementary angles.
Whether you’re a student studying geometry or a teacher looking for additional resources, Kunduz offers a wide range of educational materials tailored to your needs. With Kunduz, you can deepen your understanding of complementary angles and enhance your problem-solving skills in a fun and engaging manner.
So, if you’re ready to embark on a journey of mathematical discovery, join Kunduz today and unlock the potential of complementary angles!
