In the world of geometry, angles play a crucial role in understanding the relationships between lines and shapes. One important concept to grasp is that of consecutive angles. But what exactly are consecutive angles and how do they relate to parallel lines and transversals? In this article, we will explore the definition, types, properties, and theorems associated with consecutive angles. So, let’s dive in and unravel the mysteries of consecutive angles!
What are the Consecutive Angles?
Consecutive angles, as the name suggests, are angles that follow one another. These angles are formed when two lines are intersected by a third line, known as a transversal. The two lines being intersected can either be parallel or non-parallel. Consecutive angles can be further categorized into two types: consecutive interior angles and consecutive exterior angles.
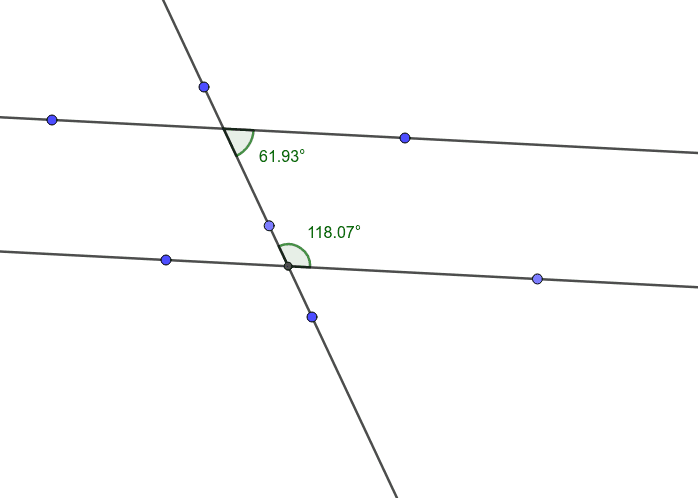
Consecutive Interior Angles
Consecutive interior angles are formed when a transversal intersects two parallel lines. These angles lie on the same side of the transversal and are found between the two parallel lines. In other words, consecutive interior angles are pairs of angles that are inside the parallel lines and on the same side of the transversal. They share a common vertex and one side.
Consecutive Exterior Angles
Consecutive exterior angles, on the other hand, are formed when a transversal intersects two non-parallel lines. These angles lie on the same side of the transversal and are outside the two non-parallel lines. Like consecutive interior angles, consecutive exterior angles also share a common vertex and one side.
How To Find Consecutive Angles?
To identify consecutive angles, you need to locate the transversal and the lines being intersected. Once you have identified the transversal and the lines, look for angles that satisfy the criteria mentioned earlier. For consecutive interior angles, check if the angles are inside the parallel lines and on the same side of the transversal, sharing a common vertex and one side. For consecutive exterior angles, check if the angles are outside the non-parallel lines, on the same side of the transversal, sharing a common vertex and one side.
Let’s take a look at an example to better understand how to find consecutive angles:
Example: Consider two parallel lines, line A and line B, intersected by a transversal line. We will label the angles formed as angle 1, angle 2, angle 3, and angle 4. To find the consecutive interior angles, we need to identify pairs of angles that satisfy the criteria. In this case, angle 1 and angle 3 are consecutive interior angles, as well as angle 2 and angle 4.
How To Find the Measure of Consecutive Angles?
Finding the measure of consecutive angles requires a good understanding of the properties and theorems associated with these angles. Let’s explore some key concepts and strategies for finding the measure of consecutive angles.
Supplementary Angles
One important property of consecutive interior angles is that they are supplementary angles. This means that the sum of the measures of two consecutive interior angles is equal to 180 degrees. In other words, if angle A and angle B are consecutive interior angles, then the measure of angle A + the measure of angle B = 180 degrees.
For example, if angle A measures 60 degrees, then angle B must measure 120 degrees to make the sum of the measures equal to 180 degrees.
Complementary Angles
Consecutive exterior angles, on the other hand, are complementary. This means that the sum of the measures of two consecutive exterior angles is equal to 90 degrees. In other words, if angle C and angle D are consecutive exterior angles, then the measure of angle C + the measure of angle D = 90 degrees.
For example, if angle C measures 30 degrees, then angle D must measure 60 degrees to make the sum of the measures equal to 90 degrees.
Using Known Angles
Sometimes, finding the measure of consecutive angles can be as simple as using the measures of known angles. If you are given the measure of one angle in a pair of consecutive angles, you can easily find the measure of the other angle by subtracting the known measure from 180 degrees (for consecutive interior angles) or 90 degrees (for consecutive exterior angles).
Let’s take a look at an example to illustrate this concept:
Example: Consider a pair of consecutive interior angles, angle X and angle Y. If angle X measures 80 degrees, we can find the measure of angle Y by subtracting 80 degrees from 180 degrees. The measure of angle Y would be 180 – 80 = 100 degrees.
Converse of Consecutive Angles Theorem
The converse of the consecutive angles theorem is another important concept to understand. The converse states that if a pair of consecutive interior angles is supplementary, then the lines intersected by the transversal must be parallel.
In other words, if the sum of the measures of two consecutive interior angles is equal to 180 degrees, then the lines being intersected by the transversal must be parallel. This converse theorem provides a way to determine whether lines are parallel based on the measures of consecutive interior angles.
Let’s take a closer look at the proof of the consecutive angles theorem and its converse:
Proof of Consecutive Interior Angles Theorem
To prove the consecutive interior angles theorem, we start with two parallel lines, line A and line B, intersected by a transversal line. We label the angles formed as angle 1, angle 2, angle 3, and angle 4. The consecutive interior angles are angle 1 and angle 3, as well as angle 2 and angle 4.
To prove that the consecutive interior angles are supplementary, we need to show that the sum of the measures of angle 1 and angle 3 is equal to 180 degrees, and the sum of the measures of angle 2 and angle 4 is equal to 180 degrees.
Proof of Converse of Consecutive Interior Angles Theorem
The converse of the consecutive interior angles theorem states that if a pair of consecutive interior angles is supplementary, then the lines being intersected by the transversal must be parallel.
To prove the converse, we start with a pair of consecutive interior angles, angle A and angle B, which are supplementary (their measures add up to 180 degrees). We need to show that the lines intersected by the transversal must be parallel.
By assuming that the lines are not parallel, we can use the properties of angles and parallel lines to arrive at a contradiction. This contradiction leads us to the conclusion that the lines must indeed be parallel.
Consecutive Angles in a Parallelogram
In a parallelogram, consecutive angles play a significant role in understanding the properties and characteristics of this special quadrilateral. A parallelogram is a quadrilateral with opposite sides that are parallel and equal in length.
In a parallelogram, each pair of adjacent angles represents consecutive angles. These pairs of angles can be identified as follows:
- Angle A and angle B are consecutive angles.
- Angle B and angle C are consecutive angles.
- Angle C and angle D are consecutive angles.
- Angle D and angle A are consecutive angles.
To further understand the relationship between consecutive angles in a parallelogram, let’s explore their properties.

Properties of Consecutive Angles
Consecutive angles have several properties that are worth noting. These properties can help us identify and analyze the relationships between angles in various geometric configurations. Let’s take a look at some key properties of consecutive angles:
Supplementary Property
One important property of consecutive interior angles is that they are supplementary. This means that the sum of the measures of two consecutive interior angles is equal to 180 degrees. In a parallelogram, this property holds true for each pair of consecutive interior angles.
For example, if angle A measures 60 degrees, then angle B, which is consecutive to angle A, must measure 120 degrees to make the sum of the measures equal to 180 degrees.
Opposite Angles Property
In a parallelogram, opposite angles are congruent. This means that the measures of the opposite angles are equal. Since consecutive angles are adjacent to each other, they are not opposite angles. Therefore, the opposite angles property does not apply to consecutive angles in a parallelogram.
Adjacent Angles Property
Consecutive angles are adjacent to each other. This means that they share a common side and a common vertex. Adjacent angles can have different measures and are not necessarily equal. In a parallelogram, the adjacent angles property applies to each pair of consecutive angles.
Complementary Property
In a parallelogram, consecutive exterior angles are complementary. This means that the sum of the measures of two consecutive exterior angles is equal to 90 degrees.
For example, if angle C measures 30 degrees, then angle D, which is consecutive to angle C, must measure 60 degrees to make the sum of the measures equal to 90 degrees.
Now that we have explored the properties of consecutive angles in a parallelogram, let’s move on to the theorems associated with these angles.
Consecutive Angles Theorem and Proof
The consecutive angles theorem is an important result in geometry that helps us understand the relationships between consecutive angles in various geometric configurations. The theorem states that if two parallel lines are intersected by a transversal, then the pairs of consecutive interior angles formed are supplementary.
To prove the consecutive angles theorem, we start with two parallel lines, line A and line B, intersected by a transversal line. We label the angles formed as angle 1, angle 2, angle 3, and angle 4. The consecutive interior angles are angle 1 and angle 3, as well as angle 2 and angle 4.
To prove that the consecutive interior angles are supplementary, we need to show that the sum of the measures of angle 1 and angle 3 is equal to 180 degrees, and the sum of the measures of angle 2 and angle 4 is equal to 180 degrees.
Let’s go through the proof of the consecutive angles theorem step by step:
Step 1: Given two parallel lines, line A and line B, and a transversal line that intersects them.
Step 2: Label the angles formed by the intersection as angle 1, angle 2, angle 3, and angle 4.
Step 3: Angle 1 and angle 3 are consecutive interior angles, as well as angle 2 and angle 4.
Step 4: To prove that angle 1 and angle 3 are supplementary, we need to show that the sum of their measures is equal to 180 degrees.
Step 5: Since line A and line B are parallel, we can use the properties of parallel lines and transversals to establish the relationship between the consecutive interior angles.
Step 6: By the corresponding angles property, we can conclude that angle 1 is equal to angle 3.
Step 7: Therefore, the sum of the measures of angle 1 and angle 3 is equal to 2 times the measure of angle 1, which is equal to 180 degrees.
Step 8: Similarly, we can prove that angle 2 and angle 4 are supplementary, with the sum of their measures equal to 180 degrees.
Step 9: Hence, we have proven the consecutive angles theorem, which states that if two parallel lines are intersected by a transversal, then the pairs of consecutive interior angles formed are supplementary.
Now that we have explored the consecutive angles theorem and its proof, let’s move on to some interesting facts about consecutive angles.
Facts About Consecutive Angles
Consecutive angles have several interesting facts and properties that help us understand their behavior and relationships. Let’s take a look at some intriguing facts about consecutive angles:
- Consecutive interior angles are supplementary: In any geometric configuration where two parallel lines are intersected by a transversal, the pairs of consecutive interior angles formed are always supplementary. This means that their measures add up to 180 degrees.
- Consecutive exterior angles are supplementary: In any geometric configuration where two non-parallel lines are intersected by a transversal, the pairs of consecutive exterior angles formed are always supplementary. This means that their measures add up to 180 degrees.
- Consecutive angles in a parallelogram are supplementary: In a parallelogram, each pair of consecutive angles is supplementary. This means that the sum of the measures of two consecutive angles in a parallelogram is always 180 degrees.
- Consecutive angles can be found in various geometric shapes: While consecutive angles are commonly associated with parallel lines and transversals, they can also be found in other geometric shapes, such as triangles and quadrilaterals. In these cases, consecutive angles can provide insights into the properties and characteristics of the shapes.
- Consecutive angles play a crucial role in proving theorems: Consecutive angles are often used in the proofs of various theorems in geometry. Their properties and relationships help establish the validity of these theorems and provide a deeper understanding of geometric concepts.
Now that we have explored some interesting facts about consecutive angles, let’s address some frequently asked questions to clarify any remaining doubts.
Frequently Asked Questions About Consecutive Angles
When are Two Angles Consecutive?
Two angles are considered consecutive when they satisfy the following criteria:
- They share a common vertex.
- They have a common side.
- They are either both inside or both outside a pair of parallel lines intersected by a transversal.
If these conditions are met, the angles are considered consecutive.
Do Consecutive Angles Equal Each Other?
Consecutive angles do not necessarily equal each other. While they share a common vertex and one side, their measures can be different. However, in certain cases, such as in a parallelogram, consecutive angles can be congruent.
Do consecutive interior angles add up to 180?
Yes, consecutive interior angles add up to 180 degrees. This is a property specific to consecutive interior angles formed by a transversal intersecting two parallel lines.
Are consecutive interior angles congruent?
Consecutive interior angles are not always congruent. While they are supplementary, meaning their measures add up to 180 degrees, their individual measures can be different.
Solved Examples on How to Use the Consecutive Angles
Now that we have explored the properties and theorems associated with consecutive angles, let’s put our knowledge into practice with some solved examples.
Example 1: Consider two parallel lines, line A and line B, intersected by a transversal line. Angle 1 measures 40 degrees. What is the measure of angle 3, which is a consecutive interior angle to angle 1?
Solution: Since angle 1 and angle 3 are consecutive interior angles, their measures add up to 180 degrees. We know that angle 1 measures 40 degrees. To find the measure of angle 3, we subtract 40 degrees from 180 degrees: Measure of angle 3 = 180 degrees – 40 degrees = 140 degrees.
Therefore, the measure of angle 3 is 140 degrees.
Example 2: Consider two non-parallel lines intersected by a transversal line. Angle X measures 50 degrees. What is the measure of angle Y, which is a consecutive exterior angle to angle X?
Solution: Since angle X and angle Y are consecutive exterior angles, their measures add up to 180 degrees. We know that angle X measures 50 degrees. To find the measure of angle Y, we subtract 50 degrees from 180 degrees: Measure of angle Y = 180 degrees – 50 degrees = 130 degrees.
Therefore, the measure of angle Y is 130 degrees.
How Kunduz Can Help You Learn Consecutive Angles?
At Kunduz, we understand the importance of mastering mathematical concepts like consecutive angles. Our comprehensive and interactive online learning platform provides students with the tools they need to excel in mathematics. With a wide range of engaging lessons, practice exercises, and personalized feedback, Kunduz ensures that students develop a deep understanding of consecutive angles and other geometric concepts.
Our experienced and dedicated instructors are here to guide students through their mathematical journey, offering support and assistance every step of the way. Whether it’s understanding the properties of consecutive angles or solving complex problems involving transversals and parallel lines, Kunduz is here to help.
Don’t let consecutive angles be a challenge. Join Kunduz today and embark on a rewarding mathematical adventure!
In conclusion, consecutive angles are an essential aspect of geometry, providing insights into the relationships between lines and shapes. Understanding the properties, theorems, and applications of consecutive angles can help students navigate the intricate world of geometry with confidence and ease. So, embrace the world of consecutive angles and embark on a journey of geometric discovery!
