The distributive property is a fundamental concept in mathematics that allows us to simplify and solve expressions involving multiplication and addition or subtraction. It is a powerful tool that helps us break down complex problems into simpler parts. In this article, we will explore the definition, formula, and examples of the distributive property, as well as its applications in various mathematical operations.
An Introduction to Distributive Property
In mathematics, the distributive property is a rule that explains how to multiply a single value by two or more values within a set of parentheses. It states that when a factor is multiplied by the sum or difference of two terms, it is essential to distribute the multiplication to each term individually and then perform the addition or subtraction operation. This property is extensively used in arithmetic calculations and algebraic expressions.
The distributive property allows us to simplify expressions by breaking them down into smaller, more manageable parts. By distributing the multiplication operation, we can perform calculations on each term individually, which makes solving complex problems much easier.
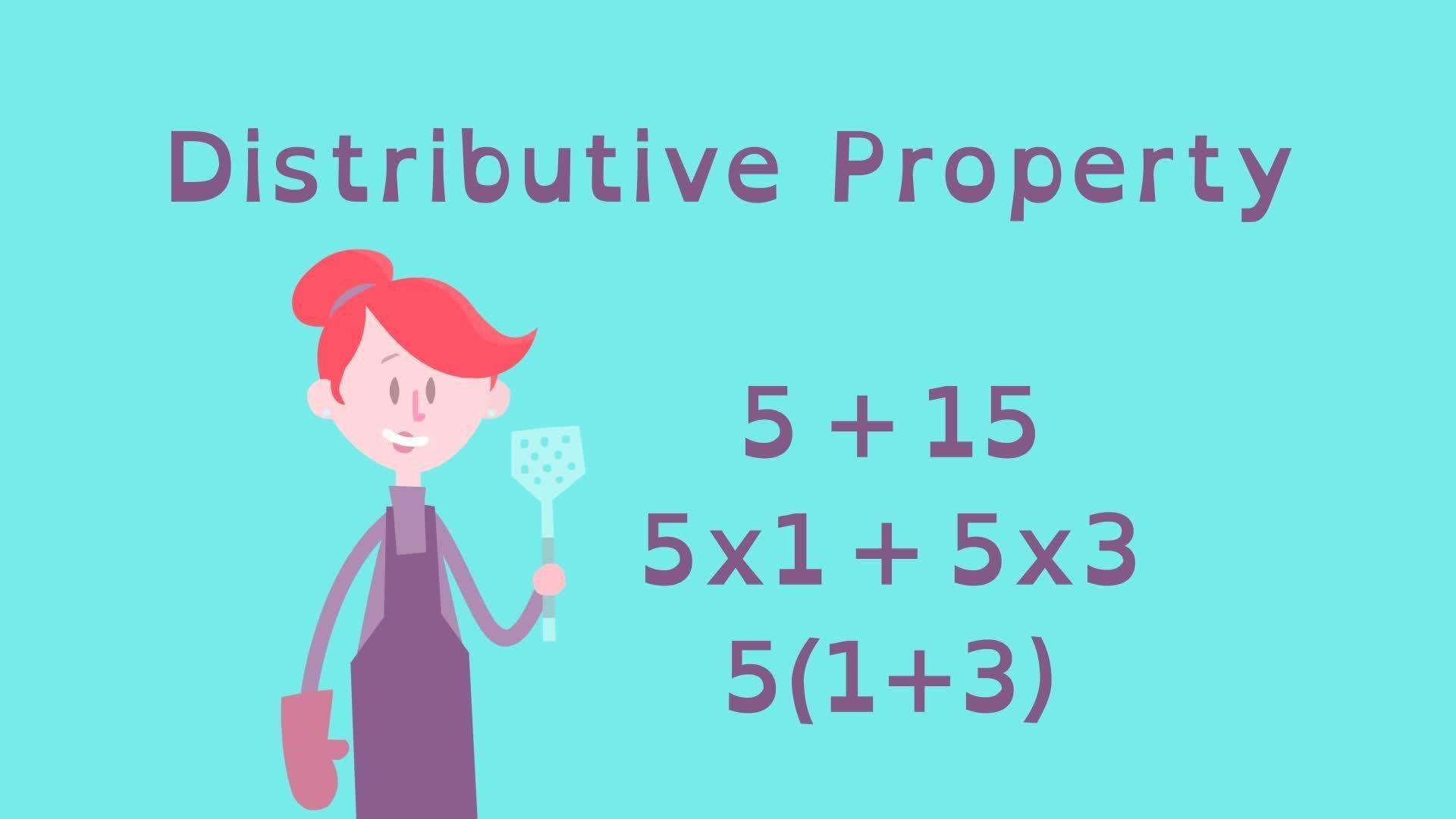
What is Distributive Property?
The distributive property states that for any real numbers A, B, and C, the product of A and the sum or difference of B and C is equal to the sum or difference of the products of A and B and A and C, respectively. Mathematically, it can be represented as:
A × (B + C) = AB + AC
This formula applies to addition. Similarly, the distributive property also applies to subtraction:
A × (B – C) = AB – AC
In both cases, the factor A is distributed to each term within the parentheses, and then the resulting products are added or subtracted to obtain the final result.

Distributive Property Formula
The distributive property formula can be stated as follows:
A × (B + C) = AB + AC
This formula illustrates the distributive property of multiplication over addition. It tells us that when we have a factor A multiplied by the sum of two terms B and C, we can distribute A to each term individually and then add the products together.
Similarly, the formula for the distributive property of multiplication over subtraction is:
A × (B – C) = AB – AC
In this case, the factor A is distributed to each term, and then the products are subtracted to obtain the final result.
Distributive Property of Multiplication Over Addition
The distributive property of multiplication over addition is used when we need to multiply a number by the sum of two or more addends. It allows us to distribute the multiplication to each addend individually and then add the resulting products.
Let’s consider an example to understand how the distributive property works in this case:
Example 1: Solve the expression 5(10 + 3) using the distributive property of multiplication over addition.
Solution: According to the distributive property, we can distribute the multiplication operation to each addend:
5(10 + 3) = 5 × 10 + 5 × 3
Now, we can perform the multiplication and addition:
= 50 + 15
= 65
Therefore, the value of 5(10 + 3) is 65.
In this example, we broke down the expression into two separate multiplications and then added the products together. This demonstrates the distributive property in action.
Distributive Property of Multiplication Over Subtraction
The distributive property of multiplication over subtraction is similar to the distributive property of multiplication over addition. It allows us to distribute the multiplication to each term individually and then subtract the resulting products.
Let’s consider an example to understand how the distributive property works in this case:
Example 2: Solve the expression 6(13 – 5) using the distributive property of multiplication over subtraction.
Solution: Using the distributive property, we can distribute the multiplication to each term:
6(13 – 5) = 6 × 13 – 6 × 5
Now, we can perform the multiplication and subtraction:
= 78 – 30
= 48
Therefore, the value of 6(13 – 5) is 48.
In this example, we distributed the multiplication to each term within the parentheses and then performed the subtraction. This showcases the distributive property of multiplication over subtraction.
Verification of Distributive Property
The distributive property can be verified by solving an expression using both the distributive property and the standard order of operations. If the results obtained from both methods are the same, then the distributive property is confirmed.
Let’s consider an example to verify the distributive property:
Example 3: Verify the distributive property by solving the expression 3(4 + 2) using both the distributive property and the order of operations.
Solution: According to the distributive property, we can distribute the multiplication to each term:
3(4 + 2) = 3 × 4 + 3 × 2
Now, we can perform the multiplication and addition:
= 12 + 6
= 18
Using the order of operations, we would first perform the addition within the parentheses:
3(4 + 2) = 3 × 6
Then, perform the multiplication:
= 18
As we can see, both methods yield the same result of 18. This verifies the distributive property in this example.
Distributive Property of Division
The distributive property can also be applied to division, although it is not as commonly used as the distributive property of multiplication. When dividing a larger number by a smaller number, we can break down the larger number into smaller factors and distribute the division operation to each factor.
Let’s consider an example to understand how the distributive property works in division:
Example 4: Divide 36 by 6 using the distributive property of division.
Solution: We can break down 36 into smaller factors:
36 = 30 + 6
Now, we can distribute the division operation to each factor:
(30 + 6) ÷ 6 = 30 ÷ 6 + 6 ÷ 6
Simplifying further:
= 5 + 1
= 6
Therefore, the result of dividing 36 by 6 using the distributive property is 6.
In this example, we broke down the dividend 36 into two smaller numbers, 30 and 6, and distributed the division operation to each number. This showcases the distributive property of division.
Distributive Property of Equality
The distributive property can also be applied to equations involving equality. It allows us to multiply both sides of an equation by a common factor without changing the equality.
Let’s consider an example to understand how the distributive property works in equations:
Example 5: Solve the equation 2(x + 3) = 10 using the distributive property.
Solution: According to the distributive property, we can distribute the multiplication to each term:
2(x + 3) = 2x + 6
Now, we can rewrite the equation:
2x + 6 = 10
Subtracting 6 from both sides of the equation:
2x = 4
Dividing both sides by 2:
x = 2
Therefore, the solution to the equation 2(x + 3) = 10 is x = 2.
In this example, we applied the distributive property to multiply both sides of the equation by 2 and simplify the expression. This allowed us to solve for the variable x.
Distributive Property with Fractions
The distributive property can also be applied to fractions, allowing us to distribute a factor to each term within the parentheses.
Let’s consider an example to understand how the distributive property works with fractions:
Example 6: Solve the expression 2(1/2 + 1/4) using the distributive property.
Solution: According to the distributive property, we can distribute the multiplication to each term:
2(1/2 + 1/4) = 2 × 1/2 + 2 × 1/4
Now, we can perform the multiplication and addition:
= 1 + 1/2
To add the fractions, we need a common denominator. In this case, the common denominator is 2:
= 2/2 + 1/2
= 3/2
Therefore, the value of 2(1/2 + 1/4) is 3/2.
In this example, we distributed the multiplication operation to each term within the parentheses and then added the fractions. This demonstrates the distributive property with fractions.
To be expert at fractions topic, you can also check our division of fractions and substracting fractions blog pages.
Distributive Property with Variables
The distributive property can also be applied to expressions containing variables. It allows us to distribute a factor to each term within the parentheses, making it easier to simplify and solve algebraic expressions.
Let’s consider an example to understand how the distributive property works with variables:
Example 7: Simplify the expression 3(x + 2y) using the distributive property.
Solution: According to the distributive property, we can distribute the multiplication to each term:
3(x + 2y) = 3x + 3(2y)
Now, we can simplify each term individually:
= 3x + 6y
Therefore, the simplified expression for 3(x + 2y) is 3x + 6y.
In this example, we distributed the multiplication to each term within the parentheses and simplified the expression by combining like terms. This showcases the distributive property with variables.
How to Use the Distributive Property
Using the distributive property involves following a few simple steps:
- Identify an expression in the form of A × (B + C) or A × (B – C), where A, B, and C are numbers or variables.
- Distribute the multiplication operation to each term within the parentheses, multiplying A with both B and C.
- Simplify each term individually by performing any necessary multiplication or addition/subtraction.
- Combine like terms, if applicable, to obtain the final result.
By following these steps, you can effectively use the distributive property to simplify and solve various mathematical expressions.
Solved Examples on Distributive Property
Let’s solve a few examples using the distributive property to further illustrate its application:
Example 8: Solve the expression 4(3 + 2) using the distributive property.
Solution: According to the distributive property, we can distribute the multiplication to each term:
4(3 + 2) = 4 × 3 + 4 × 2
Simplifying further:
= 12 + 8
= 20
Therefore, the value of 4(3 + 2) is 20.
Example 9: Simplify the expression 2(5x – 3y) using the distributive property.
Solution: According to the distributive property, we can distribute the multiplication to each term:
2(5x – 3y) = 2 × 5x – 2 × 3y
Simplifying further:
= 10x – 6y
Therefore, the simplified expression for 2(5x – 3y) is 10x – 6y.
Example 10: Solve the equation 3(x – 4) = 9 using the distributive property.
Solution: According to the distributive property, we can distribute the multiplication to each term:
3(x – 4) = 3 × x – 3 × 4
Simplifying further:
= 3x – 12
Now, we can rewrite the equation:
3x – 12 = 9
Adding 12 to both sides of the equation:
3x = 21
Dividing both sides by 3:
x = 7
Therefore, the solution to the equation 3(x – 4) = 9 is x = 7.
In these examples, we applied the distributive property to simplify expressions and solve equations. By distributing the multiplication operation, we were able to break down complex expressions into simpler terms and find their solutions.
How Kunduz Can Help You Learn Distributive Property?
Kunduz is your ultimate guide to learning and mastering the distributive property. Our platform offers comprehensive lessons, practice exercises, and interactive resources to help you understand and apply this important concept in mathematics.
Whether you’re a student seeking to improve your skills or a teacher looking for effective teaching materials, Kunduz has you covered. Our expert tutors provide step-by-step explanations, real-world examples, and personalized guidance to ensure your success.
With Kunduz, you can access high-quality educational content anytime, anywhere. Our user-friendly interface and engaging learning materials make studying the distributive property a breeze. Join Kunduz today and unlock your full potential in mathematics!
