When it comes to mathematics, fractions can sometimes be a challenge. Understanding how to divide fractions is a crucial skill that can be applied in various real-life situations. Whether you’re working with whole numbers, mixed numbers, or decimals, the process of dividing fractions follows a set of simple rules. In this article, we will explore the steps involved in dividing fractions and provide examples to help you grasp the concept. So let’s dive in and learn how to divide fractions!
What is the Division of Fractions?
The division of fractions involves splitting a fraction into smaller parts. When we divide fractions, we are essentially trying to determine how many equal parts of one fraction can fit into another fraction. The result can be a fraction or a whole number, depending on the values of the fractions being divided.
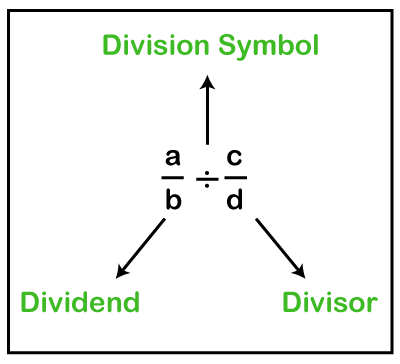
How To Divide Fractions?
Dividing fractions may seem daunting at first, but it’s actually quite straightforward. The key is to remember the three-step process: Keep, Change, and Flip. Let’s break down each step in detail.
Steps to Divide Fractions
Fraction by Fraction
To divide one fraction by another fraction, we follow these steps:
Step 1: Keep – Keep the first fraction as it is.
Step 2: Change – Change the division sign to a multiplication sign.
Step 3: Flip – Flip the second fraction, also known as finding its reciprocal, by interchanging the numerator and denominator.
Once we’ve completed these steps, we can multiply the two fractions together to obtain the result. Remember to simplify the fraction if necessary.
Fraction by Whole Number
When dividing a fraction by a whole number, the process is slightly different. Follow these steps:
Step 1: Keep – Keep the fraction as it is.
Step 2: Change – Change the division sign to a multiplication sign.
Step 3: Flip – Convert the whole number into a fraction by putting it over 1. Then flip this fraction to find its reciprocal.
After completing these steps, multiply the two fractions together and simplify the result if needed.
Dividing Fractions with Decimals
Dividing fractions with decimals follows the same rules as dividing fractions with whole numbers. Convert the decimal into a fraction and proceed with the division process as usual.
Division of Fractions and Mixed Numbers
When dividing fractions with mixed numbers, the first step is to convert the mixed number into an improper fraction. Then follow the same steps as dividing fractions by fractions to obtain the result. Simplify the fraction if necessary.
Properties of Dividing Fractions
Just like with whole numbers, there are several properties of division that apply to fractions as well. Here are a few key properties to keep in mind:
Property 1: Dividing a fraction by 1 – When you divide a fraction by 1, the result is the fraction itself.
Property 2: Dividing 0 by a non-zero fraction – Dividing 0 by a non-zero fraction always results in 0.
Property 3: Dividing a non-zero fraction by itself – When you divide a non-zero fraction by itself, the result is always 1.
Property 4: Division by 0 – Division by 0 is undefined and cannot be performed.
Understanding these properties can help you navigate the division of fractions more effectively.
How to Add or Subtract Fractions?
Performing addition or subtraction with fractions follows a different set of rules. To add or subtract fractions, you need to ensure that the fractions have the same denominator. If the denominators are different, you will need to find a common denominator before proceeding with the operation. Once you have the same denominator, you can add or subtract the numerators and keep the denominator unchanged. Finally, simplify the resulting fraction if necessary.
Least Common Denominator
Finding the least common denominator (LCD) is crucial when working with fractions. The LCD is the smallest common multiple of the denominators of two or more fractions. When adding or subtracting fractions with different denominators, you need to find the LCD and convert the fractions to have the same denominator before performing the operation.
Frequently Asked Questions About Division of Fractions
Now that we’ve covered the basics of dividing fractions, let’s address some common questions that often arise.
Q: How does one divide a fraction by a whole number?
To divide a fraction by a whole number, convert the whole number into a fraction by putting it over 1. Then follow the same steps as dividing fractions by fractions.
Q: How do you divide fractions by mixed numbers?
When dividing fractions by mixed numbers, convert the mixed number into an improper fraction and proceed with the division process as usual.
Q: How to Visualize Division of Fractions?
Visualizing the division of fractions can help in understanding the concept better. Imagine dividing a whole pizza into equal slices. The number of slices represents the denominator, and the number of slices you take represents the numerator.
Q: How To Divide Improper Fractions?
Dividing improper fractions follows the same steps as dividing proper fractions. Convert the improper fraction into a mixed number if necessary.
Q: How To Divide Negative Fractions?
Dividing negative fractions is similar to dividing positive fractions. The rules and steps remain the same.
Q: How To Divide Fractions With Exponents?
When dividing fractions with exponents, divide the numerators and denominators separately, then simplify the resulting fractions.
Q: How To Divide Fractions With Square Roots?
Dividing fractions with square roots is similar to dividing fractions without square roots. Apply the same steps and rules as you would for regular fractions.

Solved Examples on How to Use the Division of Fractions
Let’s work through a couple of examples to solidify our understanding of dividing fractions.
Example 1: Divide 2/3 by 3/7
To divide 2/3 by 3/7, we keep the first fraction as it is and change the division sign to multiplication. Then we flip the second fraction to find its reciprocal:
2/3 ÷ 3/7 = 2/3 * 7/3
Now we can multiply the numerators (2 * 7) and the denominators (3 * 3) to get the result:
2/3 * 7/3 = 14/9
Since the numerator is larger than the denominator, we convert the fraction to a mixed number:
14/9 = 1 5/9
So, 2/3 divided by 3/7 equals 1 5/9.
Example 2: Divide 4/5 by 2/6
To divide 4/5 by 2/6, we change the division sign to multiplication and find the reciprocal of the second fraction:
4/5 ÷ 2/6 = 4/5 * 6/2
Next, we multiply the numerators (4 * 6) and the denominators (5 * 2) to get the result:
4/5 * 6/2 = 24/10
Simplifying the fraction, we divide the numerator by the denominator (24/10 = 2 remainder 4) and write the answer as a mixed fraction:
24/10 = 2 4/10
Finally, we can further simplify the fraction by reducing it:
2 4/10 = 2 2/5
So, 4/5 divided by 2/6 equals 2 2/5.
How Kunduz Can Help You Learn Division of Fractions?
At Kunduz, we understand the challenges students face when it comes to learning and mastering mathematical concepts like dividing fractions. That’s why we offer a wide range of resources, including interactive lessons, practice problems, and step-by-step tutorials, to help you succeed in your math journey. Our experienced instructors are dedicated to providing clear and concise explanations, ensuring that you grasp the fundamentals and can confidently tackle any division of fractions problem. With Kunduz, you can learn at your own pace, access resources anytime, and receive personalized support to help you achieve academic excellence. Visit our website today and discover how Kunduz can make learning math enjoyable and rewarding for you.
In conclusion, dividing fractions may seem challenging at first, but with a clear understanding of the rules and steps involved, it becomes a manageable task. By following the “Keep, Change, and Flip” method, you can divide fractions with ease, whether they involve fractions, whole numbers, decimals, or mixed numbers. Remember to simplify the resulting fraction if needed and practice with various examples to solidify your understanding. With Kunduz as your learning companion, you can confidently tackle any division of fractions problem and excel in your mathematical journey.
